First principles grade 12: Practice

Kevinmathscience
19 May 202103:04
EducationalLearning
32 Likes 10 Comments

TLDRThe transcript details the process of calculating a derivative using the first principle formula. It emphasizes the importance of correct notation, especially for exam purposes. The demonstration involves substituting \( f(x) \) with \( x^2 + 3x \), expanding \( (x+h)^2 \), and simplifying the expression by canceling terms without \( h \). The final step involves factoring out \( h \), canceling it, and letting \( h \) approach zero to arrive at the derivative \( 2x + 3 \). The summary highlights the methodical approach to finding derivatives and the significance of proper mathematical expression.

Takeaways
  • 📝 Importance of using the first principle formula in calculus problems.
  • 📚 Strict adherence to correct notation is emphasized for exam purposes.
  • 🔍 Demonstration of how to write the limit notation for the first principle formula.
  • 📉 Explanation of substituting \( f(x) \) with \( x^2 + 3x \) in the formula.
  • 🔄 Step-by-step guide on factoring and simplifying the expression within the limit.
  • ✅ Confirmation that terms without an 'h' should cancel out, which is the expected outcome.
  • 🆚 Comparison of the expression before and after the cancellation to show simplification.
  • 📉 Further simplification by factoring out 'h' and then canceling it out.
  • 🎯 Final step involves letting 'h' approach zero to find the derivative.
  • 🏁 The final answer for the derivative is \( 2x + 3 \).
Q & A

  • What is the first principle formula mentioned in the script?

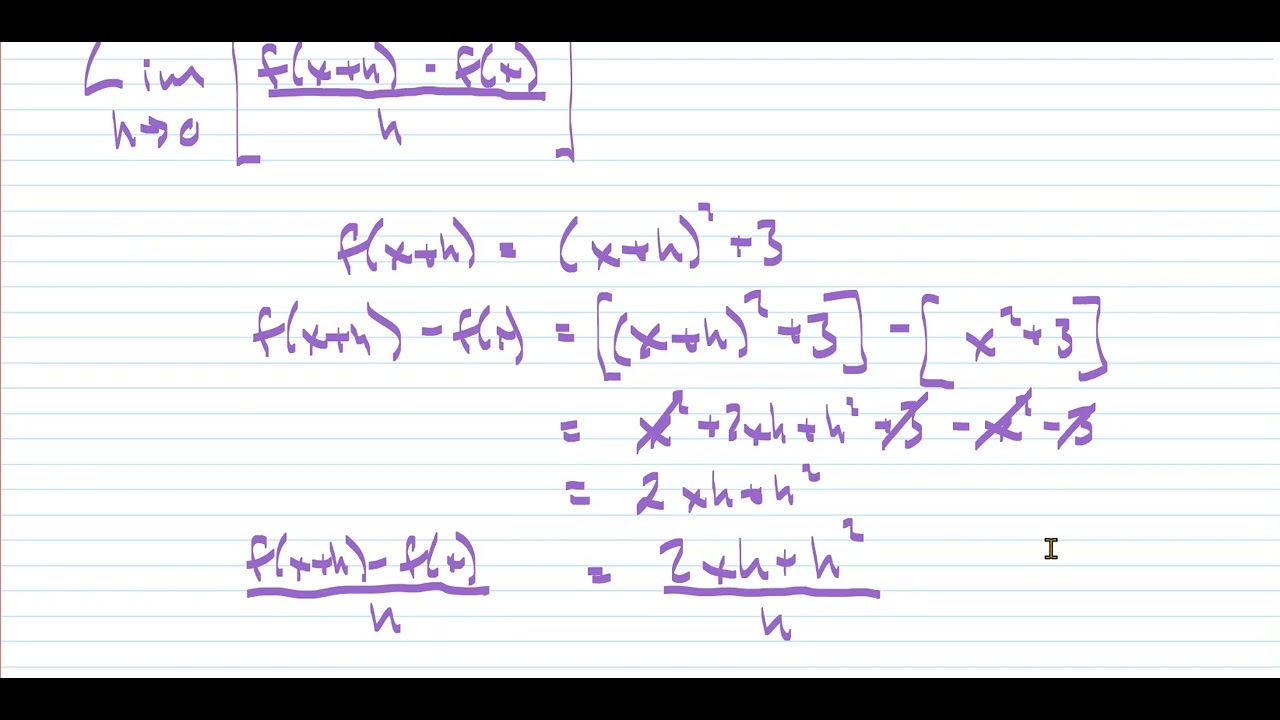
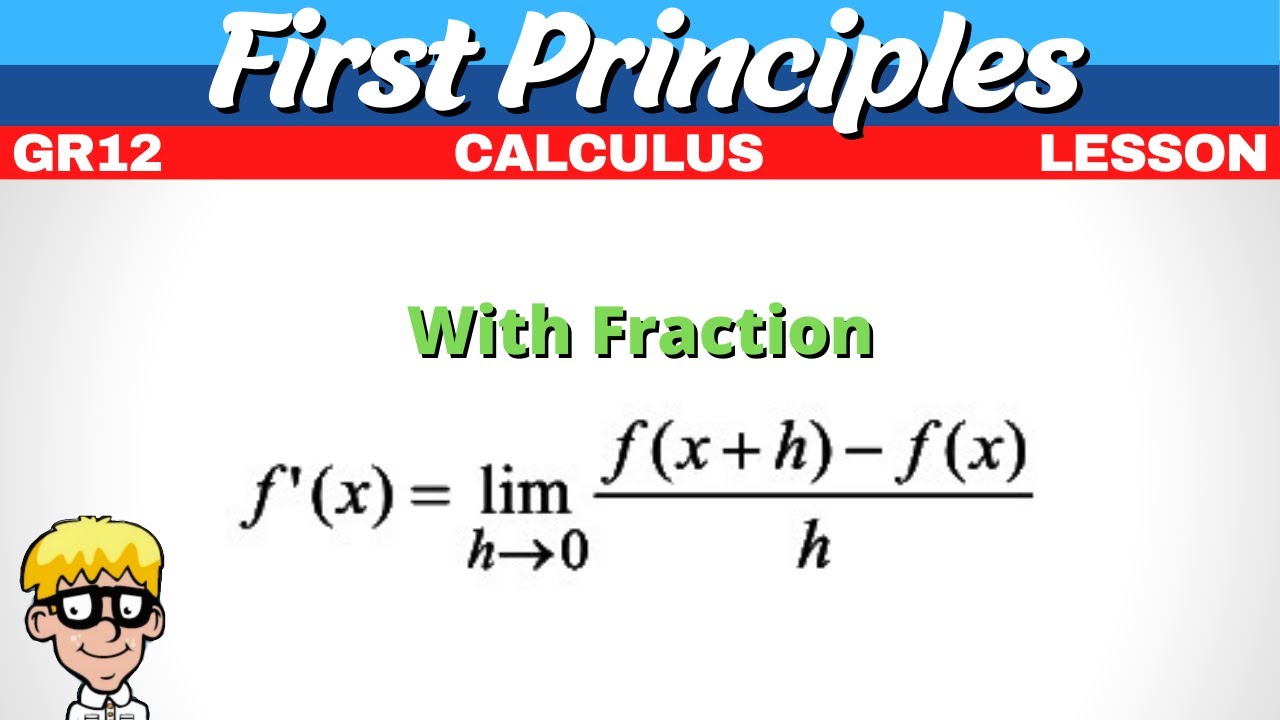
    -The first principle formula mentioned is the limit definition of the derivative, which is written as \( \lim_{h \to 0} \frac{f(x+h) - f(x)}{h} \).

  • Why is correct notation important when writing the first principle formula in exams?

    -Correct notation is important because exams are strict about it, and improper notation can lead to misunderstandings or incorrect grading.

  • What is the process of substituting x with x+h in the given function?

    -The process involves replacing every x in the function with x+h, resulting in the expression \( (x+h)^2 + 3(x+h) \).

  • What does the script suggest we should do with the function f(x) when calculating the derivative?

    -The script suggests writing f(x) as \( x^2 + 3x \) and then substituting it into the first principle formula.

  • How does the script simplify the expression after substituting f(x) into the first principle formula?

    -The script simplifies the expression by canceling out the terms that do not contain h, which are \( x^2 \) and \( 3x \), leaving \( 2xh + h^2 + 3h \) over h.

  • What happens when we factor out h from the remaining terms in the derivative formula?

    -Factoring out h from the remaining terms results in \( h(2x + h + 3) \) over h, which simplifies further by canceling out the h in the numerator and denominator.

  • Why can't we let h go to zero before factoring out h from the terms?

    -We can't let h go to zero before factoring because it would result in an undefined expression due to division by zero.

  • What is the final result of the derivative after letting h go to zero?

    -The final result of the derivative after letting h go to zero is \( 2x + 3 \).

  • Why is it important to get into the habit of writing the limit notation correctly?

    -Writing the limit notation correctly is important for clarity and to ensure that the mathematical process is accurately represented, which is especially crucial in exams.

  • What does the script emphasize about the process of finding the derivative using the first principle?

    -The script emphasizes the importance of following the correct steps, including writing out the limit notation, substituting correctly, simplifying, and factoring, to arrive at the correct derivative.

  • Can the process described in the script be applied to any differentiable function?

    -Yes, the process described in the script can be applied to any differentiable function to find its derivative using the first principle of calculus.

Outlines
00:00
📐 Understanding First Principles in Calculus

This paragraph emphasizes the importance of using the first principles formula correctly in calculus, particularly in exams. It explains the necessity of writing the correct notation, beginning with the limit as 'h' approaches zero. The author stresses the importance of getting into the habit of consistently writing this out and provides a detailed explanation of how to substitute 'x + h' into the function, leading to the correct application of the formula.

✏️ Simplifying the First Principles Formula

The paragraph continues to explain the process of working with the first principles formula, specifically focusing on expanding brackets and simplifying terms. It walks through multiplying and distributing terms, ensuring that all terms without 'h' cancel out, which is a sign that the process has been done correctly. The author then simplifies the remaining expression, preparing for the final step in the calculation.

✅ Finalizing the First Principles Derivation

In this final part, the paragraph details how to factor out 'h' from the simplified expression and cancel it out. This cancellation allows 'h' to approach zero without causing the expression to be undefined. The author concludes by substituting zero for 'h' and arriving at the final answer, illustrating the completion of the first principles method.

Mindmap
Keywords
💡First principles
First principles refer to the most basic truths or assumptions from which other truths can be derived. In the context of the video, it is the foundational method for deriving formulas or solving problems from the most basic level. The video emphasizes the importance of using the first principle formula, which is a fundamental concept in calculus, particularly when dealing with limits and derivatives.
💡Limit
A limit is a fundamental concept in calculus that describes the behavior of a function as its input approaches a certain value. In the video, the limit is used to define the derivative of a function, where the limit as 'h' approaches zero is repeatedly mentioned, illustrating the process of finding the instantaneous rate of change of a function at a specific point.
💡Notation
Notation in mathematics refers to the symbols and abbreviations used to represent mathematical concepts and operations. The video script stresses the importance of correct notation, especially in exams, as it is crucial for clear communication of mathematical ideas. The use of 'lim' to denote a limit is a specific example of mathematical notation highlighted in the transcript.
💡Derivative
The derivative of a function measures the rate at which the function's output changes with respect to changes in its input. In the video, the process of finding the derivative using the first principle formula is demonstrated, showing how to calculate the instantaneous rate of change of a function at a particular point.
💡Function
A function in mathematics is a relation between a set of inputs and a set of permissible outputs, with the property that each input is related to exactly one output. The video discusses the function 'f(x)', which is used to illustrate the process of finding its derivative using the first principle formula.
💡H
In the context of the video, 'h' is a small change in the input of the function 'f(x)', used in the limit process to find the derivative. It is a variable that approaches zero, and its role is crucial in the calculation of the instantaneous rate of change of the function.
💡Multiplication
Multiplication is a basic arithmetic operation that combines two numbers to indicate how many times one number is added to itself. In the video, multiplication is used to expand the expression '(x + h)^2' and in the process of finding the derivative, where terms are multiplied out to simplify the expression before taking the limit.
💡Cancellation
Cancellation in mathematics refers to the process of eliminating terms from an equation by performing inverse operations. In the video, terms without 'h' cancel each other out, which is a key step in simplifying the derivative expression before taking the limit.
💡Common factor
A common factor is a factor that is present in each term of a mathematical expression. In the video, a common factor of 'h' is factored out from the terms in the derivative expression, which is a step towards simplifying the expression and preparing it for the limit as 'h' approaches zero.
💡Undefined
In mathematics, a term or expression is undefined when it does not have a meaningful value, often due to division by zero. The video script mentions that the expression would be undefined if 'h' were at the bottom of the fraction before cancellation, highlighting the importance of proper simplification before taking the limit.
💡Instantaneous rate of change
The instantaneous rate of change is the rate at which a quantity changes at a specific instant in time, as opposed to the average rate of change over a period of time. The video demonstrates how to calculate this using the first principle formula, showing that the final result of the derivative represents the instantaneous rate of change of the function at a given point.
Highlights

Emphasized the importance of using the first principle formula in exams.

Stressed the need for correct notation to avoid penalties in exams.

Introduced the formula for the limit as h approaches zero.

Demonstrated the process of substituting x with x + h in the function.

Explained the step of expanding the function after substitution.

Highlighted the cancellation of terms without h when taking the limit.

Clarified the process of simplifying the expression by factoring out h.

Described the cancellation of h terms when h approaches zero.

Instructed to let h approach zero to find the final derivative.

Provided a step-by-step guide on how to write the limit notation in exams.

Encouraged the development of a habit for writing down the limit notation correctly.

Explained the concept of multiplying out the brackets in the derivative formula.

Demonstrated the simplification process by canceling terms with h in the numerator and denominator.

Gave a clear example of how to handle the common factor in the derivative formula.

Taught the method of letting h approach zero to find the derivative's final value.

Concluded with the final answer of the derivative being 2x + 3.

Transcripts

Browse More Related Video

Finding Derivative Using Limit Definition

First principles grade 12: Practice

More Difference Quotient Examples

First Principles Calculus Grade 12 | With Fraction

Definition of the Derivative

2019 AP Calculus AB Free Response Question #6

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusDerivativeFirst PrinciplesLimitEducationMathematicsTutorialLearningExam PrepStep-by-Step