AP Physics 2023 Exam Solutions|Q2. PART D | Complete Step-by-Step Answers and Explanations"

Study Circus
10 Oct 202307:05
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the instructor explores the concept of acceleration on an inclined plane, emphasizing that it remains constant regardless of the cart's direction—up or down the ramp. Assuming no friction, the acceleration is attributed to gravity's component. The lesson delves into the physics of motion, providing the formula for displacement and velocity as functions of time. The instructor illustrates how to graph these variables, demonstrating the parabolic position-time graph and the linear velocity-time graph for a cart pushed upwards with an initial velocity, highlighting the point at which the cart's velocity becomes zero.

Takeaways
  • 📚 The script is a part of the AP1 physics curriculum discussing the concept of acceleration on an inclined plane.
  • 🚀 The student aims to confirm that acceleration remains constant whether the cart rolls up or down the ramp.
  • 🔄 The scenario assumes no friction or air resistance, focusing solely on the component of gravity affecting the acceleration.
  • 📏 The x-axis is defined parallel to the ramp, with the origin at the starting point.
  • 📈 The acceleration due to gravity along the ramp is constant, regardless of the cart's direction of motion.
  • 🛤️ The student starts the cart at the bottom of the ramp and gives it an initial push upwards.
  • 📊 The position and velocity of the cart are to be graphed as functions of time for the scenario described.
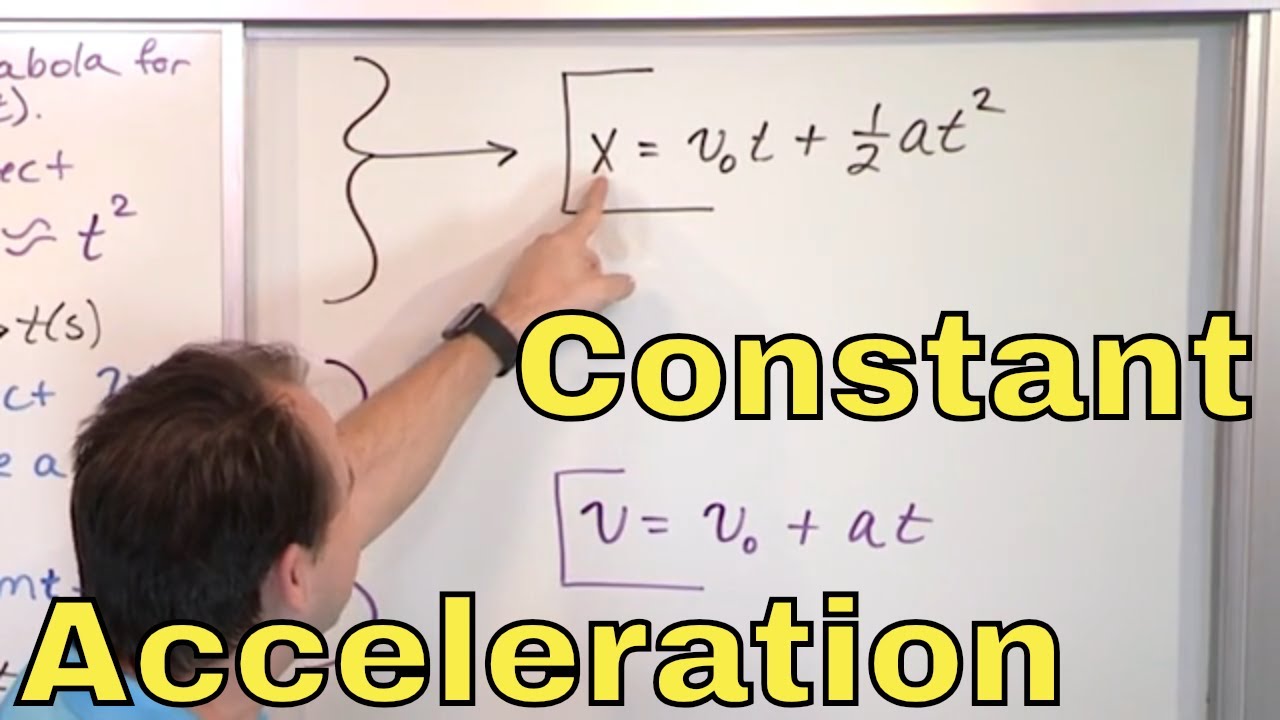
  • 📚 The displacement formula used is \( s = ut + \frac{1}{2}at^2 \), where \( u \) is the initial velocity, \( a \) is the acceleration, and \( t \) is time.
  • 📉 The graph of position versus time is a parabola opening upwards, indicating the cart's upward motion and eventual stop.
  • 📋 The final position of the cart where it stops is marked as \( X_{final} \) on the graph.
  • 🚫 The velocity-time graph is a straight line with a positive slope, starting from an initial velocity and decreasing to zero when the cart stops.
Q & A

  • What is the main topic discussed in this video script?

    -The main topic discussed in the video script is the analysis of the acceleration of a cart moving up and down a ramp, assuming no friction and air resistance, and the corresponding position and velocity graphs as functions of time.

  • Why does the acceleration of the cart remain the same whether it is moving up or down the ramp?

    -The acceleration of the cart remains the same because it is due to gravity, which acts in the same direction (downwards along the ramp) regardless of whether the cart is moving up or down.

  • What is the role of friction and air resistance in this scenario?

    -In this scenario, friction and air resistance are assumed to be non-existent, which simplifies the analysis by allowing the acceleration to be solely due to gravity.

  • What is the initial velocity (U) mentioned in the script, and what does it represent?

    -The initial velocity (U) mentioned in the script represents the speed with which the cart is pushed upwards at the start of the experiment.

  • How is the position of the cart as a function of time represented mathematically in the script?

    -The position of the cart as a function of time is represented by the equation: Position = X₀ + (-U)t + (1/2)at², where X₀ is the initial position, U is the initial velocity, a is the acceleration, and t is time.

  • What type of graph does the position-time relationship form, and why?

    -The position-time relationship forms a parabolic graph because the equation includes a term with t², indicating a quadratic relationship between position and time.

  • What is the significance of the point where the cart's velocity becomes zero?

    -The point where the cart's velocity becomes zero is significant because it represents the moment and position where the cart comes to a complete stop.

  • How is the velocity of the cart as a function of time represented mathematically in the script?

    -The velocity of the cart as a function of time is represented by the equation: Velocity = U + at, where U is the initial velocity, a is the acceleration, and t is time.

  • What type of graph does the velocity-time relationship form, and why?

    -The velocity-time relationship forms a straight-line graph because the equation is linear, with a constant acceleration resulting in a constant rate of change of velocity.

  • What is the final position (Xf) of the cart when it comes to rest, and how is it determined?

    -The final position (Xf) of the cart when it comes to rest is determined by the point on the position-time graph where the velocity becomes zero, indicating that the cart has stopped moving.

  • What additional insights can be gained from the graphs of position and velocity as functions of time?

    -The graphs of position and velocity as functions of time provide insights into the motion of the cart, such as the distance traveled, the time taken to stop, and the rate at which the cart decelerates to a halt.

Outlines
00:00
🚀 Cart's Acceleration on an Inclined Plane

This paragraph discusses the concept of acceleration on an inclined plane, emphasizing that acceleration remains constant regardless of the cart's direction—whether rolling up or down the ramp. The scenario assumes no friction and air resistance, attributing the acceleration solely to gravity's component along the ramp. The paragraph also instructs to sketch the position and velocity of the cart as a function of time, given the cart is pushed upwards with an initial velocity 'U'. The displacement formula used is \( s = UT + \frac{1}{2}at^2 \), where 'a' is the acceleration due to gravity and 't' is time. The position at any time 'T' is the sum of the initial position and the displacement. The paragraph concludes with the description of a parabolic graph for position versus time, indicating the cart's upward motion and eventual stop.

05:01
📈 Graphing Motion of a Cart on an Inclined Plane

The second paragraph continues the discussion on the cart's motion, focusing on graphing its position and velocity over time. It describes the process of plotting a parabolic graph for the cart's position, which opens upwards, indicating the cart's ascent up the incline and eventual halt. The final stopping point is marked as 'X_final'. Additionally, the paragraph explains how to graph the velocity of the cart using the equation of motion \( V = U + at \), resulting in a straight-line graph with a positive slope due to the positive acceleration. The graph starts from an initial negative velocity 'U' and ends at zero velocity when the cart comes to rest at position 'XF'. The paragraph concludes with a summary of the graphical representation of the cart's motion, providing a clear visual understanding of the scenario described.

Mindmap
Keywords
💡Acceleration
Acceleration is the rate of change of velocity of an object with respect to time. In the video's theme, it is used to describe the constant rate at which the cart's velocity changes as it moves up or down the ramp. The script mentions that the acceleration is the same whether the cart is rolling up or down due to gravity, which is a key concept in understanding the physics of the scenario.
💡Cart
The cart in the script represents the object of study in the physics problem. It is the vehicle that is pushed or allowed to roll on an inclined plane. The behavior of the cart under different conditions is central to the video's narrative, illustrating principles of motion and forces.
💡Incline
An incline is a sloping surface, and in this context, it is the ramp on which the cart moves. The incline is crucial to the video's theme as it provides the environment where the effects of gravity and acceleration are observed and analyzed.
💡Friction
Friction is the force that resists the relative motion of two surfaces in contact. The script specifies that there is no friction in the scenario, simplifying the analysis by focusing solely on the effects of gravity and acceleration on the cart's motion.
💡Gravity
Gravity is the force that attracts a body towards the center of the earth, or towards any other physical body having mass. The script mentions gravity as the cause of the acceleration of the cart along the incline, whether it is moving up or down.
💡Initial Velocity
Initial velocity, often denoted as 'U' in the script, is the velocity of the cart at the start of its motion. It is a key parameter in the equations of motion used to predict the cart's behavior as it moves up the incline.
💡Displacement
Displacement refers to the change in position of an object. In the script, displacement is calculated using the formula involving initial velocity, acceleration, and time, which helps in plotting the position-time graph of the cart's motion.
💡Equation of Motion
The equations of motion are mathematical formulas that describe the relationship between an object's motion and the forces acting upon it. The script uses these equations to derive expressions for displacement and velocity as functions of time, which are essential for understanding the cart's behavior.
💡Graph
A graph in this context is a visual representation of data, used to plot the position and velocity of the cart over time. The script describes the process of creating these graphs to visually represent the cart's motion, which is a key part of the explanation.
💡Parabolic
Parabolic refers to the shape of the graph of the cart's displacement over time, which is a parabola opening upwards due to the positive coefficient of the time squared term in the displacement formula. This shape illustrates the cart's decelerating motion as it moves up the incline.
💡Velocity
Velocity is the speed of an object in a specific direction. The script discusses the cart's velocity at different times, including its initial velocity and the point at which the cart comes to rest, which is crucial for understanding the dynamics of the motion.
Highlights

The student aims to confirm that acceleration is consistent whether the cart rolls up or down the ramp.

The cart is started at the bottom and given an initial push upwards.

The x-axis is defined parallel to the ramp with the origin at the starting point.

Acceleration due to gravity is the same in both directions, assuming no friction.

The acceleration is due to a component of gravity acting along the ramp.

A graph is to be sketched to represent position and velocity as functions of time.

The cart is pushed upwards with an initial velocity denoted as 'U'.

The displacement formula s = UT + (1/2)at² is used for motion with constant acceleration.

The direction of initial velocity 'U' is considered negative due to the upward motion.

The position at any given time T is the sum of the initial position and displacement.

The graph of position versus time T is parabolic, opening upwards.

The cart's velocity decreases with time as it moves up the incline.

The final position where the cart stops is marked as 'X final'.

The equation of motion V = U + at is used to find the velocity at any time T.

The graph of velocity versus time T is a straight line with a positive slope.

The cart comes to rest at the point where its velocity is zero.

The explanation provides a detailed analysis of the cart's motion on the ramp.

Transcripts

Browse More Related Video

Position, Velocity, and Acceleration vs. Time Graphs

Kinematics Graphs

motion graphs explained

01 - Motion with Constant Acceleration in Physics (Constant Acceleration Equations)

Velocity Time Graphs, Acceleration & Position Time Graphs - Physics

AP Physics Workbook 1.F Constant Velocity

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsAccelerationInclined PlaneCart MotionGraph AnalysisEquation of MotionFrictionlessGravity EffectVelocity GraphPosition-Time