The Wave Equation for BEGINNERS | Physics Equations Made Easy

Parth G

17 Mar 202016:59

EducationalLearning

32 Likes 10 Comments

TLDRThis video script delves into the concept of the wave equation, a fundamental concept in physics and mathematics. Aimed at high school level understanding, it explains the equation's variables, differentiation, and the behavior of waves over time and space. The script clarifies the wave equation's role in describing sinusoidal waves and their relationship with wave speed. It distinguishes the classical wave equation from other types, like the Schrödinger equation, and highlights its nature as a second-order linear partial differential equation, essential for understanding various types of waves including mechanical, light, and sound.

Takeaways

🌊 The video discusses the concept of the wave equation, a fundamental equation in physics that describes the behavior of waves.
📚 No advanced mathematical background is required to understand the wave equation, as long as one has a basic understanding of high school level physics or math.
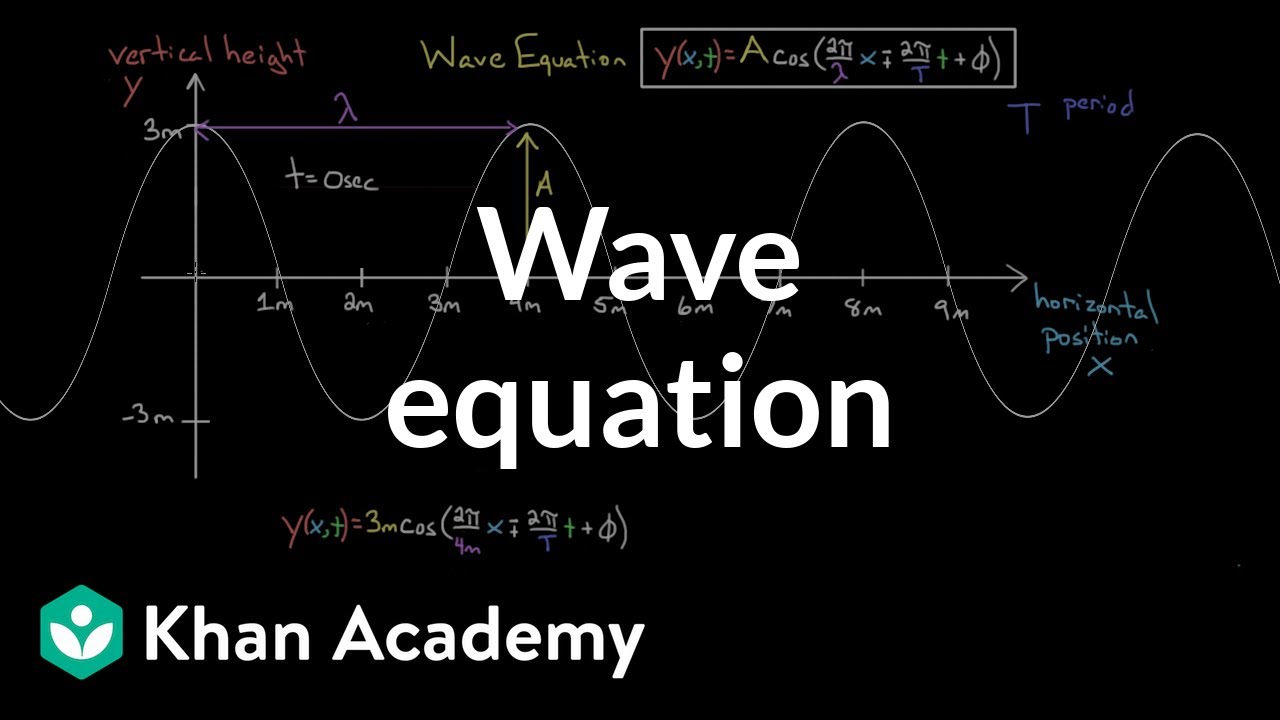
🔍 The wave equation involves variables X for spatial direction and T for time, focusing on how waves behave in space and time.
🌐 The equation is one-dimensional, meaning it only considers movement in the X direction, and is part of classical physics, not accounting for relativity or quantum mechanics.
🚀 The speed of the wave, represented by C, is a key variable in the equation, with examples including the speed of light for electromagnetic waves.
📏 Displacement, denoted by U, is the measure of how different parts of the wave oscillate up and down, representing the wave's movement in the vertical direction.
🔄 Differentiation is a mathematical process used in the wave equation to understand the rate at which the wave's properties change, such as speed and acceleration.
📈 The video uses the analogy of a car's movement to explain differentiation, where the gradient of a graph represents the speed of the car, and its change over time represents acceleration.
💡 The wave equation can be solved by sinusoidal functions, which are waves that have the same shape regardless of their size or position, and are solutions to the equation.
🔢 The wave equation is a second-order linear partial differential equation, meaning it involves twice differentiating a quantity with respect to both space and time, without involving powers of the quantity.
🌐 The video also mentions the Schrödinger equation as an example of a different kind of wave equation, which describes quantum systems and has different properties from the classical wave equation.

Q & A

What is the main topic of the video?
-The main topic of the video is the wave equation, which is a fundamental concept in physics and mathematics used to describe the behavior of waves.
What level of understanding is required to follow the video?
-A high school level understanding of physics or mathematics is sufficient to follow the video, as it does not require advanced mathematical knowledge.
What are the two variables labeled in the wave equation?
-The two variables labeled in the wave equation are X, which refers to a spatial direction, and T, which stands for time.
What does the variable C represent in the wave equation?
-In the wave equation, C represents the speed of the wave being studied, such as the speed of light for an electromagnetic wave.
What is the meaning of the variable u in the wave equation?
-The variable u in the wave equation represents the displacement of the wave, specifically the vertical displacement of different parts of the oscillating wave.
What does the letter D in the wave equation signify?
-The letter D in the wave equation signifies differentiation, indicating that the process of finding rates of change or gradients is being applied.
How is differentiation related to understanding the behavior of waves?
-Differentiation helps in understanding the rate at which wave displacement changes over space and time, allowing us to analyze wave behavior in terms of speed and acceleration.
What is the significance of the second derivative in the wave equation?
-The second derivative in the wave equation, represented as \( \frac{d^2u}{dx^2} \) or \( \frac{d^2u}{dt^2} \), helps in determining the acceleration of the wave in space and time, respectively.
Why are sinusoidal waves considered a solution to the wave equation?
-Sinusoidal waves are considered a solution to the wave equation because their mathematical form matches the requirement that the second derivative of displacement with respect to space, when multiplied by the square of the wave speed, equals the second derivative with respect to time.
What is the difference between the classical wave equation and the Schrödinger equation?
-The classical wave equation describes the behavior of classical waves like mechanical waves, light waves, and sound waves, while the Schrödinger equation describes the behavior of quantum systems' wave functions over time and space, incorporating principles of quantum mechanics.
What does it mean for the wave equation to be a second-order linear partial differential equation?
-Being a second-order linear partial differential equation means that the wave equation involves twice differentiating a quantity (u) with respect to both space (x) and time (t), and the equation is linear, meaning it does not involve any powers of u or products of derivatives.

Outlines

00:00

👋 Introduction and Overview

Parthia greets the viewers, noting his longer hair and beard. He introduces the topic of the wave equation, assuring viewers that a high school-level understanding of physics or math is sufficient. He encourages engagement by asking for thumbs-up, subscriptions, and comments on which equation to explain next.

05:00

📏 Understanding Variables in the Wave Equation

Parthia explains the variables X and T in the wave equation. X represents a spatial direction, while T stands for time. He illustrates how these variables help in studying wave behavior in space and time, using a one-dimensional wave equation. C is introduced as the speed of the wave, and U as the displacement of the wave at different points.

10:01

🔢 Differentiation in the Wave Equation

The concept of differentiation is introduced, with D representing differentiation in the wave equation. Parthia explains how differentiation helps understand the rate of change, using a car's movement graph as an example. He shows how plotting gradients over time helps determine speed and acceleration, relating it to understanding wave behavior.

15:01

🌊 Applying Differentiation to Waves

Parthia applies differentiation to a wave, explaining how to take a snapshot of a wave at a particular time and plot its vertical displacement against its horizontal position. He shows how differentiating this wave with respect to X produces another wave-like graph and explains the significance of these differentiated quantities in understanding wave behavior.

📈 Behavior of a Wave Over Time

Focusing on a single point in space, Parthia examines how its displacement changes over time, producing another sinusoidal graph. Differentiating this graph with respect to time twice leads to an important result: equating D²u/DX² and D²u/DT². This illustrates how sinusoidal waves can be solutions to the wave equation.

🔍 The Classical Wave Equation and Its Solutions

Parthia explains that the classical wave equation applies to various types of waves by adjusting the speed value. He introduces more complex wave equations, like the Schrödinger equation for quantum systems. The classical wave equation is described as a second-order linear partial differential equation, and he emphasizes the importance of understanding its basic principles.

📚 Closing Remarks and Engagement

Parthia wraps up the video, acknowledging the complexity of the topic and encouraging viewers to ask questions or point out any mistakes in the comments. He expresses gratitude for the viewers' support and invites them to suggest more equations for future videos. He ends the video with a cheerful goodbye.

Mindmap

Keywords

💡Wave Equation

The wave equation is a fundamental mathematical relationship that describes how waves propagate through space and time. It is central to the video's theme, as it is the main subject being explained. The script introduces the wave equation as a tool to understand the behavior of various types of waves, such as sound and light, and provides a basic understanding without requiring advanced mathematical knowledge.

💡Spatial Direction

Spatial direction refers to the orientation within a physical space, typically along one of the three axes in a Cartesian coordinate system. In the context of the video, 'X' is used to denote a spatial direction, specifically the horizontal direction to the right, which is essential for understanding how waves behave as they move along this axis.

💡Time (T)

Time is a measure in which events can be ordered from the past through the present into the future. In the video, 'T' symbolizes time and is used to examine the evolution of wave behavior as it progresses. The script discusses observing wave behavior at different instances of time, such as T=0 and T=5 seconds, to understand how the wave changes over time.

💡Displacement (u)

Displacement in the context of waves refers to the change in position of a point in the medium through which the wave is traveling. The script explains 'u' as the vertical displacement of a point on a wave from its equilibrium position, which is crucial for describing the oscillation of a wave in a transverse manner.

💡Speed of the Wave (C)

The speed of the wave, denoted by 'C' in the script, is the rate at which the wave propagates through its medium. It is a key parameter in the wave equation and is used to differentiate between types of waves, such as light waves traveling at the speed of light. The script mentions that 'C' is a simple concept but critical for understanding wave behavior.

💡Differentiation

Differentiation is a mathematical process that finds the rate at which a quantity changes. In the video, differentiation is used to analyze the wave's properties, such as its speed (first derivative with respect to time) and acceleration (second derivative with respect to time). The script provides an analogy of a car's movement to explain how differentiation helps in understanding the rate of change.

💡Sinusoidal Waves

Sinusoidal waves are a type of wave that follows a sinusoidal pattern, typically represented by a sine or cosine function. The script uses sinusoidal waves as an example to illustrate the behavior of waves described by the wave equation. It explains that these waves are a valid solution to the equation and are characterized by their specific shape and oscillation.

💡Partial Differential Equation

A partial differential equation (PDE) involves unknown multivariable functions and their partial derivatives. The wave equation is an example of a PDE because it includes partial derivatives with respect to both space and time. The script mentions that the wave equation is a second-order linear PDE, which is key to understanding its mathematical nature and solutions.

💡Classical Wave Equation

The classical wave equation is a specific form of the wave equation that does not account for quantum mechanics or relativistic effects. It is used to describe the behavior of mechanical waves, light waves, and other classical phenomena. The script distinguishes this from other wave equations like the Schrödinger equation, which describes quantum systems.

💡Angular Frequency (Ω) and Wave Number (k)

Angular frequency (Ω) and wave number (k) are parameters that characterize a wave's properties. Angular frequency is a measure of how fast the wave oscillates per unit time, while wave number is a measure of how many wavelengths fit into a given distance. The script briefly mentions these terms in the context of a possible solution to the wave equation, indicating their importance in describing wave behavior.

Highlights

Introduction to the wave equation without the need for advanced mathematical knowledge, aiming to be accessible to those with a high school level understanding of physics or mathematics.

Explanation of the basic variables in the wave equation: X for spatial direction and T for time, emphasizing the study of wave behavior in both space and time.

Introduction of the one-dimensional wave equation, focusing on the X direction and its implications for wave study.

Clarification of C as the speed of the wave being studied, with examples such as the speed of light for electromagnetic waves.

Description of U as the displacement of the wave, illustrating how different parts of the wave oscillate up and down.

Differentiation (D) in the wave equation explained as a mathematical tool to understand the rate of change, such as the speed of a moving object.

Use of differentiation to find the speed of an object by differentiating its position with respect to time (DX/DT).

Further differentiation to find acceleration by differentiating speed with respect to time, resulting in a constant gradient indicative of constant acceleration.

Differentiation of a wave's snapshot with respect to X to find the wave's gradient at every point, resulting in a new mathematical function.

Differentiation of the wave's displacement with respect to X squared (D^2U/DX^2) to understand the wave's curvature across space.

Exploration of a wave's behavior at a specific spatial point over time, differentiating twice with respect to T (D^2U/DT^2) to analyze the wave's temporal dynamics.

Equating the spatial and temporal second derivatives of the wave displacement to derive the wave equation, showing that sinusoidal waves are solutions to the equation.

Discussion on the constraints of the wave function arguments, relating to the angular frequency and wave number, and their importance in wave description.

Differentiation between the classical wave equation and other types, such as the Schrödinger equation, which describes quantum systems.

Characterization of the wave equation as a second-order linear partial differential equation, explaining its mathematical properties and significance.

Invitation for viewers to request the next equation to be explained, encouraging interaction and further exploration of mathematical concepts.

Closing remarks, expressing gratitude for viewers' support and interest in the content, and a prompt for feedback on understanding and potential inaccuracies.

Transcripts

Browse More Related Video

Mechanical Waves

19. Waves

Partial Derivatives of Waves

The equation of a wave | Physics | Khan Academy

Wave Equation

Waves: Light, Sound, and the nature of Reality