Pendulums | Oscillations and mechanical waves | Physics | Khan Academy

Khan Academy Physics
29 Jul 201614:45
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the concept of the simple pendulum as a type of simple harmonic oscillator. It explains that the restoring force and motion of a pendulum can be described by the simple harmonic oscillator equation, using the angular displacement instead of linear displacement. The script clarifies that while a simple pendulum is not a perfect harmonic oscillator, it closely resembles one, especially for small angles. The period of a pendulum is shown to be dependent on the length of the string and the gravitational acceleration, but not the mass of the pendulum bob. The formula for the period is provided, and it is emphasized that the approximation holds well for small angles, with deviations increasing as the angle approaches 70 degrees.

Takeaways
  • 🔍 The script discusses simple harmonic oscillators, with a focus on pendulums as the second most common example after masses on springs.
  • 🌐 A pendulum is a mass attached to a string of length L, which can be displaced and then oscillates back and forth like a simple harmonic oscillator.
  • 📚 The simple pendulum is considered simple because it consists of a single mass and string, unlike more complex systems like the double pendulum which can exhibit chaotic behavior.
  • 📉 The motion of a pendulum can be described using the simple harmonic oscillator equation, but with angular displacement (theta) instead of linear displacement (X).
  • 🔄 The restoring force in a pendulum comes from gravity, which pulls the mass back towards its equilibrium position, creating an oscillating motion.
  • 🔄 The period of a simple pendulum is not affected by the mass of the pendulum due to the canceling effects of increased inertia and gravitational force.
  • 📏 The period of a pendulum is directly proportional to the square root of the length of the string (L) and inversely proportional to the square root of the acceleration due to gravity (g).
  • 🌌 The formula for the period of a pendulum is derived from calculus and involves the moment of inertia and torque, showing that increasing the string length increases the period.
  • ⚖️ Increasing the gravitational acceleration (g) decreases the period of the pendulum because a larger force results in greater acceleration and speed, completing the cycle faster.
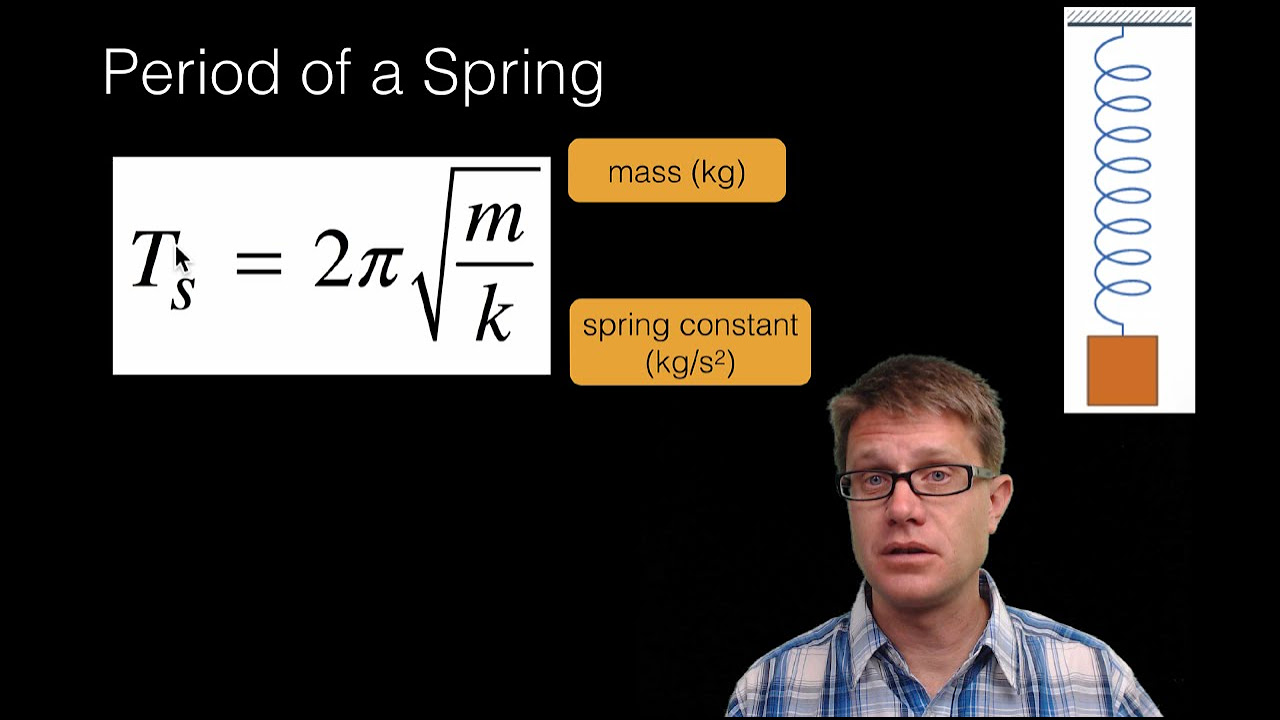
  • 🔑 The period formula for a pendulum, \( T = 2\pi \sqrt{\frac{L}{g}} \), is analogous to that of a mass on a spring, with the inertia term (mass or length) on top and the force term (spring constant or g) on the bottom.
  • 🚫 The simple pendulum's behavior as a simple harmonic oscillator and the accuracy of the period formula are only valid for small angular amplitudes, typically less than 20 degrees for minimal error.
Q & A

  • What is the most common example of a simple harmonic oscillator?

    -The most common example of a simple harmonic oscillator is a mass on a spring.

  • What is the second most common example of a simple harmonic oscillator mentioned in the script?

    -The second most common example is a pendulum, which is a mass, m, connected to a string of length, L, that swings back and forth.

  • What is a simple pendulum and how does it differ from a more complicated pendulum system?

    -A simple pendulum is a mass connected to a string without any additional masses or strings attached. A more complicated pendulum, such as a double pendulum, involves additional masses and strings, which can lead to chaotic motion and is much more complex to describe mathematically.

  • Why do we study pendulums when studying simple harmonic oscillators?

    -We study pendulums because they oscillate similarly to simple harmonic oscillators, and they can be described by the simple harmonic oscillator equation, especially when the angles are small.

  • What variable is commonly used to describe the motion of a pendulum instead of displacement (X)?

    -The variable commonly used to describe the motion of a pendulum is the angle (theta) that the pendulum makes with the vertical at any given time.

  • What is the small angle approximation and why is it used for pendulums?

    -The small angle approximation is an assumption that the pendulum's maximum angular displacement (amplitude) is small enough that the pendulum can be treated as a simple harmonic oscillator. It is used because for small angles, the deviation from true simple harmonic motion is minimal, typically less than 1% for angles less than 20 degrees.

  • How does the mass of the pendulum affect its period of oscillation?

    -Surprisingly, the mass of the pendulum does not affect its period of oscillation. This is because the increased inertia due to greater mass is offset by the increased gravitational force pulling the mass back towards equilibrium.

  • What factors determine the period of a pendulum according to the formula provided in the script?

    -The period of a pendulum is determined by the length of the string (L) and the acceleration due to gravity (g). The formula for the period is \( T = 2\pi \sqrt{\frac{L}{g}} \).

  • Why does increasing the length of the string (L) increase the period of a pendulum?

    -Increasing the length of the string increases the moment of inertia, which makes it harder to change the angular velocity of the pendulum. As a result, it takes longer to complete a cycle, thus increasing the period.

  • Why does increasing the gravitational acceleration (g) decrease the period of a pendulum?

    -Increasing the gravitational acceleration increases the force of gravity acting on the pendulum, which in turn increases the restoring force and the acceleration of the pendulum. This causes the pendulum to move faster and complete its cycle in less time, thus decreasing the period.

  • How does the amplitude of the pendulum's swing affect the period, assuming small angles?

    -For small angles, the amplitude of the pendulum's swing does not affect the period. This is because the pendulum can be approximated as a simple harmonic oscillator under these conditions.

  • What is the error percentage of the period formula for pendulums when the maximum angle is less than 20 degrees?

    -When the maximum angle is less than 20 degrees, the error percentage of the period formula for pendulums is less than 1%.

Outlines
00:00
🔍 Introduction to Simple Harmonic Oscillators and Pendulums

The instructor begins by introducing simple harmonic oscillators, with masses on springs as the most common example, followed by pendulums. A pendulum is described as a mass attached to a string of length L that can swing back and forth. The video aims to explore the pendulum's behavior as a simple harmonic oscillator, with a focus on the simple pendulum for its uncomplicated setup. The instructor clarifies that while pendulums are technically not perfect simple harmonic oscillators, they are treated as such for small angular displacements due to their close approximation. The simple harmonic oscillator equation is introduced, with the variable X replaced by the angular displacement theta, to describe the pendulum's motion over time.

05:01
🌀 The Effects of Mass on Pendulum Period

The instructor discusses the misconception that increasing the mass of a pendulum would affect its period. It is explained that while increasing mass increases inertia, making it harder to move, the gravitational force also increases, which could suggest a faster motion. However, these two effects cancel each other out, and the mass does not influence the period of the pendulum. The formula for the period of a simple pendulum is introduced without derivation, emphasizing that it requires calculus for a complete understanding. The instructor provides an intuitive explanation of why the variables in the formula, such as the length of the string (L) and the acceleration due to gravity (g), affect the period as they do.

10:02
⏳ Factors Influencing the Period of a Simple Pendulum

The instructor explains that the period of a simple pendulum is influenced by the length of the string and the acceleration due to gravity. Increasing the length of the string increases the moment of inertia, making it harder to change the pendulum's angular velocity, thus increasing the period. Conversely, increasing the gravitational acceleration increases the restoring force, leading to a faster motion and a decreased period. The instructor draws an analogy between the pendulum's formula and that of a mass on a spring, highlighting the similarities in how inertia and force terms affect the period. It is also emphasized that the amplitude of the pendulum's swing does not affect the period, provided the angles are small, aligning with the small amplitude approximation for treating the pendulum as a simple harmonic oscillator.

Mindmap
Keywords
💡Simple Harmonic Oscillator
A simple harmonic oscillator (SHO) is a system that, when displaced from its equilibrium position, experiences a restoring force proportional to the displacement. In the context of the video, SHOs are exemplified by masses on springs and pendulums. The theme of the video revolves around understanding the behavior of pendulums as a type of SHO, particularly under the small angle approximation where their motion can be described by the SHO equation.
💡Pendulum
A pendulum is a weight suspended from a pivot so that it can swing freely. In the video, the pendulum is discussed as the next most common example of an SHO after masses on springs. The script explains how a pendulum oscillates back and forth due to the restoring force of gravity, and how its motion can be analogous to that of an SHO when considering small angular displacements.
💡Restoring Force
The restoring force is the force that brings an object back to its equilibrium position when it is displaced. In the video, the restoring force for a pendulum is gravity, which pulls the pendulum bob back towards the vertical position. The script emphasizes that this force is proportional to the displacement from equilibrium, a key characteristic of SHOs.
💡Displacement
Displacement refers to the change in position of an object from its equilibrium or original position. In the context of the video, displacement is used to describe how far the pendulum's bob is from its vertical rest position, measured either as a linear distance or, more commonly for pendulums, as an angular displacement.
💡Angular Displacement
Angular displacement is the angle between the pendulum's equilibrium position and its current position. The video script explains that for a pendulum, the angular displacement (theta) is used to describe its motion over time, rather than the linear displacement used in the case of a mass on a spring.
💡Amplitude
Amplitude is the maximum displacement of the oscillating object from its equilibrium position. In the video, amplitude is discussed in relation to the pendulum's motion, where it refers to the maximum angular displacement (theta maximum). The script notes that for small amplitude oscillations, the pendulum can be approximated as an SHO.
💡Period
The period of an oscillating system is the time taken for one complete cycle of motion. The video explains how the period of a pendulum is related to its length and the acceleration due to gravity. The script provides a formula for the period and discusses how it is affected by these variables.
💡Phase Constant
A phase constant in the context of SHOs is an initial phase angle that can be added to the SHO equation to account for the starting position of the oscillation. The video script mentions that while a phase constant can be included, it is often unnecessary when dealing with SHOs, including pendulums.
💡Small Angle Approximation
The small angle approximation is an assumption used in physics where the sine of an angle is approximated as the angle itself, in radians, for angles less than about 10 degrees. The video script uses this approximation to simplify the pendulum's motion, allowing it to be treated as an SHO for small angular displacements.
💡Moment of Inertia
Moment of inertia is a measure of an object's resistance to changes in its rotation. In the video, the moment of inertia is discussed in relation to the pendulum's length (L) and how it affects the period of the pendulum. The script explains that increasing the length of the pendulum increases its moment of inertia, making it more difficult to change the angular velocity and thus increasing the period.
💡Torque
Torque is the rotational equivalent of linear force and is responsible for producing angular acceleration. In the video, torque is discussed in the context of the gravitational force acting on the pendulum, which provides the torque necessary for the pendulum to swing. The script explains that while torque increases linearly with the length of the pendulum, the moment of inertia increases with the square of the length, resulting in a longer period for a pendulum with a longer string.
💡Gravitational Acceleration
Gravitational acceleration, often denoted as 'g', is the acceleration due to gravity acting on an object. In the video, it is discussed as a factor in the formula for the period of a pendulum. The script explains that increasing the gravitational acceleration increases the force acting on the pendulum, leading to a faster oscillation and a shorter period.
Highlights

A simple pendulum is the second most common example of a simple harmonic oscillator after masses on springs.

The simple pendulum consists of a mass m connected to a string of length L that swings back and forth.

The motion of a pendulum can be described by the simple harmonic oscillator equation with angular displacement.

The amplitude in the pendulum's equation is the maximum angular displacement, not the linear displacement.

A simple pendulum is technically not a perfect simple harmonic oscillator but is treated as such for small angles.

The period of a pendulum is not affected by the mass of the pendulum bob.

The period of a pendulum is directly proportional to the square root of the length of the string.

Increasing the length of the pendulum string results in an increased period of oscillation.

The moment of inertia of the pendulum is directly related to the square of the string's length.

The period of a pendulum is inversely proportional to the square root of the gravitational acceleration.

Increasing gravitational acceleration decreases the period of the pendulum due to increased restoring force.

The simple pendulum's period formula is analogous to that of a mass on a spring system.

Amplitude does not affect the period of a pendulum as long as the oscillations are within the small angle approximation.

The small angle approximation for a pendulum to act as a simple harmonic oscillator is typically less than 20 degrees.

For angles less than 20 degrees, the error in the pendulum's period formula is less than one percent.

The error in the pendulum's period formula is less than three percent for angles less than 40 degrees.

Even at angles up to 70 degrees, the error in the pendulum's period formula is less than ten percent.

The simple pendulum's period formula is derived from the principles of torque and moment of inertia, not requiring calculus for understanding.

Transcripts

Browse More Related Video

AP Physics Workbook 6.J SMall Angles, Tensions, and Pendulum Period

Simple Harmonic Motion

8.01x - Lect 10 - Hooke's Law, Springs, Pendulums, Simple Harmonic Motion

Simple Harmonic Motion - Physics 101 / AP Physics 1 Review with Dianna Cowern

AP Physics Workbook 6.G Period and Amplitude for SHM

Introduction to Simple Harmonic Motion (SHM) | General Equation and Derivation

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Pendulum MotionHarmonic OscillatorPhysics TutorialSmall AnglesPeriod FormulaGravitational ForceAngular DisplacementInertia EffectTorque AnalysisEducational Content