AP Calculus AB: Infinity Limits Examples
TLDRIn this instructional video, Mr. Sandoval guides students through understanding infinite limits in calculus, particularly when dealing with logarithms and polynomials. He illustrates how x squared grows faster than the natural logarithm of x as x approaches infinity. For x approaching zero from the right, he explains that ln(x) approaches negative infinity while x squared approaches zero, resulting in negative infinity. Lastly, he covers the limit of e to the power of negative x divided by x, showing that the exponential function grows faster than the linear one, leading to a limit of zero. This concise tutorial is designed to clarify common confusion points for students preparing for the AP exam.
Takeaways
- 📚 The video is an educational tutorial by Mr. Sandoval focusing on calculus, specifically dealing with infinite limits.
- 🔍 The first example discusses the limit as x approaches infinity for x^2 and ln(x), emphasizing that x^2 grows faster than ln(x).
- 📈 In the first example, the conclusion is that both x^2 and ln(x) approach infinity, but x^2 does so at a faster rate.
- 👉 The second example addresses the limit as x approaches zero from the right for x^2 and ln(x), noting the different behavior as x gets closer to zero.
- 📉 For the second example, ln(x) approaches negative infinity while x^2 approaches zero, resulting in the overall limit being negative infinity.
- 🌐 The third example introduces e^(-x), a common function in calculus, and compares it with x as x approaches negative infinity.
- 📊 In the third example, e^(-x) grows very large as x approaches negative infinity, while x simply increases linearly.
- ⏫ The exponential function e^(-x) grows much faster than the linear function x, which is a key point in understanding the limit in the third example.
- 💡 The conclusion of the third example is that the limit approaches zero because the exponential growth outpaces the linear increase.
- 📝 The video aims to clarify common confusions about infinite limits, especially when dealing with logarithms and polynomials.
- 🎓 These examples are likely to be seen on advanced placement (AP) exams, indicating their importance for students preparing for such tests.
Q & A
What is the primary focus of Mr. Sandoval's calculus examples in the transcript?
-Mr. Sandoval's primary focus is on illustrating how to approach infinite limits in calculus, particularly when dealing with logarithms combined with polynomials.
What happens to the value of x squared as x approaches infinity?
-As x approaches infinity, the value of x squared also approaches infinity, but it does so at a faster rate than the natural logarithm of x.
How does the natural logarithm of x behave as x approaches infinity?
-The natural logarithm of x, denoted as ln(x), also approaches infinity, but at a slower rate compared to x squared.
In the context of the first example, which function grows faster as x approaches infinity: x squared or ln(x)?
-The function x squared grows faster than ln(x) as x approaches infinity.
What is the limit of x squared over ln(x) as x approaches infinity?
-The limit of x squared over ln(x) as x approaches infinity is positive infinity because x squared grows faster than ln(x).
What is the notation for x approaching zero from the right side?
-The notation for x approaching zero from the right side is 'x → 0⁺' or 'lim (x→0⁺)'.
What happens to the value of ln(x) as x approaches zero from the right side?
-As x approaches zero from the right side, the value of ln(x) approaches negative infinity.
What is the limit of x squared over ln(x) as x approaches zero from the right side?
-The limit of x squared over ln(x) as x approaches zero from the right side is negative infinity because ln(x) approaches negative infinity while x squared approaches zero.
What does the function e to the negative x represent and how does it behave as x approaches negative infinity?
-The function e to the negative x, denoted as e^(-x), represents an exponential decay. As x approaches negative infinity, e^(-x) approaches zero.
In the context of the third example, which function grows faster as x approaches negative infinity: e to the negative x or x?
-The function e to the negative x grows faster than the linear function x as x approaches negative infinity.
What is the limit of e to the negative x over x as x approaches negative infinity?
-The limit of e to the negative x over x as x approaches negative infinity is zero because e^(-x) approaches zero faster than x approaches infinity.
Outlines
📚 Understanding Infinite Limits in Calculus
Mr. Sandoval introduces the concept of infinite limits in calculus, particularly focusing on the behavior of logarithmic and polynomial functions as x approaches infinity. He illustrates that x squared grows faster than the natural logarithm of x, leading to the conclusion that the limit of x squared minus ln(x) as x approaches infinity is positive infinity. This segment is designed to clarify common confusions and prepare students for advanced calculus topics.
📉 Limits Involving x Approaching Zero from the Right
The second paragraph delves into the limit as x approaches zero from the right side, emphasizing the notation and the behavior of x squared and ln(x) in this scenario. Mr. Sandoval explains that ln(x) approaches negative infinity while x squared approaches zero, resulting in a limit of negative infinity. This part is crucial for understanding how different functions behave as they approach zero from the positive side and the implications for calculus problems.
📈 Comparing Exponential and Linear Growth in Limits
In the final paragraph, Mr. Sandoval discusses the limit involving the exponential function e to the power of negative x as x approaches negative infinity. He contrasts the rapid growth of the exponential function with the linear growth of x, leading to the conclusion that the limit of e to the power of negative x divided by x as x approaches negative infinity is zero. This example is meant to illustrate the dominance of exponential growth over linear growth in certain limit scenarios.
Mindmap
Keywords
💡Infinite Limits
💡Logarithms
💡Polynomials
💡Asymptote
💡Approaching Zero from the Right Side
💡Negative Infinity
💡Exponential Functions
💡Linear Functions
💡Limits
💡Division
Highlights
Introduction to practicing calculus with infinite limits.
Explanation of how to approach limits with logarithms and polynomials.
Visual representation of x squared and ln(x) as x approaches infinity.
Comparison of growth rates between x squared and ln(x).
Understanding that x squared grows faster than ln(x).
Result of a very large number divided by a smaller number leading to positive infinity.
Transition to a more complex limit example with x approaching zero from the right side.
Clarification of notation and direction of approach in limits.
Behavior of ln(x) approaching negative infinity as x approaches zero from the right.
Behavior of x squared approaching zero as x approaches zero from the right.
Outcome of a negative number divided by an extremely small number leading to negative infinity.
Introduction of the limit involving e to the power of negative x.
Understanding the behavior of e to the negative x as x approaches negative infinity.
Comparison of growth rates between the exponential function and a linear function.
Result of an exponential function growing faster than a linear function leading to a limit of zero.
Emphasis on the importance of visualizing limit behaviors for understanding.
Conclusion highlighting the relevance of these examples for the AP exam.
Transcripts
Browse More Related Video

Using L'Hopital's Rule to show that exponentials dominate polynomials

Finding limits at infinity using squeeze theorem | Squeeze or Sandwich Theorem - Calculus

Limits at infinity of quotients (Part 2) | Limits and continuity | AP Calculus AB | Khan Academy

How To Find The Limit At Infinity
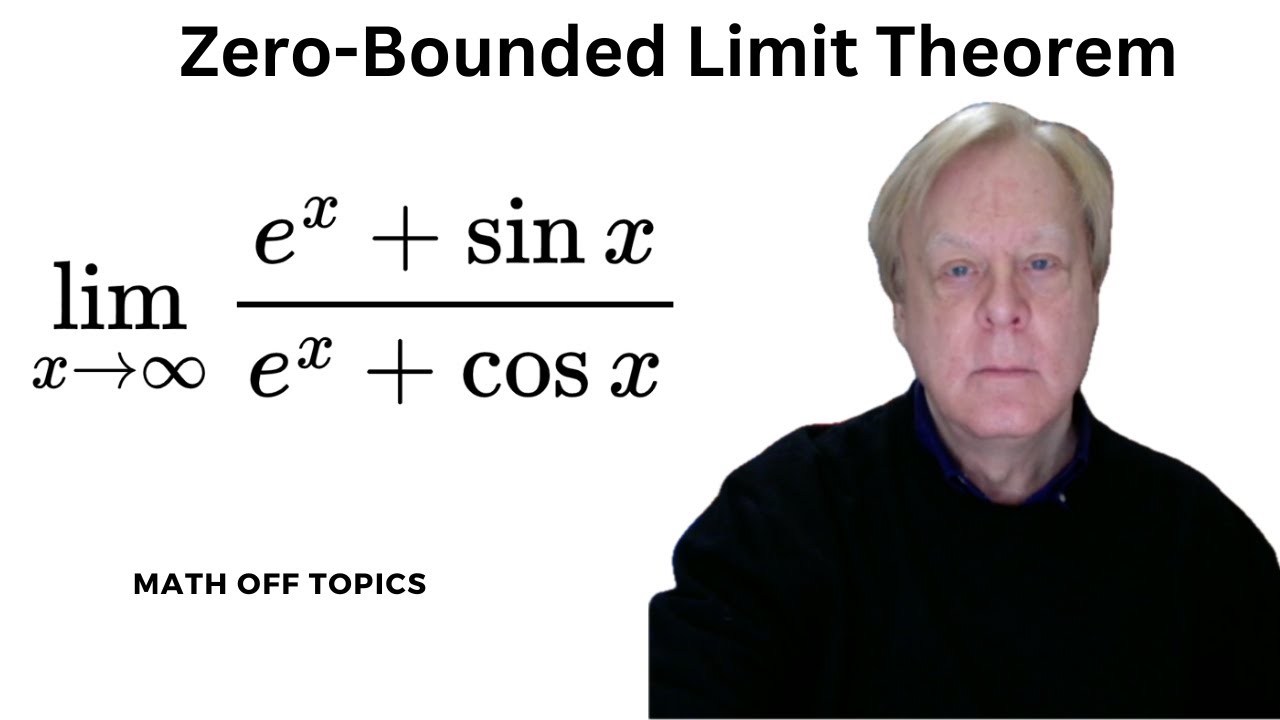
Zero-Bounded Limit Theorem (with example)
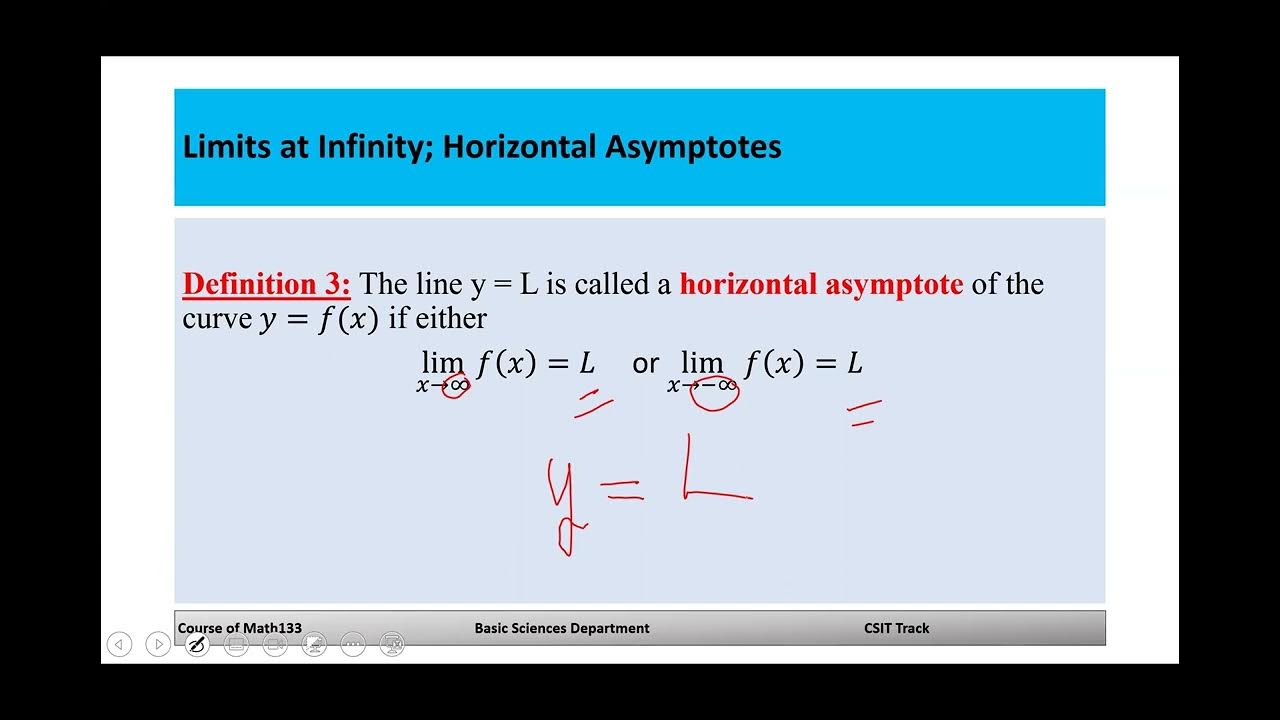
Math 133 Lecture 2 6
5.0 / 5 (0 votes)
Thanks for rating: