Calculus 1 Lecture 4.1: An Introduction to the Indefinite Integral
TLDRThis transcript details a calculus lesson focusing on the second main problem of calculus: finding the area under a curve. The instructor introduces the rectangular method and anti-derivative method for approximating areas, emphasizing the importance of understanding these concepts before applying them. The lecture also covers the properties of integrals, their relationship with derivatives, and the process of integration. The instructor illustrates these concepts with examples and provides an integration table for reference, aiming to demystify the process of finding anti-derivatives.
Takeaways
- 📚 The script introduces Chapter 4, which transitions from using derivatives to a new concept, focusing on the second main problem in calculus: finding the area under a curve.
- 📉 The instructor discusses the 'rectangular method' for approximating the area under a curve by dividing the interval into equal subsections and creating rectangles within each subsection.
- 📏 The 'anti-derivative method' is introduced as a more concise way to find the area under a curve, which involves reversing the process of taking a derivative to find the original function.
- 🔍 The concept of anti-derivatives is explained as functions that, when differentiated, yield the original function, representing the area under the curve.
- 📈 The importance of the constant 'C' in anti-derivatives is highlighted, as it represents a family of curves that could be the area function for a given derivative.
- 📝 The instructor emphasizes the need to understand the concept of anti-derivatives before practicing techniques, as it's crucial for solving calculus problems involving areas.
- 📚 The script previews the content of sections 4.3 and 4.4, where the rectangular method and anti-derivative method will be further explained and proven.
- 📉 The 'definite integral' is mentioned as a concept that will be discussed later, which involves finding the exact area between specific points on a curve.
- 📌 The instructor provides an example of finding the area under the curve for the function f(x) = x + 1 from 1 to x, using the concept of anti-derivatives.
- 📘 The script ends with a brief mention of the 'indefinite integral,' which is the process of finding anti-derivatives without specific boundaries, in contrast to the definite integral.
Q & A
What is the main focus of Chapter 4 in the calculus course?
-Chapter 4 introduces a switch from using derivatives, as covered in previous chapters, to discussing the second main problem in calculus: finding the area under a curve.
What are the two primary methods introduced in Chapter 4 for finding the area under a curve?
-The two primary methods introduced are the rectangular method and the anti-derivative method.
Can you briefly explain the rectangular method for finding the area under a curve?
-The rectangular method involves dividing the area under the curve into equal subsections and creating rectangles within each subsection. The height of each rectangle is determined by the curve at the left endpoint, right endpoint, or midpoint of the subsection.
What is an anti-derivative and how is it related to finding the area under a curve?
-An anti-derivative is a function that, when differentiated, yields the original function. It is used to find the area under a curve by 'undoing' the derivative to get back to the original function, which represents the curve.
How does the number of rectangles used in the rectangular method affect the approximation of the area under a curve?
-The more rectangles used in the rectangular method, the better the approximation of the area under the curve becomes. As the number of rectangles approaches infinity, the approximation becomes exact.
What is the significance of the constant 'C' in the context of anti-derivatives?
-The constant 'C' represents the family of curves that could be the area function for a given derivative. It accounts for the unknown vertical shift of the original function and is essential in determining the specific area function that corresponds to the given derivative.
How does the derivative of an anti-derivative relate to the original function?
-The derivative of an anti-derivative is the original function. This is because anti-derivatives are essentially the reverse process of differentiation, so taking the derivative of an anti-derivative 'undoes' the process and returns the original function.
What is the purpose of the indefinite integral in calculus?
-The purpose of the indefinite integral is to find the anti-derivative of a function, which represents the area function or the function that, when differentiated, gives back the original function. It is called 'indefinite' because it does not specify the boundaries or the exact area but rather the function that describes the area.
What is the basic rule for integrating a function of the form f(x) with respect to x?
-The basic rule for integrating a function of the form f(x) with respect to x is to add 1 to the exponent of x in the function and then divide by the new exponent. This process 'undoes' the derivative, allowing you to find the anti-derivative.
How does the process of finding the area under a curve relate to the concept of limits in calculus?
-The process of finding the area under a curve involves taking the limit as the number of subdivisions (rectangles) approaches infinity, making the width of each rectangle approach zero. This concept of limits is fundamental in calculus for understanding how to approximate values that cannot be exactly determined, such as areas under irregular curves.
Outlines
📚 Introduction to Chapter Four: Switching to Area Problems
The instructor begins by introducing Chapter Four, which transitions from the study of derivatives to a new focus on area problems in calculus. They explain that the first main problem of calculus was finding the slope of a curve at a point, while the second, which will be the focus of Chapter Four, is determining the area under a curve. Two methods for solving this problem are introduced: the rectangular method and the anti-derivative method. The rectangular method involves dividing the area under the curve into rectangles to approximate the area, while the anti-derivative method offers a more concise approach. The instructor promises an intuitive explanation of these concepts and a deeper dive into the rectangular method in later sections.
📐 The Rectangular Method: Approximating Area Under a Curve
The instructor delves into the rectangular method, explaining how to divide the area under a curve into equal sub-intervals and create rectangles within each to approximate the area. They discuss the use of left endpoints, right endpoints, and midpoints to determine the height of each rectangle. The instructor emphasizes that while this method provides an approximation, increasing the number of rectangles will improve the accuracy. They also touch on the concept of limits, explaining how as the number of rectangles approaches infinity, the approximation becomes exact.
🔍 The Anti-Derivative Method: A Concise Approach to Area Problems
The instructor introduces the anti-derivative method, which is a more concise way to find the area under a curve compared to the rectangular method. They explain that the anti-derivative is essentially the reverse process of taking a derivative. The instructor provides an intuitive understanding of anti-derivatives and promises a formal proof later in the course. They also discuss the concept of the area function and how it relates to the original function, highlighting the importance of the derivative of the area function yielding the original function back.
📉 Geometric Interpretation of Anti-Derivatives and Area Functions
The instructor provides a geometric interpretation of anti-derivatives and area functions using the example of the function f(x) = x + 1. They explain how to find the area under this curve from negative 1 to x by treating the function as the height of a triangle and calculating its area. The instructor then demonstrates how taking the derivative of the area function results in the original function, illustrating the relationship between anti-derivatives and the original functions.
🧩 The Process of Anti-Differentiation and Its Relevance to Area Problems
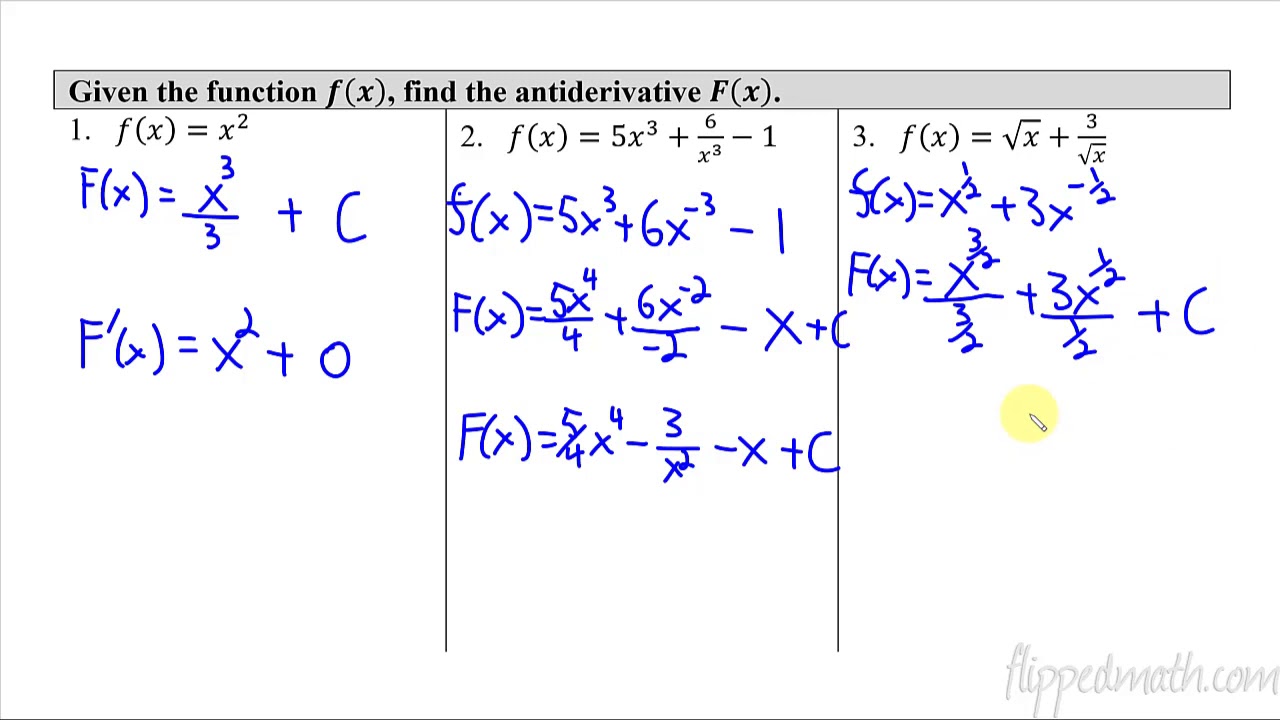
The instructor discusses the process of anti-differentiation, which is the act of finding an anti-derivative. They emphasize that the anti-derivative is a function that, when differentiated, yields the original function. The instructor uses the example of finding the area under the curve for the function f(x) = x^2 from 0 to x, and demonstrates how to find the anti-derivative by reversing the derivative process. They also introduce the concept of an indefinite integral, which represents the family of all possible anti-derivatives.
🔄 The Fundamental Relationship Between Derivatives and Anti-Derivatives
The instructor reinforces the fundamental relationship between derivatives and anti-derivatives, stating that the derivative of an anti-derivative returns the original function. They use the example of the function f(x) = x^2 and its anti-derivative F(x) = (1/3)x^3 + C to illustrate this concept. The instructor also introduces the notation for indefinite integrals and explains the significance of the constant C in representing a family of curves that are anti-derivatives of the original function.
📘 Introduction to Integration and the Integral Symbol
The instructor introduces the concept of integration as the process of finding an anti-derivative. They explain that integration is synonymous with anti-differentiation and that the integral symbol represents the operation of finding an anti-derivative. The instructor discusses the notation used in integrals, including the importance of the differential 'dx', which indicates the variable with respect to which the integration is performed.
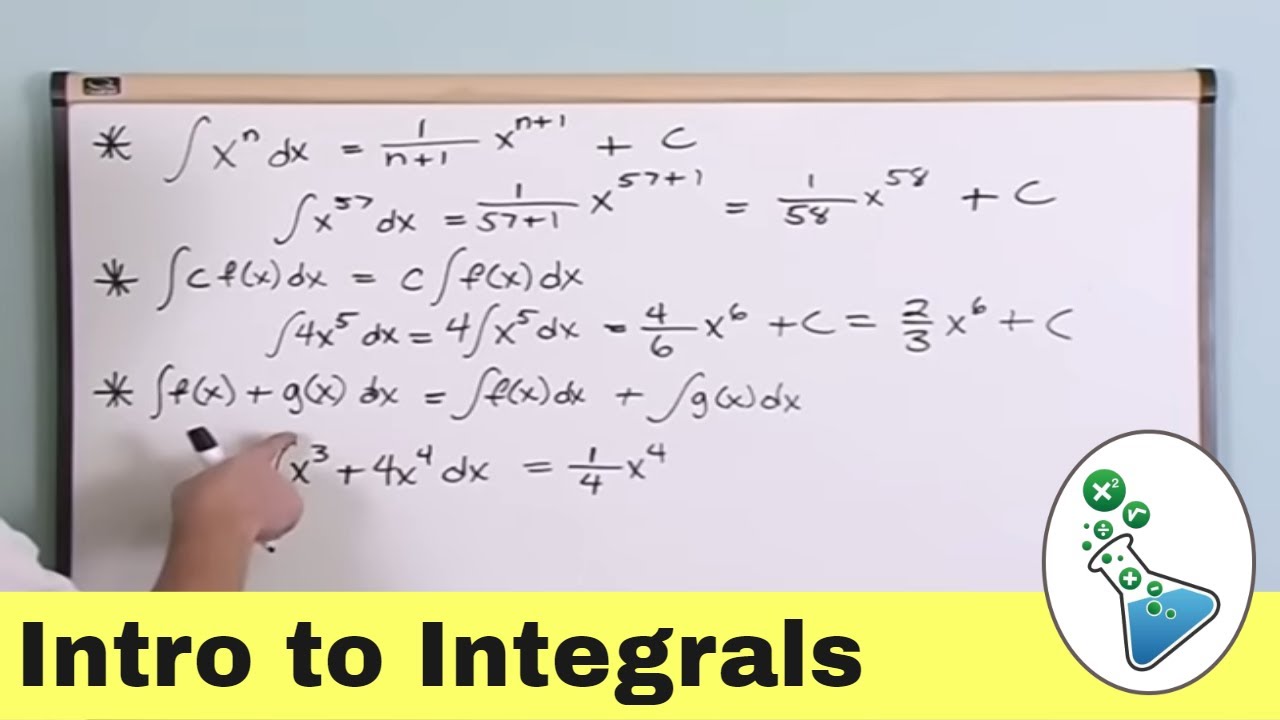
📌 The Basic Integration Table and Memorization of Integral Formulas
The instructor provides an overview of the basic integration table, which lists common functions and their corresponding integrals. They emphasize the importance of memorizing these formulas, as they are derived from the reverse operations of differentiation. The instructor also explains the process of integrating functions by reversing the operations of differentiation, such as adding to the exponent and dividing by the new exponent for power functions.
🔍 Detailed Explanation of Integration Rules for Trigonometric Functions
The instructor offers a detailed explanation of the integration rules for trigonometric functions, such as the integral of cosine, which is sine plus C, and the integral of tangent, which is negative secant plus C. They also discuss the importance of correctly applying the signs when integrating these functions and provide additional examples to illustrate the process of integration for trigonometric functions.
📝 The Process of Integration and Handling Complex Functions
The instructor discusses the process of integrating complex functions and the importance of fitting the function to be integrated into the integration table. They provide examples of how to manipulate functions, such as breaking them down into simpler components or using trigonometric identities, to make them fit the table. The instructor also emphasizes the importance of understanding the properties of integrals, such as the ability to separate integrals by addition and subtraction but not by multiplication and division.
🎯 Solving Differential Equations and Initial Value Problems
The instructor explores the concept of solving differential equations and initial value problems, which involve finding a function whose derivative matches a given function. They demonstrate how to integrate the given function to find the family of solutions and then use the initial value to solve for the constant of integration. The instructor uses examples to illustrate the process and emphasizes the importance of understanding the relationship between derivatives and integrals in solving these problems.
🚀 Applying Calculus to Physics: The Catapult Problem
The instructor applies the concepts of calculus to a physics problem involving a catapult. They discuss how to find the position function of a projectile launched straight up with an initial velocity, taking into account the effects of gravity. The instructor outlines the steps to determine the velocity and acceleration functions and then uses these to find the position function. They also explain how to calculate the maximum height reached by the projectile and the time it takes to hit the ground.
📉 Projectile Motion and Calculating Time of Flight
The instructor continues the discussion on projectile motion, focusing on calculating the time it takes for the projectile to hit the ground. They explain the process of setting the position function equal to zero to find the time of flight and emphasize the importance of using the correct time value from the quadratic formula. The instructor also discusses the practical implications of the calculations, such as the height reached by the projectile and the total time it spends in the air.
Mindmap
Keywords
💡Derivative
💡Integral
💡Rectangular Method
💡Anti-derivative
💡Area Under the Curve
💡Limit
💡Definite Integral
💡Indefinite Integral
💡Integration
💡Plus C
Highlights
Introduction to Chapter 4, which shifts focus from derivatives to a new concept.
Discussion of the two main problems in calculus: finding the slope of a curve at a point and calculating the area under a curve.
Introduction of the rectangular method for approximating the area under a curve.
Explanation of creating rectangles within a graph to estimate the area under a curve.
Different methods for determining the height of rectangles: left endpoint, right endpoint, and midpoint.
The concept that increasing the number of rectangles improves the approximation of the area under a curve.
Introduction of anti-derivatives as a method for finding the area under a curve.
The relationship between anti-derivatives and the original function, where the derivative of the area function yields the original function.
The process of undoing a derivative to find the area function, known as anti-differentiation.
An example using the function f(x) = x + 1 to demonstrate the concept of anti-derivatives.
The importance of the constant C in anti-derivatives, representing a family of curves.
Understanding the indefinite integral as a representation of the area function without specific boundaries.
The notation and process of integration, synonymous with finding the anti-derivative.
Explanation of the integral symbol and its role in indicating the variable of integration.
The concept of the integral as a sum, especially when dealing with infinite rectangles.
Introduction to the basic integration table and its importance for finding anti-derivatives.
The process of memorizing the integration table to facilitate the anti-differentiation process.
An example of integrating a polynomial function and the importance of fitting the function to the integration table.
The properties of integrals, such as the ability to factor out constants and the separation of integrals by addition and subtraction.
The limitations of integrals, such as the inability to split up integrals of multiplied functions without distribution.
The introduction of integration by substitution as a method to handle more complex integrals.
An example of a real-life physics problem involving a projectile launched from a catapult to illustrate the application of integration.
Transcripts
Browse More Related Video

Calculus 1 Lecture 4.3: Area Under a Curve, Limit Approach, Riemann Sums

BusCalc 13.4 Riemann Sums
Mastering Calculus: An Introduction to Integrals
Calculus AB/BC – 6.7 The Fundamental Theorem of Calculus and Definite Integrals

Antiderivatives

Calculus (Version #2) - 9.1 The Second Fundamental Theorem of Calculus
5.0 / 5 (0 votes)
Thanks for rating: