Chain Rule Day 1

Chad Gilliland
15 Sept 201307:49
EducationalLearning
32 Likes 10 Comments

TLDRThe video explains the chain rule in calculus, focusing on finding the derivative of composite functions. It starts by distinguishing between plain and composite functions and then presents the general chain rule formula. The instructor provides several examples, including polynomial and trigonometric functions, illustrating how to apply the chain rule step-by-step. The video also covers special cases such as using the quotient rule and handling more complex nested functions. By the end, viewers should have a solid understanding of the chain rule and its application in different scenarios.

Takeaways
  • 📚 The video discusses the chain rule in calculus, which is used to find the derivative of a composite function.
  • 🔍 Composite functions typically involve parentheses and can be tricky, especially with trigonometric functions where the notation might differ.
  • 📈 The general chain rule formula is given as \( (f(g(x)))' = f'(g(x)) \cdot g'(x) \), where \( f \) is the outer function and \( g \) is the inner function.
  • 🔢 Examples are provided to illustrate how to apply the chain rule, starting with taking the derivative of the outer function and then multiplying by the derivative of the inner function.
  • 📉 In the example of \( 5 \cdot 4x^3 + 3x - 2 \) to the fourth power, the outer function is simplified first, leaving the inner function untouched.
  • 🌐 Another example involves rewriting expressions to make the application of the chain rule clearer, such as moving a constant from the numerator to the denominator.
  • 📌 The quotient rule is also mentioned, where the derivative of a fraction involves both the numerator and the denominator's derivatives.
  • 📊 Trigonometric functions are highlighted, showing how the derivative of cosine is negative sine, and the derivative of secant is secant tangent.
  • 🔎 The script emphasizes the importance of keeping the inner function unchanged during the derivative process, as seen in examples like \( \cos(3x) \) and \( \sec(4x^3 + 5) \).
  • 🔗 The concept of hidden functions is introduced, where the chain rule might need to be applied multiple times, as in \( \cos(5x) \) raised to the fourth power.
  • 🔑 The final example demonstrates how to find the derivative of a function at a specific point by first finding the derivative in general and then substituting the point.
Q & A

  • What is the chain rule in calculus?

    -The chain rule is a fundamental theorem in calculus used to find the derivative of a composite function, which is a function composed of two or more functions.

  • Why is it important to distinguish between plain functions and composite functions when finding derivatives?

    -Plain functions are simpler and their derivatives can be found using basic rules, whereas composite functions require the application of the chain rule to correctly determine their derivatives.

  • What is an example of a composite function that does not explicitly show parentheses?

    -An example given in the script is y = cos(x^3), where the cosine function is inside the x^3 function, even though the parentheses are not written.

  • How is the chain rule expressed mathematically in the script?

    -The chain rule is expressed as (f(g(x)))' = f'(g(x)) * g'(x), where f' denotes the derivative of the outer function and g' denotes the derivative of the inner function.

  • What is the process for finding the derivative of a function raised to a power?

    -First, apply the power rule to the outside function, then multiply by the derivative of the inside function or the inside quantity.

  • Can you provide an example of using the chain rule for a function with a power of 4?

    -The script provides an example: (5 * (4x^3 + 3x - 2)^4)' = 5 * 4 * (4x^3 + 3x - 2)^3 * (12x^2 + 3).

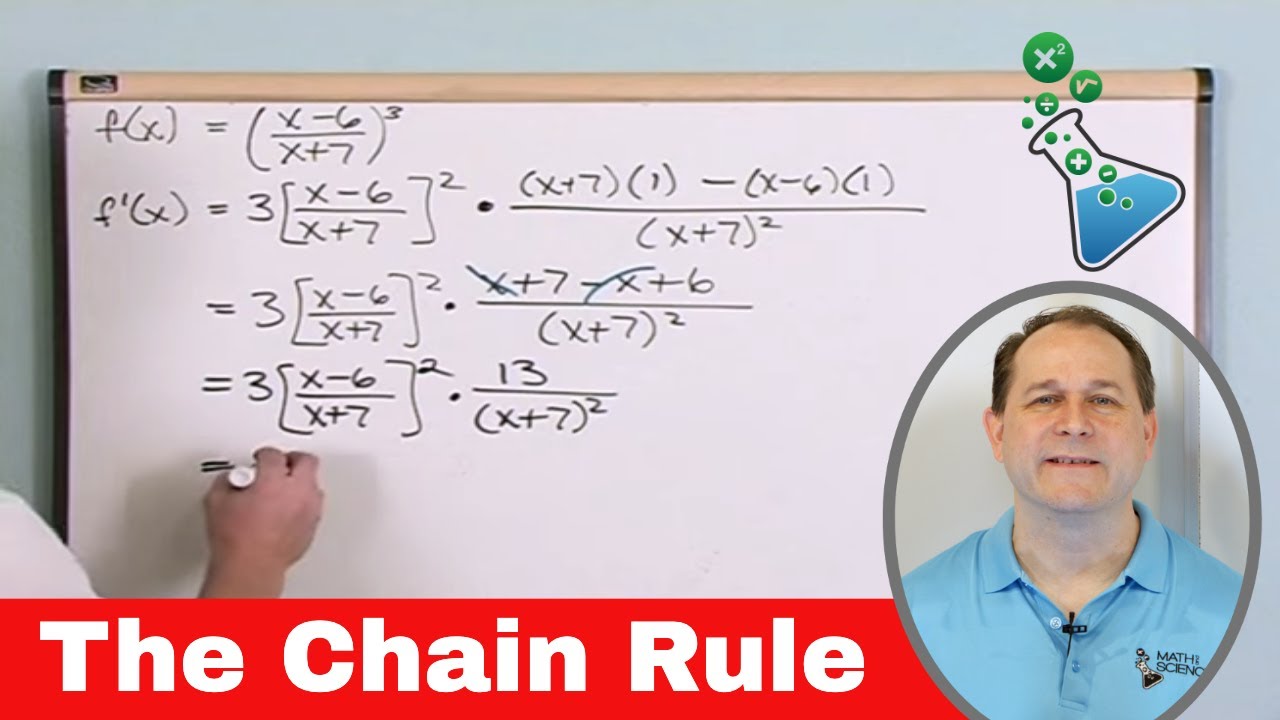
  • How does the chain rule apply when rewriting a function with a quotient?

    -The quotient rule can be combined with the chain rule. The derivative of the quotient is the derivative of the numerator times the denominator minus the numerator times the derivative of the denominator, all divided by the square of the denominator.

  • What is the derivative of the function cos(3x) using the chain rule?

    -The derivative is -3sin(3x), which is found by taking the derivative of the cosine function and multiplying by the derivative of the inside function, 3x.

  • How is the chain rule applied when there are hidden composite functions?

    -In cases where the composite nature of the function is not immediately obvious, the function may need to be rewritten to clearly identify the inner and outer functions, and then the chain rule is applied accordingly.

  • Can you explain the process of finding H'(3) given a function H(x) = f(g(x))?

    -First, find H'(x) using the chain rule, which is f'(g(x)) * g'(x). Then, substitute x with 3 to find H'(3), which is f'(g(3)) * g'(3).

  • What is the final example in the script demonstrating the process of finding a derivative at a specific point?

    -The final example finds H'(3) for H(x) = f(g(x)), where g(x) = 4x^3 + 5 and f(x) = x^2. The process involves finding g'(x) and f'(x), then substituting x with 3 to calculate H'(3).

Outlines
00:00
📚 Introduction to the Chain Rule

This paragraph introduces the concept of the chain rule in calculus, which is essential for finding the derivative of composite functions. The speaker explains the difference between plain functions and composite functions, often indicated by parentheses. An example of a composite function is given with trigonometric functions, such as y = cos(3x). The general chain rule formula is presented, and the process of finding the derivative by first taking the derivative of the outer function and then multiplying by the derivative of the inner function is described. The paragraph also includes an example of applying the chain rule to a function raised to a power, emphasizing the importance of leaving the inner function untouched during this process.

05:01
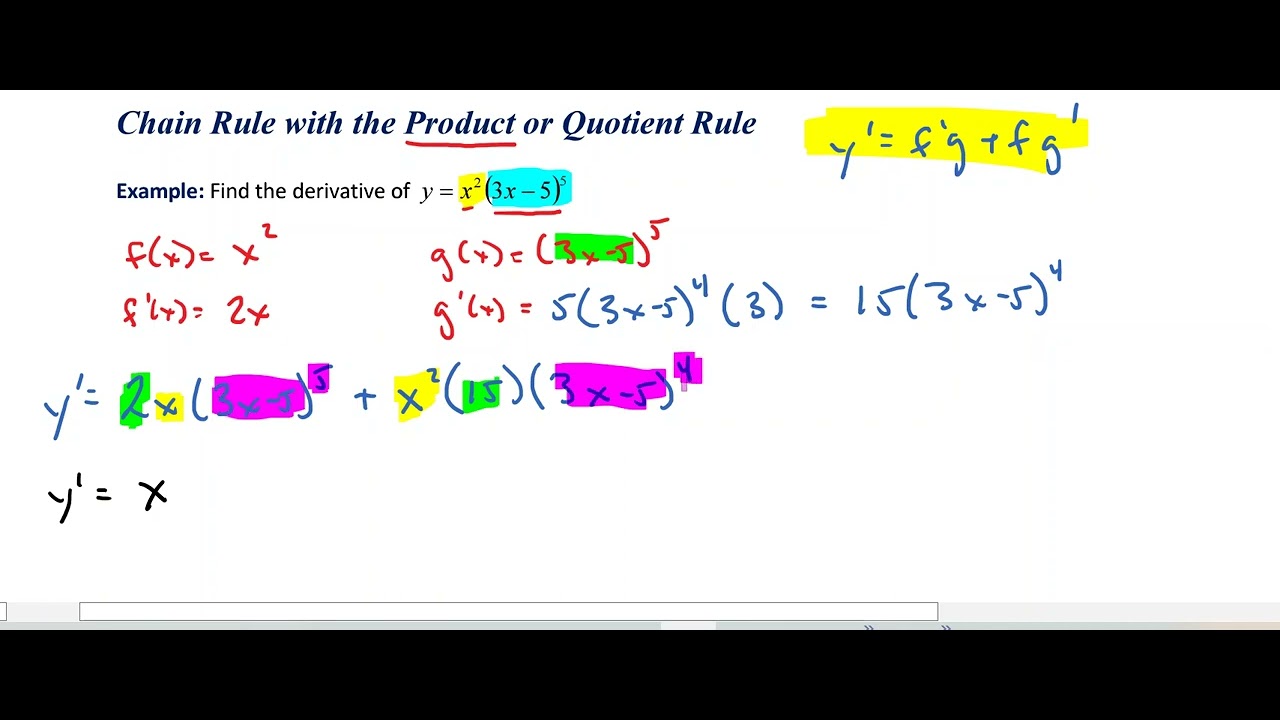
🔍 Applying the Chain Rule to Various Functions

The second paragraph delves deeper into applying the chain rule to different types of functions, including those with powers, quotients, and trigonometric functions. The speaker provides step-by-step examples of how to apply the chain rule, such as bringing down coefficients and using the power rule, as well as dealing with more complex scenarios like nested functions. The importance of rewriting expressions to simplify the application of the chain rule is highlighted, and the process of using the quotient rule in conjunction with the chain rule is demonstrated. The paragraph concludes with examples of trigonometric functions and how to handle hidden composite functions, emphasizing the need for careful rewriting and application of the chain rule.

Mindmap
Keywords
💡Chain Rule
The Chain Rule is a fundamental principle in calculus for finding the derivative of a composite function. It states that the derivative of a function composed of two functions is the derivative of the outer function evaluated at the inner function, multiplied by the derivative of the inner function. In the video, the Chain Rule is the central theme, with various examples demonstrating its application, such as '5 * 4X Cub + 3x - 2 to the 4th power'.
💡Composite Function
A composite function is a function that is formed by applying one function to the result of another function. In the script, composite functions are identified by the presence of parentheses or, in the case of trigonometric functions, by the nature of the function itself, such as 'y's cosine cubed of X'.
💡Derivative
The derivative in calculus represents the rate at which a function changes with respect to one of its variables. The video script discusses finding derivatives of various functions, including composite functions, using the Chain Rule, as seen in the example 'the derivative of the outside function which is 5 * 4X Cub + 3x - 2 to the 4th power'.
💡Power Rule
The Power Rule is a basic principle in calculus for differentiating functions of the form f(x) = x^n, where n is a constant. The rule states that the derivative of such a function is n times the function itself, reduced by one power. In the video, the Power Rule is applied when dealing with functions raised to a power, such as 'a function to a power then you do your regular power rule'.
💡Trigonometric Functions
Trigonometric functions are mathematical functions that relate the angles of triangles to the lengths of their sides. In the script, these functions, such as cosine and sine, are used within composite functions, and their derivatives are calculated using the Chain Rule, for example, 'cosine of 3x' where the derivative involves both the derivative of cosine and the derivative of the inside function, 3x.
💡Quotient Rule
The Quotient Rule is a method in calculus for finding the derivative of a quotient of two functions. The video mentions the Quotient Rule in the context of rewriting a complex fraction to apply the Chain Rule, as seen in the example 'x^2 + 2 to the 1/3 power' where the rule is used to find the derivative of a quotient that has been rewritten.
💡Exponentiation
Exponentiation is the operation of raising a number to the power of another number. In the video, exponentiation is discussed in the context of derivatives, particularly when dealing with functions raised to various powers, such as 'a function to a power' where the Power Rule is applied after using the Chain Rule.
💡Differentiation
Differentiation is the process of finding the derivative of a function. The entire video is focused on teaching differentiation, specifically using the Chain Rule for composite functions, as illustrated in the various examples provided throughout the script.
💡Secant
Secant is a trigonometric function that is the reciprocal of the cosine function. In the script, the derivative of the secant function is discussed, which is secant tangent, as seen in the example 'secant of 4X Cub + 5 all squared'.
💡Tangent
Tangent is a trigonometric function that represents the ratio of the length of the opposite side to the length of the adjacent side in a right-angled triangle. The video mentions the derivative of the tangent function, which is secant, in the context of differentiating composite functions, such as 'tangent of sin x'.
💡Cosecant
Cosecant is a trigonometric function that is the reciprocal of the sine function. The script includes an example of differentiating a cosecant function, where the derivative is negative cosecant times the derivative of the inside function, as in 'negative cosecant of 7 x 5 cent of 7 x 5th'.
Highlights

Introduction to the chain rule for functions within functions and how to find derivatives.

Explanation of the difference between plain functions and composite functions.

Composite functions often involve parentheses, especially with trigonometric functions.

General chain rule formula and its application in calculus.

Process of taking the derivative of a function to a power using the chain rule.

Example of finding the derivative of a composite function with a power.

Technique of leaving the inside function untouched during the derivative process.

Illustration of the derivative process with an example involving a power of a sum.

Use of the chain rule in rewriting and simplifying composite functions.

Application of the quotient rule in conjunction with the chain rule.

Trigonometric examples of the chain rule with functions like cosine and sine.

Complex example of nested functions and the use of the chain rule multiple times.

Explanation of the derivative of secant and tangent functions using the chain rule.

Final example involving finding the derivative of a function at a specific point.

Calculation of H Prime of 3 using the chain rule and substitution.

Conclusion and announcement of the next session.

Transcripts

Browse More Related Video

Calculus AB Lesson 3.4 The Chain Rule

The Chain Rule - Part 1

The Chain Rule

1.7 - The Chain Rule

The Chain Rule for Derivatives in Calculus - [1-5]

The Chain Rule - Part 2

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusChain RuleDerivativesMath TutorialComposite FunctionsMath PracticeTrig FunctionsEducationalStudent GuideLearning Math