Calculus AB Lesson 3.4 The Chain Rule
TLDRThis video provides a comprehensive lesson on the chain rule in calculus. It starts with an introduction to notation and basic derivative concepts, explaining how to find the derivative of linear equations. The instructor then delves into identifying inside and outside functions for composite functions and using the chain rule to find derivatives. Various examples and practice problems are worked through, illustrating different applications of the chain rule, including product and quotient rules. The video concludes with more complex examples to solidify the understanding of the chain rule.
Takeaways
- 📐 The chain rule helps find derivatives of composite functions.
- 📊 A linear equation like y = 2x + 3 has a constant slope of 2, representing the change in y with respect to x.
- 🔄 Derivatives can be represented using dy/dx for functions in terms of x and y, and other notations like dW/dT for different variables.
- 📈 Using Leibniz notation, the derivative of y = 3x^2 is dy/dx = 6x.
- 🔍 For the function w = tan(θ), the derivative is represented as dW/dθ and is equal to sec^2(θ).
- 🔢 When dealing with composite functions like h(x) = tan(2^x), identify the inner and outer functions to apply the chain rule.
- 🧮 The chain rule formula is dy/dx = (dy/du) * (du/dx), which simplifies the process of finding derivatives of composite functions.
- 🔗 For the function y = (2x + 3)^7, the chain rule helps avoid expanding the expression by identifying the inner and outer functions and their derivatives.
- 📝 To find the derivative of more complex functions like P(r) = 4√(r^6 + 2e^r), apply the chain rule by breaking down the inner and outer functions.
- 📘 The chain rule can be used with various functions, including trigonometric, exponential, and product or quotient functions.
Q & A
What is the definition of a derivative in terms of slope for a linear function?
-The derivative of a linear function, represented as dy/dx, is the constant rate of change or slope of the function. For example, in the equation y = 2x + 3, the slope is 2, indicating that as x increases by 1, y increases by 2.
How is the derivative notation different for functions with different variables?
-The derivative notation adapts to the variables used in the function. For instance, if the function is w = 2t + 3, the derivative is denoted as dw/dt, representing the change in w with respect to t.
How do you express the derivative of the function y = 3x^2 using Leibniz notation?
-For the function y = 3x^2, the derivative is expressed as dy/dx. Using the power rule, the derivative is dy/dx = 6x.
What is the derivative of w = tan(theta) with respect to theta?
-The derivative of w = tan(theta) with respect to theta is dw/d(theta) = sec^2(theta).
How do you find the derivative of a composite function like h(x) = tan(2^x)?
-To find the derivative of h(x) = tan(2^x), identify the inner function u = 2^x and the outer function f(u) = tan(u). The derivative is the product of the derivatives of these functions: dh/dx = sec^2(2^x) * 2^x * ln(2).
What is the derivative of a product of two functions, such as p(x) = 2^x * tan(x)?
-For the function p(x) = 2^x * tan(x), use the product rule: p'(x) = (d/dx of 2^x) * tan(x) + 2^x * (d/dx of tan(x)). This results in p'(x) = 2^x * ln(2) * tan(x) + 2^x * sec^2(x).
Explain the concept of inside and outside functions using the example r(x) = tan(x^2).
-For r(x) = tan(x^2), the inside function is u = x^2, and the outside function is f(u) = tan(u). The derivative is found by differentiating the outer function with respect to the inner function and then multiplying by the derivative of the inner function: dr/dx = sec^2(x^2) * 2x.
What is the chain rule and how is it applied?
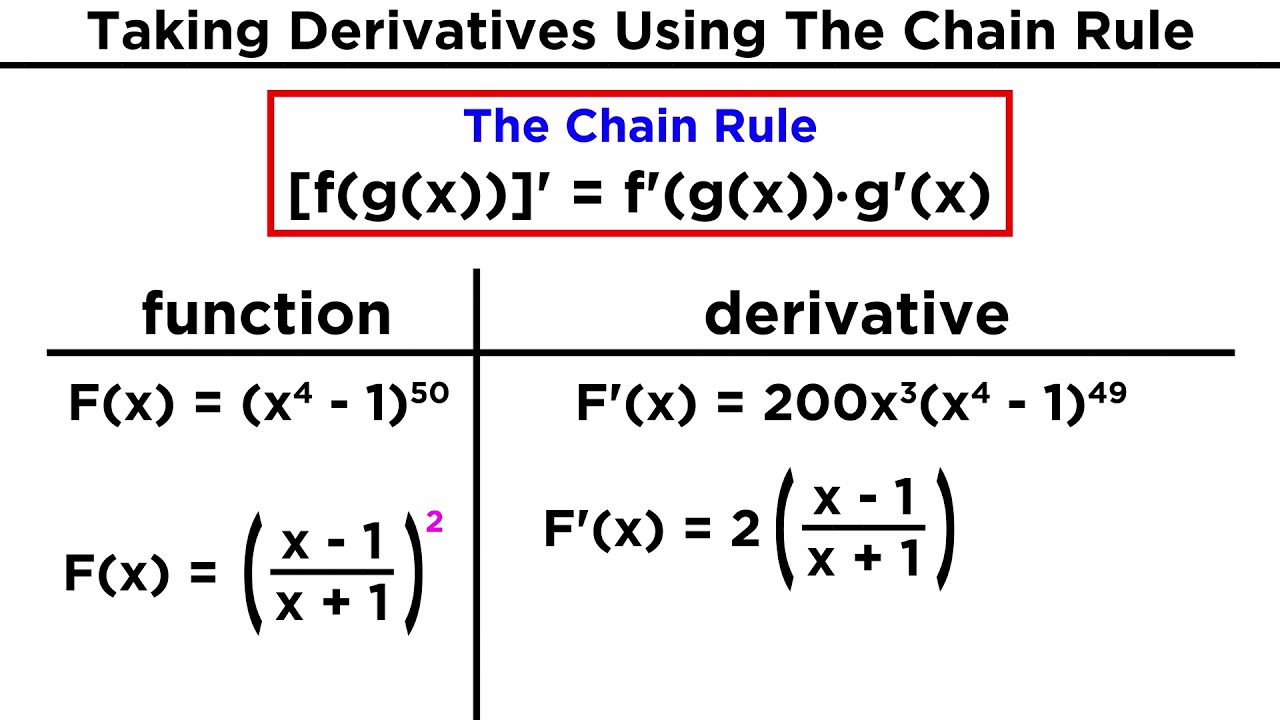
-The chain rule states that if h(x) = f(g(x)), then the derivative h'(x) = f'(g(x)) * g'(x). It is used when differentiating composite functions, where you multiply the derivative of the outer function evaluated at the inner function by the derivative of the inner function.
How would you differentiate the function y = (2x + 3)^7 using the chain rule?
-For y = (2x + 3)^7, identify the inner function u = 2x + 3 and the outer function f(u) = u^7. Applying the chain rule, dy/dx = 7(2x + 3)^6 * 2, resulting in dy/dx = 14(2x + 3)^6.
What is the importance of separating inside and outside functions in composite function differentiation?
-Separating inside and outside functions allows us to apply the chain rule effectively. It helps in identifying which parts of the function need to be differentiated and ensures that we apply the correct derivative rules to each part, simplifying the overall differentiation process.
Outlines
📐 Introduction to the Chain Rule
The video begins by introducing the concept of the chain rule, starting with basic notation. It uses the example of a linear equation y = 2x + 3 to explain the derivative, highlighting how the slope, represented as dy/dx, signifies the change in y with respect to x. The explanation extends to different variables, such as w = 2t + 3, using Leibniz notation to express derivatives of various functions, demonstrating the importance of correct symbols in notation.
🔍 Identifying Composite Functions
This section discusses how to identify composite functions and their components. It explains the process of determining the inner and outer functions using several examples. For instance, in the function h(x) = tan(2^x), 2^x is the inner function, and tan is the outer function. The section emphasizes the importance of separating these functions to apply the chain rule correctly and illustrates this with detailed examples.
🔗 Applying the Chain Rule
Here, the video delves into applying the chain rule to find derivatives. Using the example y = (2x + 3)^2, it shows how to find the derivative by expanding the function and using the power rule versus using the chain rule directly. The explanation includes defining inner and outer functions, finding their derivatives, and then combining them to apply the chain rule, highlighting the efficiency of this method.
🔢 More Examples of Composite Functions
This part provides additional examples of composite functions and their derivatives. It identifies the inside and outside functions in various scenarios, such as y = sqrt(2x - 1) and y = 1/(4x + 1), and explains how to apply the chain rule to find their derivatives. The section demonstrates the process of separating the functions and using the derivative rules effectively.
✂️ Simplifying Derivative Calculations
The video continues with more complex examples, focusing on simplifying derivative calculations using the chain rule. It covers functions like y = sin(2x) and y = cos^3(x), showing how to rewrite them to identify inner and outer functions. The explanation includes detailed steps to find the derivatives, emphasizing the importance of simplification in the calculation process.
🧩 Combining Derivative Rules
In this segment, the video integrates various derivative rules with the chain rule. It explores functions like y = e^(3x) and dz/dx = 12, dy/dx = 2, illustrating how to combine the rules to find derivatives. The section highlights the flexibility of the chain rule in dealing with different types of functions and the importance of understanding each component's derivative.
📊 Practical Application of the Chain Rule
This section focuses on the practical application of the chain rule in solving real-world problems. It presents an example of finding the equation for the tangent line to a curve at a specific point, detailing the steps to calculate the value of y and y' at that point. The explanation emphasizes the chain rule's utility in determining slopes and tangents in various contexts.
🔁 Multiple Chain Rule Applications
The video explores more complex scenarios involving multiple applications of the chain rule. It provides examples like P(r) = 4sqrt(r^6 + 2e^r) and M(e) = sin(e^2)cos(e^3), showing how to handle multiple layers of composite functions. The explanation includes detailed steps to break down each function and apply the chain rule iteratively.
🧮 Quotient Rule with Chain Rule
In this part, the video discusses the quotient rule combined with the chain rule. Using examples like H(y) = cos(10y)/(e^(4y) + 1), it demonstrates how to apply both rules to find derivatives. The section explains the process of taking derivatives of the numerator and denominator separately and then combining them using the quotient rule, incorporating the chain rule for composite components.
Mindmap
Keywords
💡Chain Rule
💡Derivative
💡Leibniz Notation
💡Composite Function
💡Power Rule
💡Inner Function
💡Outer Function
💡Product Rule
💡Quotient Rule
💡Trigonometric Functions
Highlights
Introduction to the chain rule and its notation.
Explanation of linear equations and constant rates of change.
Using Leibniz notation for expressing derivatives.
Deriving the slope of various functions using the power rule.
Identifying and separating inner and outer functions in composite functions.
Using product rule for derivatives of products of functions.
Examples of composite functions and their inner and outer functions.
Introduction to the chain rule for finding derivatives of composite functions.
Step-by-step example of using the chain rule for a composite function.
Comparison of expanding terms versus using the chain rule for complex functions.
Identifying inner and outer functions in various equations.
Explanation of the chain rule using both function notation and Leibniz notation.
Detailed examples applying the chain rule to different functions.
Finding derivatives of functions with multiple layers of composition.
Utilizing the quotient rule in combination with the chain rule.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: