Quantum Numbers
TLDRThis video explains the four quantum numbers that determine an electron's address in an atom: the principal quantum number (n), angular momentum quantum number (l), magnetic quantum number (ml), and electron spin (ms). It covers their relationships and how they define electron configurations in different energy levels and sublevels.
Takeaways
- 😀 Quantum numbers are essential in determining the location of an electron within an atom.
- 🔍 There are four quantum numbers: principal quantum number (n), angular momentum quantum number (l), magnetic quantum number (ml), and electron spin (ms).
- 📚 The principal quantum number (n) is an integer that describes the energy level of an electron and can range from 1, 2, 3, 4, and so forth.
- 🌐 The angular momentum quantum number (l) indicates the shape of the sublevel within an energy level and can be 0 (s), 1 (p), 2 (d), or 3 (f).
- 🔗 The relationship between n and l is such that l is always less than or equal to n-1.
- 🌀 The magnetic quantum number (ml) describes the orbital within a sublevel and can range from -l to +l.
- 🌟 The electron spin (ms) can be either +1/2 or -1/2, representing the direction of the electron's spin.
- 🚀 For the s sublevel, l is always 0, and ml can only be 0.
- 🌈 For the p sublevel, l is 1, and ml can be -1, 0, or 1.
- 📈 For the d sublevel, l is 2, and ml can range from -2 to +2.
- 🌠 For the f sublevel, l is 3, and ml can range from -3 to +3.
Q & A
What are quantum numbers and why are they important?
-Quantum numbers are a set of four numerical values that describe the state of an electron within an atom. They are important because they determine the energy, shape, orientation, and spin of an electron's orbital, essentially giving the electron a unique 'address' within the atom.
What is the principal quantum number (n) and how does it relate to energy levels?
-The principal quantum number (n) is an integer that describes the energy level of an electron. It can take values such as 1, 2, 3, 4, and so on. Higher values of n correspond to higher energy levels, with the first shell representing the first energy level (n=1), the second circle the second energy level (n=2), and so forth.
What is the angular momentum quantum number (l) and what does it describe?
-The angular momentum quantum number (l) describes the shape of the sublevel within an energy level. It is indicated by the symbol 'l' and can take values of 0 (s sublevel), 1 (p sublevel), 2 (d sublevel), and 3 (f sublevel). The value of l is less than or equal to n-1.
What is the relationship between n and l?
-The relationship between n and l is that l is always less than or equal to n-1. This means that as the principal quantum number increases, the possible values for the angular momentum quantum number also increase, allowing for more complex sublevels.
What are the different sublevels in an atom and how are they related to l?
-There are four main sublevels in an atom: s, p, d, and f. The s sublevel corresponds to l=0, the p sublevel to l=1, the d sublevel to l=2, and the f sublevel to l=3. Each sublevel has a distinct shape and number of orbitals.
Can the first energy level have a p sublevel?
-No, the first energy level does not have a p sublevel. According to the relationship between n and l, when n=1, l can only be 0, which corresponds to the s sublevel. Therefore, there is no p sublevel at the first energy level.
What is the magnetic quantum number (m_l) and how does it relate to orbitals within a sublevel?
-The magnetic quantum number (m_l) describes the orbital within a sublevel. It can take integer values between -l and +l. For example, when l=0 (s sublevel), m_l can only be 0; when l=1 (p sublevel), m_l can be -1, 0, or 1; and so on. Each m_l value corresponds to a specific orbital.
What is the electron spin quantum number (m_s) and what are its possible values?
-The electron spin quantum number (m_s) describes the intrinsic spin of an electron. It can have one of two values: +1/2 (spin up) or -1/2 (spin down). This quantum number is crucial in determining the electron's behavior in a magnetic field.
How many electrons can an orbital hold and why?
-An orbital can hold a maximum of two electrons. This is due to the Pauli Exclusion Principle, which states that no two electrons in an atom can have the same set of four quantum numbers. By having two electrons with opposite spins (+1/2 and -1/2), each orbital can be fully occupied.
What are the four quantum numbers for an electron in the 3d sublevel?
-For an electron in the 3d sublevel, the four quantum numbers are: n=3 (principal quantum number), l=2 (angular momentum quantum number, corresponding to the d sublevel), m_l can range from -2 to +2 (magnetic quantum number), and m_s can be either +1/2 or -1/2 (electron spin quantum number).
Outlines
🌐 Understanding Quantum Numbers
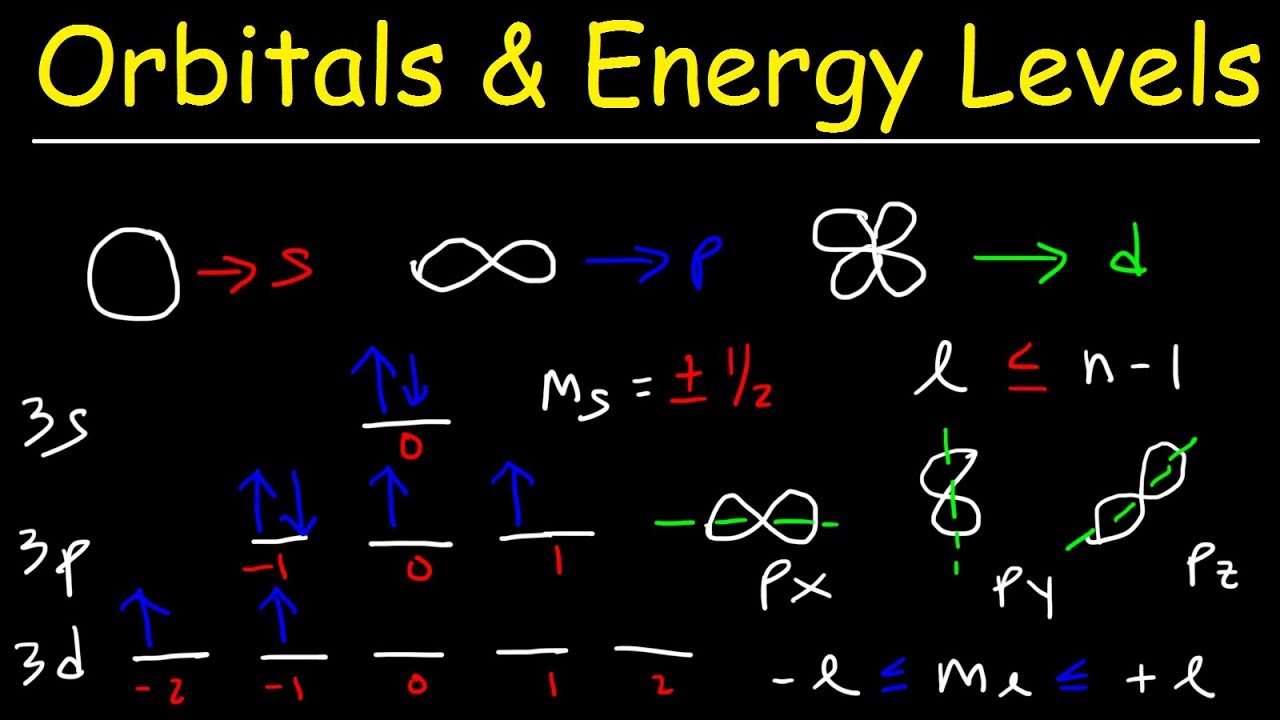
This paragraph introduces the concept of quantum numbers, which are essential for determining the location of an electron within an atom. There are four quantum numbers, each with a specific role. The principal quantum number (n) describes the energy level of an electron, with values like 1, 2, 3, etc. The angular momentum quantum number (l) indicates the shape of the sublevel, with s (l=0), p (l=1), d (l=2), and f (l=3) sublevels. The relationship between n and l is such that l is less than or equal to n-1. The video also discusses the absence of certain sublevels at specific energy levels, such as the lack of a p sublevel in the first energy level or a 2d sublevel in the second energy level.
🔍 Exploring the Magnetic Quantum Number
This paragraph delves into the magnetic quantum number (m_l), which describes the orbital within a sublevel. The number of orbitals in a sublevel depends on the value of l: s has 1, p has 3, d has 5, and f has 7 orbitals. The values of m_l range from -l to +l, with specific values for each sublevel (e.g., p has m_l values of -1, 0, 1). The paragraph also explains the relationship between l and m_l, emphasizing that m_l is an integer within the range of -l to +l. Examples are given for the 1s, 2p, and 3d sublevels, illustrating how m_l values correspond to specific orbitals.
🌀 Electron Spin and Quantum Number Configuration
The final paragraph discusses the electron spin quantum number (m_s), which can have two values: +1/2 or -1/2, representing the spin direction of an electron. The paragraph explains how the electron spin can be either up or down, affecting the m_s value. It also provides an example of the 3d sublevel, detailing how the eight electron can have different m_l and m_s values depending on its orbital and spin direction. The video concludes with a summary of the quantum numbers and encourages viewers to explore additional resources for a deeper understanding of the topic.
Mindmap
Keywords
💡Quantum Numbers
💡Pauli Exclusion Principle
💡Principal Quantum Number (n)
💡Angular Momentum Quantum Number (l)
💡Sublevels
💡Magnetic Quantum Number (m_l)
💡Electron Spin (m_s)
💡Orbital
💡Electron Configuration
💡Energy Levels
💡Bohr Model
Highlights
Four quantum numbers are essential for understanding the address of an electron inside an atom.
The Pauli Exclusion Principle states that no two electrons can have the same set of four quantum numbers.
The principal quantum number (n) describes the energy level of an electron and can be an integer starting from 1.
The energy levels are represented by concentric circles in the Bohr model of the atom.
The angular momentum quantum number (l) describes the shape of the sublevel within an energy level.
Sublevels include s (l=0), p (l=1), d (l=2), and f (l=3).
The relationship between n and l is such that l is less than or equal to n-1.
For n=1, l can only be 0; for n=2, l can be 0 or 1; for n=3, l can be 0, 1, or 2; for n=4, l can be 0, 1, 2, or 3.
The first energy level does not have a p sublevel, and the third energy level does not have an f sublevel.
An electron in the 3d sublevel has n=3 and l=2.
An electron in the 4f sublevel has n=4 and l=3.
The magnetic quantum number (m_l) describes the orbital within a sublevel.
The range of m_l is from -l to +l, and it determines the specific orbital.
For s sublevel (l=0), m_l can only be 0.
For p sublevel (l=1), m_l can be -1, 0, or 1.
For d sublevel (l=2), m_l can be -2, -1, 0, 1, or 2.
For f sublevel (l=3), m_l can be -3, -2, -1, 0, 1, 2, or 3.
The electron spin quantum number (m_s) can be either +1/2 or -1/2, representing upspin or downspin.
Each orbital can hold a maximum of two electrons with opposite spins.
For the 3d8 electron, the quantum numbers are n=3, l=2, m_l=0, and m_s can be either +1/2 or -1/2.
Transcripts
Browse More Related Video
Orbitals, Atomic Energy Levels, & Sublevels Explained - Basic Introduction to Quantum Numbers
Quantum Numbers, Atomic Orbitals, and Electron Configurations

7.4 Quantum Numbers | High School Chemistry
Using the Periodic Table to Determine Quantum Numbers - Chemistry Practice Problems

SPDF orbitals Explained - 4 Quantum Numbers, Electron Configuration, & Orbital Diagrams

Lecture 13 - Quantum numbers
5.0 / 5 (0 votes)
Thanks for rating: