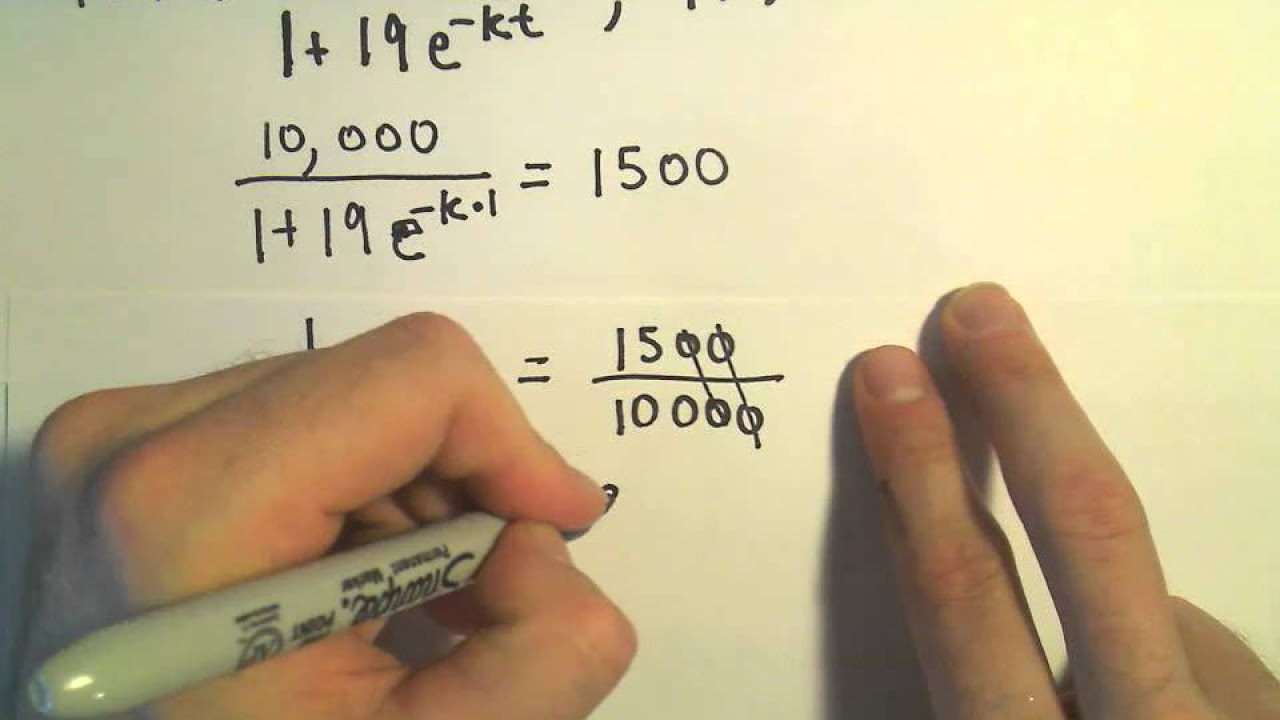AP Precalculus Practice Exam Question 83

NumWorks
23 May 202303:38
EducationalLearning
32 Likes 10 Comments

TLDRThe transcript describes a mathematical problem involving two functions, K and M, modeling sales of a product at grocery stores. K(T) is a logarithmic function, and M(T) is a linear function, both predicting sales in thousands of units after T weeks. The task is to find the first time T when the logarithmic model predicts sales 0.1 thousand units more than the linear model. The solution process involves setting up an equation, K(T) - M(T) = 0.1, and solving it using an equation solver. The result shows that the first time this condition is met is at T = 4.324 weeks, with the model only applicable for T greater than or equal to 2.

Takeaways
  • 📈 The transcript discusses a problem involving two functions, K and M, representing sales of a product in grocery stores over time.
  • 🔍 K(T) is a logarithmic function, and M(T) is a linear function, both predicting sales in thousands of units after T weeks for T > 2.
  • 📝 The goal is to find the first time T when the sales predicted by the logarithmic model exceed those predicted by the linear model by 0.1 thousand units.
  • 🧐 The difference between the two models, K(T) - M(T), is set to equal 0.1 to find the time T when the sales predictions differ by the specified amount.
  • 📑 The transcript mentions the use of an equation solver to find the value of T that satisfies the condition.
  • 🔢 The transcript provides an example of how to input the expressions for K(T) and M(T) into an equation solver.
  • 🔎 The transcript specifies that the models are only valid for T values greater than or equal to 2.
  • 📊 The transcript suggests setting a range for T from 2 to 10 to find the solution for the first time the condition is met.
  • 🔑 The transcript identifies the first time T as 4.324, which is the solution to the equation within the specified range.
  • 🎯 The answer to the problem is indicated as 'Choice B', which corresponds to the value of T found.
  • 📝 The transcript emphasizes the importance of correctly setting up and solving the equation to find the specific time when the sales predictions differ by 0.1 thousand units.
Q & A

  • What is the context of the problem described in the transcript?

    -The problem involves two functions, K(T) and M(T), which model the sales of a product in grocery stores over T weeks. K(T) is a logarithmic function, and M(T) is a linear function, both representing sales in thousands of units for T greater than two.

  • What is the goal of the problem?

    -The goal is to find the first time T when the sales predicted by the logarithmic model (K(T)) are 0.1 thousand units more than those predicted by the linear model (M(T)).

  • What is the mathematical representation of the difference between the two models?

    -The difference is represented by the equation K(T) - M(T) = 0.1, where K(T) is the logarithmic model and M(T) is the linear model.

  • What is the specific logarithmic function mentioned in the transcript?

    -The specific logarithmic function is not fully provided in the transcript, but it is mentioned to have a form of 14 - 2.885 times the natural logarithm of T.

  • What is the specific linear function mentioned in the transcript?

    -The specific linear function is also not fully provided, but it is implied to be a function of T that is subtracted from the logarithmic model in the equation.

  • Why is the minimum value for T set to 2 in the problem?

    -The models are only valid for T greater than or equal to two, which is a given condition in the problem.

  • What is the method used to solve the equation for T?

    -The method used is an equation solver, which is a tool that can find the values of T that satisfy the equation K(T) - M(T) = 0.1.

  • What is the range of T values considered in the problem?

    -The range of T values considered is from 2 to 10, as stated in the transcript.

  • What is the first time T when the condition is met according to the transcript?

    -The first time T when the logarithmic model predicts sales 0.1 thousand units more than the linear model is at T = 4.324.

  • What does the answer 'Choice B' refer to in the context of the transcript?

    -In the context of the transcript, 'Choice B' likely refers to the answer choice in a multiple-choice question related to the problem, which in this case is T = 4.324.

  • What is the significance of the number 0.1 in the problem?

    -The number 0.1 represents the difference in sales, in thousands of units, between the logarithmic and linear models that the problem is trying to find.

Outlines
00:00
📈 Logarithmic vs. Linear Sales Model Comparison

This paragraph discusses a problem involving two functions, K(T) and M(T), representing the sales of a product in thousands of units at grocery stores after T weeks. K(T) is modeled by a logarithmic function, and M(T) by a linear function. The task is to find the first time T when the sales predicted by the logarithmic model exceed those of the linear model by 0.1 thousand units. The approach involves setting up an equation where K(T) - M(T) equals 0.1 and solving for T, considering that the models are valid for T greater than two. The solution process involves using an equation solver with a specified range and finding the first instance where the condition is met, which is at T = 4.324, corresponding to answer choice B.

Mindmap
Keywords
💡Logarithmic Model
A logarithmic model is a mathematical representation that uses logarithmic functions to describe the relationship between variables. In the context of the video, it represents the sales of a product at a group of grocery stores, where sales are modeled as a function of time, with the natural logarithm of time being a key component. The script mentions 'K of T is equal to this natural log function,' indicating that the sales are expected to increase at a decreasing rate over time, which is a common characteristic of logarithmic growth.
💡Linear Model
A linear model is a type of mathematical model that represents a direct relationship between two variables, where the change in one variable leads to a proportional change in the other. In the video script, 'M of T is equal to this linear function,' which suggests that the sales are expected to increase at a constant rate as time progresses. This is in contrast to the logarithmic model, where the rate of increase slows down over time.
💡Sales
Sales in the video refer to the quantity of a product that is sold at a group of grocery stores. The script discusses two different models predicting sales in thousands of units after a certain number of weeks. The term is central to the video's theme, as it is the subject of the mathematical models being compared and contrasted.
💡Weeks
Weeks is a unit of time used in the video to measure the duration over which sales are being analyzed. The script specifies 'after T weeks,' indicating that the models are predicting sales over a period that starts at two weeks and extends beyond. The term is crucial for understanding the time frame of the sales predictions.
💡Natural Logarithm
The natural logarithm, often denoted as 'ln', is a logarithm to the base 'e', where 'e' is an irrational number approximately equal to 2.71828. In the script, the natural logarithm of 'T' is used in the logarithmic model to represent the sales of the product. It is an essential part of the model, as it captures the diminishing returns in sales growth over time.
💡Difference
In the context of the video, the difference refers to the discrepancy between the sales predicted by the logarithmic model and the linear model. The script states the need to find when 'K of T minus M of T' equals 0.1, indicating a specific point in time where the logarithmic model predicts 100 units more in sales than the linear model.
💡Equation Solver
An equation solver is a tool used to find the values of variables that make an equation true. In the script, the presenter uses an equation solver to find the value of 'T' when the sales predicted by the two models differ by 0.1 thousand units. This tool is integral to solving the problem presented in the video.
💡Execute
To execute, in the context of the video, means to run or perform a calculation or operation. The script mentions 'I'm going to hit execute,' referring to the action of running the equation solver to find the solution for 'T'. This action is a key step in determining the first time the sales models predict a difference in sales.
💡Solution
A solution in the video refers to the value of 'T' that satisfies the condition set by the equation. The script discusses finding the 'first time' or 'only one time' when the sales models predict a difference of 0.1 thousand units, which is the solution to the equation presented.
💡Range
The range in the video is the set of values within which the solution for 'T' is expected to be found. The script specifies a range from 2 to 10, indicating that the presenter expects the solution to lie within this interval. This range is set based on the conditions of the models, which are only valid for 'T' greater than or equal to two.
💡First Time
The 'first time' in the video refers to the initial instance at which the condition of the logarithmic model predicting 0.1 thousand units more in sales than the linear model is met. The script emphasizes finding this specific point in time, which is determined to be at '4.324' weeks.
Highlights

Two functions, K(T) and M(T), are constructed to represent sales of a product at grocery stores.

K(T) and M(T) represent sales in thousands of units after T weeks for T > 2.

K(T) is modeled by a natural logarithm function.

M(T) is modeled by a linear function.

The goal is to find the first time T when K(T) is 0.1 thousand units more than M(T).

The difference K(T) - M(T) is set to equal 0.1 to find the desired time T.

Equation solver is used to solve for T when the difference between models equals 0.1.

K(T) is expressed as 14 - 2.885 * ln(T).

M(T) is expressed within the equation, grouped with parentheses.

The equation is set up to solve for the first time the sales models predict the specified difference.

The models are only valid for T greater than or equal to 2.

The range for solving the equation is set from 2 to 10.

The equation solver finds one solution for T in the specified range.

The first time T when the sales models differ by 0.1 thousand units is at T = 4.324.

The answer to the problem is Choice B, indicating the time T = 4.324.

Transcripts

Browse More Related Video

2023 AP Calculus AB & BC FRQ #3

Solving the logistic differential equation part 2 | Khan Academy

AP Calc BC: How to Solve a Logistic Growth Application Problem

Average Value of a Function

The Logistic Equation and Models for Population - Example 1, part 1

2022 AP Calculus AB Free Response #6

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Sales AnalysisLogarithmic ModelLinear ModelMath EquationEquation SolverSales PredictionProduct SalesGrocery StoresSales GrowthModel ComparisonFirst Occurrence