Average Value of a Function
TLDRIn this educational video, Professor Moana explains the concept of finding the average value of a function with a practical application. Using the example of weekly sales modeled by the function s(t) = 4e^t, she demonstrates how to calculate the average weekly sales for weeks two through five. The process involves integrating the function over the given interval (from 1 to 5, representing weeks one to five) and then dividing by the number of weeks considered. The integral of e^t is e^t, leading to the final calculation of (e^5 - e^1)/4. After evaluating this expression, the average sales per week are approximately $14,569.49. The video emphasizes the importance of understanding the integral as a method to find totals and then using division to find averages, a concept applicable to various units beyond just sales figures.
Takeaways
- 🧮 To find the average value of a function over an interval, you calculate the integral of the function over that interval and then divide by the length of the interval.
- 📈 The total sales for a given period can be modeled by the area under the sales curve, which is found using integration.
- ⌛ The integral for calculating the total sales over weeks two through five is from 1 to 5, not from 2 to 5, due to the way intervals are defined.
- 🔢 The average is obtained by dividing the total integral by the number of weeks, which in this case is the difference between 5 and 1, giving us 4 weeks.
- 📐 The general formula for the average value of a function from 'a' to 'b' is (1/(b-a)) * ∫(from a to b) f(t) dt.
- 📝 When integrating the function s(t) = 4e^t, the antiderivative is 4e^t.
- 🧵 The definite integral from 1 to 5 of 4e^t is calculated as 4e^5 - 4e^1.
- 🤑 Factoring out constants from the integral can simplify the calculation, and in this case, the constant 4 can be factored out and then canceled out.
- 🧮 The exact answer from the integration is e^5 - e^1, which needs to be calculated using a calculator for a numerical value.
- 💡 It's important to remember that e^0 equals 1, not e^1, which is a common mistake when calculating with the exponential function.
- 💵 The calculated average sales per week can be interpreted in units or converted to a more practical measure like hundreds of dollars if needed.
- 📊 Understanding the concept of integration and how it applies to finding averages is crucial for solving such problems.
Q & A
What is the general approach to finding the average value of a function?
-The general approach to finding the average value of a function involves calculating the total by integrating the function over the interval of interest and then dividing by the number of units or the length of the interval.
How is the total sales for a certain period represented in the context of the given problem?
-The total sales for a certain period is represented by the area under the sales curve, which is calculated by integrating the sales function over the time period of interest.
Why do we start the integral from '1' instead of '2' when calculating the average weekly sales for weeks two through five?
-We start the integral from '1' instead of '2' because the interval '1 to 5' encompasses the first five weeks of sales, with '1 to 2' representing the second week, '2 to 3' the third week, and so on, up to the fifth week.
What is the formula used to calculate the average value of a function over an interval [a, b]?
-The formula used to calculate the average value of a function over an interval [a, b] is (1/(b-a)) * ∫(from a to b) f(t) dt.
What is the sales function given in the script?
-The sales function given in the script is s(t) = 4e^t.
How do you find the anti-derivative of e^t?
-The anti-derivative of e^t is simply e^t, as e^t is its own integral.
What is the result of the integral of s(t) from 1 to 5?
-The result of the integral of s(t) = 4e^t from 1 to 5 is 4e^5 - 4e^1.
Why is it important to be careful when calculating e^0?
-It is important to be careful when calculating e^0 because e^0 equals 1, not 0, which is a common misconception when dealing with polynomials.
What is the final calculated average weekly sales for weeks two through five?
-The final calculated average weekly sales for weeks two through five is approximately $14,569.49.
How can the final average be adjusted if the sales were measured in hundreds of dollars?
-If the sales were measured in hundreds of dollars, you would multiply the final average by 100 to get the average in dollars.
What is the advice given for those who are working on similar problems?
-The advice given is to keep working on these problems, understanding the process, and to like and subscribe for more helpful content.
Outlines
📈 Calculating the Average Value of a Function
Professor Moana introduces the concept of finding the average value of a function, using the example of weekly sales modeled by the function s(t) = 4e^t. To find the average weekly sales for weeks two through five, one must calculate the integral of s(t) from t=1 to t=5, which represents the total sales over the specified weeks. The average is then found by dividing this total by the number of weeks, which is calculated as (5 - 1) or four weeks in this case. The integral of e^t is e^t, leading to the expression (1/4) * (4e^5 - 4e^1). After simplification, this results in e^5 - e^1. Using a calculator, the average sales per week are found to be approximately $14,569.49, demonstrating the process of integrating a function to find an average value over a specified interval.
📚 Generalizing the Process for Average Value Calculation
The second paragraph emphasizes the general approach to finding the average value of a function over an interval. It reiterates the process of integrating the function over the interval and then dividing by the number of units, in this case, weeks. The paragraph also suggests a notational convenience where the division can be factored out front as 1/(number of units), making the expression more compact. Professor Moana encourages viewers to continue practicing these calculations, offers a call to action to like and subscribe to the channel, and provides a simple method for subscribing by clicking on the video's thumbnail.
Mindmap
Keywords
💡Average Value
💡Function
💡Integral
💡Anti-Derivative
💡Exponential Function
💡Total Sales
💡Weeks
💡Calculator
💡Sales Modeling
💡Numerical Value
💡Units
Highlights
Professor Moana discusses the method to find the average value of a function with an application in weekly sales modeling.
The average is found by calculating the total and dividing by the number of units, analogous to calculating an average test score.
For a function, the total is the area under the curve, which is found by integrating the function over the interval of interest.
The integral of s(t) with respect to dt for weeks two through five is calculated from one to five, not two to five, due to the way weeks are counted.
The reason for starting the integral at one instead of two is to align with the first week of sales being from zero to one.
The general formula for the average sales is given by (1/(b-a)) * ∫[a, b] s(t) dt, where a and b are the limits of the interval.
The average weekly sales are calculated by dividing the total sales by the number of weeks, which is (5 - 1) in this case.
The antiderivative of e^t is e^t, which simplifies the integration process.
The exact answer for the average sales is e^5 - e^1, which is then calculated using a calculator for a numerical value.
It's important to remember that e^0 equals 1, not zero, when calculating the final numerical value.
The calculated average sales per week is 145.69 units, which can be converted to monetary value if needed.
The integral method is a standard approach to finding totals, but it requires division by the number of units for an average.
The formula can be rearranged for clarity and simplicity, making it easier to apply in different contexts.
Understanding the process is key to solving similar problems, emphasizing the importance of grasping the underlying concepts.
Professor Moana encourages viewers to like, subscribe, and engage with the content for more educational videos.
The video aims to help viewers understand the concept of finding the average value of a function and its practical applications.
Transcripts
Browse More Related Video

AP Precalculus Practice Exam Question 83
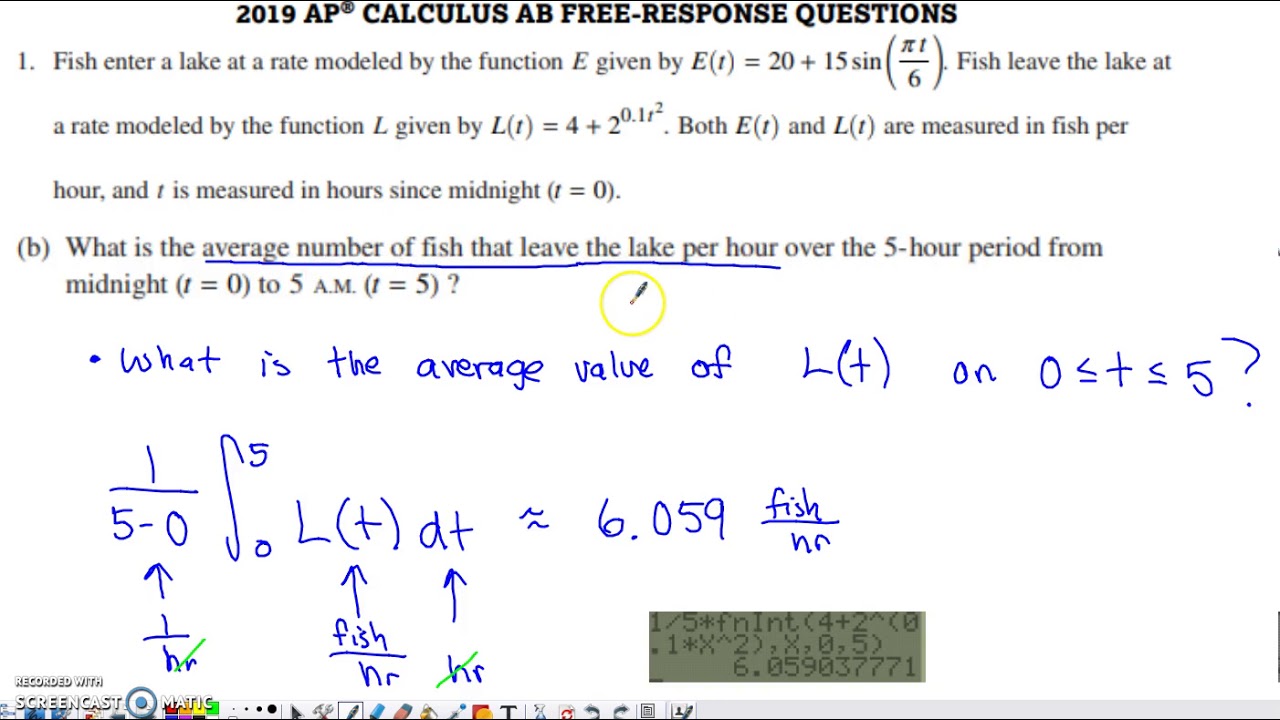
2019 AP Calculus AB & BC Free Response Question #1
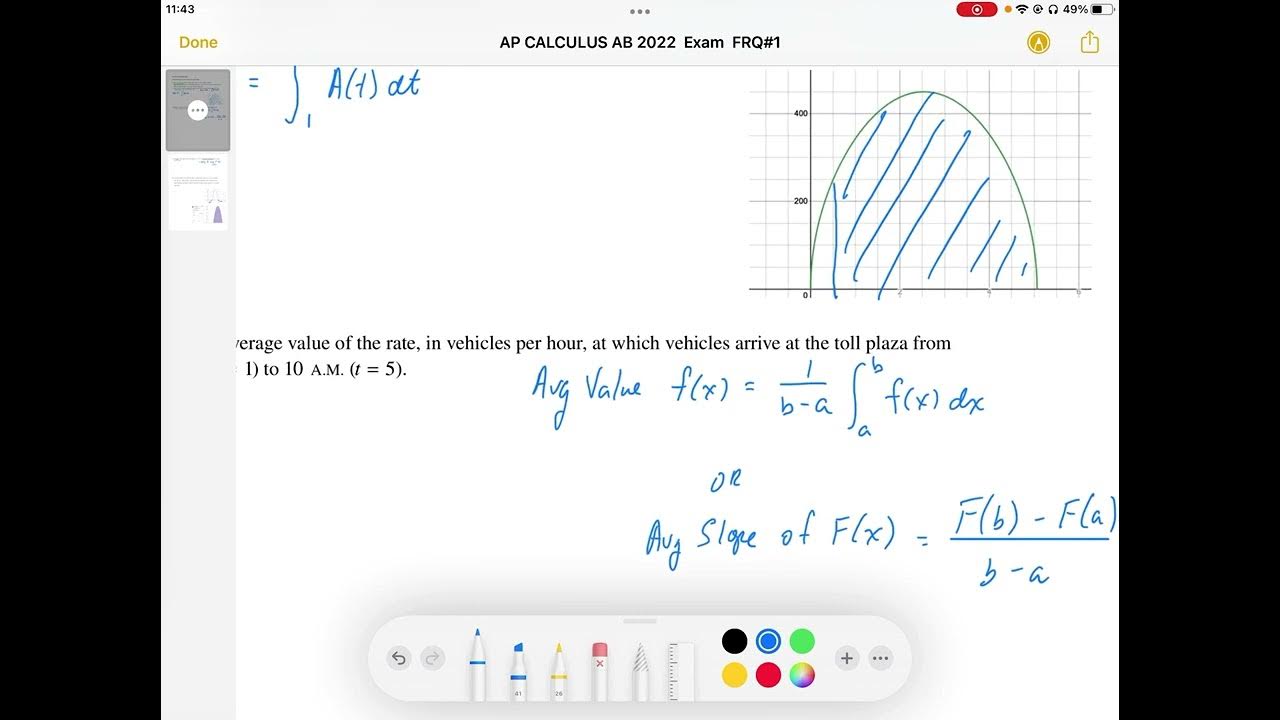
AP CALCULUS AB 2022 Exam Full Solution FRQ#1(a,b)

2022 AP Calculus AB & AP Calculus BC Exam FRQ #1

Continuous Money Flow: Future Value with increasing rate of growth

Average acceleration over interval | AP Calculus BC | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: