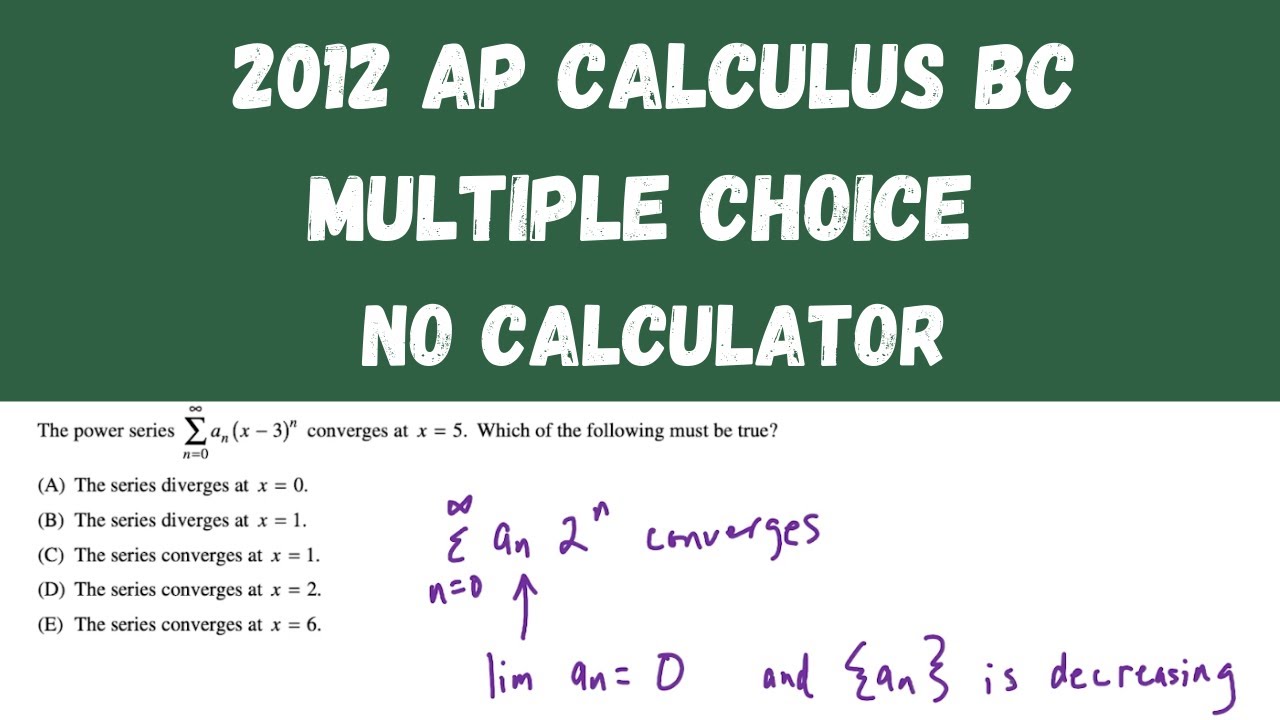
Calc AB 2022 FRQs 1-6 Compilation

turksvids

12 May 202248:18

EducationalLearning

32 Likes 10 Comments

TLDRThis video script offers a detailed walkthrough of calculus problems from the 2022 AP exam, covering rate of change, integral expressions, average value, and the behavior of functions. It explores solving differential equations using separation of variables and examines particle motion, including velocity, acceleration, and position. The script emphasizes the importance of understanding the problem's context and applying the correct calculus techniques to arrive at accurate solutions.

Takeaways

📚 The video covers a range of calculus problems from the 2022 exam, focusing on various concepts such as rate of change, integrals, derivatives, and differential equations.
🚦 The first problem involves calculating the total number of vehicles arriving at a toll plaza and finding the average rate of vehicles per hour, using integrals and derivatives.
📈 The video demonstrates the use of the average value of a rate, which is found by dividing the integral of the rate function over a given interval by the length of the interval.
🔍 The script discusses how to determine if a rate is increasing or decreasing by evaluating the derivative of the rate function at a specific point.
📉 The concept of a candidate's test is introduced to find the maximum number of vehicles in line at a toll plaza, by analyzing the behavior of the integral function over a closed interval.
🌟 The video explains how to find the area enclosed by two functions and the volume of a solid with a square cross-section, using integrals.
🔑 The importance of understanding the domain of functions and their derivatives is highlighted, especially when dealing with natural logarithms and their constraints.
⚖️ The script uses the intermediate value theorem to determine the existence of a solution for a specific rate of change within a given interval.
📝 The process of approximating derivatives using average rates of change is shown, along with its application in solving for unknown rates in a table of values.
🧩 The video script walks through solving differential equations using separation of variables, a common technique in calculus for finding particular solutions.
🏁 The final problem examines particle motion, involving finding velocities and positions over time, and determining which particle will be farther from the origin as time approaches infinity.

Q & A

What is the integral expression for the total number of vehicles that arrive at the toll plaza between t=1 and t=5?
-The integral expression is ∫ from 1 to 5 of a(t) dt, where a(t) represents the rate at which vehicles arrive at the toll plaza in vehicles per hour.
How is the average value of the rate at which vehicles arrive at the toll plaza calculated?
-The average value is calculated by dividing the integral of the rate function a(t) over the interval from 1 to 5 by the length of the interval, which is (5 - 1). So, it is (∫ from 1 to 5 of a(t) dt) / 4.
What does the derivative of the rate function a(t) at t=1 indicate about the vehicles' arrival at the toll plaza?
-The derivative of a(t) at t=1, denoted as a'(1), indicates whether the rate of vehicles arriving is increasing or decreasing at that specific time. If a'(1) is greater than 0, it means the rate is increasing at t=1.
How can you determine when a line forms at the toll plaza based on the given information?
-A line forms at the toll plaza whenever the rate of vehicles arriving, a(t), is greater than or equal to 400. You need to find the values of t for which a(t) = 400 and analyze the behavior of the function around these points.
What is the maximum number of vehicles in line at the toll plaza between time a and 4, and how is it found?
-The maximum number of vehicles in line is found using the candidate's test for absolute extrema on a closed interval. It involves finding the critical points and endpoints of the function n(t) and determining the maximum value among them.
What is the area of the region enclosed by the graphs of functions f(x) and g(x), and how is it calculated?
-The area is calculated by integrating the top function f(x) minus the bottom function g(x) over the interval from the intersection points of the two functions. The specific values of the intersection points are used to define the interval of integration.
What does the derivative of the vertical distance function h(x) at x=-0.5 indicate about the distance between the graphs of f and g?
-The derivative of h(x) at x=-0.5, denoted as h'(-0.5), indicates whether the vertical distance between the graphs of f and g is increasing or decreasing at that point. If h'(-0.5) is negative, it means the distance is decreasing.
How is the volume of the solid with square cross-sections, formed by the region enclosed by the graphs of f and g, calculated?
-The volume is calculated by integrating the square of the difference between f(x) and g(x) over the interval from the intersection points of the two functions. The area of the square cross-sections is given by the side length squared, which is the difference between the two functions.
What is the rate of change of the area of the cross-section above a vertical line moving at a constant rate of seven units per second, when the line is at x=-0.5?
-The rate of change of the area with respect to time is found by multiplying the derivative of the area function a(x) at x=-0.5 by the speed of the moving line (dx/dt = 7). It involves using the chain rule to relate the rate of change of the area to the rate of change of x.
How can you find the x-coordinates of all points of inflection of the graph of f on the interval 0 to 7?
-Points of inflection occur where the concavity of the function changes. This can be found by analyzing the first derivative of the function, f'(x), and identifying where it changes from increasing to decreasing or vice versa within the given interval.
What is the absolute minimum value of the function g(x) on the interval from 0 to 7, and how is it found?
-The absolute minimum value of g(x) is found by evaluating g(x) at the critical points and endpoints of the interval. The critical points are found where the first derivative g'(x) equals zero, and the endpoints are the starting and ending points of the interval.
How do you approximate r''(8.5) using the average rate of change of r' over the interval from 7 to 10?
-The approximation is done by calculating the difference in r' values at t=10 and t=7, divided by the length of the interval (10 - 7), which gives the average rate of change of r' over that interval. This average rate is then used as an approximation for r''(8.5).
Can there be a time t between 0 and 3 for which r'(t) is equal to -6, and how do you justify this?
-Yes, there can be a time t between 0 and 3 for which r'(t) is equal to -6. This is justified by the Intermediate Value Theorem, which states that if a function is continuous on a closed interval and takes on different values at the endpoints, it must take on every value between those endpoints at some point within the interval.
How do you approximate the value of the integral from 0 to 12 of r'(t) dt using a right Riemann sum with four subintervals indicated in the table?
-A right Riemann sum is calculated by summing the values of r'(t) at the right endpoint of each subinterval, multiplied by the width of each subinterval. The sum of these products gives an approximation of the integral over the entire interval from 0 to 12.
What is the rate of change of the volume of the cone with respect to time at time t=3 days, given the radius and height of the cone at that time?
-The rate of change of the volume with respect to time is found by differentiating the volume formula of a cone with respect to time and plugging in the given values for the radius, height, and their respective rates of change at time t=3 days.
How do you determine which particle, P or Q, will eventually be farther from the origin as time approaches infinity?
-By analyzing the limit of the position functions of both particles as time approaches infinity, you can determine which particle will be farther from the origin. The particle with the greater limit value of its position function will be at a greater distance from the origin.
What is the differential equation given in the problem, and how do you find its particular solution with the initial condition f(1) = 2?
-The given differential equation is dy/dx = (1/2) * sin(π/2 * x) * sqrt(y + 7). To find the particular solution, you can use separation of variables and integrate both sides of the equation with respect to their respective variables.
How do you sketch the solution curve for the given differential equation through the point (1, 2)?
-The solution curve can be sketched by following the slope field, which represents the direction and steepness of the slope of the solution curve at various points. Starting at the point (1, 2), you can trace the curve by following the flow of the slope field.
What is the equation of the tangent line to the solution curve at the point (1, 2), and how do you use it to approximate f(0.8)?
-The equation of the tangent line is found by calculating the slope of the solution curve at the point (1, 2) and using the point-slope form of a line. The slope is given by the derivative of the solution at x=1. The tangent line equation can then be used to approximate f(0.8) by plugging in x=0.8 into the equation.
Is the approximation found in part b an overestimate or an underestimate for f(0.8), and why?
-The approximation is an underestimate for f(0.8).

Outlines

00:00

📚 Rate of Change and Vehicle Arrivals

This paragraph discusses a calculus problem from the 2022 exam involving the rate of change. It describes a scenario where vehicles arrive at a toll plaza at a rate given by a function of time. The problem involves finding the total number of vehicles that arrive between specific hours without evaluating the integral, just writing the expression. It also asks for the average rate of vehicle arrivals over a time interval and concludes with finding if the rate of arrival is increasing or decreasing at a particular time, using the derivative of the rate function.

05:01

📈 Calculus Problems: Average Rates and Maximum Values

The second paragraph continues the theme of calculus problems, focusing on finding the average rate of vehicle arrivals at a plaza and determining when a line forms based on the rate exceeding a certain value. It uses integrals to calculate areas under the curve and applies the candidate's test to find absolute maximum values. The paragraph also touches on the concept of differentiability and continuity in the context of finding maximum values of a function over an interval.

10:02

📐 Volume and Area Problems in Calculus

This paragraph presents a problem involving the area and volume calculations for a solid with square cross-sections, based on the graphs of two functions. It discusses finding the area enclosed by the graphs, determining whether a function is increasing or decreasing at a specific point, calculating the volume of the solid, and finding the rate of change of the area of a cross-section with respect to time as a vertical line moves through the solid at a constant speed.

15:03

📉 Analysis of a Derivative Graph and Function Behavior

The fourth paragraph examines a problem where the graph of a derivative function is given, and various questions about the original function are asked. It covers finding function values at specific points using the Fundamental Theorem of Calculus, identifying points of inflection, and determining intervals where a related function is decreasing. The paragraph also involves calculating the absolute minimum value of a function on a given interval.

20:04

📊 Table Analysis and Cone Volume Dynamics

The fifth paragraph presents a table problem involving a cone's changing dimensions and volume. It asks for the approximation of a function's second derivative at a specific point using the average rate of change, determining if a certain rate of change occurs within a time interval, and using a right Riemann sum to approximate an integral. The paragraph concludes with calculating the rate of change of the cone's volume with respect to time, given the rate of change of its height and dimensions at a specific time.

25:06

🔍 Differential Equations and Particle Motion

The sixth paragraph introduces a differential equation problem and a particle motion scenario. It involves sketching a solution curve through a given point on a slope field, writing an equation for the tangent line to the solution curve at a specific point, and approximating a function value using the tangent line. The paragraph also discusses whether the approximation is an overestimate or underestimate based on the concavity of the function and concludes with solving the given differential equation using separation of variables.

30:07

🚀 Particle Motion Analysis Over Time

The seventh paragraph delves into the motion of two particles moving along different axes, with one moving horizontally and the other vertically. It covers finding the velocity and acceleration of each particle, determining when the speed of one particle is decreasing, calculating the position of a particle over time, and predicting which particle will be farther from the origin as time approaches infinity. The paragraph involves the application of derivatives to find instantaneous rates and the use of limits to analyze long-term behavior.

35:08

📘 Final Thoughts on the 2022 AP Calculus Exam

The final paragraph wraps up the discussion on the 2022 AP Calculus exam problems. It reflects on the types of problems presented, the themes observed in the exam, and the strategies used to solve them. The paragraph concludes with a note of encouragement and a wish for success, highlighting the importance of understanding the problems and applying the correct mathematical techniques.

Mindmap

Keywords

💡Integral

An integral in calculus represents the area under a curve, which can be thought of as the accumulated change over a given interval. In the context of the video, integrals are used to calculate the total number of vehicles arriving at a toll plaza and the area under the curves of functions, which is crucial for understanding rate of change problems and area- or volume-related questions.

💡Rate of Change

The rate of change is a measure of how quickly a quantity changes with respect to another quantity. In the video, the rate of change is exemplified by the function 'a of t', which describes the rate at which vehicles arrive at a toll plaza over time. Understanding rate of change is essential for solving problems related to growth, decay, and motion.

💡Average Value

The average value of a function over an interval is a measure that represents the 'mean' value that the function takes over that interval. In the script, the average value is calculated to determine the mean rate of vehicles arriving at a toll plaza between specific times, which is a fundamental concept in understanding the overall behavior of a function over a period.

💡Derivative

A derivative in calculus gives the rate at which a function is changing at a given point, often represented as the slope of the tangent line to the function at that point. The video discusses finding the derivative to determine whether the rate of vehicles arriving at a toll plaza is increasing or decreasing at a particular time, which is key for analyzing the instantaneous behavior of a function.

💡Critical Points

Critical points of a function are points where the derivative is zero or undefined, indicating potential changes in the function's behavior, such as local maxima or minima. The video references finding critical points to determine the maximum number of vehicles in line at a toll plaza, which is a common application of critical points in optimization problems.

💡Inflection Points

Inflection points are points on a curve where the concavity changes. In the video, the x-coordinates of points of inflection are sought to understand the intervals on which the graph of a function changes from concave up to concave down or vice versa, which is important for analyzing the overall shape and behavior of the function.

💡Differential Equation

A differential equation is an equation that relates a function with its derivatives. The video presents a differential equation problem where the goal is to find a particular solution that satisfies both the equation and an initial condition, illustrating a key application of differential equations in modeling real-world phenomena.

💡Separation of Variables

Separation of variables is a method used to solve certain types of differential equations by rearranging the equation so that all instances of one variable and its derivative are on one side, allowing for easier integration. The video script describes using this technique to solve a given differential equation, which is a fundamental skill in differential calculus.

💡Tangent Line

A tangent line to a curve at a given point is a straight line that touches the curve at that point without crossing it. The video discusses finding the equation of the tangent line to a solution curve at a specific point, which is important for approximating function values and understanding local linear behavior.

💡Concavity

Concavity refers to the curvature of a function, indicating whether the function is bending upward (concave up) or downward (concave down). The video mentions concavity to determine whether an approximation of a function value is an overestimate or an underestimate, which is crucial for understanding the shape and behavior of the function.

💡Particle Motion

Particle motion in physics involves studying the movement of an object under various forces. In the video, the motion of two particles along different axes is described using position and velocity functions, which is a common scenario in kinematics to analyze the behavior of objects in motion.

Highlights

Explaining the first problem from the 2022 Calc AB and BC exams, focusing on a rate of change problem involving vehicle arrivals at a toll plaza.

Part A: Writing an integral expression for the total number of vehicles arriving between 5 AM and 10 AM.

Part B: Finding the average value of the rate of vehicle arrivals over a specified interval using integral calculus.

Analyzing the rate of vehicle arrival at a specific time to determine if it is increasing or decreasing.

Using a candidate's test to find the greatest number of vehicles in line at the toll plaza during a specified interval.

Describing the steps to solve a problem involving the intersection of functions and the calculation of areas between curves.

Determining if the vertical distance between graphs of two functions is increasing or decreasing at a specific point.

Calculating the volume of a solid with a known cross-sectional area, specifically with square cross-sections.

Finding the rate of change of the area of a cross-section as a vertical line moves at a constant rate.

Using the Fundamental Theorem of Calculus to determine specific function values based on a given derivative graph.

Identifying points of inflection for a function based on the graph of its derivative.

Finding the intervals on which a function is decreasing by analyzing its derivative.

Using the candidate's test to find the absolute minimum value of a function on a given interval.

Modeling the melting of a cone-shaped ice sculpture and calculating its changing radius using differentiable functions.

Using a right Riemann sum to approximate the value of an integral based on provided table data.

Finding the rate of change of the volume of a cone given the rate of change of its height and radius.

Solving a differential equation problem by sketching solution curves and finding particular solutions with given initial conditions.

Using separation of variables to find the particular solution to a given differential equation.

Analyzing the motion of two particles, one moving horizontally and one vertically, to determine their velocities and positions.

Determining which particle will be farther from the origin as time approaches infinity based on their respective motion equations.

Transcripts

Browse More Related Video

2012 AP Calculus: AB FRQ Solutions

2022 AP Calculus AB Exam FRQ #6

AP Calculus BC 2008 Multiple Choice (no calculator) - questions 1 - 28

AP Calculus BC Practice Exam 2012 - Multiple Choice questions 1-28

2022 AP Calculus AB BC Free Response #1

The Guess Method to Solve Every Physics Problem (Easy)

Related Tags

AP Calculus 2022 Exam Rate of Change Integration Velocity Particle Motion Math Solutions Calculus Problems Exam Prep Student Resources