AP Calculus BC Practice Exam 2012 - Multiple Choice questions 1-28
TLDRThe video script is a comprehensive walkthrough of an AP Calculus BC 2012 practice test. The presenter, Van, meticulously solves a variety of calculus problems, starting with the differentiation of a trigonometric function using the chain rule. He progresses to finding the position of a particle at rest, evaluates a piecewise function's definite integral, calculates the length of a curve using arc length formula, and assesses the convergence of series through tests like the ratio test and the alternating series test. Van also explores differential equations, including logistic differential equations, and applies Euler's method for approximation. The script concludes with the evaluation of limits, particularly using L'Hôpital's rule for indeterminate forms. Throughout the session, Van emphasizes the importance of understanding calculus concepts and applying them correctly to solve complex problems, providing a valuable resource for students preparing for the AP Calculus BC exam.
Takeaways
- 📚 The video provides a walkthrough of an AP Calculus BC 2012 practice test, covering a range of calculus topics.
- 🔑 The first question involves using the chain rule to find the derivative of y = (sin(x))^3, which matches choice E.
- ⏰ For the second question, determining the particle at rest involves finding when the velocity in both the x and y components is zero, which occurs at t = 2, leading to the point (-4, 12).
- 📏 The third question deals with evaluating a definite integral involving a piecewise function, which results in zero, corresponding to choice B.
- 🧮 The fourth question is about finding the length of the curve y = ln(x) from x = 1 to x = 2, using the arc length formula, and the correct answer is choice A.
- 📈 In the fifth question, evaluating a Maclaurin series at x = 3 involves rewriting the series and using the formula for a geometric series, with the result being choice C.
- 🎚 The sixth question uses u-substitution to transform an integral, with the final answer being choice C after substituting back and evaluating.
- 🔁 The seventh question involves implicit differentiation of arcsine(x) = ln(y) to find dy/dx, which matches choice A.
- 🚛 The eighth question is about calculating the amount of oil in a tank at t = 15 hours, using a Riemann sum with the given rates, and the correct answer is choice C.
- 🌟 The ninth question tests the understanding of series convergence, with the conclusion that the series with factorials in the denominator converge, while the others diverge, making choice T correct.
- ✅ The last question uses L'Hôpital's rule to evaluate a limit as x approaches 1, with the final answer being choice D.
Q & A
What is the derivative of y = (sin(x))^3 using the chain rule?
-The derivative of y = (sin(x))^3 is obtained by applying the chain rule. The outer function is (sin(x))^3, and the inner function is sin(x). The derivative of the outer function is 3 * (sin(x))^2, and the derivative of the inner function is cos(x). Thus, the derivative dy/dx = 3 * (sin(x))^2 * cos(x), which matches choice E in the transcript.
At what point is the particle at rest given the position equations for x(t) and y(t)?
-The particle is at rest when both its x and y velocity components are zero. For the given x(t) = 2^(t^3) - 3t^2 and y(t) = 12t - 3t^2, the particle is at rest at t = 2. Plugging t = 2 into the position functions gives x(2) = -4 and y(2) = 12, so the particle is at rest at the point (-4, 12), which is choice A.
How do you evaluate the definite integral with a piecewise function from zero to four?
-The definite integral with a piecewise function from zero to four is evaluated in two parts due to the change in the function at x = 2. From zero to two, the area under the curve forms a trapezoid, and from two to four, it forms a rectangle. The area of the trapezoid is calculated using the average height times the sum of the bases, and the area of the rectangle is found by multiplying its length by its (negative) height. The integral evaluates to zero, corresponding to choice B.
What is the length of the curve y = ln(x) from x = 1 to x = 2?
-The length of the curve y = ln(x) from x = 1 to x = 2 is found using the arc length formula, which involves the square root of 1 plus the derivative of the function squared. The derivative of ln(x) is 1/x, so the arc length formula becomes ∫(1+(1/x)^2)^(1/2) dx from 1 to 2. This simplifies to ∫(1 + 1/x^2)^(1/2) dx, which corresponds to choice A.
How do you evaluate the Maclaurin series for f(x) at x = 3?
-The Maclaurin series for f(x) is given by a summation from n=0 to infinity of (-x/4)^n. To evaluate this at x = 3, substitute -3/4 into the series and recognize it as a geometric series. The sum of a geometric series is given by 1 / (1 - r), where r is the common ratio. In this case, r = -3/4, so the sum is 1 / (1 - (-3/4)) = 4/7, which is choice C.
What is the value of the integral from negative one to one of (x^2 - 3) / (x^2) dx using u-substitution?
-Using u-substitution, set u = x^2 - 3, so du = 2x dx or (1/2) du = x dx. The integral becomes (1/2) ∫ u^(5/2) du from the new limits, which are u = -2 (when x = -1) and u = 13 (when x = 4). After finding the antiderivative, the final answer matches choice C.
What is the derivative of y = arcsin(x) with respect to x?
-The derivative of y = arcsin(x) with respect to x is found by differentiating both sides with respect to x and applying the chain rule. The derivative d/dx of arcsin(x) is 1/√(1 - x^2), and the derivative d/dx of ln(y) is (1/y)(dy/dx). Solving for dy/dx gives y / √(1 - x^2), which corresponds to choice A.
How much oil is in the tank at t = 15 hours, given the initial amount and the rate function?
-The amount of oil in the tank at t = 15 hours is calculated by adding the initial amount to the accumulation of oil from t = 4 to t = 15. This is done by integrating the rate function r(t) from 4 to 15 and then adding the initial 50 liters. Using a right Riemann sum and the given values, the total amount of oil is 114.9 liters, which is choice C.
Which series converge based on the provided terms?
-To determine which series converge, consider the behavior of factorials, exponentials, and power functions. The first series has a factorial in the denominator, which grows faster than exponentials and power functions, leading to convergence. The second series has a power function in the denominator, which suggests divergence by the nth term test. The third series resembles a convergent p-series, as the leading term is 1/n^2. By the ratio test, the first series converges, the second diverges, and the third also converges, making choice T correct.
What is the value of the definite integral from t = 1 to t = 4 of (-3/2 + 1) dt?
-The definite integral from t = 1 to t = 4 of (-3/2 + 1) dt is evaluated by finding the antiderivative of the function, which is (-3/2 * t^(-1/2) + t) evaluated from 1 to 4. After performing the evaluation, the result is -2 * (1/√4 - 1), which simplifies to -2 * (1/2 - 1) = 1, corresponding to choice E.
What is the value of f(-5) given that f(2) = 1 and the graph of the derivative f'?
-To find the value of f(-5), one must integrate the derivative function from -5 to 2 and then subtract f(2). The area under the curve from -5 to 2 is composed of a triangle and a semi-circle. The triangle has an area of 3, and the semi-circle has an area of -2π. Thus, f(2) = 1 - f(-5), and solving for f(-5) gives 2 - 2π as the value, which corresponds to choice A.
Outlines
📚 AP Calc BC 2012 Practice Test Walkthrough
Van, the presenter, guides viewers through the AP Calc BC 2012 practice test. He begins with question one, which involves differentiating y = (sin x)^3 using the chain rule, and matches the result to choice E. For question two, he determines the particle's rest position by finding when the velocity in both the x and y components is zero, which occurs at t=2, leading to the position (-4, 12), corresponding to choice A. Question three deals with a piecewise function's definite integral from 0 to 4, which evaluates to zero, aligning with choice B. The arc length of y = ln(x) from x=1 to x=2 is found in question four, and Van uses the arc length formula to find the correct answer, choice A. In question five, Van evaluates the Maclaurin series for f(x) at x=3, which simplifies to 4/7, matching choice C.
🔍 U-Substitution and Implicit Differentiation
The video continues with a discussion on u-substitution in integrals, where Van shows how to transform the integral of (x^2 - 3) with respect to x by setting u = x^2 - 3 and finding du. He also addresses the limits of integration in terms of u and solves the integral, which corresponds to choice C. For the next question, Van tackles an implicit differentiation problem involving arcsine x = ln y, solving for dy/dx and matching the result to choice A. He then discusses a tank problem where oil is being pumped in at a rate given by a table of values, using the data to find the amount of oil in the tank after 15 hours, which is choice C. Van also evaluates the convergence of various series by recognizing factorials, exponentials, and power functions, and determines that only one series converges, which is identified as choice T.
🧮 Application of Ratio and Limit Comparison Tests
Van applies the ratio test to a series with the term (8^(n+1))/(n+1)!, showing that the series converges as the limit approaches zero. He then uses the limit comparison test to evaluate the convergence of another series, comparing it to 1/n^2, and concludes that the series converges. The video moves on to question 10, where Van uses the fundamental theorem of calculus to find the antiderivative of a function and evaluates it from 1 to 4, selecting choice E. For question 11, Van analyzes the function f(x) = sqrt(|x-2|) and determines that it is continuous but not differentiable at x=2, eliminating certain choices and concluding that the correct answer is choice A.
📉 Analysis of a Tank's Oil Level and Differential Equations
The video examines a scenario where a tank has an initial 50 liters of oil, and oil is being added at a variable rate. Van uses a Riemann sum with the given data points to find the total amount of oil in the tank after a specific time period, which is identified as choice C. He also discusses the convergence of series by comparing the terms and using the ratio test, concluding that one series converges, which is choice T. Van then explores a differential equation related to a tank's oil level and uses the second derivative test to find that a specific point on the graph represents a local maximum, leading to choice C.
🔢 Power Series Convergence and Logistic Differential Equations
Van rewrites a given power series to identify its convergence properties, determining that the series converges when |x - 4|^2 < 3, which corresponds to choice C. He then quickly identifies a logistic differential equation from the given options as choice E. For a function representing the area under a curve, Van discusses the function's properties and its derivatives, evaluating them at specific points and finding that the order from least to greatest matches choice A.
📝 Euler's Method and Power Series Recognition
Using Euler's method, Van approximates the value of a function at x=2, starting with the function's value at x=1, and performs two steps of equal length to find the approximation, which is choice C. He then deals with a power series and recognizes it as a modified version of the sine function's power series, concluding that the function f(x) is sin(x)/x, which is choice C. Van also evaluates the antiderivative of a function from negative five to two and uses the geometric properties of the graph to find the area under the curve, leading to choice A.
🔍 Finding Tangent Slopes and Horizontal Asymptotes
Van sets up an equation to find the points on the graph of a function where the slope of the tangent line is one-half, using the quotient rule and algebraic manipulation to find the points x=0 and x=-4, which correspond to choice C. He then discusses how to find the horizontal asymptote of a function by examining the limit as x approaches infinity, identifying the correct function as choice E. For a power series that converges at x=5, Van uses the alternating series test to find the values of x that make the series converge, which is choice D.
📐 Linear Growth Differential Equations and Integration by Parts
Van identifies the differential equation that represents linear growth in a population as choice A, based on the constant rate of change. He then uses integration by parts to find the function f(x) that, when integrated with sine(x)dx, results in a specific expression, concluding that f(x) = x^4 is the correct answer, which is choice E. Van evaluates an improper integral by performing a u-substitution and taking the limit as b approaches infinity, finding the correct answer to be choice B.
🧮 Polar Curves and Convergence of Series
Van calculates the slope of the line tangent to a polar curve at a specific theta value by using the relationships x = r*cos(θ) and y = r*sin(θ), and finds that the slope is 1/2, which corresponds to choice B. He then finds the values of p for which two series converge, solving inequalities to find the common interval for p, which is between 1/2 and 2, matching choice C. Lastly, Van evaluates a limit using l'Hôpital's rule after recognizing an indeterminate form, finding that the limit is 2, leading to the final answer of choice D.
Mindmap
Keywords
💡Chain Rule
💡Velocity
💡Definite Integral
💡Arc Length
💡Maclaurin Series
💡U-Substitution
💡Implicit Differentiation
💡Riemann Sum
💡nth Term Test
💡Ratio Test
💡Convergence
Highlights
The derivative of y = sin^3(x) is found using the chain rule, resulting in the expression 3sin^2(x)cos(x), matching choice E.
A particle is at rest when both its x and y velocity components are zero, found by setting the derivatives of the position functions to zero and solving for t.
The position of a particle at rest is determined by plugging the time t back into the position functions, resulting in the point (-4, 12), corresponding to choice A.
The definite integral of a piecewise function from 0 to 4 is evaluated as the sum of the areas of a trapezoid and a rectangle, resulting in a total area of zero, aligning with choice B.
The arc length of y = ln(x) from x = 1 to x = 2 is calculated using the arc length formula, leading to the expression √(1 + (1/x)^2)dx, which matches choice A.
Evaluating a Maclaurin series for f(x) at x = 3 involves rewriting the series with the new value and using the formula for the sum of a geometric series, resulting in the answer 4/7, corresponding to choice C.
U-substitution is used to transform an integral by replacing the integrand and finding du, which is then used to rewrite the integral and match the correct choice, which is C in this case.
The derivative dy/dx of arcsin(x) = ln(y) is found using implicit differentiation, resulting in the expression y/√(1 - x^2), which matches choice A.
The amount of oil in a tank at t = 15 hours is found by integrating the rate function from t = 4 to t = 15, using a right Riemann sum and table of values, resulting in 114.9 liters, aligning with choice C.
The convergence of a series with factorial terms is determined by comparing the rate of increase of factorials to exponentials and power functions, suggesting the series converges, which is confirmed by the ratio test.
The fundamental theorem of calculus is applied to find the antiderivative of a function and evaluate it between two points, resulting in the answer -2(√4 - 1), corresponding to choice E.
The function f(x) = √|x - 2| is analyzed for continuity and differentiability at x = 2, with the conclusion that it is continuous but not differentiable at that point, which is not one of the provided choices.
Investigating a differential equation satisfied by two points on the graph of y = f(x) reveals that the point (-1, -1) is a local maximum, corresponding to choice C.
Rewriting a power series in a recognizable form helps identify it as the power series for sine(x)/x, leading to the conclusion that f(x) = sine(x)/x, which matches choice C.
Using the fundamental theorem of calculus to find f(-5) given f(2) = 1 involves finding the area under the curve of f'(x) from -5 to 2 and subtracting, resulting in f(-5) = 2π - 2, aligning with choice A.
The slope of the line tangent to a polar curve at a given point is found by converting the polar equation to Cartesian coordinates and using the derivatives dx/dt and dy/dt, leading to a slope of 1/2, corresponding to choice B.
The values of p for which two series converge are found by applying the p-series test and the geometric series test, resulting in the interval p > 1/2 and -2 < p/2 < 2, which matches choice C.
An indeterminate limit is resolved using l'Hôpital's rule, leading to the evaluation of g(1)/g'(1), which simplifies to 6/3, resulting in the answer 2, corresponding to choice D.
Transcripts
Browse More Related Video

AP Calculus BC 2008 Multiple Choice (no calculator) - questions 1 - 28

AP Calculus AB/BC Unit 7 Practice Test

Infinite Series Multiple Choice Practice for Calc BC (Part 5)

AP Calculus BC 2003 Multiple Choice (calculator) - Questions 76 - 92
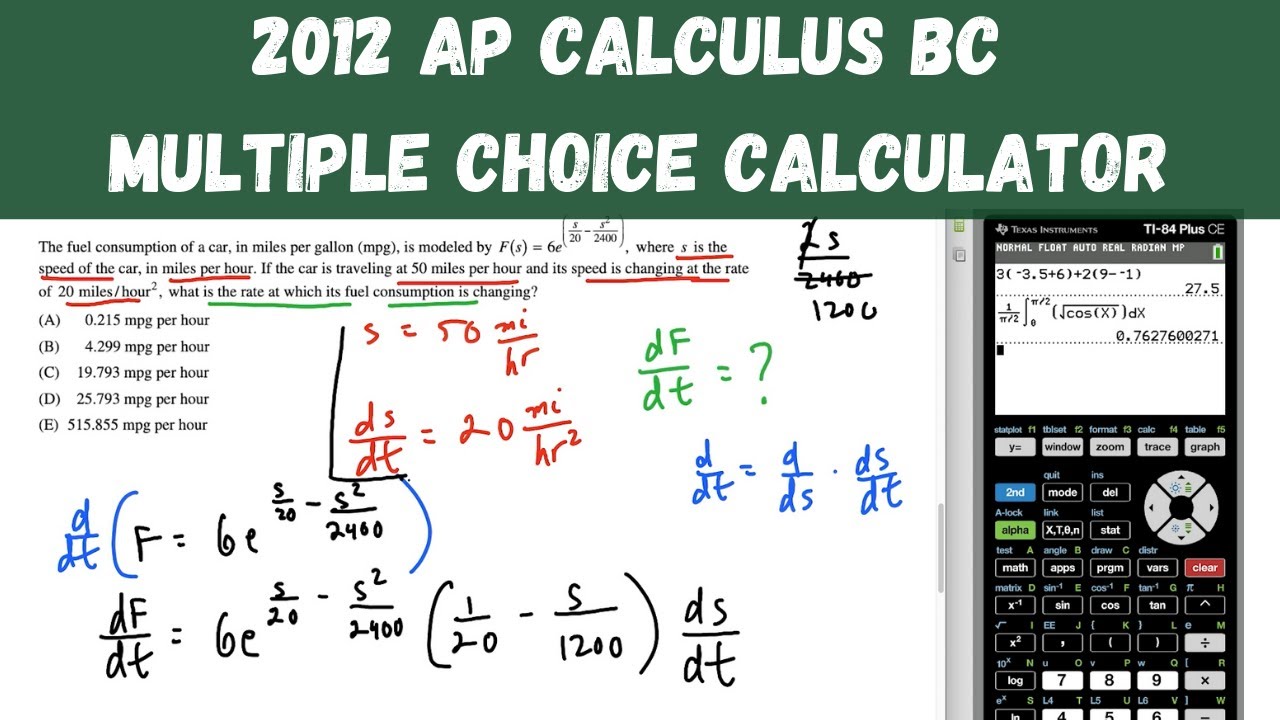
AP Calculus BC Practice Exam 2012 - Calculator Multiple Choice questions 76-92

AP Calculus BC 2003 Multiple Choice (no calculator) - questions 1 - 28
5.0 / 5 (0 votes)
Thanks for rating: