The precise definition of the limit EXPLAINED! (KristaKingMath)
TLDRThis video script delves into the epsilon-delta definition of a limit in calculus, demonstrating how to prove the limit of the function 7x - 9 as x approaches -3 equals 30. The presenter explains the intuitive approach of substitution and then systematically applies the precise definition of a limit to establish a relationship between epsilon and delta. By setting delta to be less than epsilon over seven, the video illustrates the rigorous mathematical proof that the limit exists, showcasing the foundational concepts of limits in a clear and engaging manner.
Takeaways
- 📘 The video discusses the precise definition of the limit, also known as the epsilon-delta definition.
- 🔢 The example provided is to prove that the limit as X approaches -3 of the function 7x - 9 is equal to -30.
- 📉 Substitution is a straightforward way to evaluate a limit, demonstrated by substituting -3 into the function.
- 🧮 The precise definition involves two inequalities, one relating X and delta, and the other relating the function's value and epsilon.
- 🔍 The definition is explained using a linear function graph and illustrating the relationship between epsilon and delta.
- 📐 The epsilon-delta definition confirms that as X gets closer to a point, the function's value gets closer to the limit.
- 📏 The video emphasizes finding a relationship between epsilon and delta to prove the limit.
- 📝 An example is given where epsilon is a range around the limit, and delta is the corresponding range around X.
- 🔄 The process involves showing that if the distance between X and a point is less than delta, the function's value will be within epsilon of the limit.
- ✅ The relationship between epsilon and delta is used to prove the correctness of the limit equation for the function.
Q & A
What is the epsilon-delta definition of a limit?
-The epsilon-delta definition of a limit is a precise way to describe a limit in calculus. It states that for every small distance (epsilon) from the limit value L, there exists a corresponding small distance (delta) from the point 'a' such that if the input to the function is within delta of 'a', the output is within epsilon of L.
What is the limit of the function 7x - 9 as x approaches -3 according to the script?
-The limit of the function 7x - 9 as x approaches -3 is -30, as stated in the script.
How does the script demonstrate the limit of the function using substitution?
-The script demonstrates the limit by substituting x with -3 in the function 7x - 9, which results in 7*(-3) - 9, equaling -21 - 9, which is -30. This shows that as x gets very close to -3, the function's value gets very close to -30.
What is the relationship between epsilon and delta in the epsilon-delta definition of a limit?
-In the epsilon-delta definition, there is an inequality that relates epsilon and delta. If the absolute value of x - a is less than delta, then the absolute value of f(x) - L is less than epsilon. The challenge is to find a specific relationship between epsilon and delta that can prove the limit.
How does the script illustrate the concept of epsilon and delta graphically?
-The script uses a graph of a linear function to illustrate the concept. It shows how epsilon represents a distance away from the limit value L on the y-axis, and delta represents a distance away from the point 'a' on the x-axis, such that the function's value stays within the epsilon range as x approaches 'a'.
What is the function f(x) used in the script to demonstrate the epsilon-delta definition?
-The function f(x) used in the script is 7x - 9, which is the function for which the limit is being discussed.
What is the value of 'a' in the epsilon-delta definition as per the script?
-In the script, the value of 'a' is -3, as that is the point at which the limit of the function 7x - 9 is being evaluated.
How does the script derive the relationship between epsilon and delta for the given limit problem?
-The script derives the relationship by setting up the inequalities based on the epsilon-delta definition, substituting the function and the limit value into these inequalities, and then simplifying to find that the absolute value of x + 3 must be less than epsilon over 7, leading to the conclusion that delta must be less than epsilon over 7.
What does the ability to state a relationship between Delta and Epsilon prove in the context of the script?
-The ability to state a relationship between Delta and Epsilon proves that the limit equation is true according to the epsilon-delta definition of a limit.
What is the significance of the script's conclusion that Delta must be less than epsilon over 7?
-The conclusion that Delta must be less than epsilon over 7 is significant because it establishes the necessary condition to ensure that the function's value will be within the epsilon range of the limit value L as x approaches 'a', thus proving the existence of the limit.
Outlines
📚 Understanding the Epsilon-Delta Definition of Limits
This paragraph introduces the epsilon-delta definition of limits, a fundamental concept in calculus for proving the value of a limit. The video aims to demonstrate the proof of the limit of the function 7x - 9 as x approaches -3, which is known to be 30. The explanation begins with an intuitive understanding of the limit through substitution, showing that plugging in x = -3 into the function yields the limit value. The paragraph then delves into the precise definition of the limit, explaining how it relates the absolute value of x - a to the absolute value of f(x) - L, where L is the limit value. The concept is illustrated using a graph and a linear function to clarify the relationship between epsilon and delta, emphasizing that as x approaches a, the function value approaches L.
🔍 Applying the Epsilon-Delta Definition to a Limit Problem
The second paragraph continues the discussion on the epsilon-delta definition, focusing on how to apply it to prove a specific limit equation. It uses the function 7x - 9 and the limit as x approaches -3, setting the stage for an in-depth exploration of the relationship between epsilon and delta. The paragraph explains how to visualize the epsilon range on a graph and how moving within this range affects the function's value relative to the limit L. It then translates this visual understanding into mathematical inequalities, demonstrating the process of finding a suitable delta for a given epsilon to ensure the function's value stays within the epsilon range of the limit. The explanation includes substituting the specific values for a, f(x), and L into the inequalities and simplifying them to find the relationship between delta and epsilon.
🔗 Establishing the Delta-Epsilon Relationship for Limit Proof
The final paragraph concludes the video script by establishing the relationship between delta and epsilon, which is crucial for proving the limit equation. It reiterates the two inequalities derived from the epsilon-delta definition and identifies the common value, the absolute value of x + 3, in both. The paragraph then defines the relationship by setting delta to be less than epsilon over seven, which ensures that for any positive epsilon, there exists a corresponding delta that will keep the function's value within the epsilon range of the limit. This relationship confirms the limit's existence and completes the proof, demonstrating the power of the epsilon-delta definition in rigorously proving limits in calculus.
Mindmap
Keywords
💡Limit
💡Epsilon-Delta Definition
💡Substitution
💡Function
💡Absolute Value
💡Inequality
💡Discontinuity
💡Linear Function
💡Graph
💡Proximity
💡Factoring
Highlights
The video discusses the epsilon-delta definition of a limit, a precise mathematical concept.
A limit equation is presented to be proven using the epsilon-delta definition: lim(x→-3)(7x - 9) = 30.
Substitution is used to intuitively evaluate the limit, showing that f(-3) = -30.
The precise definition of the limit is explained, relating two inequalities involving epsilon and delta.
Epsilon is defined as the allowable distance away from the limit value L.
Delta is the distance from 'a' along the x-axis that ensures the function's value stays within the epsilon range.
A graphical explanation is provided to visualize the epsilon-delta definition.
The process of proving a limit using the epsilon-delta definition involves finding a relationship between epsilon and delta.
The video demonstrates how to apply the epsilon-delta definition to the given limit equation.
The function f(x) = 7x - 9 is substituted into the epsilon-delta inequality to find a relationship with epsilon.
The limit L is set to -30, and the function is manipulated to fit the epsilon-delta definition.
An inequality involving the absolute value of f(x) - L is simplified to find a relationship with epsilon.
The video concludes that a relationship between Delta and epsilon/7 can be established, proving the limit equation.
The proof demonstrates that for any positive epsilon, there exists a corresponding delta to satisfy the limit condition.
The epsilon-delta definition is shown to be a powerful tool for proving limits in calculus.
The video concludes by emphasizing the importance of the epsilon-delta definition in understanding limits.
Transcripts
Browse More Related Video

Precise Definition of a Limit - Example 1 Linear Function

Epsilon delta limit (Example 2)

Epsilon-delta definition of limits
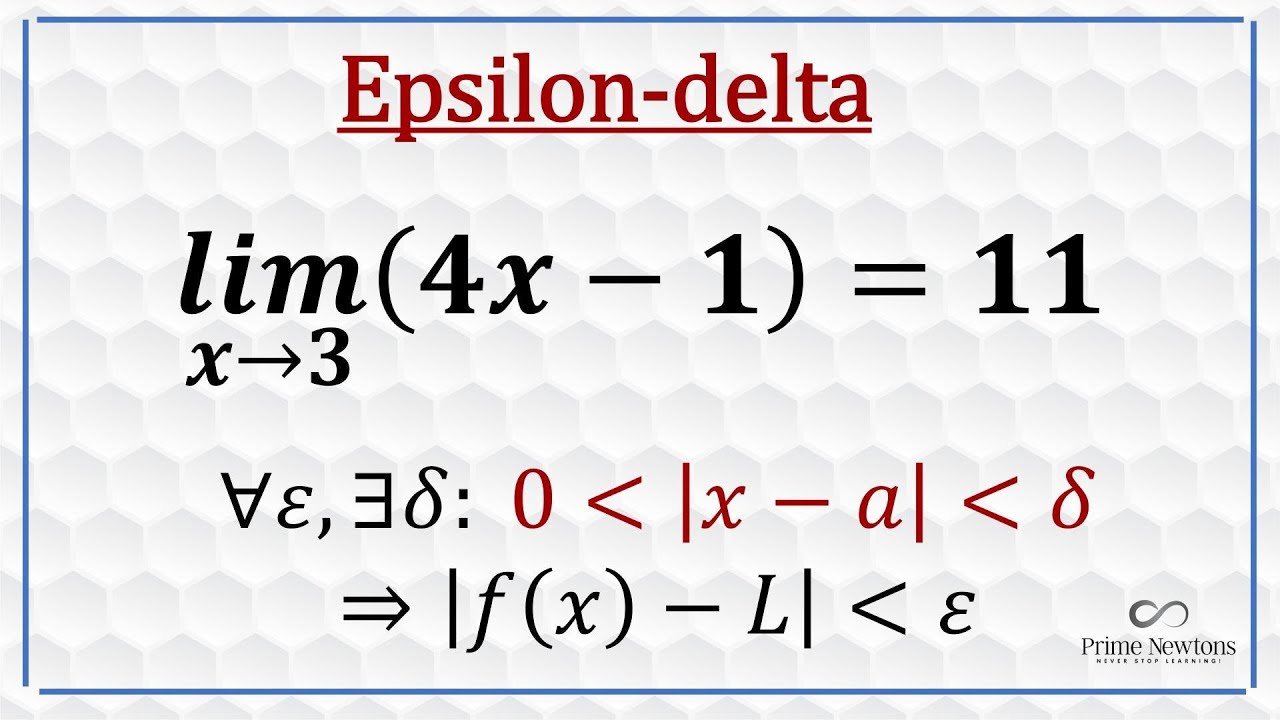
Epsilon - Delta Proof (precise definition of the limit)

Epsilon-delta limit definition 2 | Limits | Differential Calculus | Khan Academy

Using the epsilon-delta defintion of a limit for a quadratic function
5.0 / 5 (0 votes)
Thanks for rating: