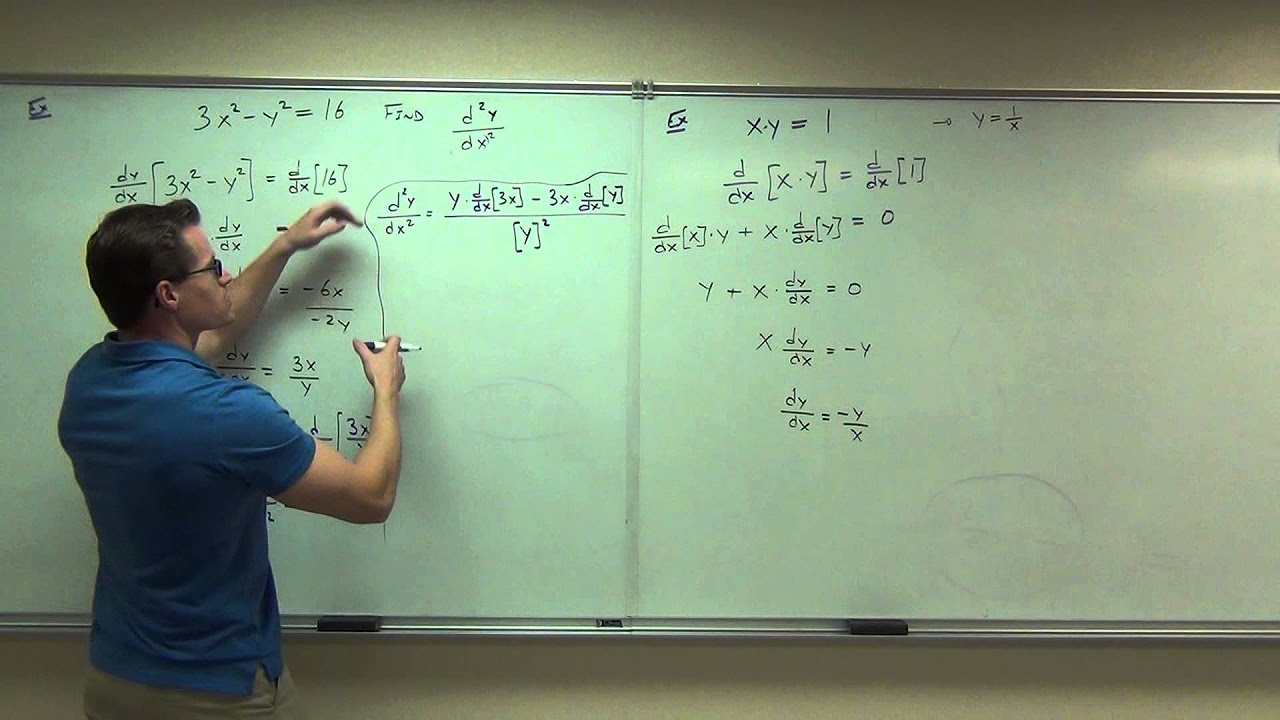Lec 5 | MIT 18.01 Single Variable Calculus, Fall 2007
TLDRThis MIT OpenCourseWare lecture delves into implicit differentiation, a powerful technique for differentiating complex functions. The professor illustrates the method through examples, starting with rational exponents and moving on to more intricate cases like circles and fourth-degree equations. The lecture highlights the ease of implicit differentiation compared to explicit methods, especially when dealing with inverse functions such as arctan(x) and arcsin(x). The professor emphasizes the importance of understanding the range of applicability and simplifies the derived expressions using trigonometric identities, showcasing the practicality of implicit differentiation in calculus.
Takeaways
- 📚 The lecture introduces the concept of implicit differentiation, a technique for differentiating functions that are not easily expressed in explicit form.
- 🔑 The chain rule is emphasized as a powerful tool for extending the types of functions that can be differentiated, particularly useful in implicit differentiation.
- 📈 The process involves two main steps: rewriting the equation to a form that allows for differentiation, and then applying the chain rule to differentiate with respect to x.
- 🌟 An example given is differentiating y = x^(m/n) by rewriting it as y^n = x^m and then differentiating both sides to eventually solve for dy/dx.
- 🔍 The lecture demonstrates that implicit differentiation can handle complex functions, including those involving rational exponents and nth roots.
- 📘 The technique is illustrated with several examples, including differentiating the equation of a circle, x^2 + y^2 = 1, using both explicit and implicit methods.
- 🤔 The professor highlights the implicit method's advantage over the explicit method, especially when dealing with complicated functions or equations that are difficult to simplify.
- 📉 The lecture also covers the differentiation of inverse trigonometric functions, such as arctan(x) and arcsin(x), using implicit differentiation.
- 📌 The importance of understanding the range of applicability for implicit differentiation is stressed, as the method's validity can depend on the domain of the function.
- 📝 The process involves algebraic manipulation to express dy/dx solely in terms of x, which can be complex but is a streamlined approach once mastered.
- ⚠️ The professor cautions students to be careful with the application of implicit differentiation and to ensure they understand the underlying concepts and limitations.
Q & A
What is the main topic of the fifth lecture?
-The main topic of the fifth lecture is implicit differentiation, a technique that allows the differentiation of functions that may not be easily expressed explicitly.
What is the chain rule and why is it significant in the context of this lecture?
-The chain rule is a fundamental principle in calculus for differentiating compositions of functions. It is significant in this lecture because implicit differentiation often involves applying the chain rule to differentiate complex functions that are not easily solvable for y in terms of x.
What is the purpose of rewriting the equation y = x^(m/n) to y^n = x^m before differentiating?
-Rewriting the equation y^n = x^m allows the application of known differentiation rules for integer powers, which simplifies the process of differentiating the original equation y = x^(m/n), especially when dealing with rational exponents.
Why is it necessary to use the chain rule when differentiating y^n with respect to x?
-The chain rule is necessary because y is a function of x (y = f(x)). When differentiating y^n with respect to x, you must account for the rate of change of y with respect to x, which is done by multiplying the derivative of y^n with respect to y (n y^(n-1)) by the derivative of y with respect to x (dy/dx).
How does implicit differentiation help in finding the derivative of functions like y = x^(m/n)?
-Implicit differentiation allows you to differentiate functions like y = x^(m/n) without explicitly solving for y in terms of x. By differentiating both sides of the equation with respect to x and applying algebraic manipulations, you can find an expression for dy/dx in terms of x and y.
What is the advantage of using implicit differentiation over explicit differentiation in certain cases?
-The advantage of using implicit differentiation is that it can simplify the differentiation process, especially when the function is difficult to express explicitly or when dealing with complex functions. It allows for direct differentiation of the given equation, avoiding the need to solve for y in terms of x first.
Why does the professor emphasize the importance of understanding the range of applicability for implicit differentiation?
-The professor emphasizes the importance of understanding the range of applicability because implicit differentiation may not always be valid for all values of x and y. It is crucial to consider the domain and range of the original function to ensure that the derived derivative makes sense within those constraints.
How does the lecture illustrate the process of differentiating the equation x^2 + y^2 = 1 using implicit differentiation?
-The lecture illustrates the process by first showing the explicit differentiation of y as a function of x, then contrasting it with implicit differentiation. In the implicit method, the equation is left in its original form, and both sides are differentiated with respect to x, leading to an equation that can be solved for the derivative y'.
What is the derivative of y = sqrt(x) with respect to x, and how does the lecture demonstrate finding it?
-The derivative of y = sqrt(x) with respect to x is 1/(2*sqrt(x)). The lecture demonstrates finding it by first expressing y as x^(1/2), then using implicit differentiation to find dy/dx = x^(-1/2), and simplifying it to the final form.
How does the lecture explain the concept of inverse functions and their derivatives?
-The lecture explains inverse functions by showing that if y = f(x), then the inverse function g(y) = x, and vice versa. It demonstrates that implicit differentiation can be used to find the derivatives of inverse functions, provided the derivative of the original function is known, using the example of the inverse tangent function.
Outlines
📚 Introduction to Implicit Differentiation
The professor begins the lecture by expressing gratitude for the support of MIT OpenCourseWare and introduces the topic of implicit differentiation. This technique is highlighted as a powerful method for differentiating functions that are not easily expressed in explicit form. The lecture aims to extend the differentiation capabilities to rational exponents and nth roots using the chain rule in an innovative algebraic manner. The first example involves differentiating y = x^(m/n) by rewriting the equation to y^n = x^m and applying the chain rule, leading to the discovery of the derivative dy/dx in terms of x.
🔍 Implicit Differentiation with Rational Exponents
Building on the foundation of implicit differentiation, the professor demonstrates how to handle exponents that are rational numbers, specifically focusing on the case where 'a' is expressed as m/n, with m and n being integers. The process involves rewriting the function to a form that allows the application of known differentiation rules, such as integer powers, and then solving algebraically for dy/dx. The lecture illustrates this with a detailed walkthrough of the steps and the algebraic manipulations required to arrive at the derivative in terms of x.
📘 Differentiating the Circle Equation Implicitly
The professor presents a classic example of implicit differentiation by differentiating the equation x^2 + y^2 = 1, which defines a circle. The process involves differentiating both sides of the equation with respect to x and then solving for the derivative of y with respect to x, denoted as y'. The professor contrasts this implicit method with the explicit method, which involves solving for y first and then differentiating, highlighting the simplicity and directness of the implicit approach.
🤔 Implicit vs. Explicit Differentiation: A Comparison
The lecture continues with a comparison between implicit and explicit differentiation methods. The professor demonstrates that while explicit differentiation can be more complex and requires solving for y in terms of x, implicit differentiation allows for direct differentiation of the given equation. This approach is shown to be more efficient, especially when dealing with complex functions, as it avoids the need for simplification before differentiation.
🧩 Solving a Fourth-Order Implicit Equation
The professor tackles a more complex example involving a fourth-order equation, y^4 + xy^2 - 2 = 0. The solution process involves using implicit differentiation to find the derivative of y with respect to x, denoted as y'. The professor shows that by differentiating the equation and rearranging terms, a formula for y' can be derived without needing to solve for y explicitly. This method is particularly useful for equations that are difficult to solve explicitly.
📌 Implicit Differentiation and Inverse Functions
The lecture concludes with an exploration of how implicit differentiation can be applied to find the derivatives of inverse functions. The professor uses the example of the inverse tangent function, or arctan(x), to illustrate this. By starting with the defining equation for the inverse function, differentiating both sides, and then solving for the derivative, the professor demonstrates that implicit differentiation provides a powerful tool for finding derivatives of functions that are not easily invertible.
📐 Derivatives of Inverse Trigonometric Functions
The professor provides a detailed explanation of how to find the derivatives of inverse trigonometric functions, specifically focusing on the inverse tangent and inverse sine. Using implicit differentiation, the professor derives the derivative of arctan(x) as 1 / (1 + x^2) and the derivative of arcsin(x) as 1 / sqrt(1 - x^2). The importance of understanding the range of applicability for these derivatives is emphasized, and the professor warns about the need to be careful with the domain and range of the functions involved.
Mindmap
Keywords
💡Chain Rule
💡Implicit Differentiation
💡Exponents
💡Rational Numbers
💡Derivative
💡Square Root
💡Inverse Functions
💡Tangent Function
💡Quotient Rule
💡Secant Function
💡Trigonometric Functions
Highlights
Introduction to the fifth lecture and the topic of implicit differentiation.
Explanation of the chain rule as a powerful technique for differentiating functions.
The importance of implicit differentiation in differentiating functions that are not easily expressed explicitly.
Derivation of the formula for the derivative of x to a rational power (m/n).
Step-by-step process of implicit differentiation applied to the equation y = x^(m/n).
Conversion of the equation y^n = x^m to facilitate differentiation.
Application of the chain rule to differentiate equations involving y as a function of x.
Solving for dy/dx using algebraic manipulation.
Example of differentiating the equation x^2 + y^2 = 1 using implicit differentiation.
Comparison between explicit and implicit differentiation methods for the circle equation.
Explanation of why the implicit method does not differentiate between the top and bottom halves of the circle.
Differentiating a fourth-order equation y^4 + xy^2 - 2 = 0 using implicit differentiation.
Philosophical discussion on the limitations of finding derivatives when the function itself is complex.
Application of implicit differentiation to find the derivatives of inverse functions.
Derivation of the derivative of the inverse tangent function (arctan) using implicit differentiation.
Simplification of the derivative of arctan(x) using trigonometric identities.
Differentiation of the inverse sine function (arcsin) and its simplification.
Importance of considering the range of applicability when using implicit differentiation.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: