Algebra Basics: Solving Basic Equations Part 1 - Math Antics

mathantics
22 May 201511:08
EducationalLearning
32 Likes 10 Comments

TLDRIn this Math Antics video, Rob teaches viewers how to solve simple algebraic equations involving addition and subtraction. He emphasizes the importance of keeping equations balanced by making the same changes to both sides, whether adding, subtracting, multiplying, or dividing. Using clear examples, he demonstrates how to isolate the unknown variable by undoing operations, such as subtracting 7 from both sides of an equation to solve for 'x'. Rob also tackles a tricky variation where an unknown is subtracted from a number, showing an alternative method to avoid negative unknowns. The video is a practical guide for beginners to understand and practice fundamental algebraic problem-solving techniques.

Takeaways
  • 🧩 Algebra involves equations with variables or unknown values, and solving them means finding these unknown values.
  • 🔍 The goal in solving algebraic equations is to isolate the unknown value on one side of the equation.
  • ⚖️ Algebraic equations are like balance scales; both sides must have the same value to maintain balance.
  • 🔄 To rearrange an equation, you must apply the same operation to both sides to keep the equation balanced.
  • ➕ When 'x' is combined with a number through addition, subtract the same number from both sides to isolate 'x'.
  • ➖ To undo subtraction from 'x', add the subtracted number to both sides of the equation to isolate 'x'.
  • 🔄 The commutative property states that the order of addition does not change the sum, which is useful for rearranging equations.
  • 🤔 When faced with a tricky subtraction problem, adding the unknown to both sides can simplify the equation before isolating the variable.
  • 📚 Practice is essential for mastering algebraic equations, so solving basic equations on your own is highly recommended.
  • 📉 The process of solving equations by addition and subtraction applies to decimals and fractions as well.
  • 📝 The symbol used for the unknown value doesn't matter; it could be any letter such as x, y, z, or a, b, c.
Q & A

  • What is the main focus of the video?

    -The main focus of the video is to teach viewers how to solve simple algebraic equations involving addition and subtraction.

  • What is the key strategy for solving an equation with an unknown value?

    -The key strategy is to rearrange the equation until the unknown value is alone on one side of the equal sign and all known numbers are on the other side.

  • Why is it important to keep the equation balanced when rearranging it?

    -Keeping the equation balanced ensures that the equation remains true. Any change made to one side must be mirrored on the other side to maintain this balance.

  • What is the commutative property mentioned in the script, and how does it relate to solving equations?

    -The commutative property states that the order of addition or multiplication does not change the result. In the context of equations, it means that adding or multiplying the same numbers on both sides will keep the equation balanced.

  • How do you isolate the unknown value 'x' in the equation x + 7 = 15?

    -To isolate 'x', subtract 7 from both sides of the equation, which simplifies to x = 8.

  • What is the process to solve the equation 40 = 25 + x?

    -To solve for 'x', subtract 25 from both sides of the equation, resulting in 15 = x or x = 15.

  • How can you solve an equation where an unknown is being subtracted from a number, like in the example x - 5 = 16?

    -To solve for 'x', add 5 to both sides of the equation, which simplifies to x = 21.

  • In the equation 10 = x - 32, what operation should you perform to isolate 'x'?

    -Add 32 to both sides of the equation to isolate 'x', resulting in x = 42.

  • What is a tricky variation of the subtraction problem that can confuse students, and how is it handled in the script?

    -A tricky variation is when an unknown is being subtracted from a number, such as 12 - x = 5. Instead of subtracting 12 from both sides, the script suggests adding 'x' to both sides to avoid getting a negative unknown, which simplifies the equation to 12 = 5 + x, and then subtracting 5 from both sides to solve for 'x'.

  • Does the process of solving simple algebraic equations change if the unknown is represented by a different letter?

    -No, the process remains the same regardless of the letter used to represent the unknown, whether it's x, y, z, or any other letter.

  • What advice does the video give for learning algebraic equations?

    -The video advises viewers to practice solving basic equations on their own to reinforce what they've learned.

Outlines
00:00
📚 Introduction to Simple Algebraic Equations

This paragraph introduces the concept of algebraic equations, emphasizing the role of variables and the importance of solving for unknown values. It outlines the strategy for solving equations by rearranging them to isolate the unknown on one side of the equal sign. The paragraph also introduces the balance scale analogy to illustrate the need for equations to be balanced, highlighting the importance of applying the same operations to both sides of the equation to maintain balance and accuracy.

05:07
🔢 Solving Equations with Addition and Subtraction

In this paragraph, the speaker continues the explanation on solving algebraic equations, focusing on the use of addition and subtraction. It demonstrates the process of isolating the unknown by applying the commutative property of addition and the principle of balance. The paragraph provides examples of equations with unknowns on both sides, such as 40 = 25 + x and x - 5 = 16, and explains how to use addition and subtraction to isolate the unknown. It also introduces a variation of the subtraction problem, where the unknown is subtracted from a number, and explains how to handle such cases by adding the unknown to both sides of the equation to avoid getting a negative unknown.

10:10
🧠 Practicing and Understanding Algebraic Equations

This paragraph concludes the video by summarizing the basics of solving simple algebraic equations that involve addition and subtraction. It emphasizes the importance of practice and the applicability of the solution process to various types of equations, including decimals and fractions. The paragraph encourages viewers to apply the learned methods to solve basic equations on their own. It also provides a final thank you and a call to visit the website www.mathantics.com for more information.

Mindmap
Keywords
💡Algebra
Algebra is a branch of mathematics that deals with symbols and the rules for manipulating those symbols. It is a unifying thread, a way of thinking, that can stretch from simple arithmetic problems to problems involving quantities that are changing and those that are unknown. In the context of the video, Algebra is the main theme, as it discusses solving equations with unknown values using basic arithmetic operations. The script uses Algebra as the foundation for teaching how to isolate and solve for variables.
💡Equation
An equation is a mathematical statement that asserts the equality of two expressions. In the video script, equations are presented as problems involving unknown values, or variables, which the viewer is taught to solve. The process involves manipulating the equation to isolate the variable and determine its value, as demonstrated in the examples provided.
💡Variable
A variable is a symbol, often a letter, that stands for an unknown or changeable value. In Algebra, variables are used to represent unknown quantities that we are trying to find. The script explains that the goal of solving an equation is to determine the value of these variables, which is central to the video's educational purpose.
💡Addition
Addition is one of the four basic arithmetic operations, combining two or more numbers to find their total or sum. In the video, addition is used as part of the process to solve equations. For instance, when an unknown variable is added to a number, the script teaches viewers to use subtraction to isolate the variable.
💡Subtraction
Subtraction is the arithmetic operation of removing one quantity from another. The script uses subtraction as a method to undo an addition or to isolate a variable in an equation. For example, if a variable is being subtracted by a number, the viewer is shown how to add that number to both sides of the equation to solve for the variable.
💡Balance Scale
A balance scale is an instrument used to measure the mass of an object by comparing it with known masses. In the script, the balance scale is used as an analogy to explain the concept of balance in equations. It emphasizes that any operation performed on one side of an equation must be performed on the other side to maintain equality, just as adding weight to one side of a scale would upset its balance.
💡Commutative Property
The commutative property states that the order in which certain mathematical operations are performed does not change the result. In the context of the video, the script mentions the commutative property in relation to addition, explaining that adding a number to a variable is the same as adding the variable to the number, which simplifies the process of isolating the variable.
💡Multiplication
Multiplication is the arithmetic operation of scaling one number by another. While the video script does not focus on multiplication in the examples provided, it does mention that in future videos, it will cover equations involving multiplication and division. Multiplication is an important operation in Algebra for scaling variables and constants.
💡Division
Division is the arithmetic operation of splitting a quantity into a number of equal parts or groups. Similar to multiplication, the script does not provide examples involving division in this video but foreshadows its inclusion in subsequent lessons. Division is essential in Algebra for solving equations where a variable is divided by a number.
💡Isolate
To isolate, in the context of Algebra, means to manipulate an equation so that the variable is alone on one side of the equation. The script repeatedly emphasizes the importance of isolating the variable to solve for its value. This is achieved by performing inverse operations on both sides of the equation to eliminate other terms and leave the variable by itself.
Highlights

Algebra involves equations with variables or unknown values.

Solving an equation means determining the unknown values.

This video teaches solving simple algebraic equations with addition and subtraction.

Next video will cover solving equations with multiplication and division.

To solve an equation, isolate the unknown value on one side of the equal sign.

Use arithmetic operations to rearrange equations while maintaining balance.

An equation is balanced like a scale, with equal values on both sides.

Ensure changes to the equation are made to both sides to maintain balance.

Addition, subtraction, multiplication, and division can be used to rearrange equations.

Example: Solving x + 7 = 15 by subtracting 7 from both sides.

The commutative property allows rearranging terms in an equation.

Example: Solving 40 = 25 + x by subtracting 25 from both sides.

To isolate x, subtract what is added to it from both sides.

Example: Solving x - 5 = 16 by adding 5 to both sides.

Example: Solving 10 = x - 32 by adding 32 to both sides.

Handling subtraction of an unknown from a number by rearranging the equation.

Example: Solving 12 - x = 5 by adding x to both sides and then subtracting 5.

The process works the same with decimals, fractions, and any variable symbol.

Importance of practicing solving basic equations.

Transcripts

Browse More Related Video

Algebra Basics: Solving Basic Equations Part 2 - Math Antics

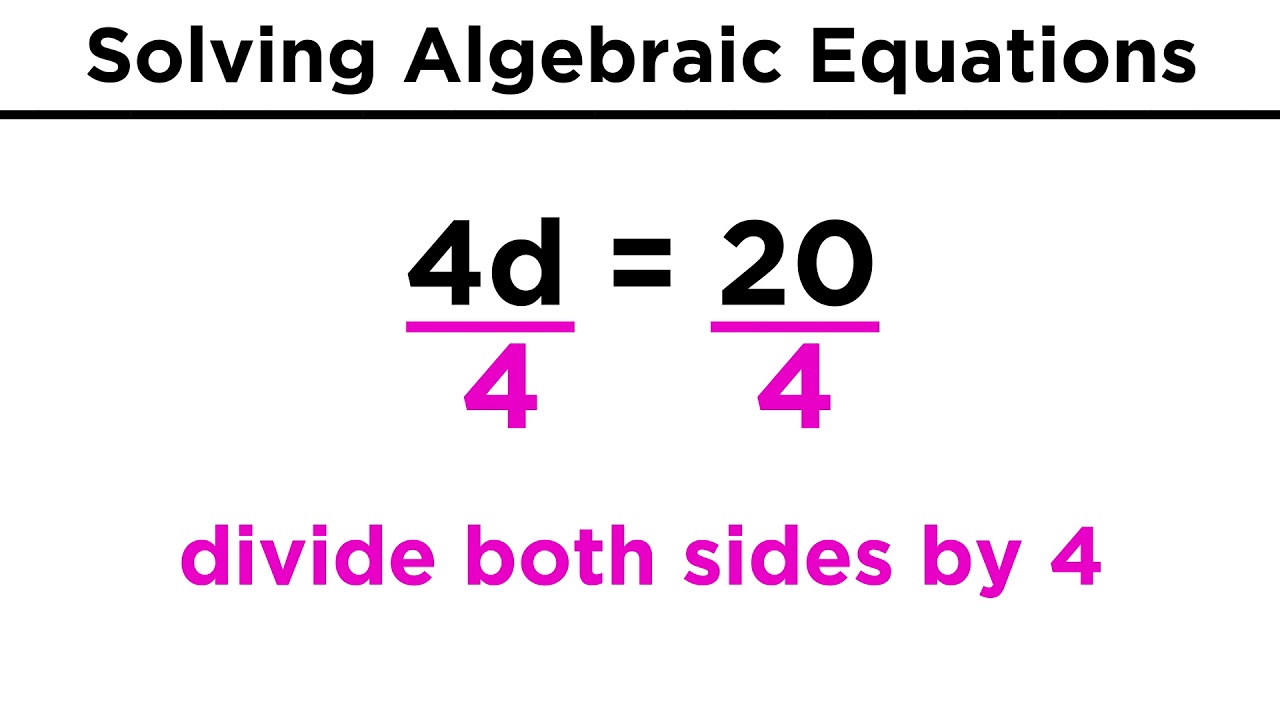
Algebraic Equations and Their Solutions

Algebra Basics: Solving 2-Step Equations - Math Antics

The Maths Prof: Changing Subject of Formula EXAM QUESTIONS

Algebra Basics: The Distributive Property - Math Antics

Algebra Basics: Exponents In Algebra - Math Antics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Algebra BasicsEquation SolvingMath LessonsAdditionSubtractionBalance ScaleUnknown ValuesMath PracticeSimple EquationsEducational Video