Algebra Basics: Solving Basic Equations Part 2 - Math Antics

mathantics
22 May 201509:34
EducationalLearning
32 Likes 10 Comments

TLDRIn this Math Antics video, Rob teaches viewers how to solve simple algebraic equations involving single multiplication or division operations. He emphasizes the importance of balancing equations by performing the same operation on both sides. Through examples, Rob demonstrates how to isolate the unknown variable by using inverse operations: dividing by a number to undo multiplication and multiplying by a number to undo division. He also addresses a tricky case where the unknown is on the bottom of a division, showing how to solve it by multiplying both sides by the variable. The video encourages practice and re-watching for better understanding.

Takeaways
  • 🔍 The video focuses on solving algebraic equations with a single multiplication or division operation.
  • 📚 Key strategy for solving equations is to rearrange them using arithmetic so the unknown is isolated on one side of the equal sign.
  • 💡 When rearranging, whatever operation is done to one side must be mirrored on the other to maintain balance.
  • 🔄 Multiplication and division are inverse operations, which can be used to undo each other in equations.
  • 📝 In algebra, if a number is next to a variable with no operation sign, it implies multiplication.
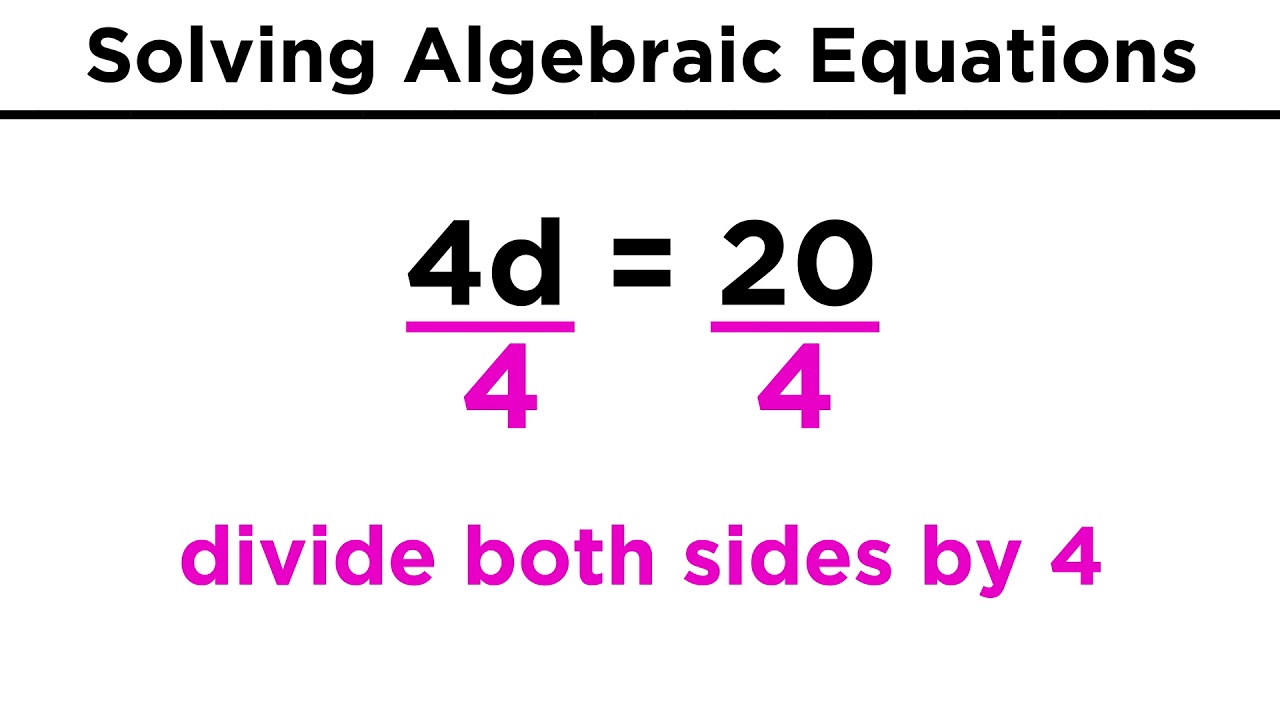
  • 🔢 To solve '3x = 15', divide both sides by 3 to isolate 'x', simplifying to 'x = 5'.
  • 📉 For equations like '12x = 96', dividing both sides by 12 isolates 'x', resulting in 'x = 8'.
  • 📈 Division problems like 'x ÷ 2 = 3' should be rewritten in fraction form for easier solving, leading to 'x = 6'.
  • 🔄 When the unknown is on the bottom in division, such as '4 / x = 2', multiply both sides by 'x' to isolate 'x', which gives 'x = 2'.
  • 🚫 Division does not have the commutative property; 'x / 4' is not the same as '4 / x'.
  • 📝 Practice is essential to solidify understanding of solving one-step algebraic equations involving basic arithmetic operations.
Q & A

  • What is the key strategy for solving an equation with an unknown value?

    -The key strategy for solving an equation with an unknown value is to use arithmetic to rearrange the equation so that the unknown is all by itself on one side of the equal sign.

  • Why is it important to do the same operation on both sides of an equation?

    -It is important to do the same operation on both sides of an equation to keep the equation in balance and ensure that the equation remains true after the operation.

  • What is the relationship between multiplication and division in the context of solving equations?

    -Multiplication and division are inverse operations, meaning they can be used to undo each other when solving equations.

  • How do you solve the equation 3x = 15?

    -To solve the equation 3x = 15, you divide both sides by 3, which simplifies to x = 5.

  • Why is it customary to list the known number first and the unknown number second in multiplication?

    -It is customary to list the known number first and the unknown number second in multiplication because it follows the convention of writing the multiplicand before the multiplier, and it helps in maintaining consistency in algebraic expressions.

  • What is the default operation in algebra when a number and a variable are written next to each other without an operator?

    -The default operation in algebra when a number and a variable are written next to each other without an operator is multiplication.

  • How do you solve the equation 12x = 96?

    -To solve the equation 12x = 96, you divide both sides by 12, which simplifies to x = 8.

  • What should you do if you encounter a division problem written with the traditional division symbol?

    -If you encounter a division problem written with the traditional division symbol, you should rewrite it using the fraction form for division to simplify the process of solving the equation.

  • How do you solve the equation x ÷ 2 = 3?

    -To solve the equation x ÷ 2 = 3, you rewrite it in fraction form as (x/2) = 3, then multiply both sides by 2, which simplifies to x = 6.

  • What is the process for solving an equation where an unknown is on the bottom of a fraction, like in 4/x = 2?

    -To solve an equation where an unknown is on the bottom of a fraction, like 4/x = 2, you multiply both sides by 'x' to cancel out the unknown in the denominator, then divide both sides by 2 to isolate 'x', resulting in x = 2.

  • Why is practice important for learning how to solve equations?

    -Practice is important for learning how to solve equations because it helps to reinforce the understanding of the concepts and techniques involved, ensuring that you can apply them effectively when solving different types of equations.

Outlines
00:00
📚 Introduction to Solving Basic Algebraic Equations

Rob, the host of Math Antics, introduces the topic of solving algebraic equations with a single multiplication or division operation. He emphasizes the importance of isolating the unknown variable and maintaining the balance of the equation by performing the same operation on both sides. The video builds on the concept that multiplication and division are inverse operations, which can be used to solve for the unknown. An example equation, 3x = 15, is presented to illustrate the process of dividing both sides by the coefficient of the unknown to isolate it.

05:02
🔍 Simplifying Equations with Multiplication and Division

This paragraph delves deeper into solving equations involving multiplication and division. It explains the process of rewriting division in fraction form for easier simplification and demonstrates how to cancel common factors to isolate the unknown variable. Examples such as 12x = 96 and x/2 = 3 are used to illustrate the steps of dividing or multiplying both sides by the appropriate number to solve for x. The paragraph also addresses a tricky variation where the unknown is in the denominator, showing how multiplying both sides by the unknown can simplify the equation to a form that can be easily solved.

Mindmap
Keywords
💡Algebraic Equations
Algebraic equations are mathematical statements that assert the equality of two expressions, typically involving one or more variables. In the context of this video, the focus is on simple equations that involve either multiplication or division by a number. The script discusses how to solve these equations by isolating the unknown variable, which is the central theme of the video.
💡Unknown Value
An unknown value, often represented by a variable like 'x', is a quantity that we are trying to determine in an equation. The script emphasizes the importance of using arithmetic to rearrange the equation so that the unknown value is isolated on one side of the equal sign, which is essential for solving the equation.
💡Arithmetic
Arithmetic refers to the branch of mathematics dealing with the properties and manipulation of numbers. In this video, arithmetic is used to describe the process of solving equations by performing operations such as addition, subtraction, multiplication, and division. The script illustrates how arithmetic operations are applied to both sides of an equation to maintain balance and solve for the unknown.
💡Inverse Operations
Inverse operations are mathematical operations that 'undo' each other. In the script, it is mentioned that addition and subtraction are inverse operations, as are multiplication and division. For example, if an unknown is multiplied by a number, division by that number is used to isolate the unknown, illustrating the concept of inverse operations in the context of solving equations.
💡Multiplication
Multiplication is one of the four basic arithmetic operations, where two numbers are combined to find their product. The video script uses multiplication in the context of algebraic equations, explaining that when a number and a variable are written next to each other without an operation sign, it implies multiplication.
💡Division
Division is the arithmetic operation of splitting a number into equal parts. In the script, division is discussed in relation to algebraic equations, where an unknown variable might be divided by a number. The process of solving such equations involves undoing the division by multiplying both sides of the equation by the divisor.
💡Fraction Form
Fraction form is a way of representing division as a ratio of two numbers. In the video, the script explains that dividing an equation by a number is often represented in fraction form, which simplifies the process of isolating the unknown variable by canceling common factors.
💡Simplification
Simplification in mathematics refers to the process of reducing a complex expression to a simpler form. The script demonstrates simplification by canceling common factors in fractions or by performing basic arithmetic to solve for the unknown variable in an equation.
💡Commutative Property
The commutative property states that the order of factors does not change the product in multiplication. However, the script points out that this property does not apply to division, which is why dividing by an unknown (e.g., 'x over 4') is not the same as multiplying by the unknown (e.g., '4 over x').
💡Practice
Practice is the act of repeatedly performing an activity to improve proficiency. The video script concludes by emphasizing the importance of practice in mastering the skill of solving algebraic equations, suggesting that viewers should work through many problems to solidify their understanding.
💡Equation Balance
Equation balance refers to the property that an equation remains true even after the same operation is performed on both sides. The script uses the analogy of not making one side 'jealous' by ensuring that whatever operation is done to one side is mirrored on the other, maintaining the balance of the equation.
Highlights

Introduction to solving basic algebraic equations involving multiplication or division.

Key strategy for solving equations: rearrange the equation to isolate the unknown on one side.

Importance of performing the same operation on both sides to maintain balance in the equation.

Recap of inverse operations: addition/subtraction and multiplication/division undo each other.

Explanation that multiplication is the default operation in algebra when no operator is shown.

Example problem: solving 3x = 15 by dividing both sides by 3 to isolate x.

Simplification process: canceling common factors to solve equations.

Example problem: solving 12x = 96 by dividing both sides by 12 to isolate x.

Introduction to division problems: re-writing division equations in fraction form for simplification.

Example problem: solving x ÷ 2 = 3 by multiplying both sides by 2 to isolate x.

Clarification on using fraction form to better understand and solve division equations.

Example problem: solving x/10 = 15 by multiplying both sides by 10 to isolate x.

Addressing the tricky variation: solving equations where the unknown is in the denominator.

Example problem: solving 4/x = 2 by multiplying both sides by x, then dividing by 2 to isolate x.

Encouragement to practice solving equations and re-watch videos to reinforce understanding.

Transcripts

Browse More Related Video

Algebra Basics: Solving Basic Equations Part 1 - Math Antics

Algebraic Equations and Their Solutions

Algebra Basics: Solving 2-Step Equations - Math Antics

How to Rearrange Equations in Chemistry

Solving Algebraic Equations With Roots and Exponents

Math Antics - Number Patterns

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Algebra SolvingMath TutorialEquation BalancingMultiplicationDivisionInverse OperationsArithmetic RulesEducational ContentMathematicsSolving TechniquesMath Antics