Sets, Indices, and Algebra Test 1 - JS Learning Academy
TLDRIn this educational YouTube video by ASI Chamber Jacob, the host guides viewers through Test 1 on sets, indices, and algebra without the use of a calculator. The video covers simplifying algebraic expressions, factorizing, solving equations using laws of indices, and set operations like union and complement. The host demonstrates problem-solving techniques for quadratic equations and provides step-by-step solutions to various math problems, aiming to enhance viewers' understanding of mathematical concepts. The video concludes with an invitation to join Jets Learning Academy for online tuition in various subjects.
Takeaways
- 📚 The video is a tutorial on 'Sets Indices and Algebra' by ASI Chamber Jacob.
- ✍️ The test covers three topics and requires no calculator usage.
- 🔍 The first question involves simplifying an algebraic expression by removing brackets and combining like terms.
- 📐 The second question demonstrates factorization using the difference of squares formula.
- 🧩 The third question involves solving an equation by balancing the bases of exponents.
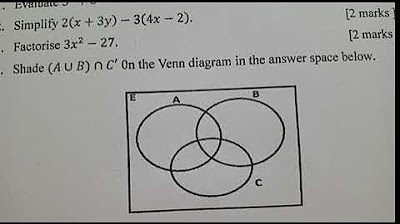
- 🔢 In the fourth question, the concept of union and complement of sets is explained, resulting in an empty set.
- 📉 The fifth question solves a quadratic equation by applying square roots to both sides.
- 📚 The sixth question involves factorizing an expression by grouping common terms.
- 🔑 The seventh question explains how to evaluate expressions with negative exponents, using cube roots.
- 🔄 The eighth question balances bases to solve an equation involving exponents.
- 🤝 The ninth question discusses the intersection of sets and how to find elements not found in the union of two sets.
- 📉 The final question solves a standard form quadratic equation by factorization and finding roots.
Q & A
What is the first topic covered in the video script?
-The first topic covered in the video script is simplifying algebraic expressions.
How does the script simplify the expression 3A - 2A - 3(a - 2B)?
-The script simplifies the expression by first removing the brackets, resulting in 3A - 3A + 6B, and then combining like terms to get the final answer of 4B.
What is the method used to factorize the expression 5(x^2 - 1) in the script?
-The script uses the method of factoring out the common factor, which is 5, and then applies the difference of squares to completely factorize the expression to 5(x - 1)(x + 1).
How does the script solve the equation 25^x = 5?
-The script balances the bases by expressing both sides with the base of 5, resulting in 5^(2x) = 5^1. It then equates the exponents, solving for x to get x = 1/2.
What is the result of the union of sets A and B given in the script?
-The union of sets A and B, as given in the script, includes all elements from both sets, which are 1, 2, 3, 4, 5, 6, 7, and 8.
What is the complement of the union of sets A and B in the script?
-The complement of the union of sets A and B is empty, as the union includes all elements from the universal set E.
How does the script solve the quadratic equation √(2x - 1) = ±5?
-The script solves the quadratic equation by setting up two separate equations: 2x - 1 = 5 and 2x - 1 = -5. Solving these gives x = 3 and x = -2, respectively.
What is the method used to factorize the expression 6ax - 4ay - 3bx + 2by in the script?
-The script uses the method of grouping to factorize the expression, resulting in 3a(x - y) - b(3x - 2y).
How does the script evaluate the expression (125)^(-2/3)?
-The script evaluates the expression by recognizing it as the cube root of the reciprocal of 125, which simplifies to 1/(5^3) or 1/125.
What is the solution to the equation 2^(2x - 1) = 16 in the script?
-The script solves the equation by recognizing that 16 is 2^4, and then equating the exponents to get 2x - 1 = 4, resulting in x = 5/2.
What is the final solution to the quadratic equation x^2 + 7x - 8 = 0 in the script?
-The script solves the quadratic equation by factoring it into (x + 8)(x - 1) = 0, resulting in solutions x = -8 and x = 1.
Outlines
📚 Algebra Test Introduction and Simplification
The video script begins with an introduction to a test on sets, indices, and algebra without the use of a calculator. The first question involves simplifying an algebraic expression by removing brackets and combining like terms, resulting in the simplified form of '4B'. The presenter then proceeds to the next question, which is about factorization using the difference of squares technique.
🔍 Solving Equations and Factorization by Grouping
This paragraph covers solving equations using the laws of indices and factorization by grouping. The presenter balances the bases of an equation involving powers of 5 to find the solution 'x = 1/2'. In the next part, the presenter solves a quadratic equation by applying square roots to both sides, resulting in two solutions, 'x = 3' and 'x = -2'. The paragraph also includes factorization by grouping, where common factors are identified and used to simplify the expression.
🌐 Set Theory and Quadratic Equations
The presenter discusses set theory operations such as union and complement, and applies them to sets A and B, concluding that the complement of the union of A and B is empty. The paragraph also includes solving a quadratic equation by finding factors that satisfy the equation, leading to the solutions 'x = -1' and 'x = 8'. The video concludes with an invitation to join the learning academy for online tuitions in various subjects.
Mindmap
Keywords
💡Simplify
💡Factorize
💡Indices
💡Union
💡Complement
💡Quadratic Equation
💡Factorize by Grouping
💡Negative Power
💡Cube Root
💡Intersect
💡Standard Form
Highlights
Introduction to the YouTube channel 'ASI chamber Jacob' and the test on sets, indices, and algebra without the use of a calculator.
Simplification of algebraic expression 3A - 2A - 3(a - 2B) by removing brackets and combining like terms to get 4B.
Factorization of the expression 5(x^2 - 1) using the difference of squares method.
Solving the equation 25^x = 5 by applying the laws of indices to find x = 1/2.
Listing the union of sets A and B and finding the complement to be empty, indicating all elements are in the union.
Solving the quadratic equation √(2x - 1) = ±5 to find the solutions x = 3 and x = -2.
Factorization by grouping for the expression 6ax - 4ay - 3bx + 2by, highlighting the common factors.
Evaluation of a negative fractional power 125^(-3/2) by taking the cube root of the numerator and denominator.
Balancing bases in the equation 2^(2x - 1) = 2^4 to simplify and solve for x = 1/10.
Finding the complement of sets A and B and then the intersection with set C to identify the element 7.
Solving a quadratic equation by putting it in standard form, finding factors, and factorizing by grouping.
Identifying the solutions to the quadratic equation as x = -1 and x = 8.
Invitation to join Jets Learning Academy for online tuitions in various subjects.
Emphasis on the importance of tests after every three topics to reinforce learning.
Overview of the subjects offered by Jets Learning Academy, including mathematics, science, English, and more.
Closing remarks and a thank you note for watching the video on Test 1.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: