Lesson 3 - Hyperbolic Functions (Calculus 2 Tutor)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRThis advanced calculus tutorial introduces hyperbolic functions, which are essential for students studying engineering and physics. Hyperbolic functions, such as sine, cosine, tangent, and cotangent, have analogous versions to regular trigonometric functions but with a distinct difference in their mathematical representation. The hyperbolic sine, for instance, is derived from the complex exponential form of the sine function, with the imaginary unit 'i' omitted. The tutorial aims to explain the importance of understanding these functions and their derivatives, providing a foundational understanding before delving into problem-solving.

Takeaways
  • πŸ“š The video is part of an advanced calculus tutorial focusing on hyperbolic functions.
  • πŸ” Hyperbolic functions are important for students to know as they appear in engineering and physics studies.
  • πŸ“ˆ The tutorial will cover not only the functions themselves but also their derivatives, which are essential for understanding rates of change.
  • πŸ€” The script poses the question of why hyperbolic functions are important, emphasizing their relevance in various scientific fields.
  • πŸ“ The hyperbolic functions have analogous counterparts to regular trigonometric functions, such as sine, cosine, and tangent.
  • πŸ‘€ The script provides a brief explanation of the sine function's true form in advanced mathematics, involving complex exponentials.
  • πŸ” The hyperbolic sine function is similar to the sine function but with a key difference, as it lacks the imaginary unit 'i' in its formula.
  • πŸ“˜ The hyperbolic sine is defined as "e^x - e^{-x}" over 2, highlighting its relationship with the regular sine function.
  • πŸ’‘ The tutorial aims to present hyperbolic functions and their derivatives, with an intention to solve problems involving these functions.
  • πŸ“ The script serves as motivation and an introduction to the topic, with the detailed exploration of hyperbolic functions and their applications to follow.
Q & A

  • What is the main topic of this tutorial section?

    -The main topic of this tutorial section is hyperbolic functions and their derivatives in the context of advanced calculus.

  • Why are hyperbolic functions important to learn?

    -Hyperbolic functions are important to learn because they appear in various fields such as engineering and physics, and understanding them helps in analyzing certain systems and their behavior.

  • What are some of the hyperbolic functions mentioned in the script?

    -The script mentions hyperbolic sine (sinh), hyperbolic cosine (cosh), hyperbolic tangent (tanh), and hyperbolic cotangent as analogous to regular trigonometric functions.

  • What is the significance of the 'H' in the notation for hyperbolic functions?

    -The 'H' in the notation for hyperbolic functions stands for 'hyperbolic', distinguishing them from the regular trigonometric functions.

  • How does the hyperbolic sine function relate to the regular sine function?

    -The hyperbolic sine function is similar to the regular sine function but without the imaginary unit 'i' in its complex exponential form, making it a real-valued function.

  • What is the complex exponential form of the regular sine function?

    -The complex exponential form of the regular sine function is \( e^{i\theta} - e^{-i\theta} \) over \( 2i \), where \( e \) is the base of the natural logarithm, \( i \) is the imaginary unit, and \( \theta \) is the angle.

  • How is the hyperbolic sine function defined mathematically?

    -The hyperbolic sine function is defined as \( e^x - e^{-x} \) over 2, where \( x \) is the input to the function.

  • What is the purpose of studying derivatives of hyperbolic functions?

    -Studying the derivatives of hyperbolic functions is important to understand the rates of change of these functions, which is a fundamental concept in calculus.

  • Why does the instructor emphasize the importance of knowing what a hyperbolic function is?

    -The instructor emphasizes this because hyperbolic functions are not only part of the advanced calculus curriculum but also have practical applications in fields like engineering and physics.

  • What does the instructor suggest about the relationship between hyperbolic and trigonometric functions?

    -The instructor suggests that hyperbolic functions are analogous to trigonometric functions, with similar forms but adapted for different mathematical and physical contexts.

Outlines
00:00
πŸ“š Introduction to Hyperbolic Functions

This paragraph introduces the topic of hyperbolic functions in the context of advanced calculus. The speaker aims to expand the viewer's mathematical knowledge by discussing hyperbolic functions, which are also covered in calculus textbooks. The importance of understanding these functions is emphasized, as they appear in fields such as engineering and physics. The paragraph also touches on the connection between hyperbolic functions and their trigonometric counterparts, highlighting that hyperbolic functions like sine, cosine, tangent, and cotangent have analogous forms to their regular trigonometric functions. The speaker uses the example of the sine function's complex exponential form to illustrate the relationship and naming convention of hyperbolic functions, specifically the hyperbolic sine function, which is derived by modifying the sine function's formula.

Mindmap
Keywords
πŸ’‘Hyperbolic functions
Hyperbolic functions are a set of mathematical functions that are analogs of the standard trigonometric functions but involve hyperbolas instead of circles. In the video, they are presented as an advanced topic in calculus, which students are expected to learn alongside their derivatives. The script emphasizes the importance of understanding these functions as they appear in fields like engineering and physics.
πŸ’‘Derivatives
Derivatives in calculus represent the rate of change of a function with respect to its variable. The video script discusses how, in addition to learning about hyperbolic functions themselves, students will also need to understand how to calculate their derivatives, which are essential for analyzing the behavior of these functions and solving related problems.
πŸ’‘Trigonometric functions
Trigonometric functions, such as sine and cosine, are fundamental in mathematics and have numerous applications in various fields. The script mentions these functions to draw an analogy with hyperbolic functions, explaining that hyperbolic functions are similar but have distinct properties and applications.
πŸ’‘Hyperbolic sine (sinh)
The hyperbolic sine function, denoted as sinh, is one of the hyperbolic functions. It is defined as (e^x - e^{-x}) / 2. The script explains that it is named 'hyperbolic' because it resembles the standard sine function but with modifications, specifically the absence of the imaginary unit 'i' in its definition.
πŸ’‘Complex exponential
Complex exponentials are expressions of the form e^{ix}, where 'i' is the imaginary unit and 'x' is a real number. In the script, the complex exponential is introduced as the 'true form' of the sine function, which is a more advanced concept that students will encounter in their mathematical studies.
πŸ’‘Unit circle
A unit circle is a circle with a radius of one and is often used in trigonometry to define trigonometric functions. The script uses the unit circle to explain the geometric interpretation of the sine function, which is the y-coordinate of a point on the unit circle at a given angle.
πŸ’‘Advanced math
Advanced math refers to higher-level mathematical concepts and theories that build upon foundational principles. The script mentions advanced math to set the stage for the discussion of hyperbolic functions and their derivation from complex exponentials, indicating that these are not elementary topics.
πŸ’‘Rate of change
The rate of change is a fundamental concept in calculus that describes how one quantity changes in relation to another. In the context of the video, the rate of change is applied to hyperbolic functions, where the derivatives provide information about how these functions behave as their input values change.
πŸ’‘Solutions
In mathematics, a solution refers to the answer to a problem or equation. The script implies that students will not only learn about hyperbolic functions and their derivatives but also how to apply this knowledge to find solutions to problems involving these functions.
πŸ’‘Engineering and Physics
The fields of engineering and physics are mentioned in the script as areas where hyperbolic functions are relevant. This underscores the practical applications of these mathematical concepts, suggesting that understanding hyperbolic functions can be useful in analyzing and designing systems in these disciplines.
Highlights

Introduction to hyperbolic functions in the context of advanced calculus.

Hyperbolic functions are relevant in engineering and physics.

Hyperbolic functions include sine, cosine, tangent, and cotangent.

Hyperbolic sine (sinh) is analogous to the regular sine function.

The true form of the sine function is e^(iΞΈ) - e^(-iΞΈ) / 2i.

Hyperbolic sine is written as e^x - e^(-x) / 2.

Hyperbolic functions are derived from complex exponentials.

Hyperbolic functions are a real version of the sine function.

Hyperbolic functions will be covered in calculus textbooks.

Learning about hyperbolic functions includes understanding their derivatives.

Derivatives of hyperbolic functions are an essential part of the study.

Hyperbolic functions can be found in various systems' behavior analysis.

The importance of recognizing hyperbolic functions in advanced mathematics.

Hyperbolic functions are related to the behavior of certain systems in physics.

Hyperbolic functions are essential for students of calculus to learn.

The presentation will include hyperbolic functions and their derivatives.

Problem-solving with hyperbolic functions will be part of the session.

Transcripts

Browse More Related Video

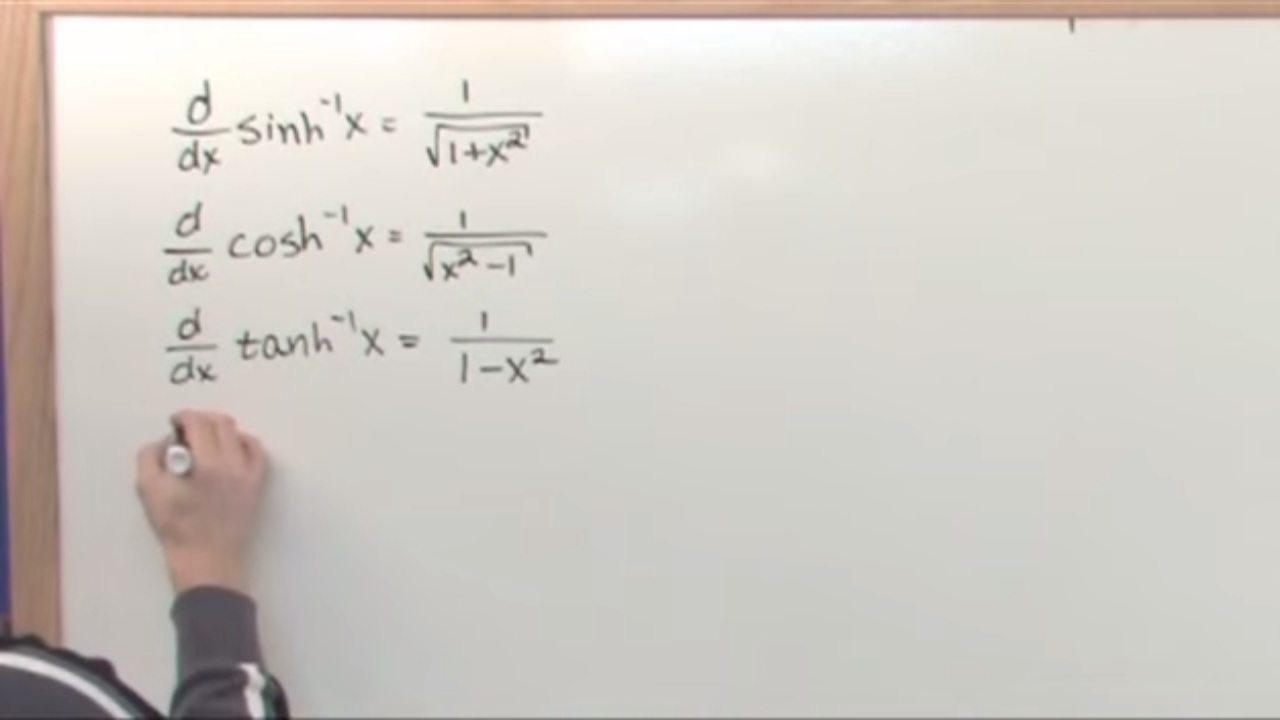
Lesson 4 - Inverse Hyperbolic Functions (Calculus 2 Tutor)

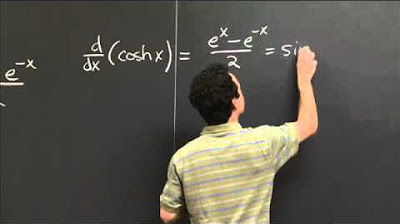
Hyperbolic trig functions | MIT 18.01SC Single Variable Calculus, Fall 2010

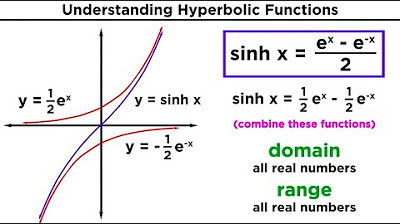
Hyperbolic Functions: Definitions, Identities, Derivatives, and Inverses

Calculus 2 Lecture 6.6: A Discussion of Hyperbolic Functions

Finding Taylor's Series | MIT 18.01SC Single Variable Calculus, Fall 2010

DERIVATIVES that keep getting HARDER 🀯 | jensenmath.ca

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Advanced CalculusHyperbolic FunctionsTrigonometryDerivativesEngineeringPhysicsSine FunctionComplex ExponentialEducational ContentMath Tutorial