Interest (part 2) | Interest and debt | Finance & Capital Markets | Khan Academy

Khan Academy
22 Apr 200808:01
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script explores the concepts of simple and compound interest, using a clear and engaging approach. It begins by explaining the basics of borrowing a principal amount 'P' at an interest rate 'r' over a period 't' years. The script then breaks down the calculations for both simple and compound interest, illustrating the difference between paying a fixed amount of interest annually (simple) versus the interest growing on the accumulated amount (compound). It uses an example of borrowing $50 at a 15% interest rate for 20 years, showing the significant impact of compounding on the final amount owed. The video concludes by emphasizing the importance of understanding the type of interest applied to loans in real-world scenarios.

Takeaways
  • 📚 The script discusses the concept of borrowing money with interest, distinguishing between simple and compound interest.
  • 💰 It defines 'P' as the initial principal amount borrowed, 'r' as the interest rate, and 't' as the time in years the money is borrowed for.
  • 🔢 The formula for simple interest is presented as the principal P plus the interest (r * P), which can be simplified to P * (1 + r).
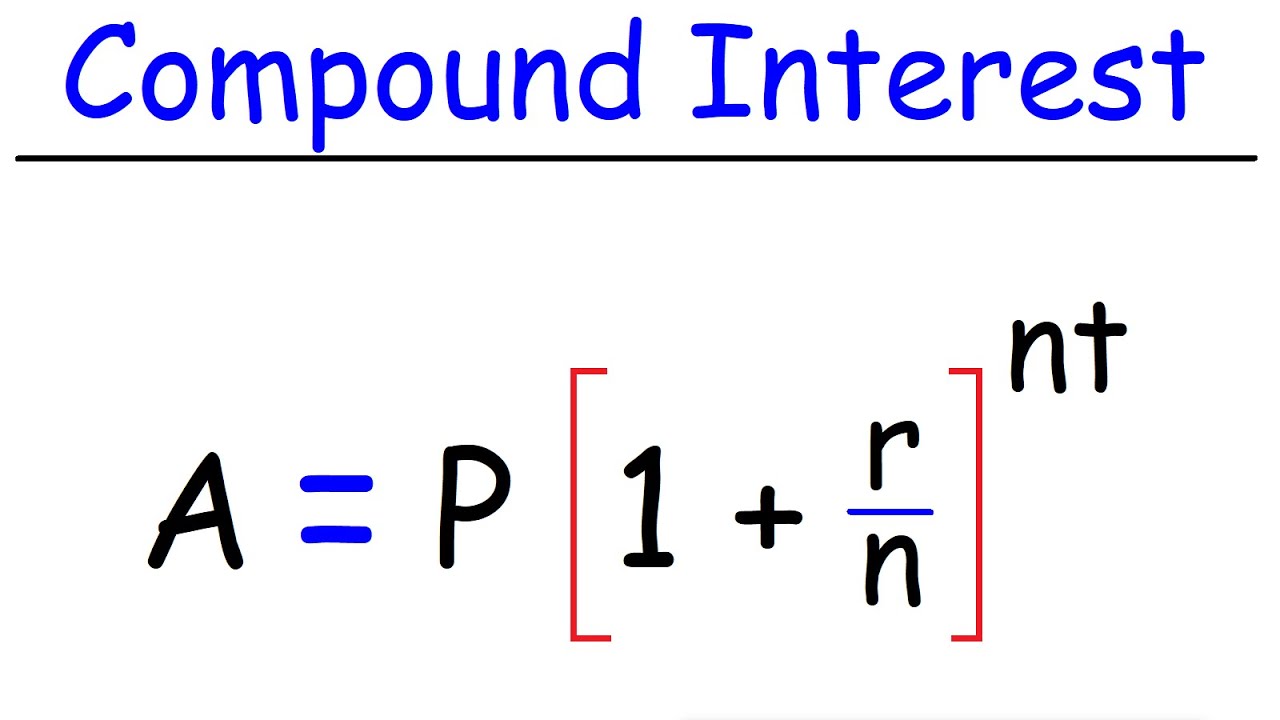
  • 📈 For compound interest, the formula is more complex, with the amount owed after t years being P * (1 + r)^t, indicating that interest is calculated on the accumulated amount of principal and interest from previous periods.
  • 🕒 The difference between simple and compound interest is highlighted, showing that compound interest results in a higher total amount owed over time.
  • 💡 An example is given to illustrate the calculation of simple interest: borrowing $50 at 15% interest for 20 years results in a total payment of $200.
  • 📊 Another example is used for compound interest, showing how borrowing $50 at 15% for 20 years would result in a much higher total payment of approximately $818.
  • 🚨 The importance of understanding whether a loan is offered at simple or compound interest is emphasized, as it significantly affects the total repayment amount.
  • 🌐 The script mentions that in the real world, most interest is compounded, not just annually or semi-annually, but often continuously.
  • 🔮 The concept of continuously compounding interest is introduced, hinting at the use of the mathematical constant 'e' in such calculations.
  • 👋 The presenter invites viewers to watch the next videos for more information on continuously compounding interest and the role of 'e'.
Q & A

  • What is the initial principal referred to in the script?

    -The initial principal, denoted as 'P' in the script, refers to the amount of money that is borrowed at the beginning of the loan period.

  • What does 'r' represent in the context of the script?

    -In the script, 'r' stands for the interest rate at which the money is borrowed, which can also be expressed as 100r%.

  • How is simple interest calculated according to the script?

    -Simple interest is calculated by multiplying the principal (P) by the interest rate (r) for each year, and then adding this amount to the original principal. The formula used is P * (1 + rt), where 't' is the number of years.

  • What is the difference between simple and compound interest as explained in the script?

    -The main difference is that with simple interest, the interest is calculated only on the original principal each year, whereas with compound interest, the interest is calculated on the growing principal, including previously accumulated interest.

  • How does the script illustrate the calculation of compound interest for year 2?

    -For year 2, the script explains that the new principal is the original principal plus the interest from year 1 (P * (1 + r)), and then this new principal is again increased by the interest rate for year 2, resulting in P * (1 + r)^2.

  • What is the general formula for calculating the amount owed after 't' years with compound interest?

    -The general formula for calculating the amount owed after 't' years with compound interest is P * (1 + r)^t.

  • How much would one owe after 20 years if they borrowed $50 at a 15% simple interest rate according to the script?

    -According to the script, if one borrowed $50 at a 15% simple interest rate for 20 years, they would owe $200 at the end of the period.

  • What is the impact of compounding frequency on the total amount owed as mentioned in the script?

    -The script implies that the impact of compounding frequency is significant, as more frequent compounding leads to a higher total amount owed due to the interest being calculated on an increasingly larger principal amount.

  • What does the script suggest about the importance of understanding the type of interest when taking a loan?

    -The script suggests that understanding whether the interest is simple or compound is crucial, as it can significantly affect the total amount to be repaid, with compound interest generally resulting in a higher repayment amount.

  • What is the concept of continuously compounding interest mentioned in the script?

    -Continuously compounding interest is a method of calculating interest where the principal amount is continuously increasing, not just at fixed intervals like annually or semi-annually, but every instant, which can be represented mathematically using the concept of 'e'.

Outlines
00:00
📚 Introduction to Simple and Compound Interest

The speaker begins by reviewing the concept of borrowing money, introducing the terms 'principal', 'interest rate', and 'time'. They explain the difference between simple and compound interest. For simple interest, the formula is derived step by step, showing how the total owed increases linearly with time. The example of borrowing $50 at a 15% interest rate for 20 years is used to illustrate the calculation, resulting in a total payment of $200. The speaker emphasizes the importance of understanding the interest calculation method, as it significantly affects the total amount to be repaid.

05:03
🔍 Exploring Compound Interest and Its Impact

This paragraph delves into the concept of compound interest, contrasting it with simple interest. The speaker explains that with compound interest, the interest earned each period is added to the principal, and the new total becomes the principal for the next period. They use the same $50 at 15% interest rate example but over 20 years to demonstrate the exponential growth of the owed amount due to compounding. The speaker calculates the total owed with compound interest, which results in a significantly higher amount of $818, highlighting the substantial difference compared to simple interest. The paragraph concludes with a teaser for upcoming videos on continuously compounding interest and the mathematical constant 'e'.

Mindmap
Keywords
💡Principal
In the context of the video, 'principal' refers to the initial amount of money that is borrowed or invested. It is the base sum from which interest is calculated. The script clearly defines it as 'P dollars, that's what I borrowed so that's my initial principal.' It is central to the video's theme as it is the starting point for understanding both simple and compound interest calculations.
💡Interest Rate
The 'interest rate' is the percentage at which interest is paid by borrowers to lenders, typically expressed as an annual percentage rate (APR). In the script, it is denoted by 'r' and can be written as '100r%'. It is a key concept in the video as it directly influences the amount of interest accrued over time, whether under simple or compound interest schemes.
💡Simple Interest
Simple interest is calculated only on the principal amount initially borrowed and does not take into account the addition of interest to the principal. The script explains it as 'So at time 0... if I paid it back immediately, I would just owe P, right?' and further illustrates it with an example of borrowing $50 at 15% for 20 years, resulting in a total payment of $200.
💡Compound Interest
Compound interest is interest on a loan or deposit calculated based on both the initial principal and the accumulated interest from previous periods. The video script differentiates it from simple interest by stating 'Now year 2 is where compound and simple interest diverge.' It shows how the interest is compounded annually, leading to a significantly higher total amount owed compared to simple interest.
💡Time Axis
The 'time axis' in the script is used metaphorically to represent the passage of time over which the borrowed money accrues interest. It is introduced when the script says 'So let's make this the time axis-- how much am I going to owe?' and is essential for visualizing the growth of debt or investment over time in the context of interest calculations.
💡Rent on Money
In the script, 'rent on money' is used as a metaphor to describe the interest paid on borrowed money. It is mentioned as 'plus you can kind of, view it as the rent on that money, and that's r times P.' This concept helps to conceptualize interest as a cost of using the money over time, similar to how rent is a cost of using a property.
💡Formula
The term 'formula' in the video refers to the mathematical expressions used to calculate the total amount owed under simple and compound interest. The script emphasizes the importance of understanding these formulas rather than just memorizing them, as seen in 'You could work it out this way, and I recommend you do it. You shouldn't just memorize formulas.'
💡Excel
In the context of the script, 'Excel' refers to the Microsoft Office software used for performing calculations, such as calculating compound interest. The script mentions it when the speaker says 'let me use my Excel' to compute the compound interest for a $50 loan at 15% over 20 years, demonstrating a practical application of the concepts discussed.
💡Continually Compounding Interest
Continually compounding interest, also known as continuous compounding, is a concept mentioned towards the end of the script where interest is calculated an infinite number of times at smaller intervals, rather than annually or semi-annually. The script hints at this concept by stating 'And so you should watch the next several videos on, continuously compounding interest,' suggesting a more complex and real-world application of compound interest.
💡Magic of e
The 'magic of e' is a reference to the mathematical constant e, which is approximately equal to 2.71828 and is the base of the natural logarithm. It is mentioned in the script as part of the introduction to continuously compounding interest, where the speaker says 'then you'll, actually start to learn about the magic of e.' This concept is foundational in understanding the mathematics behind continuous compounding.
Highlights

Introduction to calculating debt owed after borrowing a principal amount (P) with a given interest rate (r) over a period of years (t).

Explanation of simple interest formula: P + (r * P), which simplifies to P * (1 + r).

Example calculation: Borrowing $100 at 10% interest for one year results in a $110 payment.

General formula for simple interest after t years: P * (1 + tr).

Illustration of how simple interest accumulates over multiple years without compounding.

Introduction to compound interest as an alternative to simple interest.

Comparison of simple and compound interest for the first year, showing they are the same.

Explanation of how compound interest works differently in year 2 and beyond, multiplying the new principal by (1 + r) each year.

General formula for compound interest after t years: P * (1 + r)^t.

Example calculation: Borrowing $50 at 15% interest compounded annually for 20 years results in an $818 payment.

Emphasis on the importance of understanding whether a loan uses simple or compound interest due to the significant difference in total payment.

Introduction to the concept of continuously compounding interest and its real-world applications.

Mention of the mathematical constant 'e' in relation to continuously compounding interest.

Encouragement for viewers to watch subsequent videos to learn more about continuously compounding interest and the magic of 'e'.

Highlight of the practical implications of interest calculations in financial decisions.

Recommendation to not just memorize formulas but to understand the process behind interest calculations.

Transcripts

Browse More Related Video

Introduction to interest | Interest and debt | Finance & Capital Markets | Khan Academy

Compound interest and e (part 4) | Exponential and logarithmic functions | Algebra II | Khan Academy

Compound Interest & Population Growth Word Problems - Logarithms

Review of Compound Interest (Precalculus - College Algebra 65)

Compound Interest

Compound interest and e (part 2) | Exponential and logarithmic functions | Algebra II | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Interest CalculationLoan RepaymentFinancial EducationSimple InterestCompound InterestInvestment StrategyMoney ManagementBorrowing MoneyInterest RateEconomic Concepts