Graphing Natural logarithmic functions and Exponential Functions

The Organic Chemistry Tutor
1 Feb 201805:44
EducationalLearning
32 Likes 10 Comments

TLDRThis lesson delves into the art of graphing natural logarithm and exponential functions with base e. The instructor emphasizes that the principles from earlier sections apply here, highlighting the similarities in graphing techniques between regular and natural logs, as well as between exponential functions with different bases. Through step-by-step examples, the video demonstrates how to find key points and asymptotes for functions like e^(x-2)+3 and ln(x-1)+2. The instructor illustrates the process of determining domain and range, and guides viewers in plotting these functions, reinforcing the idea that understanding the fundamental principles of graphing is crucial for tackling more complex mathematical functions.

Takeaways
  • ๐Ÿ“Š The lesson focuses on graphing natural log functions and exponential functions with base e.
  • ๐Ÿ“š The principles for graphing regular logs and exponential functions apply to natural logs and e-based exponentials.
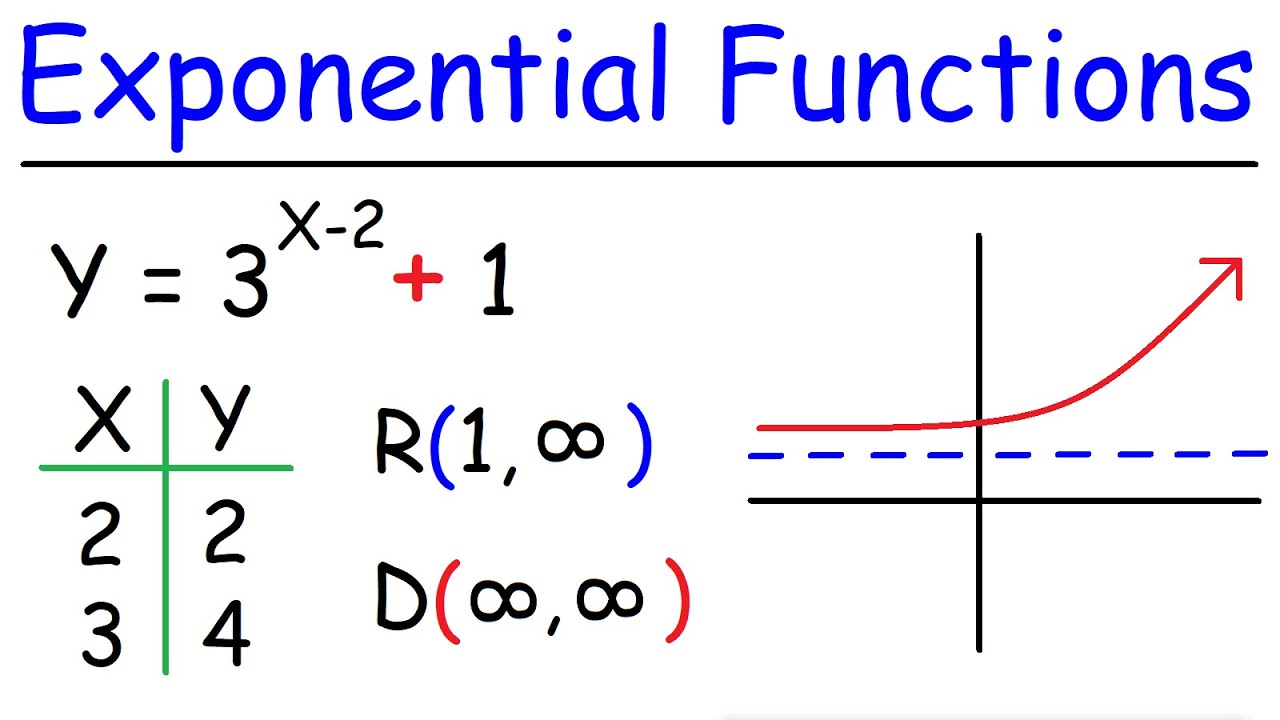
  • ๐Ÿ” To graph e^x - 2 + 3, set the exponent equal to zero and one, then create a table.
  • ๐Ÿงฎ For e^x - 2 + 3, the horizontal asymptote is y = 3.
  • โœ๏ธ When x is 2, y equals 4; when x is 3, y is approximately 5.7.
  • ๐Ÿ“ˆ The domain of e^x - 2 + 3 is all real numbers, and the range is from 3 to infinity.
  • ๐Ÿ“ For ln(x - 1) + 2, set the inside part equal to zero, one, and e to find the values of x.
  • ๐Ÿ›‘ The vertical asymptote for ln(x - 1) + 2 is at x = 1.
  • ๐Ÿ”ข When x is 2, y equals 2; when x is approximately 3.7, y equals 3.
  • ๐Ÿ“‰ The domain of ln(x - 1) + 2 is from 1 to infinity, and the range is all real numbers.
Q & A

  • What is the main focus of the lesson?

    -The lesson focuses on graphing natural log functions and exponential functions with the base e.

  • How is the graphing of natural log functions similar to regular log functions?

    -The graphing techniques used for regular log functions are the same as those used for natural log functions, with the only difference being the base e for natural logs.

  • What is the significance of setting the exponent to zero and one when graphing exponential functions?

    -Setting the exponent to zero and one helps determine key points on the graph, such as the horizontal asymptote and specific y-values for given x-values.

  • What is the horizontal asymptote for the function e^(x-2)+3?

    -The horizontal asymptote for the function e^(x-2)+3 is y = 3, which is determined by the constant term in the function.

  • What are the x-values chosen to find specific points on the graph of e^(x-2)+3?

    -The x-values chosen are 2 and 3, which come from solving the exponent for x to be zero and one, respectively.

  • What is the y-value of the function e^(x-2)+3 when x is equal to 2?

    -When x is equal to 2, the y-value is 4, as e^(0) is 1 and 1 + 3 equals 4.

  • How is the y-value calculated when x is equal to 3 for the function e^(x-2)+3?

    -When x is equal to 3, the y-value is approximately 5.7, calculated as e^(1) which is about 2.7, and 2.7 + 3.

  • What is the domain and range of the function e^(x-2)+3?

    -The domain of the function e^(x-2)+3 is all real numbers from negative infinity to infinity, and the range is from 3 to infinity.

  • What is the vertical asymptote for the natural log function ln(x-1)+2?

    -The vertical asymptote for the natural log function ln(x-1)+2 is x = 1, as this is where the argument of the natural log becomes zero.

  • How are the x-values determined for the natural log function ln(x-1)+2?

    -The x-values are determined by setting the inside of the log function to zero, one, and e, which are key points for graphing.

  • What are the y-values for the natural log function ln(x-1)+2 at specific x-values?

    -The y-values are found by plugging in the specific x-values into the function. For example, when x = 2, y = 2, and when x = e + 1, y = 3.

  • What is the domain and range of the natural log function ln(x-1)+2?

    -The domain of the natural log function ln(x-1)+2 is from 1 to infinity, and the range is all real numbers from negative infinity to infinity.

Outlines
00:00
๐Ÿ“ˆ Graphing Exponential Functions with Base e

This paragraph introduces the process of graphing natural logarithm and exponential functions with base e. The graphing principles from previous sections are applicable here. The example given is the function e^(x-2)+3, where the exponent is set to zero and one to find key points. A table is constructed with x values of 2 and 3, determining the horizontal asymptote at y=3. The y values when x is 2 and 3 are calculated, resulting in points (2,4) and approximately (3,5.7). The graph starts from the asymptote and follows these points, with a domain of all real numbers and a range from 3 to infinity.

05:00
๐Ÿ“š Graphing Natural Logarithm Functions

The second paragraph focuses on graphing natural logarithm functions, using the function ln(x-1)+2 as an example. The inside of the function is set to zero, one, and e to find the vertical asymptote at x=1 and other key points. The y values for these points are calculated, resulting in a graph that starts from the vertical asymptote and follows the points (2,2) and approximately (3.7,3). The domain is from x=1 to infinity, and the range is all real numbers from negative infinity to infinity, showcasing that the techniques for graphing regular log functions apply to natural logs as well.

Mindmap
Keywords
๐Ÿ’กGraphing
Graphing refers to the process of plotting data points on a graph to visualize the relationship between variables. In the video, graphing is used to illustrate the behavior of natural log and exponential functions, which is central to understanding their properties and applications.
๐Ÿ’กNatural Log Functions
Natural log functions, denoted as ln(x), are logarithms with base e (approximately 2.718). They are used in mathematics to describe growth and decay processes. In the script, the natural log function is graphed and analyzed to understand its asymptotic behavior and its relation to exponential functions.
๐Ÿ’กExponential Functions
Exponential functions are mathematical functions where the variable is in the exponent, such as e^x. They are used to model a wide range of phenomena from population growth to compound interest. The video focuses on graphing exponential functions with base e, highlighting their growth towards infinity.
๐Ÿ’กBase e
The base e, or Euler's number, is a mathematical constant approximately equal to 2.718. It is the base of the natural logarithm and is often used in calculus and other mathematical contexts. The script emphasizes the importance of base e in both natural log and exponential functions.
๐Ÿ’กHorizontal Asymptote
A horizontal asymptote is a horizontal line that a function approaches, but never intersects, as the function's input (or x-values) increase or decrease without bound. In the video, y=3 is identified as the horizontal asymptote for the given exponential function, indicating the limit of the function's growth.
๐Ÿ’กVertical Asymptote
A vertical asymptote is a vertical line where a function is undefined and the function's values increase or decrease without bound as the input approaches a certain point. The script identifies x=1 as the vertical asymptote for the natural log function, showing where the function is not defined.
๐Ÿ’กDomain
The domain of a function is the set of all possible input values (x-values) for which the function is defined. In the context of the video, the domain for the exponential function is all real numbers, while for the natural log function, it is x values greater than 1, due to the vertical asymptote at x=1.
๐Ÿ’กRange
The range of a function is the set of all possible output values (y-values) that result from the input values in the domain. The video explains that for the exponential function, the range is from 3 to infinity, and for the natural log function, it is all real numbers from negative infinity to infinity.
๐Ÿ’กTable of Values
A table of values is a method used to determine specific points on the graph of a function by calculating the output for selected input values. In the script, a table is created to find points on the graph of the exponential function e^(x-2)+3 and the natural log function ln(x-1)+2, which helps in plotting the graphs.
๐Ÿ’กGraphing Points
Graphing points refers to the process of plotting specific points on a graph that correspond to the solutions of a function. The video demonstrates how to use points calculated from the table of values to plot the graphs of the exponential and natural log functions, showing how these points guide the shape of the graph.
๐Ÿ’กAsymptotic Behavior
Asymptotic behavior describes how a function approaches a certain value or line without ever reaching it. The video discusses the asymptotic behavior of both the exponential and natural log functions, showing how they relate to their respective horizontal and vertical asymptotes.
Highlights

Lesson focuses on graphing natural log functions and exponential functions with base e.

Principles from previous sections apply to graphing natural logs and exponential functions.

Graphing a regular log is similar to graphing a natural log.

Graphing 2 to the x is analogous to graphing e to the x.

Example graphing e to the (x - 2) + 3 begins with setting the exponent to zero and one.

Horizontal asymptote for e to the x minus two plus three is y equals three.

Y value when x is 2 is 4, found by e to the zero plus 3.

Y value when x is 3 is approximately 5.7, calculated using e to the one plus 3.

Domain for the graph is all real numbers from negative infinity to infinity.

Range for the graph is from 3 to infinity, based on the y values.

Example with natural log function ln(x - 1) + 2 involves setting the inside to zero, one, and e.

Vertical asymptote for ln(x - 1) + 2 is at x equals 1.

Point (2, 2) is found by plugging in x equals 2 into the natural log function.

Point (e + 1, 3) is derived from x equals e + 1 in the natural log function.

Range for the natural log graph is all real numbers from negative infinity to infinity.

Domain for the natural log graph is from 1 to infinity.

Techniques for graphing regular log functions apply to natural log functions, with consideration of e.

Techniques for graphing 2 to the x are transferable to graphing e to the x.

Transcripts

Browse More Related Video

How To Graph Exponential Functions

Calc AB & Calc BC Natural Logs Review

Derivatives of Log Functions

2.3 - Derivatives of Logarithmic Functions

Derivatives of Exponential Functions

Graphing Exponential Functions with Transformations (Precalculus -College Algebra 53)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
GraphingNatural LogExponential FunctionsBase eMathematicsEducationalHorizontal AsymptoteVertical AsymptoteLogarithme to the x