What's so special about Euler's number e? | Chapter 5, Essence of calculus
TLDRThis video script delves into the derivatives of exponential functions, highlighting the unique properties of 'e' to the power of 'x'. It begins with an analogy of population growth to explain the derivative of 2^x, revealing that the rate of change is proportional to the function's value, with a constant of approximately 0.6931. The script then explores how different bases lead to different proportionality constants, culminating in the discovery of the constant 'e' (approximately 2.71828), where the derivative equals the function itself. The video also explains the significance of using 'e' in exponential functions, especially in natural phenomena where rates of change are proportional to the variable itself, showcasing the practicality and elegance of 'e' in calculus applications.
Takeaways
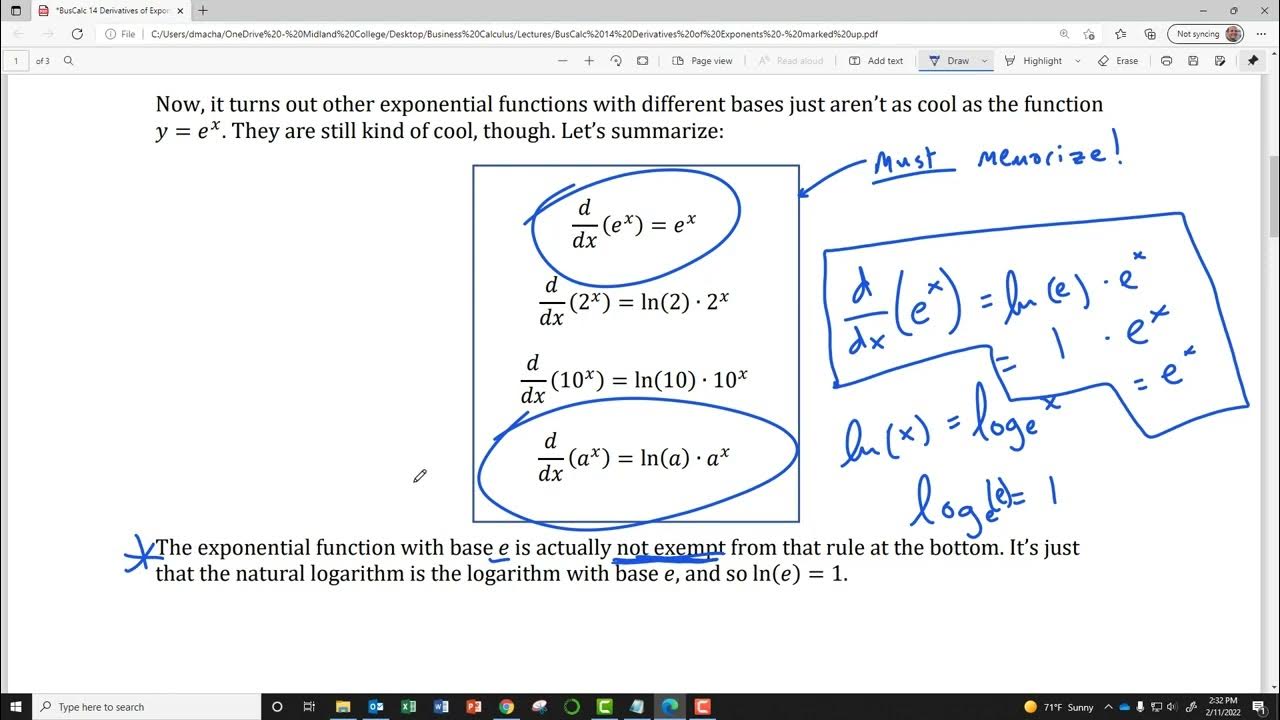
- 📚 The script introduces the concept of derivatives of exponential functions, focusing on functions like 2^x and e^x.
- 🧮 It explains that the derivative of an exponential function is proportional to the function itself, with a constant of proportionality.
- 🔍 The function 2^t is used as an example to illustrate the concept, where t could represent time and 2^t the population size or mass of a hypothetical species.
- 🐾 The rate of growth of the population mass is shown to be equal to the population size at the start of the time period, hinting at the derivative being equal to the function itself.
- 📈 The derivative of 2^t is derived to be 2^t multiplied by a constant, which is found to be approximately 0.6931 when t is very small.
- 🔑 The constant of proportionality for different exponential bases (like 3^t, 8^t) is shown to be related to the natural logarithm of the base.
- 🆚 The number e (approximately 2.71828) is identified as the unique base for which the derivative of e^t is exactly equal to e^t, making it a fundamental constant in mathematics.
- 🌐 The script discusses the importance of e in calculus and how it naturally arises in various applications involving rates of change proportional to the quantity itself.
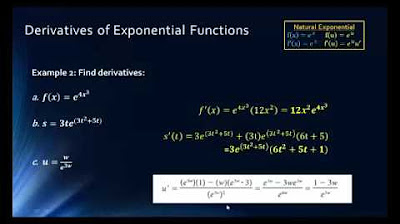
- ⛓ The chain rule is used to explain how the derivative of e^(kt), where k is a constant, is found, emphasizing the relationship between the derivative and the function.
- 🔢 The natural logarithm is shown to be key in understanding the proportionality constants for different bases, with the constant for base 'a' being the natural log of 'a'.
- 📘 The script concludes by emphasizing the preference for writing exponential functions in the form of e^(ct) in applications, due to its clear representation of the rate of change.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is the derivatives of exponential functions, particularly focusing on why e^x is arguably the most important of the exponentials.
Why does the script introduce the concept of population mass to explain the derivative of exponential functions?
-The script introduces the concept of population mass to illustrate the continuity of exponential functions and to make sense of asking about tiny changes in mass over a tiny fraction of time, which is essential for understanding derivatives.
What is the significance of the number 0.6931 mentioned in the script?
-The number 0.6931 is the value that the expression 2^(dt) - 1 / dt approaches as dt approaches 0, which is the proportionality constant for the derivative of 2^t.
How does the script explain the relationship between the derivative of an exponential function and the function itself?
-The script explains that the derivative of an exponential function like 2^t is proportional to itself, with a proportionality constant that is a specific value depending on the base of the exponent.
What property of exponentials allows us to separate the dt term from the t term in the derivative calculation?
-The core property of exponentials that allows this separation is that when you add two values in the exponent, you can express the output as a product, which in this case is 2^t * (2^(dt) - 1) / dt.
What is the special property of the constant e that makes it unique among exponential bases?
-The special property of the constant e is that its proportionality constant in the derivative of e^t is exactly 1, meaning that e^t is equal to its own derivative.
How does the script relate the concept of e to the natural logarithm?
-The script relates e to the natural logarithm by explaining that the derivative of a function like 2^t can be expressed as e^(ln(2) * t), where ln(2) is the natural logarithm of 2, and this is the proportionality constant for the derivative.
What is the chain rule, and how is it used in the script to explain derivatives of exponential functions?
-The chain rule is a fundamental principle in calculus that allows you to compute the derivative of a composite function. In the script, it is used to explain that the derivative of e^(3t) is 3 * e^(3t), by taking the derivative of the outer function (which is e^t) and multiplying it by the derivative of the inner function (which is 3t).
Why is e^x often preferred over other exponential bases in applications of calculus?
-e^x is often preferred because it simplifies the expression of functions where the rate of change is proportional to the variable itself, making the constant in the exponent a natural representation of the proportionality constant.
How does the script connect the concept of exponential growth to real-world phenomena?
-The script connects exponential growth to real-world phenomena by giving examples such as population growth, cooling of hot water, and money investment, where the rate of change is proportional to the current state of the variable.
Outlines
📚 Derivatives of Exponential Functions
The paragraph introduces the concept of derivatives for exponential functions, specifically focusing on the function 2^x. It uses the analogy of a population size or mass that doubles daily to explain the concept of the derivative as the rate of change of the function. The script discusses the intuition behind the derivative of an exponential function and how it relates to the function's value at a given time, suggesting that the derivative of 2^t equals the function itself multiplied by a constant. The paragraph sets the stage for a deeper exploration of the derivatives of exponential functions and their properties.
🔍 The Constant Proportionality in Exponential Derivatives
This paragraph delves into the specifics of the constant of proportionality found in the derivatives of exponential functions. It explains that for any base 'a' raised to the power of 't', the derivative is proportional to the function itself with a constant that depends on the base. The script provides examples with bases 2, 3, and 8, demonstrating how these constants can be calculated and hinting at a pattern. It then introduces the special constant 'e' (approximately 2.71828), which is unique because its derivative equals the function itself, with a proportionality constant of 1. The paragraph also touches on the concept of the natural logarithm and how it relates to these constants, setting the stage for understanding the broader implications of these proportionality constants.
🌟 The Significance of the Number 'e' and Exponential Functions
The final paragraph discusses the significance of the number 'e' in the context of exponential functions and their derivatives. It explains that 'e' is the base for which the derivative of the exponential function equals the function itself, with a proportionality constant of 1. The script uses the chain rule to illustrate how derivatives of exponential functions involving 'e' are calculated and emphasizes the natural logarithm's role in determining the proportionality constants for different bases. It also highlights the practical applications of exponential functions in various natural phenomena, where rates of change are proportional to the quantities themselves, and underscores the preference for expressing exponential functions in terms of 'e' due to its meaningful representation of the proportionality constant.
Mindmap
Keywords
💡Exponential Functions
💡Derivative
💡Rate of Change
💡Constant of Proportionality
💡Base of Exponentiation
💡Natural Logarithm
💡Chain Rule
💡Tangent Line
💡Proportionality
💡Natural Phenomena
Highlights
Introduction to derivatives of exponential functions, focusing on 2^t and e^t.
Intuitive explanation of 2^t as a population size that doubles every day.
The rate of change of 2^t over a full day is equal to the population size at the start of the day.
For smaller changes in time (dt), we need to understand the difference between 2^(t+dt) and 2^t.
2^(t+dt) can be expressed as 2^t * 2^dt, demonstrating a key property of exponents.
The derivative of 2^t is proportional to itself, with a proportionality constant of approximately 0.6931.
The proportionality constant for other bases: 1.0986 for 3^t, 2.079 for 8^t.
e^t is the unique exponential function where the derivative is equal to itself.
Explanation of the natural logarithm and its role in defining the base e.
Deriving the derivative of e^(3t) using the chain rule.
The proportionality constant for the derivative of a^t is the natural logarithm of the base a.
Expressing exponentials in terms of e simplifies understanding their properties.
Natural phenomena often involve rates of change proportional to the changing variable itself.
Examples: population growth, cooling of hot water, and investment growth.
Conclusion: Expressing exponentials as e^(constant*t) gives the constant a meaningful interpretation.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: