Business Calculus - Math 1329 - Section 4.3 - Derivatives of Exponential and Logarithmic Functions
TLDRThis video script is an educational walkthrough on differentiation involving exponential and logarithmic functions. It begins with an interactive group activity to find a limit by creating a table of values, encouraging viewers to pause and solve it themselves. The script then delves into calculating the derivative of e^x, revealing its unique property of being equal to itself, which signifies that the rate of change of the function is equal to the function's value at any given point. Several examples follow, illustrating the use of product and chain rules to find derivatives of more complex functions, including those involving e^x and logarithms. The video also discusses monotonicity, extrema, and inflection points. Key formulas for derivatives of exponential and logarithmic functions are emphasized, and the script concludes with applying these concepts to practical problems, such as calculating revenue and marginal revenue for a demand function. The comprehensive explanation is aimed at reinforcing the understanding of calculus concepts and their real-world applications.
Takeaways
- 📚 In section 4.3, the focus is on differentiation with exponential and logarithmic functions, applying calculus properties to these types of functions.
- 🔢 A group activity is suggested to find a limit by creating a table of values and decreasing H towards zero to understand the concept of limits.
- 🎓 The derivative of e^x is found using the limit definition of a derivative, resulting in e^x itself, which is a unique property as it's one of the two functions whose derivative equals the function.
- 📈 The rate of change (derivative) of the e^x function at any point is equal to the y-value of the function at that point, indicating a constant rate of change proportional to the function's value.
- ✅ Example problems demonstrate the use of the product rule and chain rule for finding derivatives of functions involving exponential and logarithmic expressions.
- 🔍 To analyze the behavior of functions, such as increasing/decreasing (monotonicity), extrema (max/min), and inflection points, derivatives are crucial.
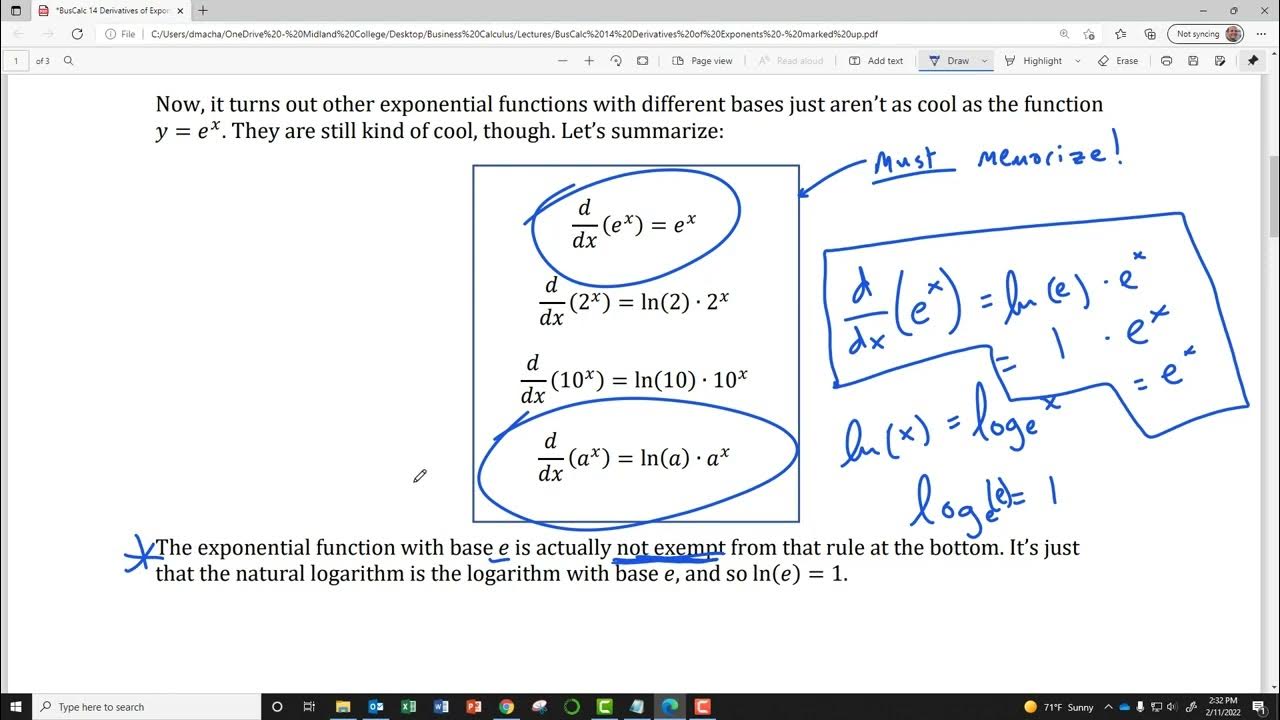
- 📌 Memorizing the derivatives of exponential functions (b^x) and logarithmic functions (ln(x) and log_b(x)) is essential for solving calculus problems.
- 🤔 The derivative of b^x (where b > 0 and b ≠ 1) is b^x times the natural log of b, highlighting the importance of the base of the exponential function in the derivative.
- 🧮 The derivative of ln(x) is 1/x, and for log_b(x) it's 1/x times the natural log of b, which corrects a common mistake of confusing the base with the variable.
- 🔑 The natural logarithm function fills a gap in derivatives of polynomials, allowing for the derivative of x^(-1) to be represented as 1/x or ln(x).
- 🍫 A real-world example of a price-demand function for chocolate candies is used to demonstrate how to find revenue and marginal revenue functions, and how marginal analysis can estimate additional revenue from increased production.
Q & A
What is the main focus of section 4.3 in the transcript?
-The main focus of section 4.3 is differentiation with exponential and logarithmic functions, applying calculus properties to these functions, and discussing concepts such as monotonicity, extrema, and inflection points.
How does the group activity in this section relate to limits?
-The group activity involves finding a limit by creating a table of values and decreasing the value of H towards zero. This is done to understand the behavior of a function as H approaches zero, which is a fundamental concept in calculus for determining limits.
What is the derivative of e^x, and why is it significant?
-The derivative of e^x is itself, e^x. This is significant because it is one of the only two functions whose derivative is exactly equal to the function. It implies that the rate of change of the function e^x at any point is equal to the value of the function at that point, which is a unique property in calculus.
How is the product rule applied in the example where f(x) = x^4 * e^x?
-The product rule is applied by taking the derivative of both factors in the function and adding them together. For f(x) = x^4 * e^x, the derivative F'(x) is calculated as 4x^3 * e^x (derivative of the first factor times the second factor) plus x^4 * e^x (first factor times the derivative of the second factor).
What is the chain rule, and how is it used in the context of derivatives?
-The chain rule is used when taking the derivative of a composite function, which is a function made up of other functions. It involves taking the derivative of the outer function and then multiplying it by the derivative of the inner function. This rule is essential for differentiating complex functions involving exponents and logarithms.
How does the sign chart help in determining the increasing and decreasing intervals of a function?
-A sign chart is a tool used to determine where a function is increasing or decreasing. By analyzing the sign of the derivative at different intervals, we can conclude whether the function's slope is positive (increasing) or negative (decreasing) in those intervals.
What is the significance of finding horizontal tangent lines in a function?
-Horizontal tangent lines are significant because they indicate points of inflection or critical points where the function's slope is zero. These points help us understand the behavior of the function, and they can represent local maxima, local minima, or points of inflection.
How does the base of an exponential function affect the rate of change?
-The base of an exponential function affects the rate of change by scaling the regular exponential graph. The derivative of an exponential function B^x is B^x times the natural log of B, which means that different bases result in different rates of change or slopes for the function at any given point.
What is the relationship between the power rule and the natural log function in derivatives?
-The power rule states that the derivative of x^n is n * x^(n-1). When n is negative one, the derivative is 1/x, which can be understood as the derivative of the natural log function. This relationship fills the gap in the power rule for negative exponents and connects polynomial and logarithmic functions in calculus.
How is marginal analysis used in the context of the price demand function?
-Marginal analysis is used to determine the additional revenue or cost from making a small change in production or sales. In the context of the price demand function, the marginal revenue function gives us the approximate increase in revenue from selling an additional unit of product.
What is the process for finding the revenue and marginal revenue functions for a given price demand function?
-To find the revenue function, we multiply the price demand function by the quantity. For the marginal revenue function, we take the derivative of the revenue function. This gives us the rate of change of revenue with respect to the quantity sold, which can be used for decision-making in terms of production and sales strategies.
Outlines
📚 Introduction to Exponential and Logarithmic Differentiation
This paragraph introduces the topic of differentiation with exponential and logarithmic functions. It emphasizes the application of calculus properties to these functions, including finding derivatives, analyzing business calculus problems, and understanding concepts like monotonicity, extrema, and inflection points. A group activity is suggested to find a limit by creating a table of values as H approaches zero. The paragraph concludes with an example of computing the derivative of e^X, demonstrating that the derivative of this function is the function itself, a unique property.
🔄 Derivatives of Exponential Functions and Product Rule
The paragraph delves into the concept that the derivative of e^X is e^X, highlighting the rate of change of the function equals the function's Y value at each point. It then proceeds to solve example problems using this property and the product rule. The examples include finding derivatives of functions combining polynomials with exponential terms and applying the chain rule when the exponent is a function of X.
📈 Analyzing the Behavior of Exponential Functions
This section focuses on analyzing the behavior of exponential functions, specifically where they have horizontal tangent lines, and determining intervals of increase and decrease. It also covers how to find extrema of a function. An example is provided to illustrate these concepts, showing how to find critical values, use a sign chart, and identify the nature of the extrema (relative or absolute minimum).
🧮 Derivatives of Logarithmic Functions and Memorization Tips
The paragraph discusses the derivatives of logarithmic functions, both the natural logarithm and logarithms with arbitrary bases. It emphasizes the importance of memorizing these derivatives for various base functions. The paragraph also clarifies common mistakes students make, such as confusing the natural log of the variable with the natural log of the base. Example problems are provided to demonstrate the application of these derivatives.
🔢 Power Rule and Natural Logarithm Derivatives
This part of the script connects the power rule for polynomials to the natural logarithm function. It explains the gap in derivatives for negative exponents and how the natural logarithm fills this gap. The paragraph also includes a proof that the derivative of e^X equals e^X, which is a special case of the general formula for derivatives of B^X, where B is the base and X is the exponent.
📉 Chain Rule and Revenue Functions in Economics
The paragraph covers the application of the chain rule to more complex functions, including those raised to a power. It provides an example of finding the derivative of a function that combines a logarithm with a polynomial raised to a power. The concept of revenue and marginal revenue functions is introduced, using an economic example of a price-demand function for chocolate candies. The paragraph concludes with an application of marginal analysis to estimate the additional revenue from selling an extra 1,000 candies.
💰 Marginal Analysis in Revenue Estimation
The final paragraph focuses on using marginal revenue to estimate the additional revenue from increasing production. It explains the concept of marginal analysis in the context of the chocolate candy example, showing how to calculate the approximate revenue from selling an additional 1,000 candies based on the marginal revenue function. The calculation is performed, resulting in an additional revenue of approximately $1,076.
Mindmap
Keywords
💡Derivative
💡Exponential Function
💡Logarithmic Function
💡Chain Rule
💡Product Rule
💡Monotonicity
💡Extrema
💡Inflection Points
💡Limit
💡Natural Logarithm
💡Marginal Analysis
Highlights
Differentiation of exponential and logarithmic functions is discussed.
Application of calculus properties to exponential and logarithmic functions is covered, including derivatives, monotonicity, extrema, and inflection points.
A group activity is suggested to find a limit by creating a table of values as H approaches zero.
The derivative of e^x is computed, revealing it to be e^x itself, a unique property.
The rate of change in the e^x function is shown to be equal to the function's Y value at each point.
The product rule is applied to find the derivative of a function that is a product of two other functions.
Chain rule is used to find the derivative of a function involving e to a non-linear exponent.
The derivative of a function involving a product and an exponential with a variable exponent is simplified.
Criteria for horizontal tangent lines, increasing, and decreasing functions are explored.
The process to find extrema of a function is demonstrated using both first and second derivative tests.
Memorization of derivatives for exponential and logarithmic functions is emphasized.
The derivative of natural log and log base B functions are detailed, highlighting common mistakes to avoid.
The power rule for derivatives is connected to the natural log function to fill a gap in derivative powers.
A proof is provided to show that the derivative of e^x is a special case of the general formula for derivatives of B^x.
The chain rule is applied to find the derivative of a logarithmic function with a squared argument.
Multiple chain rules are utilized to find the derivative of a complex function involving exponentiation and logarithms.
The revenue and marginal revenue functions for a price-demand function of chocolate candies are derived and analyzed.
Marginal analysis is used to determine the approximate revenue from selling an additional 1,000 candies.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: