Why hyperbolic functions are actually really nice

Dr. Trefor Bazett
30 Oct 202316:03
EducationalLearning
32 Likes 10 Comments

TLDRThis video delves into the fascinating world of hyperbolic functions, exploring their geometric connection to hyperbolas and their applications in various mathematical fields. Beginning with the analytical perspective, the video introduces hyperbolic cosine and sine as even and odd components of the exponential function e^x. It then transitions to a geometric interpretation, defining these functions based on the areas enclosed by points on a hyperbola. The presenter demonstrates that the analytical and geometric definitions align, showcasing the unity between the two. The video also touches on the relationship between hyperbolic functions and Euler's identity, presenting them as complementary aspects of complex functions. A sponsor plug for Brilliant.org, an interactive learning platform, concludes the video.

Takeaways
  • πŸ“š The video discusses the connection between hyperbolic functions and their geometric representation related to hyperbolas.
  • πŸ” Hyperbolic functions often appear in various mathematical contexts such as bubbles, the math of war, and hyperbolic geometry.
  • πŸ“‰ The video starts by examining the analytical side of hyperbolic functions, beginning with the function e^x and its transformations.
  • πŸ“ˆ Hyperbolic cosine (cosh) and hyperbolic sine (sinh) are defined as the even and odd components of the exponential function e^x.
  • πŸ“Š The video explains how to graph hyperbolic functions and how they visually represent even and odd functions.
  • πŸ”’ The Taylor series expansion for e^x is used to derive power series definitions for hyperbolic cosine and sine.
  • πŸ” The video shows the relationship between the derivatives of hyperbolic functions and their connection to the derivatives of regular trigonometric functions.
  • πŸ“ The geometric definition of hyperbolic functions is explored by considering the areas enclosed by points on a hyperbola.
  • 🌐 The video connects the geometric definition of hyperbolic functions to their analytical definition, showing that they are indeed the same.
  • πŸŽ“ The video concludes by discussing the complex function perspective and how Euler's identity relates to hyperbolic functions.
  • πŸ‘¨β€πŸ« The video is sponsored by Brilliant.org, an online learning platform that offers interactive courses to improve mathematics skills.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is hyperbolic functions, exploring their connection to the geometry of hyperbolas and their applications in various mathematical contexts.

  • How does the video introduce the concept of hyperbolic functions?

    -The video introduces hyperbolic functions by first discussing the properties of even and odd functions, and then by showing how hyperbolic cosine and hyperbolic sine can be derived as the even and odd components of the exponential function e^x.

  • What is the geometric interpretation of hyperbolic cosine and hyperbolic sine?

    -The geometric interpretation of hyperbolic cosine and hyperbolic sine is that they represent the horizontal and vertical components, respectively, of a point on a hyperbola that encloses a specific area.

  • How are hyperbolic functions related to the equation of a hyperbola?

    -Hyperbolic functions are related to the equation of a hyperbola because when x is defined as hyperbolic cosine of theta and y as hyperbolic sine of theta, the equation x^2 - y^2 = 1 is satisfied, which is the standard form of a hyperbola.

  • What is the significance of the area property in defining hyperbolic functions geometrically?

    -The area property is significant because it allows for a geometric definition of hyperbolic functions where the area enclosed by a point on the hyperbola determines the values of hyperbolic cosine and hyperbolic sine, providing a visual and intuitive understanding of these functions.

  • How does the video connect the analytic definition of hyperbolic functions to their geometric definition?

    -The video connects the analytic definition (as even and odd components of e^x) to the geometric definition by showing that the area under the hyperbola defined by hyperbolic functions is equal to the parameter used in their definition, thus proving that the two definitions are equivalent.

  • What is the role of integration in finding the area under the hyperbola?

    -Integration is used to calculate the area under the hyperbola by summing up an infinite number of infinitesimally small vertical strips (slices) between the hyperbola and the x-axis, which is a common technique in calculus for finding areas.

  • How does the video relate hyperbolic functions to complex functions?

    -The video relates hyperbolic functions to complex functions by drawing a parallel between Euler's identity e^(iΞΈ) = cos(ΞΈ) + i*sin(ΞΈ) and the definition of hyperbolic functions as the sum of hyperbolic cosine and hyperbolic sine, suggesting that they are different aspects of a larger mathematical structure.

  • What is the role of the exponential function e^x in defining hyperbolic functions?

    -The exponential function e^x plays a crucial role in defining hyperbolic functions as it is used to derive the even and odd components that correspond to hyperbolic cosine and hyperbolic sine, respectively.

  • Why are hyperbolic functions important in mathematics?

    -Hyperbolic functions are important in mathematics because they have wide-ranging applications in various fields, including the study of bubbles, the math of war, and the geometry of hyperbolic spaces, as mentioned in the video.

  • What is the educational platform recommended in the video for improving mathematical skills?

    -The educational platform recommended in the video is Brilliant.org, which offers interactive courses and animations to help learners visualize and understand mathematical concepts.

Outlines
00:00
πŸ“š Introduction to Hyperbolic Functions

The video begins by discussing hyperbolic functions, which are often encountered in complex mathematical expressions. The speaker explains how these functions are geometrically connected to hyperbolas, which have various applications in mathematics. The video starts by examining the properties of exponential functions and their relation to even and odd functions. It then introduces the concepts of hyperbolic cosine and hyperbolic sine as even and odd components of the exponential function, respectively. The speaker also covers the graphical representation of these functions and their connection to the Taylor series of the exponential function.

05:00
πŸ“ Geometry of Hyperbolas and Hyperbolic Functions

The second paragraph delves into the geometric aspect of hyperbolic functions. The speaker presents plots of a circle and a hyperbola, highlighting their similarities. Using the Pythagorean identity, the video connects trigonometric functions to the circle's equation and then explores a similar relationship for hyperbolas. The hyperbolic cosine and sine are shown to satisfy the hyperbola's equation, and the video discusses why this particular parametrization is significant. The geometric definitions of trigonometric functions are revisited, and the video presents a method to define hyperbolic functions based on the area enclosed by a point on the hyperbola and the x-axis.

10:02
πŸ” Proving the Area Property of Hyperbolic Functions

In this paragraph, the speaker aims to prove that the geometric definition of hyperbolic functions aligns with their analytic definition. The video describes how to calculate the area enclosed by the hyperbola, a point, and the x-axis using integration. By subtracting the area of a specific region under the hyperbola from the area of a right triangle, the video establishes that the area is indeed proportional to the hyperbolic cosine and sine of the point's parameter. The speaker then shows that the area property holds true for the analytic definition of hyperbolic functions, confirming that the two definitions are equivalent.

15:04
🌐 Complex Functions and the Connection to Trigonometry

The final paragraph touches on the topic of complex functions, drawing a parallel between the famous Euler's identity and the definitions of hyperbolic functions. The video suggests that cosine and hyperbolic cosine, as well as sine and hyperbolic sine, can be seen as different facets of a broader mathematical concept. The speaker emphasizes the importance of engaging with mathematics actively and promotes the use of an online learning platform, Brilliant.org, for enhancing mathematical skills through interactive courses. The video concludes with an invitation for viewers to explore the platform and a teaser for more mathematical discussions in future videos.

Mindmap
Keywords
πŸ’‘Hyperbolic functions
Hyperbolic functions are mathematical functions that resemble the shape of a hyperbola. They are analogous to the more familiar trigonometric functions but are defined in terms of exponential functions. In the video, the presenter explains that hyperbolic functions are not only mathematically interesting but also have geometric interpretations related to hyperbolas, which are conic sections with two separate branches. The video delves into the definitions and properties of hyperbolic cosine and hyperbolic sine, showing how they are connected to the geometry of hyperbolas.
πŸ’‘Even and odd functions
In mathematics, even functions are those where the function value remains the same when the sign of the input is changed (f(-x) = f(x)), while odd functions have values that are the negative of the original when the sign of the input is flipped (f(-x) = -f(x)). The video uses these concepts to define hyperbolic cosine as an even function and hyperbolic sine as an odd function, derived from the exponential function e^x by considering its even and odd components.
πŸ’‘Hyperbolic cosine (cosh)
Hyperbolic cosine, often denoted as cosh, is a hyperbolic function that represents the even component of the exponential function e^x. It is defined as cosh(x) = (e^x + e^(-x))/2. In the video, the presenter shows how cosh(x) can be graphed and how it relates to the geometry of a hyperbola, satisfying the equation x^2 - y^2 = 1.
πŸ’‘Hyperbolic sine (sinh)
Hyperbolic sine, denoted as sinh, is the odd component of the exponential function e^x and is defined as sinh(x) = (e^x - e^(-x))/2. The video explains that sinh(x) is an odd function and, like cosh(x), it is related to the hyperbola, satisfying the same equation x^2 - y^2 = 1.
πŸ’‘Taylor series
A Taylor series is a representation of a function as an infinite sum of terms calculated from the values of the function's derivatives at a single point. In the video, the presenter uses the Taylor series expansion of e^x to break down the exponential function into its even and odd components, which correspond to the hyperbolic cosine and sine functions, respectively.
πŸ’‘Geometric definition
The geometric definition of a function refers to defining it based on its graphical representation or geometric properties. The video presents a geometric definition of hyperbolic functions by considering the areas enclosed by points on a hyperbola and relating these areas to the horizontal and vertical components of those points, which correspond to cosh and sinh.
πŸ’‘Parametric representation
A parametric representation of a curve describes the curve using a parameter that varies over a range. In the context of the video, the presenter mentions that there are many ways to parametrically travel a hyperbola, and the hyperbolic functions cosh and sinh represent one such parametrization.
πŸ’‘Euler's identity
Euler's identity is a famous equation in mathematics that states e^(iΟ€) + 1 = 0. It is often considered one of the most beautiful equations due to its concise representation of fundamental mathematical constants. The video draws a parallel between Euler's identity and the definitions of hyperbolic functions, suggesting a deeper connection between complex exponentials and hyperbolic functions.
πŸ’‘Integration
Integration is a fundamental concept in calculus that involves finding the area under a curve or the accumulated value of a function. In the video, the presenter uses integration to calculate the area enclosed by a hyperbola and relates this area to the definition of hyperbolic functions, showing that the area is equal to the parameter used in the geometric definition.
πŸ’‘Brilliant.org
Brilliant.org is an online learning platform mentioned in the video that offers interactive courses in various subjects, including mathematics. The video's sponsor is used as an example of a resource that can help viewers improve their mathematical skills and engage with mathematical concepts in a hands-on manner.
Highlights

Introduction to the concept of hyperbolic functions and their geometric connection to hyperbolas.

Exploration of the analytic side of hyperbolic functions starting with the function e^x.

Explanation of flipping functions over the y-axis and x-axis to derive new functions.

Definition of even and odd functions and their properties.

Introduction of F_even and F_odd as the even and odd components of any function f(x).

Demonstration of how any function can be written as the sum of an even and an odd component.

Specific function e^x is used to derive hyperbolic cosine and hyperbolic sine as even and odd components.

Graphical representation of hyperbolic cosine and hyperbolic sine functions.

Connection between Taylor series expansion of e^x and the definitions of hyperbolic cosine and sine.

Derivative relationships between hyperbolic cosine and hyperbolic sine, similar to sine and cosine.

Geometric representation of hyperbolas and their equations compared to circles.

Parametric satisfaction of the hyperbola equation by hyperbolic cosine and sine.

Geometric definition of trigonometric functions based on the unit circle area.

Introduction of the geometric definition of hyperbolic functions based on the hyperbola area.

Derivation of the area under the hyperbola curve using integration.

Proof that the geometric and analytic definitions of hyperbolic functions are consistent.

Complex function perspective on hyperbolic functions and their relation to Euler's identity.

Sponsorship mention of Brilliant.org as an online learning platform for mathematics.

Encouragement for viewers to engage with the material and practice mathematics.

Transcripts

Browse More Related Video

Hyperbolic trig functions | MIT 18.01SC Single Variable Calculus, Fall 2010

Hyperbolic Functions: Definitions, Identities, Derivatives, and Inverses

Lesson 3 - Hyperbolic Functions (Calculus 2 Tutor)

Proving the Most Beautiful Equation Bob Ross Style

Trigonometry fundamentals | Ep. 2 Lockdown live math

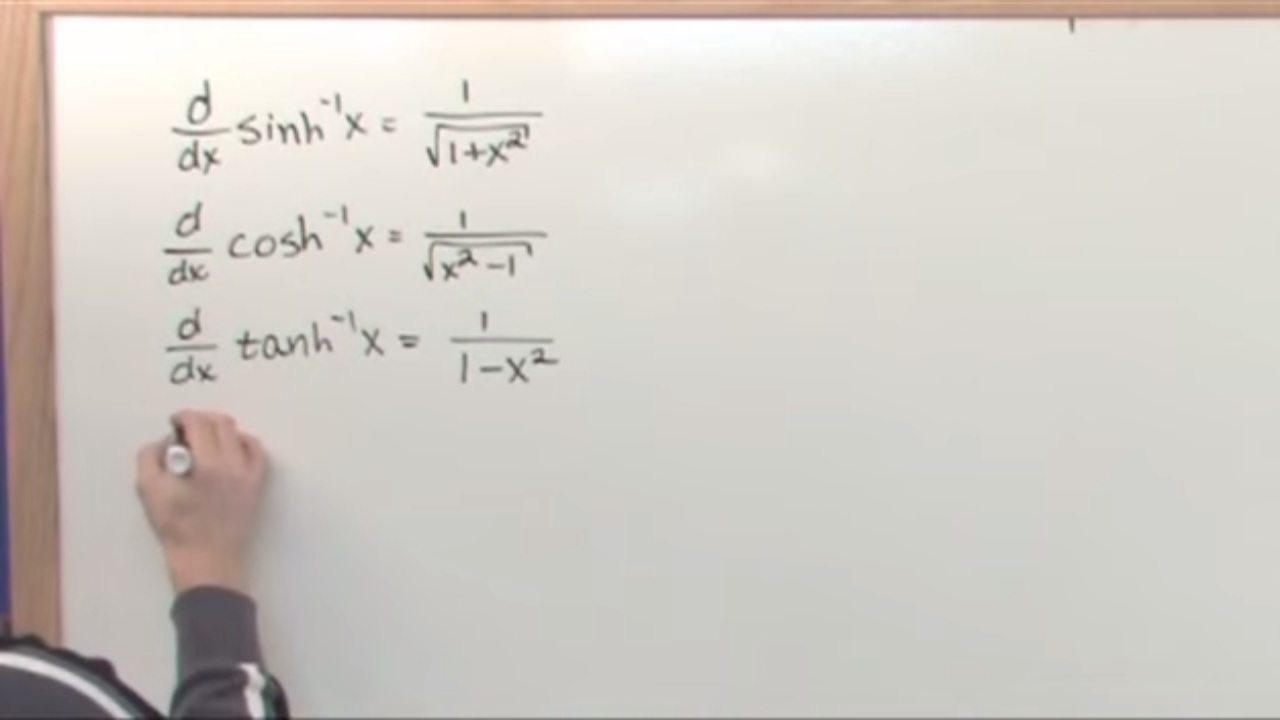
Lesson 4 - Inverse Hyperbolic Functions (Calculus 2 Tutor)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Hyperbolic FunctionsMathematicsGeometryAnalytical MathEven and Odd FunctionsExponential FunctionsTrigonometryHyperbolasMath EducationMath Videos