Trigonometry fundamentals | Ep. 2 Lockdown live math
TLDRIn this engaging lecture, the connection between trigonometry and complex numbers is explored, emphasizing the relationship between sine, cosine, and trigonometric functions. The discussion begins with basic trigonometric functions and their graphical representations, using a graphing calculator to demonstrate non-trivial facts about trigonometry. The lecture then delves into the unit circle and the geometric interpretation of sine and cosine, highlighting the Pythagorean theorem's role in trigonometric identities. The video also introduces the concept of radians over degrees for a more natural understanding of trigonometric functions. The exploration of the tangent function and its geometric interpretation precedes a live quiz on the graph of the tangent function. The lecture concludes with a sneaky proof of the Pythagorean theorem using trigonometric functions and a look at the cosine squared function's geometric representation on a unit circle.
Takeaways
- 📚 The core goal is to establish a connection between trigonometry and complex numbers, enhancing understanding of both subjects.
- 🤔 Trigonometric functions can be explored without prior knowledge by using graphing calculators to discover non-trivial facts about their behavior.
- 📈 The cosine function's graphing can reveal its relevance to wave dynamics, with frequency adjustments demonstrated by tweaking the input.
- 🔄 The squaring of the cosine function results in a graph that resembles a scaled version of the original cosine wave, leading to the identity cos(x)^2 = 1 + cos(2x)/2.
- 🎶 The live element of the lecture includes interactive quizzes and real-time problem-solving, engaging the audience in mathematical concepts.
- 🌀 The unit circle is central to understanding sine and cosine, with the input representing a distance around the circle and the outputs corresponding to y and x coordinates.
- 📐 Trigonometry is often taught using right triangles, but the circle-based approach provides a different and often more intuitive understanding.
- 🏗️ The leaning tower of Pisa example illustrates the application of trigonometric functions in real-world scenarios, specifically using the shadow to determine the length based on the tower's height and angle.
- 🔢 The concept of radians is introduced as a more natural unit for angles than degrees, especially when working with calculus and trigonometric functions.
- 🔍 The equilateral triangle serves as a basis for calculating sine and cosine values due to its symmetry, leading to concrete calculations for angles like 30 and 60 degrees.
- 📊 The Pythagorean theorem is inherently linked to trigonometry, with the theorem's relationship to the leg lengths of a right triangle forming the basis for key trigonometric identities.
Q & A
What is the main goal of the lecture discussed in the transcript?
-The main goal of the lecture is to draw a connection between trigonometry and complex numbers, emphasizing that understanding this connection strengthens the understanding of both subjects.
How does the lecturer introduce the concept of trigonometry to someone unfamiliar with it?
-The lecturer suggests using a graphing calculator to explore the cosine function without prior knowledge of trigonometry, allowing the person to discover non-trivial facts about the function through observation and manipulation of the graph.
What is the significance of the cosine function in the context of wave dynamics?
-The cosine function is significant in wave dynamics because it exhibits a cycling phenomenon, making it relevant for studying anything associated with cycling, such as sinusoidal waves.
How does the lecturer demonstrate the relationship between the cosine function and its square?
-The lecturer demonstrates the relationship by squaring the cosine function and asking the audience to predict the resulting graph, then revealing that the squared cosine function looks like a sinusoidal wave, but all positive and oscillating more quickly.
What is the identity that the lecturer derives from the graph of cosine squared?
-The lecturer derives the identity cos(x)^2 = (1 + cos(2x)) / 2, which shows that cosine squared is related to a scaled version of itself.
How does the lecturer connect trigonometry to circles?
-The lecturer connects trigonometry to circles by explaining that sine and cosine can be thought of in terms of distances around a unit circle, with the input being the angle and the output being the y or x coordinate of a point on the circle.
What is the significance of the unit circle in trigonometry?
-The unit circle is significant in trigonometry because it provides a visual and geometric interpretation of sine and cosine values, allowing for a deeper understanding of their behavior and relationships.
How does the lecturer explain the concept of radians in relation to trigonometry?
-The lecturer explains radians as a more natural unit for trigonometry than degrees, relating angles to the distance around the unit circle. Radians allow for easier computation and understanding in calculus and other advanced mathematical contexts.
What is the Pythagorean identity derived from the definitions of sine and cosine?
-The Pythagorean identity derived from the definitions of sine and cosine is cos(x)^2 + sin(x)^2 = 1, which reflects the relationship between the leg lengths of a right triangle and the hypotenuse when scaled down.
How does the lecturer use the concept of equilateral triangles to find trigonometric values?
-The lecturer uses the symmetry of equilateral triangles to find trigonometric values, leveraging the known side lengths and angles to calculate sine and cosine for specific angles like 30 and 60 degrees (or π/6 and π/3 radians).
What is the tangent function in the context of the unit circle?
-In the context of the unit circle, the tangent function represents the ratio of the opposite side to the adjacent side of a right triangle formed by a radius and a line tangent to the circle at a point on the circumference.
Outlines
📚 Introduction to Trigonometry and Complex Numbers
The lecturer aims to establish a connection between trigonometry and complex numbers, emphasizing the importance of understanding this relationship for a deeper comprehension of both subjects. The lecture begins with a basic review of trigonometric functions, suggesting an exploratory approach to learning by using a graphing calculator to discover non-trivial facts about trigonometry. The lecturer uses the cosine function as an example to illustrate how its graph can be manipulated and interpreted, leading to insights about its relevance to wave dynamics.
🎨 Graphing Trigonometric Functions
The lecturer continues the exploration of trigonometric functions through graphing, focusing on the cosine function. The discussion involves the observation of how squaring the cosine function affects its graph, particularly within the range of 0 to 2 pi. The lecturer engages the audience with a live quiz, highlighting the consensus towards a graph that resembles a sinusoidal wave. The lecture then delves into the mathematical identity of the squared cosine function, demonstrating its relationship with the original cosine graph.
🔍 Discovering Trigonometric Identities
The lecturer encourages a playful approach to learning by using a graphing calculator to discover non-trivial facts about trigonometric functions. The discussion moves to the second question of the lecture, which involves identifying an identity based on the graph of the squared cosine function. The lecturer emphasizes the importance of active participation and problem-solving, using a musical interlude to foster a conducive atmosphere for thinking. The lecture reveals the correct identity and explains the step-by-step process of its derivation.
🌀 Trigonometry and Exponential Functions
The lecturer explores the relationship between trigonometric functions and exponentials, using the squared cosine function as a starting point. The discussion involves the observation of the cosine graph's resemblance to an exponential function when squared. The lecturer introduces a question about the relationship between functions that satisfy a specific condition, leading to the identification of the exponential function as the correct answer. The lecture aims to build an intuition about the connection between trigonometry and exponents, setting the stage for further exploration in the next lecture.
📐 Trigonometry and the Unit Circle
The lecturer shifts the focus to the unit circle to explain sine and cosine functions, emphasizing the transition from thinking about trigonometry in terms of triangles to circles. The lecture introduces the concept of walking around a unit circle and how the sine and cosine of an angle can be visualized as the y and x coordinates, respectively, of a point on the circle. The讲师 uses animations to illustrate the concepts and engages the audience with a quiz to guess the values of sine and cosine for specific angles. The lecture highlights the importance of understanding the unit circle and the concept of radians in trigonometry.
🏗️ The Leaning Tower of Pisa Problem
The lecturer presents a practical problem involving the Leaning Tower of Pisa to demonstrate the application of trigonometric functions in real-world scenarios. The problem involves calculating the length of the tower's shadow when the sun is directly overhead and the tower makes an 80-degree angle with the ground. The lecturer encourages the audience to draw the problem and engage with the question. The correct answer is revealed to be 100 times the cosine of 80 degrees, highlighting the use of trigonometric functions in solving practical problems.
🤔 Converting Degrees to Radians
The lecturer discusses the importance of converting degrees to radians when working with trigonometric functions, as radians are more naturally integrated with calculus and other advanced mathematical concepts. The lecture introduces the concept of radians and their relationship with degrees, using the unit circle to illustrate the conversion. The lecturer also touches on the historical and mathematical preference for radians over degrees, emphasizing the universality of radians in mathematical conventions.
🔢 Computing Trigonometric Values
The lecturer delves into the challenge of computing trigonometric values without the aid of advanced techniques or calculators. The discussion involves leveraging geometric settings, such as equilateral triangles, to derive concrete values for sine and cosine. The lecturer uses the symmetry of an equilateral triangle to calculate the sine and cosine of specific angles, highlighting the Pythagorean theorem's role in these computations. The lecture emphasizes the importance of understanding the underlying geometry of trigonometric functions.
📐 Trigonometric Identities and the Pythagorean Theorem
The lecturer discusses the interplay between trigonometric identities and the Pythagorean theorem, using the concept of squaring cosine and its relationship with the original cosine function. The lecture corrects a common misconception about the notation used in trigonometry, explaining the convention of writing 'cosine squared' instead of 'cosine of x squared'. The lecturer also hints at the potential to use trigonometric functions to prove the Pythagorean theorem in a visually satisfying way, setting the stage for future discussions.
🌀 Negative Angles and Trigonometric Functions
The lecturer explores the concept of negative angles in trigonometry, emphasizing that cosine and sine are fundamentally about circles rather than triangles. The discussion involves visualizing the cosine of a negative angle using the unit circle and leveraging the understanding of triangles to interpret the result. The lecturer engages the audience with a live quiz to guess the cosine of a negative angle, leading to a deeper understanding of the periodic nature of trigonometric functions.
🔄 Trigonometric Identities and Graphs
The lecturer revisits the trigonometric identity involving the squared cosine function and extends the discussion to include the cosine of a double angle. The lecture uses the Desmos graph to illustrate the identity and its implications for understanding the behavior of trigonometric functions. The lecturer poses a question about the cosine of a specific angle, leading to a complex calculation that demonstrates the power of trigonometric identities in computation.
📊 Understanding the Tangent Function
The lecturer addresses the tangent function, explaining its geometric interpretation and its location on the unit circle. The discussion involves the construction of a right triangle with a hypotenuse of one and the use of the tangent line to define the tangent function. The lecturer uses the concept of the tangent function to pose a question about its graph, leading to a live quiz and a discussion on the behavior of the tangent function as it approaches specific angles.
🌟 The Unit Circle and Trigonometric Functions
The lecturer concludes the lecture by highlighting the geometric representation of trigonometric functions on the unit circle, specifically addressing the locations of the tangent and squared cosine functions. The lecture provides a sneaky proof of the Pythagorean theorem using the unit circle and discusses the geometric interpretation of the squared cosine and sine functions. The lecturer emphasizes the importance of visualizing trigonometric functions and their relationships for a deeper understanding.
Mindmap
Keywords
💡Trigonometry
💡Complex Numbers
💡Sine Function
💡Cosine Function
💡Graphing Calculator
💡Sinusoidal Wave
💡Pi (π)
💡Desmos
💡Unit Circle
💡SOHCAHTOA
Highlights
The lecture aims to establish a connection between trigonometry and complex numbers, emphasizing that understanding this relationship strengthens the comprehension of both subjects.
Trigonometric functions can be explored even without prior knowledge by using graphing calculators, such as Desmos, to discover non-trivial facts about trigonometry.
The cosine function's graph can be manipulated to understand its behavior, such as frequency and amplitude adjustments.
The graph of cosine squared (cos^2(x)) resembles a sinusoidal wave, but with a different frequency and all positive values.
An identity derived from graphing cosine squared is cos^2(x) = 1 + cos(2x), which reveals a non-obvious relationship between the function and its input.
The properties of trigonometric functions can be intuitively understood by considering the unit circle and the distance around it.
Trigonometry is often taught using right triangles, but the perspective shifts to circles as one gains a deeper understanding.
The sine and cosine functions can be visualized as coordinates on a unit circle, with sine representing the y-coordinate and cosine the x-coordinate.
The Pythagorean theorem is implicitly used in trigonometry, as the definitions of sine and cosine are based on the ratios of sides in a right triangle.
The identity cos^2(x) + sin^2(x) = 1 is a fundamental relationship in trigonometry, derived from the Pythagorean theorem and the definitions of the trigonometric functions.
The concept of radians is introduced as a more natural unit for angles in trigonometry, as opposed to degrees, which are more intuitive for humans.
The lecture demonstrates that trigonometric functions are not only about triangles but are fundamentally about circles and the concept of angles measured in radians.
The tangent function is geometrically represented by a line tangent to the unit circle, with the tangent value corresponding to the length of the tangent line.
The graph of the tangent function starts at zero and approaches infinity as the angle approaches 90 degrees (pi/2 radians), demonstrating its undefined nature at this point.
The concept of cosine squared is visually represented on the unit circle by the length of a perpendicular line segment from the circle's edge to the x-axis, providing geometric insight into the half-angle identity.
The lecture concludes with a teaser about the upcoming exploration of how complex numbers relate to trigonometric functions, hinting at a deeper mathematical connection.
Transcripts
Browse More Related Video

Trigonometry: Unit Circle
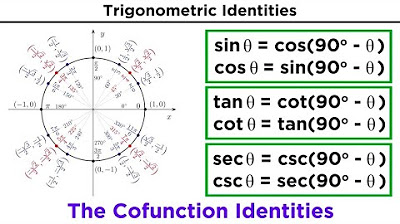
Basic Trigonometric Identities: Pythagorean Identities and Cofunction Identities

Calculus 1 Lecture 0.3: Review of Trigonometry and Graphing Trigonometric Functions

Trigonometric Functions of Any Angle - Unit Circle, Radians, Degrees, Coterminal & Reference Angles
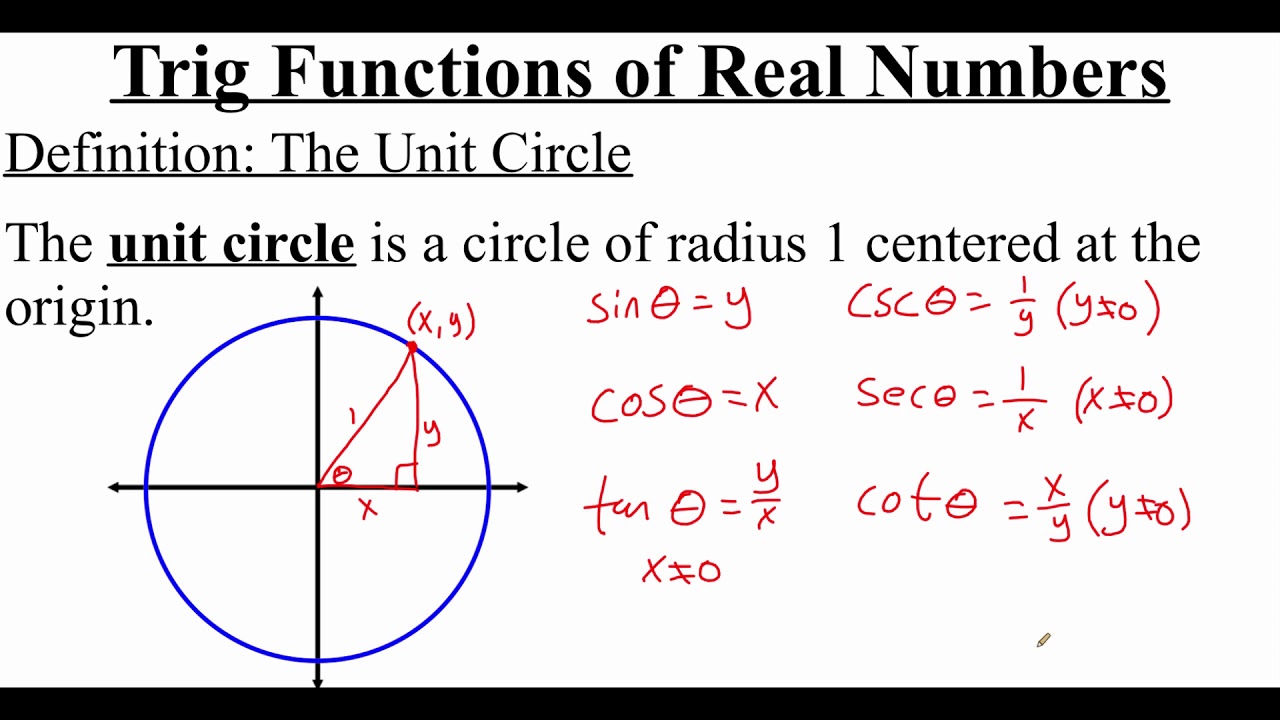
4.3.4 Trigonometric Functions of Real Numbers

Pythagorean Identities for Trigonometric Functions (Precalculus - Trigonometry 10)
5.0 / 5 (0 votes)
Thanks for rating: