BusCalc 13.1 Antiderivatives

Drew Macha

29 Mar 202260:06

EducationalLearning

32 Likes 10 Comments

TLDRThe transcript is a detailed explanation of anti-derivatives, which are mathematical functions that reverse the process of differentiation. The speaker introduces the concept by explaining that the antiderivative of a function is the inverse operation to taking the derivative. Using the integral sign, the antiderivative of a function can be found by applying various rules, such as the power rule, which involves adjusting the exponent of the function and multiplying by the new exponent. The importance of including a constant 'c' when finding an antiderivative is emphasized, as it accounts for the constant of integration. The transcript also covers the anti-derivatives of exponential functions, reciprocal functions, and the properties of additivity and homogeneity. Practical applications, such as modeling population growth and distance over time, are discussed to illustrate the real-world relevance of anti-derivatives. The speaker provides several examples to demonstrate how to calculate anti-derivatives for different types of functions and emphasizes the need to memorize key rules for solving problems involving anti-derivatives.

Takeaways

📚 The concept of anti-derivatives is introduced as the inverse operation to derivatives, where the antiderivative of a function is found by reversing the process of differentiation.
📝 The notation for anti-derivatives is represented by a tall squiggly symbol (\[ \int \]), which is read as 'the antiderivative of' when spoken aloud.
✍️ When finding the antiderivative, a constant (C) is always added to the end of the expression, as it represents an arbitrary constant that remains after differentiation.
🔢 The antiderivative power rule is the reverse of the power rule for derivatives, where you add one to the exponent and then divide by the new exponent.
🔁 The antiderivative of a sum of functions is the sum of the antiderivatives of each function, reflecting the additivity property.
🔄 The antiderivative of a constant times a function is the constant times the antiderivative of the function, showcasing the homogeneity property.
📈 The antiderivative of exponential functions (e^(kx)) is found by keeping the exponential term and multiplying by the reciprocal of the exponent.
📊 The antiderivative of the reciprocal function (1/x) is the natural logarithm of the absolute value of x, which is a special case due to the non-integer exponent.
🧮 The process of finding antiderivatives can be checked by differentiating the result and ensuring it matches the original function.
🏃‍♂️ In real-life scenarios, such as calculating the distance traveled by a runner, anti-derivatives are used to find quantities of interest from rates of change.
📊 For word problems involving growth rates, the antiderivative can be used to find cumulative quantities like population, given an initial value and a rate of change over time.

Q & A

What is the relationship between a derivative and an antiderivative?
-The antiderivative has an inverse relationship to the derivative. If a function f is the derivative of another function F, then F is considered the antiderivative of f.
What is the notation used for antiderivatives?
-The notation for antiderivatives is a tall little squiggle, sometimes called the integral sign or the antiderivative sign, represented as ∫.
How do you read the antiderivative notation out loud?
-You read the antiderivative notation out loud as 'the antiderivative of' followed by the expression inside the integral sign and ending with 'with respect to x' (or the relevant variable).
Why is a constant (usually denoted as 'c') always added to an antiderivative?
-A constant is added to an antiderivative because when you take the derivative of a function, constants disappear. Therefore, the antiderivative, which is the reverse process, must account for an arbitrary constant that could have been present in the original function.
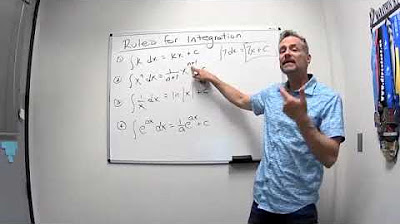
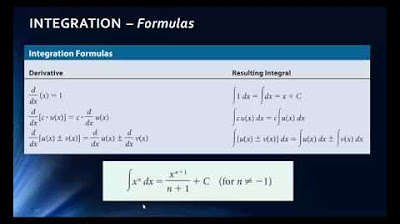
What is the antiderivative of x^n according to the power rule?
-The antiderivative of x^n, where n is a constant, is (1/(n+1)) * x^(n+1) + c, where c is any constant.
How do you check if you have found the correct antiderivative?
-You can check if you have found the correct antiderivative by taking the derivative of your antiderivative and verifying that it equals the original function you started with.
What is the antiderivative of an exponential function e^(kx)?
-The antiderivative of e^(kx) with respect to x is (1/k) * e^(kx) + c, where k is any constant.
What is the antiderivative of the reciprocal function 1/x?
-The antiderivative of the reciprocal function 1/x is the natural logarithm of the absolute value of x, or ln|x| + c.
What are the properties of antiderivatives that are similar to the properties of derivatives?
-Antiderivatives share the properties of additivity and homogeneity with derivatives. This means that the antiderivative of a sum of functions is the sum of the antiderivatives of each function, and a constant factor can be taken out of the antiderivative.
How do you find the antiderivative of a function that is a sum of several terms?
-You can find the antiderivative of a sum of several terms by taking the antiderivative of each term separately and then adding them together, along with a constant c.
What is the antiderivative of a constant with respect to x?
-The antiderivative of a constant with respect to x is the constant times x plus c, as the antiderivative of a constant is the constant multiplied by the variable of integration.

Outlines

00:00

😀 Introduction to Anti-derivatives

The video begins with an introduction to anti-derivatives, explaining that they are the inverse of derivatives and have a relationship with integrals. The notation for anti-derivatives is discussed, along with the concept of the integrand and the constant 'c' that is added to the antiderivative. The importance of the constant 'c' is emphasized, as it can be any value and still yield the original derivative when applied.

05:01

📚 Anti-derivative Power Rule and Memorization

The video then delves into the anti-derivative power rule, which is the reverse of the derivative power rule. The presenter corrects a common mistake regarding the rule, emphasizing the importance of the 'n plus one' term in the denominator. The video also covers the anti-derivative of exponential functions and provides a method to check work by differentiating the antiderivative to confirm it matches the original function.

10:03

🔢 Anti-derivative Rules for Exponential and Reciprocal Functions

The presenter continues with examples of anti-derivatives for exponential functions and the reciprocal function. It is shown that the anti-derivative of a reciprocal function is the natural logarithm. The video also explains why the power rule for anti-derivatives does not apply when the exponent is negative one. The properties of additivity and homogeneity of anti-derivatives are introduced, which are similar to those of derivatives.

15:05

🧮 Applying Anti-derivative Rules to Specific Examples

The video script provides a series of examples where the anti-derivative rules are applied to various functions, including polynomials and exponentials. The process of applying the power rule, additivity rule, and homogeneity rule is demonstrated. The presenter also uses these examples to show how to find the antiderivative of a function and emphasizes the importance of including the constant 'c'.

20:14

🏃‍♂️ Anti-derivatives in Real-life Scenarios: Runner's Distance

The application of anti-derivatives is extended to real-life scenarios with a word problem involving a runner's velocity and distance. The concept that the derivative represents a rate of change, and the antiderivative undoes this to find the original property, is highlighted. The video concludes with solving for the time it takes for the runner to finish a race by using the derived distance function.

25:15

📈 Projected Population Growth Using Anti-derivatives

The video concludes with another real-life application, projecting the population growth of Midland, Texas. The growth rate function is given, and the antiderivative is taken to find the population function. The presenter shows how to find the constant 'c' in the antiderivative using a given data point and then uses the population function to estimate the population in a future year.

30:16

📚 Summary and Preview of Upcoming Material

In the final part of the video script, the presenter summarizes the key points covered in the lecture, emphasizing the importance of memorizing the anti-derivative rules. The presenter also teases the next topic, which will be integration by substitution, comparing it to the derivative chain rule but noting it is more complex.

Mindmap

Keywords

💡Anti-derivatives

Anti-derivatives, also known as integrals, are mathematical constructs that serve as the inverse operation to differentiation. They are used to find the original function when given its derivative. In the context of the video, anti-derivatives are central to the discussion as they are used to find functions whose derivatives are provided, exemplified by the function f(x) being the antiderivative of 2x, which means 2x is the derivative of x^2.

💡Derivative

The derivative of a function measures the rate at which the function changes with respect to a variable. It is a fundamental concept in calculus and is the direct counterpart to the anti-derivative. In the video, the concept of derivatives is mentioned in contrast to anti-derivatives to illustrate the inverse relationship between the two, such as stating that the derivative of x^2 is 2x.

💡Integral Sign

The integral sign, represented by the symbol ∫, is used to denote an integral, specifically an antiderivative, in mathematical notation. The video explains that this symbol is sometimes referred to as the anti-derivative sign and is used to indicate the process of integration or finding an antiderivative of the function that lies between the integral sign and the 'dx' at the end.

💡Indefinite Integral

An indefinite integral represents a family of functions that differ by a constant, which is typically denoted by '+ C'. In the video, it is emphasized that when finding an antiderivative, one must always append a '+ C' to account for the constant of integration, reflecting the fact that any differentiable function can have an infinite number of anti-derivatives differing by a constant.

💡Power Rule

The power rule is a fundamental rule in calculus that allows for the differentiation of polynomial terms. In the context of anti-derivatives, the video discusses the reverse power rule, which is used to find the antiderivative of a term like x^n by dividing by 'n + 1' and adding 1 to the exponent, as shown when finding the antiderivative of x^2 which results in (1/3)x^3 + C.

💡Exponential Function

An exponential function is a function of the form e^(kx), where e is the base of the natural logarithm and k is a constant. The video covers the antiderivative of exponential functions, stating that the antiderivative of e^(kx) is (1/k)e^(kx) + C, which is a direct application of one of the rules for finding anti-derivatives.

💡Reciprocal Function

The reciprocal function refers to the function 1/x. The video explains that the antiderivative of the reciprocal function, or 1/x, is the natural logarithm of the absolute value of x, denoted as ln|x| + C. This is a special case where the antiderivative results in a logarithmic function rather than another power function.

💡Additivity

Additivity is a property of integrals that allows for the separation of the integral of a sum into the sum of integrals. In the video, it is mentioned that the antiderivative of a sum of functions, f(x) + g(x), is equal to the antiderivative of f(x) plus the antiderivative of g(x), which simplifies the process of finding anti-derivatives of more complex functions.

💡Homogeneity

Homogeneity in the context of anti-derivatives refers to the property that allows a constant factor within an integral to be moved outside of the integral sign. The video illustrates this by showing that the antiderivative of a constant times a function, such as 5/x, can be found by taking the antiderivative of the function and then multiplying by the constant, which simplifies calculations.

💡Real-life Application

The video concludes with an example of applying anti-derivatives to a real-life scenario, specifically modeling population growth. It demonstrates how an initial condition, such as a known population at a specific time, can be used to determine the constant of integration, C, in a population growth model, thereby finding a function that describes the population over time.

Highlights

Chapter 13 focuses on finding anti-derivatives, which have an inverse relationship to derivatives.

If a function f is the derivative of F, then F is the antiderivative of f.

The notation for anti-derivatives is a tall squiggly symbol, often called the integral sign.

The antiderivative of 2x with respect to x is x squared plus c, illustrating the process of anti-differentiation.

The constant c in anti-derivatives can be any number, as it disappears when taking the derivative.

The antiderivative of x to the power n uses the reverse power rule, adding 1 to the exponent and dividing by the new exponent.

The antiderivative of an exponential function e to the kx is e to the kx times (1/k) plus c.

The antiderivative of the reciprocal function 1/x is the natural log of the absolute value of x plus c.

Anti-derivatives possess properties of additivity and homogeneity, similar to derivatives.

The anti-derivative power rule is crucial and frequently used, requiring memorization.

Examples are provided to illustrate the process of finding anti-derivatives for various functions.

The antiderivative of a constant times a function is the constant times the antiderivative of the function.

The concept of integrating by substitution is introduced as a method to be learned in the next class.

The importance of including the constant c in anti-derivatives is emphasized for accuracy in further calculations.

Real-life scenarios are used to demonstrate the application of anti-derivatives, such as calculating the distance a runner has traveled.

The antiderivative is used to find the projected population of Midland, Texas, given a growth rate model.

The value of c in a real-world problem can be determined by using known conditions, such as an initial population.

The antiderivative method is applied to find the function that gives the projected population at a future year.

Transcripts

Browse More Related Video

Antidifferentiation

4.1 - Antidifferentiation

Rules for Integration

Antiderivatives

AP Calculus AB: Lesson 6.1 Finding Antiderivatives

Math 1325 Lecture 12 1

Related Tags

Anti-derivatives Calculus Derivatives Integrals Math Education Power Rule Exponential Functions Reciprocal Functions Additivity Homogeneity Real-life Applications