Integration using substitution method
TLDRThe video script is an in-depth tutorial on the technique of integration by substitution, a method used to simplify complex integrals. The speaker begins by explaining the concept of substitution, where a function of 'x' is converted to a function of a new variable 'u' to make integration easier. Several examples are provided to illustrate the process, starting with an integral involving a polynomial expression. The speaker then demonstrates how to identify when to use substitution by comparing the powers of 'x' inside and outside the integral. The tutorial also covers integration involving exponential functions and trigonometric functions, emphasizing the importance of differentiating the chosen 'u' and manipulating the integral accordingly. The script highlights the use of logarithmic functions in integration and the application of integration by parts towards the end. The speaker encourages students to attend classes for a comprehensive understanding and to access exam revision materials.
Takeaways
- 📚 **Substitution Method**: When the power of x inside a bracket differs by one from the power of x outside, substitution can simplify the integration process.
- 🔑 **Identifying Substitution**: Check the powers of x; if the power inside a bracket is exactly one higher than outside, use substitution by letting u be the expression inside the bracket.
- 🧮 **Derivative for Substitution**: After setting u, differentiate it with respect to x to find du, which will help in rewriting the integral in terms of u.
- 🔗 **Rewriting the Integral**: Replace the original expression with u and du, and solve for dx in terms of du to rewrite the integral solely in terms of u.
- 📈 **Integration of Powers**: Integrating a power of u is straightforward; increase the power by one and divide by the new power.
- 🔄 **Reverting to x**: After integrating with respect to u, substitute back the original x expression to return to the integral in terms of x.
- 🚫 **Avoiding Division by Zero**: Ensure that the substitution and rewriting process does not lead to division by zero, which is undefined.
- 📉 **Logarithmic Integration**: When dealing with fractions where the denominator is a function of x and the numerator is a derivative of that function, use the natural logarithm (ln) for integration.
- 🌐 **Chain Rule for Differentiation**: Use the chain rule to differentiate composite functions, such as cosine or sine of an expression involving x.
- ✅ **Parts Method**: For certain integrals that do not easily yield to substitution, consider using integration by parts, especially when the integral is a product of two functions.
- ⏯️ **Integration of Products**: When integrating a product of functions, consider what the original function might have been before differentiation, which can guide the choice of u and dv in integration by parts.
Q & A
What is the main idea behind using substitution in integration?
-The main idea behind using substitution in integration is to simplify the integral by changing the variable from X to another variable, U, which makes the integral easier to solve using standard methods.
How can you determine if you should use the substitution method for a given integral?
-You can determine if you should use the substitution method by checking if the power of X inside the brackets is exactly one higher than the power of X outside the brackets.
What is the process of changing the variable in a substitution method?
-The process involves setting U equal to an expression involving X, differentiating U with respect to X to find du/dx, and then substituting these into the integral to express it solely in terms of U and du.
What is the advantage of using the substitution method in the given example of ∫(x * (7 + 6x^2)^9) dx?
-The advantage is that instead of expanding (7 + 6x^2)^9, which would be complex, you can use substitution to transform the integral into a simpler form involving U, making it easier to integrate.
How do you integrate a function like ∫(4x^3 - 12x) / (x^4 - 6x^2) dx using substitution?
-You would let U be the denominator (x^4 - 6x^2), differentiate U to find du/dx, and then rewrite the integral in terms of U and du, which simplifies the integration process.
What is the purpose of introducing the natural logarithm function, ln(x), in certain integration problems?
-The natural logarithm function is introduced when the derivative of the function inside the integral is in the form of the function itself over the function, which simplifies the integration process.
What is the correct approach when you encounter an integral with exponential functions like ∫(9e^x / (e^x + 4)) dx?
-You would set U to be e^x + 4, differentiate U to get du/dx = e^x, and then rewrite the integral in terms of U and du, which allows you to integrate more easily.
Why do we sometimes not carry the whole power when substituting in an integral?
-When substituting, you only need to consider the expression inside the brackets as U and not carry the power, because after differentiation, you'll substitute back into the integral with the original power.
How do you approach an integral that includes a product of functions like x^2 * cos(x^3 + 2)?
-You would use a combination of substitution and integration by parts, setting U and V for different parts of the integral, differentiating to find du and dv, and then integrating the simplified product.
What is the role of the chain rule in differentiating functions like cos(x^3 + 2)?
-The chain rule is used to differentiate composite functions like cos(x^3 + 2) by differentiating the outer function (cosine) and then multiplying by the derivative of the inner function (x^3 + 2).
What is the importance of considering the sign when integrating functions involving sine or cosine?
-The sign is important because the integral of sine is negative cosine, and vice versa. It determines the correct function to be used when substituting back after integration.
Why is it recommended to attend classes and not just rely on video content for learning integration techniques?
-Attending classes provides a more comprehensive understanding, opportunities for clarification, and the chance to engage with the material actively, which can be more beneficial than just watching video content.
Outlines
📚 Integration by Substitution
This paragraph introduces the concept of integration by substitution, a technique used to simplify complex integrals. It explains that instead of working with the variable x, one can substitute it with another variable, say u, to make the integral easier to solve. The paragraph uses an example from a literature sheet to demonstrate how to apply this method, emphasizing the importance of checking the power of x inside and outside brackets to determine if substitution is applicable.
🔍 Identifying Substitution Candidates
The second paragraph focuses on how to identify when to use the substitution method. It discusses checking the powers of x in both the numerator and the denominator of a fraction. If the power in the denominator is exactly one higher than in the numerator, substitution is a viable approach. The paragraph also provides another example, illustrating the process of differentiating the chosen u and integrating the resulting expression.
🔗 Relating du and dx in Substitution
This paragraph delves into the relationship between du and dx when changing variables from x to u. It explains how to solve for dx in terms of du, which is crucial for substituting back into the integral after performing the substitution. The paragraph emphasizes the importance of ensuring that the integral is expressed in terms of u before carrying out the integration.
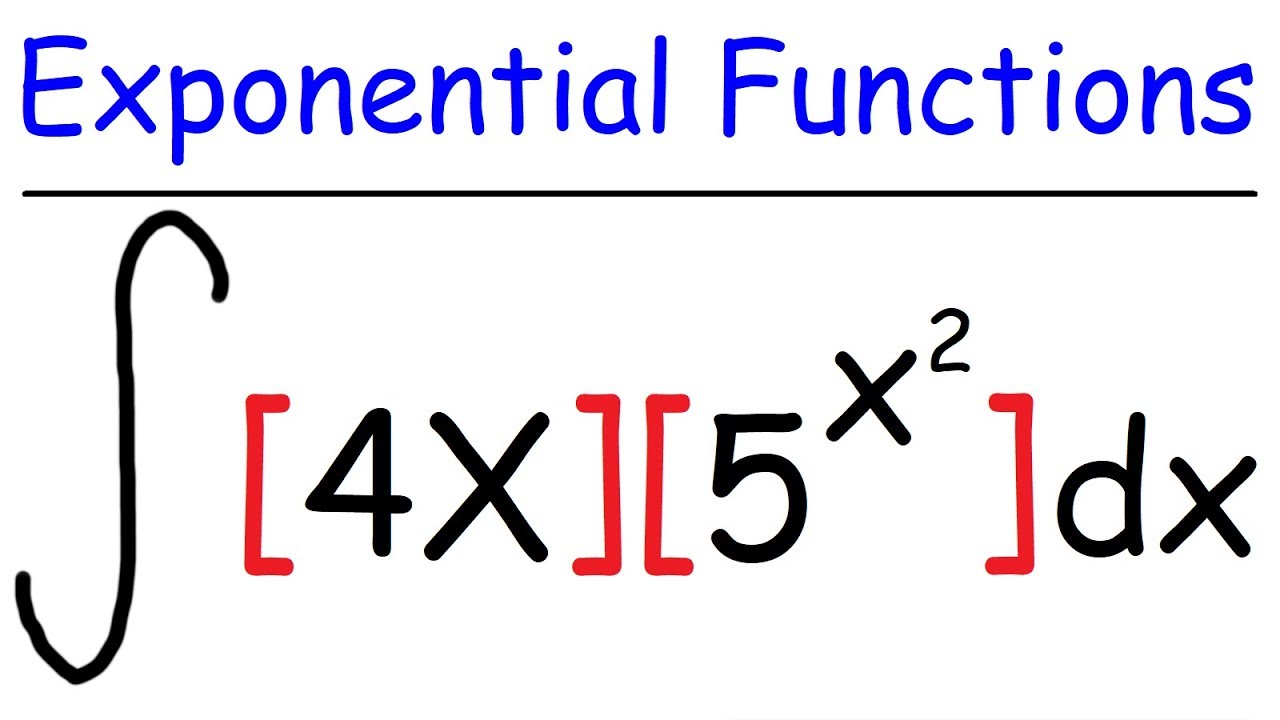
🧮 Integrating Exponential Functions
The fourth paragraph tackles the integration of exponential functions. It demonstrates how to separate an integral into parts that can be integrated individually and how to use substitution for exponential terms. The paragraph shows the process of letting u be equal to a specific exponential expression, differentiating u to find du/dx, and then integrating the simplified form of the integral.
📐 Handling Trigonometric and Exponential Products
The fifth paragraph discusses the integration of products involving trigonometric and exponential functions. It outlines the process of using substitution for such integrals, emphasizing the need to differentiate the trigonometric part to find du/dx. The paragraph also covers the integration of the resulting expression and the importance of ensuring that the inside of the logarithm remains positive.
🧬 Integration by Parts
The sixth paragraph briefly mentions the method of integration by parts, which is another technique for integrating products of functions. Although not elaborated upon in detail, it suggests that this method is applicable when the integral does not lend itself to simple substitution or other elementary methods.
📉 Differentiating Trigonometric Functions
The seventh paragraph provides a detailed explanation of how to differentiate trigonometric functions, specifically focusing on the cosine of a power function. It covers the use of the chain rule to find the derivative and how to apply it to the integration process. The paragraph also discusses the importance of considering the form of the integral and what might have been differentiated to achieve the given function.
🔢 Integrating Products of Polynomials and Trigonometric Functions
The final paragraph presents a method for integrating the product of a polynomial and a trigonometric function. It demonstrates the use of substitution to simplify the integral and the application of the chain rule for differentiation. The paragraph concludes with the integration of the simplified expression and the substitution back to the original variable to find the final solution.
Mindmap
Keywords
💡Integration by Substitution
💡Power Rule
💡Derivative
💡Natural Logarithm
💡Chain Rule
💡Trigonometric Functions
💡Exponential Functions
💡Integration by Parts
💡Binomial Expansion
💡Constant of Integration
💡Differential Equation
Highlights
The concept of integration by substitution is introduced, which involves converting a function to deal with a new variable u instead of x to simplify integration.
A substitution rule is explained where if the power of x inside the brackets is one higher than the power of x outside, substitution is applicable.
The process of differentiating u with respect to x to find du/dx is demonstrated using an example.
An example integral is solved using substitution, showcasing the steps to change the variable and integrate.
The importance of ensuring the substitution variable u is positive is emphasized.
The transcript illustrates how to handle integrals with exponential functions by using substitution with u as the exponential part.
The method of integrating a product of functions is discussed, suggesting to consider what might have been differentiated to lead to the integrand.
The use of the chain rule for differentiating functions like cos(x^3 + 2) is explained.
Integration by parts is hinted at as an alternative method for certain types of integrals.
The transcript provides a detailed walkthrough of integrating the product of x^2 and cos(x^3 + 2) using substitution with u and v.
The process of integrating a function and then substituting back the original variable is demonstrated.
The transcript explains the differentiation of exponential functions and the importance of the power of x in the process.
An example is given where the integral of a function with a higher power in the numerator compared to the denominator is solved using substitution.
The transcript emphasizes the need to attend classes for a comprehensive understanding and access to exam revisions.
The concept of integrating a function where the derivative is in the numerator is discussed, introducing the natural logarithm as a solution method.
The transcript provides a method to solve integrals with a difference in powers that is not one, suggesting alternative approaches.
The importance of recognizing when not to use substitution and when to use other integration methods is highlighted.
The transcript concludes with a reminder about the importance of the positive value of u in the context of natural logarithms.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: