Calculus AB/BC – 8.7 Volumes with Cross Sections: Squares and Rectangles
TLDRIn this engaging calculus lesson, Mr. Bean introduces the concept of calculating the volume of solids, focusing on solids with square and rectangular cross-sections. He emphasizes the importance of understanding the base of the solid and the cross-sections to determine the shape of the solid. Using the example of a graph with a square root of x and an x squared parabola, he illustrates how to visualize the solid in three dimensions by adding multiple cross-sections. The lesson covers setting up integrals for both square and rectangular cross-sections, highlighting the need to consider the direction of the cross-section (perpendicular to the x-axis or y-axis) and the distance between the functions representing the top and bottom of the cross-section. Mr. Bean also provides a step-by-step guide on how to calculate the volume by integrating the area of the cross-sections from the given boundaries. The lesson concludes with a reminder of the significance of mastering this topic for the AP exam, as it is a common problem area.
Takeaways
- 📐 **Understanding Volume of Solids**: The lesson focuses on calculating the volume of three-dimensional solids using cross-sectional areas.
- 🔍 **Bounded Regions as Bases**: The area formed by bounded regions in two dimensions becomes the base of the solid in three dimensions.
- 🔺 **Cross-Sections**: Cross-sections are slices of the solid perpendicular to a given axis (x or y), and their shapes (squares or rectangles in this lesson) are key to calculating volume.
- ⊥ **Perpendicularity**: The orientation of the cross-section is crucial, determining whether it is perpendicular to the x-axis or y-axis.
- 📏 **Calculating Cross-Section Area**: The area of a square cross-section is calculated as the square of the side length (s^2), and for a rectangle, it's width times height.
- ∫ **Integral Setup**: To find the volume, set up an integral from the lower to upper bounds of the solid, integrating the area of the cross-section with respect to x or y.
- 📈 **Graphs and Cross-Sections**: The script uses graphs to visualize how cross-sections relate to the overall shape of the solid and how they contribute to its volume.
- 🔢 **Solving for Boundaries**: When no graph is provided, solve equations to find where the functions intersect, which sets the bounds for the integral.
- 🎲 **Practical Application**: The method is applied to various problems, including those where the cross-sections are not squares or rectangles, to practice setting up integrals.
- 📝 **Unit Consistency**: Ensure that units are consistent when calculating volume, as the volume will be in cubic units if the base area is in square units.
- 📚 **AP Exam Focus**: The script emphasizes the importance of mastering this technique, as it is a common problem type on the AP Calculus exam, particularly with squares and with respect to the x-axis.
Q & A
What is the main topic of the calculus lesson?
-The main topic of the lesson is the volume of solids, specifically focusing on how to calculate the volume when the cross sections are squares and rectangles.
What is a cross section in the context of the lesson?
-A cross section is the shape obtained when an object is sliced perpendicularly to a certain axis, in this case, either the x-axis or y-axis, to reveal its internal structure.
Why is it important to know the direction of the cross section?
-Knowing the direction of the cross section is crucial because it determines whether the calculation is with respect to the x-axis or y-axis, which affects the setup of the integral for finding the volume.
What is the formula for the area of a square?
-The formula for the area of a square is s^2, where s is the length of one side of the square.
How does the shape of the cross section influence the calculation of volume?
-The shape of the cross section directly influences the calculation of volume because the volume is found by integrating the area of the cross sections over the given interval. Different shapes (squares, rectangles, etc.) will have different area formulas.
What is the process for setting up an integral to find the volume of a solid with square cross sections?
-The process involves identifying the side of the square from the difference between two functions, squaring this side to get the area of the cross section, and then integrating this area from the lower to the upper limit of the interval.
How does one determine the boundaries for the integral when given a graph?
-The boundaries for the integral are determined by finding the points where the two graphs intersect, which are the limits between which the integral is evaluated.
What is the difference between calculating the volume of a solid with cross sections that are squares and rectangles?
-For squares, the area is simply the side length squared (s^2). For rectangles, the area is the product of the width and height, where the width is the distance between the two functions and the height is given or can be determined from the problem statement.
Why is it essential to consider the units when calculating the volume?
-The units are essential because they determine the physical dimensions of the volume. For instance, if the area is in square centimeters, then the volume will be in cubic centimeters.
What is the strategy for setting up an integral when the cross sections are perpendicular to the y-axis?
-When the cross sections are perpendicular to the y-axis, the setup involves expressing the functions in terms of y, determining the y-values where the functions intersect, and integrating the area of the cross sections with respect to y over that interval.
Why is it beneficial to visualize the three-dimensional shape when calculating volume?
-Visualizing the three-dimensional shape helps in understanding how the cross sections fit together to form the solid, making it easier to conceptualize the integration process and the resulting volume.
Outlines
📐 Introduction to Calculus and Volume of Solids
This paragraph introduces the topic of calculus, specifically focusing on the volume of solids. The speaker, Mr. Bean, explains that the area formed by bounded regions in a two-dimensional plane becomes the base of a three-dimensional solid. The concept of viewing the solid from above, where only the outline is visible, is introduced. The importance of cross-sections in determining the shape of the solid is emphasized, with the perpendicular direction to the x-axis being a key factor. The lesson plans to cover squares and rectangles as shapes for cross-sections and uses a graphical example to illustrate how stacking multiple cross-sections can help visualize the solid's shape. The mathematical process involves setting up integrals to calculate the volume by summing up the areas of the cross-sections.
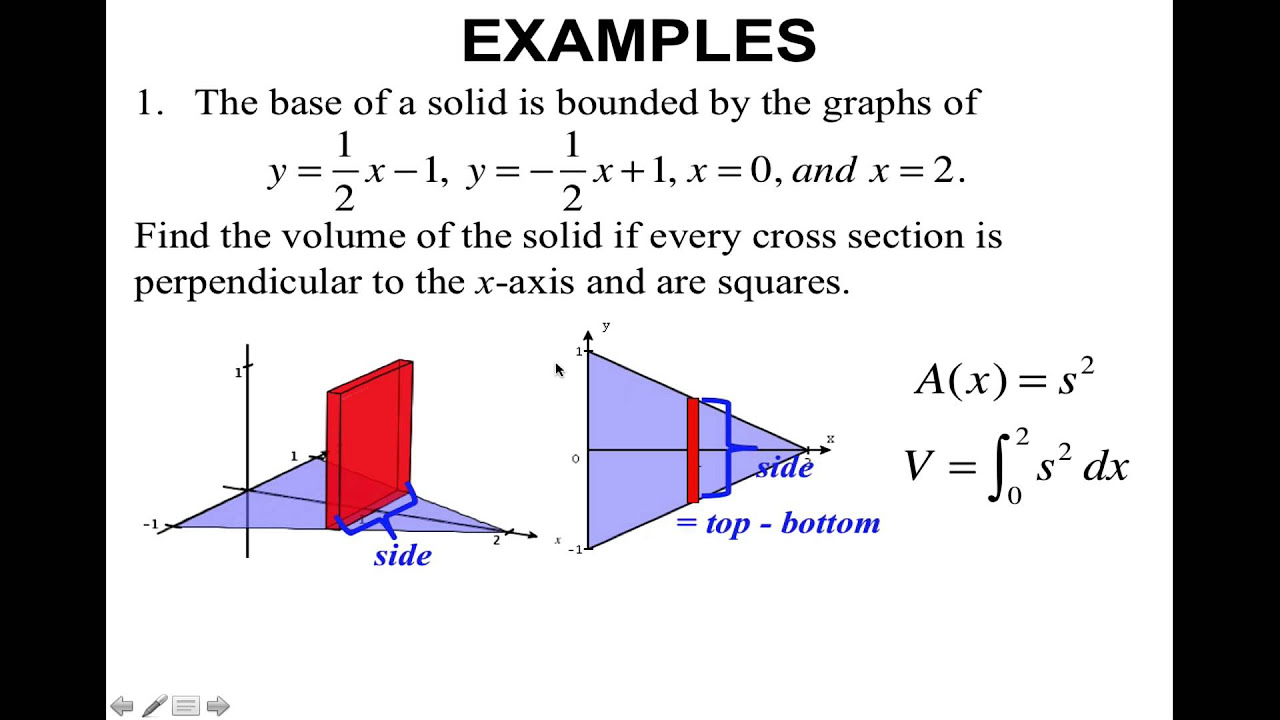
📏 Calculating Volume with Cross-Sections
The second paragraph delves into the process of calculating the volume of a solid using its cross-sectional areas. The speaker discusses the formula for the area of a square, which is the side length squared (s^2), and how to determine the side length (s) by subtracting the bottom function from the top function of the graph. The integral calculus method is introduced to sum up all possible cross-sections between given boundaries, in this case, from zero to one. The speaker also touches on the process of calculating the volume of a solid with rectangular cross-sections, emphasizing the need to know the height and width of the rectangle, which are derived from the problem's context. The importance of units in the calculation is highlighted, and the speaker provides an example of setting up an integral for a solid with square cross-sections perpendicular to the y-axis.
📈 Setting Up Integrals for Volume Calculations
The final paragraph focuses on setting up integrals for volume calculations when the cross-sections are squares or rectangles, and when the orientation is perpendicular to the y-axis. The speaker explains the process of converting equations into terms of y when necessary and setting the boundaries for the integral based on the smallest and largest y-values. Examples are provided to illustrate the setup for both square and rectangular cross-sections, including how to handle expressions that involve multiples of the width or a fixed height. The speaker also addresses the scenario when no graph is provided, requiring the solver to determine the boundaries where two functions intersect. The paragraph concludes with a reminder of the importance of mastering this technique, especially for the AP exam, and a preview of the next lesson, which will cover semi-circles and triangles.
Mindmap
Keywords
💡Volume of Solids
💡Cross Sections
💡Perpendicular
💡Integration
💡Squares and Rectangles
💡Graphs
💡Bounded Regions
💡Units
💡AP Exam
💡Mastery Check
💡Limits of Integration
Highlights
Introduction to the concept of volume of solids, moving beyond area calculations.
Understanding that the area formed with bounded regions becomes the base of a solid.
The importance of recognizing cross sections and their perpendicular orientation to the x-axis or y-axis.
Exploring the shape of a solid by visualizing it from above and considering cross sections.
Demonstration of how a three-dimensional object can be constructed from adding multiple squares or cross sections.
The method of setting up integrals to find the volume of a solid with cross sections that are squares or rectangles.
Calculating the area of a square as the side length squared and integrating this over a given interval.
The process of finding the volume of a solid with rectangular cross sections given the width and height.
Using a calculator to find the numerical answer to the integral representing the volume of a solid.
The difference in approach when the cross sections are perpendicular to the y-axis instead of the x-axis.
Solving for the boundaries of integration when no graph is provided by finding where functions intersect.
The application of integral calculus to find the volume of complex shapes using the method of cross sections.
The significance of mastering the setup of integrals for the AP exam, especially for solids with square cross sections.
The use of units in calculations to ensure the volume is expressed in the correct units, such as cubic centimeters.
The upcoming lesson's focus on semi-circles and triangles as cross sections, building on the current lesson's foundation.
The commonality of square cross sections in the context of the AP exam, emphasizing the importance of this lesson's content.
Encouragement for students to practice setting up integrals and using a calculator for the mastery of the subject.
Transcripts
Browse More Related Video
Calculus AB/BC – 8.8 Volumes with Cross Sections: Triangles and Semicircles
Topic 43-Volumes of Solids with Known Cross Sections

Volume of Solids with Known Cross Sections

Calculating volume by known cross sections

Finding the Volumes of Known Cross Sections ❖ AP Calculus AB

Calculating the Volume of a Solid of Revolution by Integration
5.0 / 5 (0 votes)
Thanks for rating: