What is Calculus? (Mathematics)
TLDRCalculus, a mathematical discipline developed in the late 1600s by Isaac Newton and Gottfried Leibniz, revolves around two fundamental questions: how steep is a function at a point, and what is the area under the graph over a certain region. The derivative, which measures the rate of change or slope of a function at a point, is used to address the first question. The integral, on the other hand, is employed to calculate the area under curves, such as that of the function g(x) = sin x from x=0 to x=π. The concept of limits is central to both derivatives and integrals, as they represent the values that approximations approach. The video script delves into the techniques and rules for computing these mathematical tools, starting with basic functions and progressing to higher dimensions with partial derivatives and multiple integrals. It also highlights the application of calculus in solving real-world problems, promising more in-depth content in future videos.
Takeaways
- 📚 Calculus was developed in the late 1600s by Isaac Newton and Gottfried Wilhelm Leibniz, independently of each other.
- ❓ Calculus addresses two fundamental questions: the rate of change at a point (derivative) and the area under a curve (integral).
- 📈 The derivative measures the steepness or rate of change of a function at a specific point, often visualized as the slope of the tangent line.
- 📉 To find the derivative, one can use the limit process by taking points closer to the point of interest and finding the slope of the line through these points.
- 🔍 The integral is used to find the area under a curve, which can be approximated by summing the areas of rectangles that fit under the curve.
- 🔢 The limit is a key concept in calculus, representing the value that a function or sequence approaches as the input approaches a certain point.
- 📝 Basic functions in calculus include polynomials, trigonometric functions, exponential functions, and logarithmic functions.
- 🔧 Calculus involves learning various rules for derivatives and integrals, such as the Product Rule, Quotient Rule, Chain Rule, U-substitution, and Integration by Parts.
- 📏 After mastering basic functions, calculus extends to higher dimensions, involving partial derivatives and double integrals for functions with two or more variables.
- 🌐 In higher dimensions, the concept of a tangent plane replaces the tangent line, and calculus is used to describe phenomena in multi-dimensional spaces.
- 🌟 Calculus has practical applications in solving real-world problems, from physics to engineering to economics, by analyzing rates of change and accumulations.
- 📹 The video script suggests subscribing or supporting the channel for more calculus content, indicating the importance of continuous learning in this field.
Q & A
Who are the two mathematicians credited with the creation of calculus, and in what century was it developed?
-Calculus was developed in the late 1600s by Isaac Newton and Gottfried Wilhelm Leibniz, who independently created the subject without collaboration.
What are the two fundamental questions that calculus addresses regarding functions?
-The two fundamental questions of calculus are: 1) How steep is a function at a specific point (addressed by the derivative), and 2) What is the area underneath the graph of the function over a certain region (addressed by the integral).
How does the concept of a derivative relate to the rate of change of a function?
-A derivative measures the rate of change of a function at a specific point. It is essentially the slope of the tangent line to the graph of the function at that point, indicating how quickly the function's value is changing.
What is the process of finding the slope of a curve at a point called, and how is it achieved?
-The process is called finding the derivative. It is achieved by picking points closer and closer to the point of interest, drawing a line through these points, and computing the slope of this line. As the points get closer, the slope of these lines approaches the slope of the tangent line at the point, which is the derivative.
How does the concept of an integral relate to finding the area under a curve?
-An integral is a tool that allows you to find the area under a curve by approximating it with rectangles. By slicing the region under the curve into thinner and thinner rectangles and summing their areas, you get a sequence of areas that approach the actual area under the curve, which is the integral.
What is the term for the number that a sequence of approximations is approaching, and why is it important in calculus?
-The term is 'limit'. Limits are important in calculus because they are key to computing both derivatives and integrals. They allow us to find the exact value that a function is approaching at a certain point or over a range of numbers.
What are some of the basic functions that are typically the starting point for learning calculus?
-The basic functions typically studied first in calculus include polynomials, trigonometric functions (sine, cosine, and tangent), exponential functions, and logarithmic functions. These serve as building blocks for more complex functions.
What are partial derivatives, and how do they relate to functions in higher dimensions?
-Partial derivatives are derivatives computed in multiple dimensions, specifically for functions with more than one input (like f(x, y)). They describe the rate of change of the function in each input direction separately and are used to define the tangent plane to a surface in higher dimensions.
How does a double integral generalize the concept of an integral to three-dimensional regions?
-A double integral generalizes the concept of an integral by approximating the volume under a surface using a series of 'skinny boxes' that spread out in two dimensions. By summing up the volumes of these boxes, you can compute the total volume, which corresponds to the area under the curve in two-dimensional calculus.
What is a scalar field, and how does it differ from a vector field in the context of calculus?
-A scalar field is a function with three inputs (x, y, and z) and one output, such as a function returning the temperature at any point in space. It differs from a vector field, which has three inputs and a vector output, like a function giving the force vector due to gravity at every point in space.
What are some of the derivative rules that are essential for finding derivatives of complex functions in calculus?
-Some essential derivative rules include the Product Rule, Quotient Rule, and Chain Rule. These rules help in finding the derivatives of functions that are products, quotients, or compositions of simpler functions.
How does the study of calculus extend beyond basic functions to real-world applications?
-After mastering the derivatives and integrals of basic functions, calculus is applied to higher dimensions using partial derivatives and multiple integrals. It is then used to solve real-world problems involving rates of change, areas, volumes, and other phenomena that can be mathematically modeled using calculus.
Outlines
📚 Introduction to Calculus
The first paragraph introduces the subject of calculus, which was developed in the late 1600s by Isaac Newton and Gottfried Leibniz independently of each other. It explains that calculus revolves around two fundamental questions: determining the steepness (rate of change) of a function at a specific point, known as finding the derivative, and calculating the area under a curve over a certain region, which is done using integrals. The paragraph also uses the function f(x) = x^3 - x^2 - 4x + 4 to illustrate how derivatives can be approximated by examining the slope between two points that get progressively closer, eventually leading to the concept of the tangent line and the derivative as the limit of this process. The integral is introduced as a tool for finding areas under curves, using the function g(x) = sin x and the technique of slicing the area under the curve into thin rectangles to approximate the total area, which is refined as the slices become thinner, leading to the concept of the integral.
🔍 Techniques and Applications in Calculus
The second paragraph delves into the techniques used in calculus, emphasizing the role of limits in both finding derivatives and integrals. It highlights that calculus initially focuses on basic functions such as polynomials, trigonometric functions, exponential functions, and logarithmic functions, which serve as the foundation for more complex functions created through mathematical operations and function composition. The paragraph outlines various rules for computing derivatives and integrals, including the Product Rule, Quotient Rule, Chain Rule, U-substitution, Integration by Parts, and Partial Fraction Decomposition. It also touches on the extension of calculus to higher dimensions, involving partial derivatives and double integrals for functions with multiple inputs, such as scalar and vector fields. The paragraph concludes by encouraging the learner to start their study of calculus by mastering these tools and applying them to solve real-world problems, with a teaser for upcoming videos on the subject.
Mindmap
Keywords
💡Calculus
💡Derivative
💡Integral
💡Limit
💡Function
💡Tangent Line
💡Rate of Change
💡Polynomials
💡Trigonometric Functions
💡Partial Derivatives
💡Multiple Integrals
Highlights
Calculus was created in the late 1600s by Isaac Newton and Gottfried Leibniz independently of each other.
Calculus begins with two fundamental questions about functions: the steepness at a point and the area under the graph over a region.
The derivative is a tool used to measure the steepness or rate of change of a function at a specific point.
The integral is used to calculate the area under a curve, such as that of the function g(x) = sin x between x=0 and x=pi.
To find the steepness of a curve, one can approximate it by finding the slope between two nearby points and narrowing the distance for increased accuracy.
The process of finding the derivative involves the concept of a limit, which is a key technique in calculus.
Integrals are computed by approximating the area under a curve with thinner and thinner rectangles, leading to a sequence of areas converging to a limit.
Calculus involves learning to find derivatives and integrals of basic functions such as polynomials, trigonometric, exponential, and logarithmic functions.
Complex functions can be constructed by combining basic functions through addition, subtraction, multiplication, division, and composition.
Derivative rules like the Product Rule, Quotient Rule, and Chain Rule, as well as integral rules such as U-substitution, Integration by Parts, and Partial Fraction Decomposition, are essential in calculus.
Calculus extends to higher dimensions, involving partial derivatives and double integrals for functions with two or more inputs.
In higher dimensions, the concept of a tangent plane replaces the tangent line, and the volume under a surface is calculated instead of area.
Scalar fields and vector fields are functions with three inputs and one or vector output, respectively, which are important in physics and engineering.
Calculus is not only theoretical but also has practical applications in solving real-world problems.
The study of calculus involves a significant amount of time spent computing derivatives and integrals of various functions.
Learning calculus begins with understanding limits, which are foundational to both derivatives and integrals.
Socratica will release more calculus videos to help learners understand the subject in detail.
Transcripts
Browse More Related Video

Why Are Slope and Area Opposite: The Fundamental Theorem of Calculus
Introduction to calculus [IB Maths AI SL/HL]
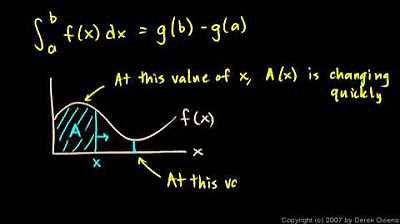
Calculus - The Fundamental Theorem, Part 2

Techniques for Finding Derivatives

01 - Basic Derivatives in Calculus, Part 1 - Learn what a Derivative is and how to Solve Them.

Math 1325 Lecture 13 2
5.0 / 5 (0 votes)
Thanks for rating: