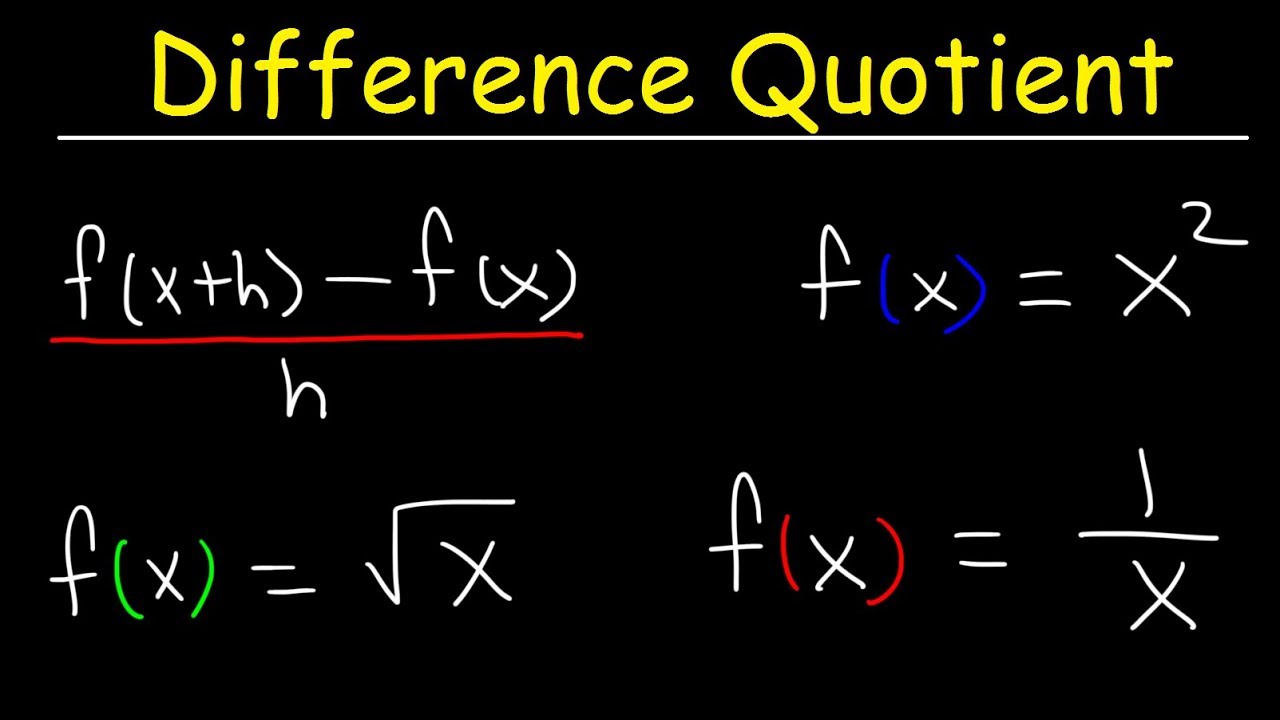Average Rate of Change Using Difference Quotient

Sun Surfer Math
5 Feb 202209:26
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses a method for calculating the average rate of change using the difference quotient. The presenter explains that the difference quotient formula, f(a+h) - f(a) / h, is a versatile tool for finding the average rate of change over different intervals without starting the problem from scratch each time. The example given involves a function f(x) = 27t - 24t^2, with the value of 'a' set to 1 and 'h' values of 0.01 and 0.05. The presenter demonstrates how to apply the formula and suggests using Desmos, an online graphing calculator, to simplify the calculations. By plugging in different values of 'h', the presenter shows how to find the average rate of change for various intervals, highlighting the efficiency of using the difference quotient over the traditional formula.

Takeaways
  • 📐 The video discusses an average rate of change problem using the difference quotient formula.
  • 🔢 The difference quotient is defined as f(a+h) - f(a) / h, which is used to find the average rate of change over an interval.
  • ✍️ The formula f(b) - f(a) / (b - a) is another version of the average rate of change formula, but it's not used in this case due to varying h values.
  • 📈 The function given in the problem is f(x) = 27t - 24t^2, where 't' represents time.
  • ⏱️ The value of 'a' (the starting time) is set to 1, and two different values of 'h' (0.01 and 0.05) are used to find the rate of change.
  • 📉 The interval for the first calculation is from 1 to 1.01, and for the second is from 1 to 1.05, representing small changes in time.
  • 🧮 The function is evaluated at f(1.01) and f(1) for the first interval, and similarly for the second interval with h=0.05.
  • 📊 Desmos is suggested as a tool to help with the calculations, providing a slider to adjust the value of 'h'.
  • 📌 When h=0.01, the average rate of change is calculated to be -21.24.
  • 📉 For h=0.05, the average rate of change is found to be -22.2.
  • 🔧 The process can be repeated for other values of 'h', such as 0.0001, to find the corresponding rate of change.
  • ✨ The advantage of using the difference quotient is that it allows for easy recalculations with different 'h' values without starting the problem from scratch.
Q & A

  • What is the main topic of the video?

    -The video is about solving an average rate of change problem using the difference quotient.

  • What is the formula for the difference quotient?

    -The difference quotient is given by the formula (f(a + h) - f(a)) / h.

  • How is the difference quotient related to the average rate of change formula?

    -The difference quotient is another version of the average rate of change formula, which is f(b) - f(a) / (b - a). They are related in that they both measure the rate of change over an interval, but the difference quotient allows for variable h values.

  • What is the function f(x) used in the problem?

    -The function f(x) used in the problem is 27t - 24t^2.

  • What value is chosen for 'a' in the formula?

    -The value chosen for 'a' is 1, representing a specific time.

  • What are the two values of 'h' used in the problem?

    -The two values of 'h' used are 0.01 and 0.05.

  • What tool or software is suggested for evaluating the function?

    -Desmos, an online graphing calculator, is suggested for evaluating the function.

  • What is the result of the first part of the problem when h is 0.01?

    -The result of the first part when h is 0.01 is -21.24.

  • How does changing the value of 'h' affect the calculation?

    -Changing the value of 'h' alters the interval and the values used in the function, thus providing a different rate of change.

  • What is the result when h is changed to 0.05?

    -When h is changed to 0.05, the result of the calculation is -22.2.

  • Why is the difference quotient method preferred in this context?

    -The difference quotient method is preferred because it allows for easy recalculations with different 'h' values without starting the problem from scratch.

  • What is the final value obtained when h is set to 0.0001?

    -When h is set to 0.0001, the final value obtained is -30.

Outlines
00:00
📐 Calculating Average Rate of Change Using the Difference Quotient

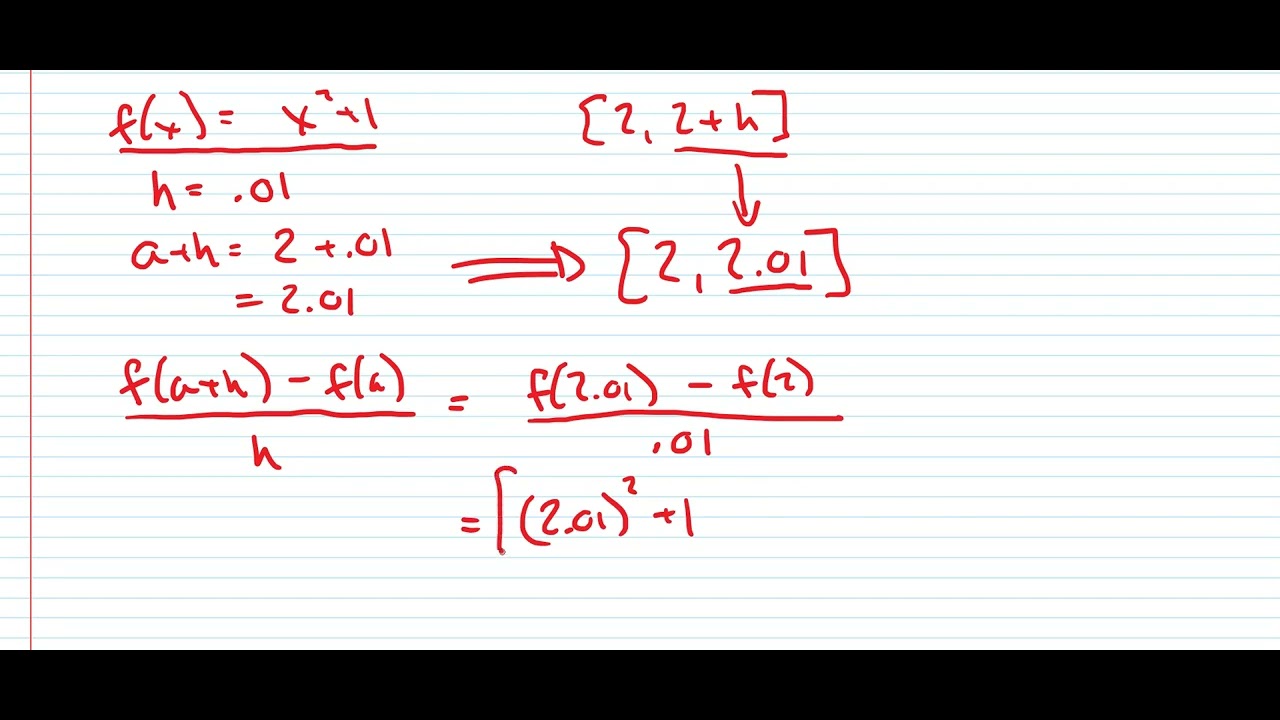
This paragraph introduces the concept of the average rate of change in the context of a mathematical problem. The presenter explains the use of the difference quotient formula, which is f(a+h) - f(a) / h, as opposed to the traditional average rate of change formula f(b) - f(a) / (b - a). The choice of the difference quotient is justified by the need to use different values of h without starting the problem from scratch. The function f(x) = 27t - 24t^2 is given, with a specific value of 'a' (time equals one) and two values of h (0.01 and 0.05) to calculate the rate of change over intervals from a to a+h. The presenter demonstrates the calculation process for h=0.01, using Desmos to simplify the computation, and arrives at an approximate value of 2.7876 for the rate of change.

05:03
🔢 Further Calculations with Different Values of h

The second paragraph continues the discussion on calculating the average rate of change with different values of h. The presenter reiterates the process using the function f(x) = 27t - 24t^2, this time with h=0.05. The calculation is simplified by using Desmos, which allows for easy adjustments of the h value and recalculations. The presenter demonstrates changing the h value in Desmos and computes the rate of change for h=0.05, resulting in a value of approximately -22.2. The paragraph also explores the flexibility of the method by showing how to calculate the rate of change for an even smaller h value of 0.0001, which results in a rate of change of approximately -30. The presenter emphasizes the efficiency of using the difference quotient and Desmos for such calculations, especially when needing to recalculate for different values of h.

Mindmap
Keywords
💡Difference Quotient
The difference quotient is a mathematical formula used to estimate the rate of change of a function at a given point. It is defined as the function's value at 'a plus h' minus the function's value at 'a', all divided by 'h'. In the video, it is used to calculate the average rate of change for the function f(x) = 27t - 24t^2 at different intervals, which is central to the problem-solving process.
💡Average Rate of Change
The average rate of change is a measure of how a function's value changes over an interval. It is calculated by finding the difference in the function's values at two points and dividing by the difference in the x-coordinates of those points. In the video, the concept is applied to the function f(x) to find the rate of change over specific intervals, demonstrating its application in calculus.
💡Function
In mathematics, a function is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. In the video, the function f(x) = 27t - 24t^2 is used as an example to illustrate the calculation of the difference quotient and average rate of change.
💡Desmos
Desmos is an online graphing calculator that allows users to plot functions and manipulate variables to see how they affect the graph. In the video, Desmos is used to simplify the calculation of the difference quotient by allowing the user to input the function and see the result for different values of 'h' without manual calculation.
💡Interval
In mathematics, an interval refers to a continuous range of values within a given set, often used in the context of functions to define the domain or the range over which a function is being considered. In the video, the intervals from 1 to 1.01 and from 1 to 1.05 are used to calculate the average rate of change for the given function.
💡H Value
The 'h' value in the context of the difference quotient represents the change in the independent variable (usually time or a similar continuous variable). It is used to calculate the rate of change over small intervals. In the video, 'h' values of 0.01, 0.05, and 0.0001 are used to demonstrate how the average rate of change varies with different intervals.
💡Slider
A slider in the context of Desmos is a user interface element that allows for the input of a variable by sliding it along a range. In the video, a slider is used to easily change the 'h' value and observe the corresponding change in the difference quotient, which aids in understanding how the rate of change varies with different 'h' values.
💡Calculus
Calculus is a branch of mathematics that deals with rates of change and the accumulation of small quantities to find the total quantity. It includes both differential and integral calculus. The video is focused on differential calculus, specifically using the difference quotient to find the average rate of change of a function.
💡Squared
In mathematics, 'squared' refers to the operation of multiplying a number by itself. It is represented by the exponent 2. In the video, the term is used in the function f(x) = 27t - 24t^2, where t^2 denotes the square of the variable t, which is a key component in the calculation of the function's rate of change.
💡Negative Number
A negative number is a number that is less than zero. In the context of the video, the negative number arises from the calculation of the difference quotient when the function's value at 'a plus h' is less than the function's value at 'a'. This indicates a decrease in the function's value over the interval considered.
💡Recalculation
Recalculation is the process of reevaluating or recomputing a value or result. In the video, recalculation is mentioned in the context of using the difference quotient formula with different 'h' values. It highlights the convenience of using the formula and a tool like Desmos, which allows for quick recalculations without starting the problem from scratch.
Highlights

The video discusses an average rate of change problem using the difference quotient.

The difference quotient formula is f(a + h) - f(a) / h, which is used to find the average rate of change.

The formula is a variation of the average rate of change formula f(b) - f(a) / (b - a).

The video demonstrates how to use the difference quotient with different values of h.

The function f(x) used in the problem is 27t - 24t^2, with a value of a being 1.

Two h values are used: 0.01 and 0.05, creating intervals from 1 to 1.01 and from 1 to 1.05 respectively.

The difference quotient is set up as f(1.01) - f(1) / 0.01 for the first interval.

Desmos is suggested for evaluating the function, especially for the first part with h = 0.01.

When h = 0.01, the average rate of change is calculated to be -21.24.

For h = 0.05, the calculation is repeated with the new value, resulting in -22.2.

The video also shows how to adjust the calculation for other values of h, like 0.0001, using a slider in Desmos.

Using the difference quotient allows for easy recalculations without starting the problem from scratch.

The method is particularly useful for problems that require multiple calculations with different h values.

The video emphasizes the efficiency of using the difference quotient over the traditional formula.

Desmos is used as a tool to facilitate the calculation process and visualize the results.

The final calculation for h = 0.0001 results in an average rate of change of -30.

The video concludes by highlighting the practicality of the difference quotient in solving rate of change problems.

Transcripts

Browse More Related Video

Introduction to the Difference Quotient

Math 11 - Sections 1.3-1.4

1.3 - Average Rates of Change

Difference Quotient

Average Rate of Change of a Rational Function

More Difference Quotient Examples

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Difference QuotientAverage RateChange CalculationDynamic FunctionDesmos ToolMath ProblemInterval AnalysisFunction EvaluationNumerical MethodsEducational ContentMathematics Tutorial