Difference Quotient

The Organic Chemistry Tutor
10 Feb 201813:38
EducationalLearning
32 Likes 10 Comments

TLDRThis lesson delves into calculating the difference quotient of various functions. Starting with a simple function f(x) = 7x, the instructor demonstrates the formula f(x+h) - f(x)/h, leading to a simplified result of 7. The process is then applied to more complex functions, such as f(x) = 5x+4, resulting in a quotient of 5, and f(x) = x^2, yielding 2x+h. The lesson further explores the difference quotient for functions like the square root of x and 1/x, concluding with a comprehensive example for f(x) = 3x^2 + 4x - 5. Each step is meticulously explained, ensuring a clear understanding of the concept.

Takeaways
  • 📚 The lesson focuses on finding the difference quotient of a function, which is a mathematical concept used to approximate the derivative of a function.
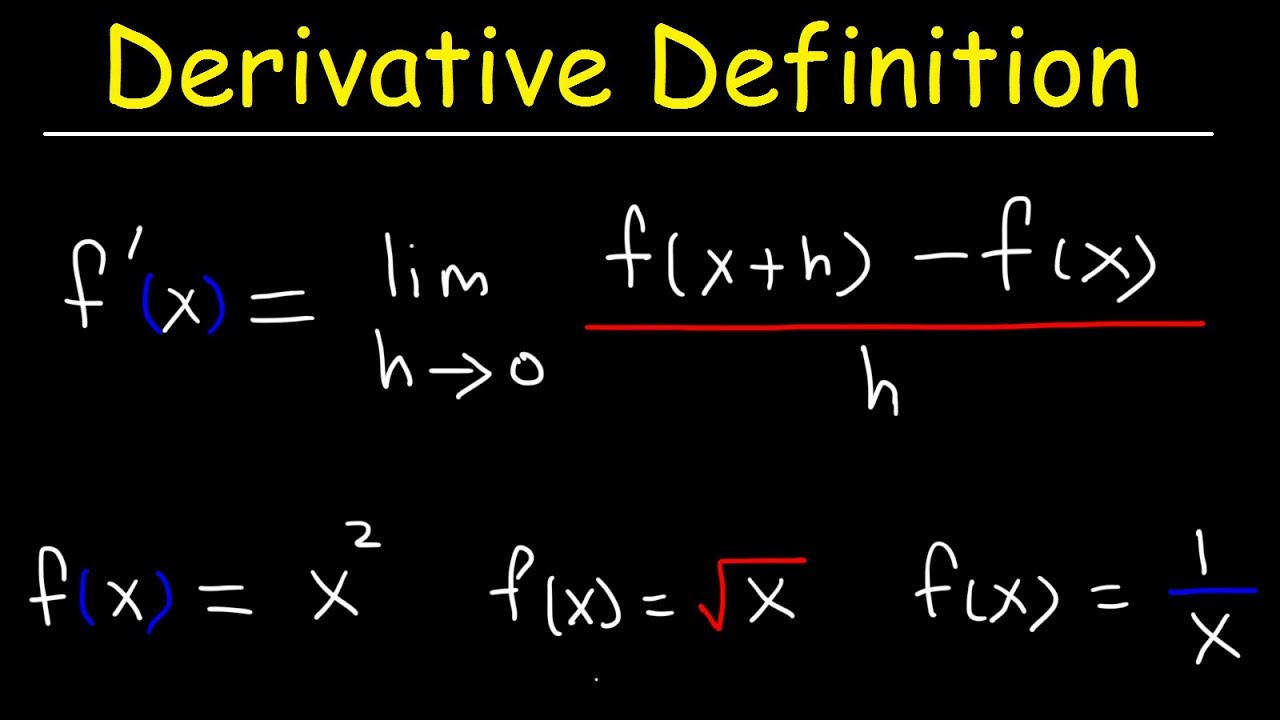
  • 🔍 The formula for the difference quotient is \( \frac{f(x + h) - f(x)}{h} \), where \( f(x) \) is the function and \( h \) is a small change in \( x \).
  • 👉 For the function \( f(x) = 7x \), the difference quotient is found by replacing \( x \) with \( x + h \) and simplifying, resulting in \( 7 \).
  • 📈 When \( f(x) = 5x + 4 \), the process involves distributing the 5 and simplifying the expression to get a difference quotient of \( 5 \).
  • 🎯 For a quadratic function \( f(x) = x^2 \), the difference quotient is calculated using the FOIL method and simplification, leading to \( 2x + h \).
  • 🛑 The difference quotient of \( \sqrt{x} \) involves multiplying by the conjugate to rationalize the denominator, resulting in \( \frac{1}{\sqrt{x + h} + \sqrt{x}} \).
  • 📉 For the function \( f(x) = \frac{1}{x} \), the difference quotient is found by multiplying by a common denominator and simplifying to get \( -\frac{1}{x(x + h)} \).
  • 🔢 In the case of \( f(x) = 3x^2 + 4x - 5 \), the difference quotient involves expanding and simplifying the expression, leading to \( 6x + 3h + 4 \).
  • 🌟 The process of finding the difference quotient involves algebraic manipulation, including distributing, combining like terms, and factoring out common factors.
  • 📝 Each example in the script demonstrates a step-by-step approach to finding the difference quotient, emphasizing the importance of algebraic simplification.
Q & A

  • What is the difference quotient and how is it represented?

    -The difference quotient is a mathematical expression used to estimate the rate of change of a function at a certain point. It is represented by the formula \( \frac{f(x + h) - f(x)}{h} \).

  • What is the function f(x) equal to in the script's first example?

    -In the first example of the script, the function f(x) is equal to 7x.

  • How do you find the difference quotient of the function f(x) = 7x?

    -To find the difference quotient of f(x) = 7x, you replace x with (x + h) in the function to get f(x + h) = 7(x + h), then subtract f(x) from f(x + h) and divide by h, which simplifies to 7.

  • What is f(x + h) for the function f(x) = 5x + 4?

    -For the function f(x) = 5x + 4, f(x + h) is found by replacing x with (x + h), resulting in 5(x + h) + 4.

  • What is the difference quotient of the function f(x) = 5x + 4?

    -The difference quotient of f(x) = 5x + 4 is 5, after simplifying the expression obtained by substituting x with (x + h) and following the difference quotient formula.

  • How do you determine the difference quotient for the function f(x) = x^2?

    -To determine the difference quotient for f(x) = x^2, you replace x with (x + h) to get (x + h)^2, then apply the difference quotient formula, which simplifies to 2x + h after canceling terms.

  • What is the difference quotient of the square root function, f(x) = √x?

    -The difference quotient of the square root function, f(x) = √x, is found by multiplying the numerator and denominator by the conjugate of the radical, which simplifies to \( \frac{1}{\sqrt{x + h} + \sqrt{x}} \).

  • What is the function f(x + h) for the reciprocal function, f(x) = 1/x?

    -For the reciprocal function f(x) = 1/x, f(x + h) is 1/(x + h).

  • What is the difference quotient of the reciprocal function f(x) = 1/x?

    -The difference quotient of the reciprocal function f(x) = 1/x is \( -\frac{1}{x(x + h)} \) after simplifying the expression obtained by substituting x with (x + h) and following the difference quotient formula.

  • How do you calculate the difference quotient for the quadratic function f(x) = 3x^2 + 4x - 5?

    -To calculate the difference quotient for f(x) = 3x^2 + 4x - 5, you replace x with (x + h), apply the difference quotient formula, and simplify the expression, which results in 6x + 3h + 4 after canceling terms.

  • What is the significance of the difference quotient in calculus?

    -The difference quotient is significant in calculus as it is used to find the derivative of a function, which represents the slope of the tangent line to the function at a given point.

Outlines
00:00
📚 Introduction to Difference Quotient

This paragraph introduces the concept of the difference quotient in the context of a function, f(x) = 7x. It explains the formula for the difference quotient, which is (f(x + h) - f(x)) / h. The process involves substituting x with (x + h) to find f(x + h), and then simplifying the expression to find the difference quotient. The example provided simplifies to 7, indicating that the difference quotient for the function 7x is 7. The paragraph also encourages the viewer to try the same process with the function f(x) = 5x + 4.

05:01
🔍 Calculating Difference Quotients for Various Functions

This paragraph demonstrates the process of finding the difference quotient for different functions. It starts with f(x) = 5x + 4, where the difference quotient simplifies to 5 after canceling terms. Next, it tackles f(x) = x^2, using the FOIL method to expand (x + h)^2 and simplifying the expression to get the difference quotient, 2x + h. The paragraph also covers the square root function, f(x) = √x, and explains how to manipulate the expression to arrive at the difference quotient of 1/(√x + h + √x). Lastly, it discusses the function f(x) = 1/x, where multiplying by the common denominator simplifies the expression to -1/(x(x + h)).

10:02
📘 Advanced Difference Quotient Calculations

The final paragraph delves into more complex functions, starting with f(x) = 3x^2 + 4x - 5. It guides through the process of finding f(x + h) by substituting x with (x + h) and expanding the terms. The paragraph uses the distributive property to simplify the expression, canceling out terms that contain x and arriving at the difference quotient of 6x + 3h + 4. This demonstrates the application of the difference quotient concept to polynomial functions, highlighting the steps of expansion, simplification, and cancellation to reach the final result.

Mindmap
Keywords
💡Difference Quotient
The difference quotient is a mathematical concept used to approximate the rate of change of a function at a certain point. It is defined as the ratio of the change in the function's value to the change in its input variable. In the video, the difference quotient is represented by the formula \( \frac{f(x + h) - f(x)}{h} \), and it is used to find the derivative of various functions, illustrating how it is applied to functions like \( f(x) = 7x \) and \( f(x) = x^2 \).
💡Function
A function in mathematics is a relation between a set of inputs and a set of permissible outputs, with the property that each input is related to exactly one output. In the context of the video, functions such as \( f(x) = 7x \), \( f(x) = 5x + 4 \), and \( f(x) = x^2 \) are used to demonstrate the process of finding their difference quotients, which is key to understanding the concept of derivatives.
💡Derivative
The derivative of a function at a chosen input value, when taken as the limit of the difference quotient as \( h \) approaches zero, represents the rate at which the function is changing at that point. Although not explicitly defined in the script, the concept of the derivative is central to the process of finding the difference quotient, as it is the foundation for understanding the instantaneous rate of change.
💡Variable
In mathematics, a variable represents a value that can change. In the video, 'x' is the variable for which the function's value is determined. The process of finding the difference quotient involves manipulating the variable 'x' by adding 'h' to it, as seen in examples like \( f(x + h) \), to understand how the function changes as 'x' changes.
💡Rate of Change
The rate of change refers to how much a function's value changes in response to changes in its input variable. In the video, the difference quotient is used to approximate the rate of change of functions like \( f(x) = 7x \) and \( f(x) = x^2 \), providing insight into how these functions behave as 'x' varies.
💡Foil Method
The foil method is a technique used to multiply two binomials. It stands for 'First, Outer, Inner, Last', which refers to the steps of multiplying each term in the first binomial by each term in the second. In the video, the foil method is used when finding the difference quotient of \( f(x) = x^2 \), where \( (x + h)^2 \) is expanded to \( x^2 + 2xh + h^2 \).
💡Conjugate
In the context of the video, the conjugate refers to a mathematical expression that is the same as another but with the opposite sign. When dealing with square roots in the difference quotient, the conjugate is used to rationalize the denominator, as shown when finding the difference quotient of \( f(x) = \sqrt{x} \), where the conjugate \( \sqrt{x} + \sqrt{x+h} \) is multiplied to eliminate the radical in the denominator.
💡Greatest Common Factor (GCF)
The greatest common factor of two or more numbers is the largest positive integer that divides each of the numbers without a remainder. In the video, the GCF is used to simplify expressions, such as when finding the difference quotient of \( f(x) = x^2 \), where \( h \) is factored out from the terms \( 2xh \) and \( h^2 \) and then canceled with the \( h \) in the denominator.
💡Complex Fraction
A complex fraction is a fraction that has another fraction as its whole number part, numerator, or denominator. In the script, when finding the difference quotient of \( f(x) = \frac{1}{x} \), a complex fraction arises, and the method of multiplying by the common denominator is used to simplify it, illustrating how to handle such expressions.
💡Distributive Property
The distributive property states that the product of a number and a sum is the same as the sum of the products of the number and each addend. In the video, this property is used multiple times, such as when finding the difference quotient of \( f(x) = 3x^2 + 4x - 5 \), where the terms are distributed to simplify the expression.
Highlights

Introduction to finding the difference quotient of a function.

Explanation of the difference quotient formula: f(x+h) - f(x) / h.

Example calculation for f(x) = 7x, resulting in a difference quotient of 7.

Method to find f(x+h) by substituting x with x+h.

Simplification process by canceling out like terms.

Application of the difference quotient formula to f(x) = 5x + 4, yielding a result of 5.

Demonstration of distributing and simplifying expressions within the difference quotient.

Finding the difference quotient for f(x) = x^2, resulting in 2x + h.

Use of FOIL method for multiplying binomials in the difference quotient calculation.

Introduction to the difference quotient of the square root function, √x.

Strategy for dealing with radicals in the difference quotient by multiplying by the conjugate.

Calculation of the difference quotient for √x, resulting in 1/(√(x+h) + √x).

Approach to finding the difference quotient for the function f(x) = 1/x.

Multiplication of complex fractions in the difference quotient calculation.

Final result for the difference quotient of 1/x, which is -1/(x(x+h)).

Complex example with f(x) = 3x^2 + 4x - 5, and the steps to find its difference quotient.

Detailed foiling process for the quadratic term in the difference quotient.

Final expression for the difference quotient of 3x^2 + 4x - 5, which simplifies to 6x + 4 + 3h.

Transcripts

Browse More Related Video

Decomposing Functions - Composition of Functions

Definition of the Derivative

Finding Derivative Using Limit Definition

Composite Functions

Average Rate of Change of a Function Over an Interval

Composite Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Difference QuotientMathematicsFunction AnalysisEducational ContentStep-by-Step GuideAlgebraCalculus BasicsSimplificationMath TutorialLearning Resource