Introduction to the Derivative
TLDRThis video script introduces the concept of the derivative, a fundamental concept in calculus. It begins by explaining the difference quotient, which is used to find the average rate of change between two points and the slope of the secant line between them. The script then explores the idea of letting the distance 'h' between the points become infinitesimally small, which leads to the definition of the derivative as the limit of the difference quotient as 'h' approaches zero. The derivative has two key interpretations: it represents the instantaneous rate of change at a single point on a graph and the slope of the tangent line at that point. The video aims to clarify when to use the difference quotient for average rates and secant slopes, and when to use the derivative for instantaneous rates and tangent slopes. The next video in the series will demonstrate how to apply the derivative to calculate these values.
Takeaways
- 📈 The concept of the derivative is introduced as a fundamental concept in calculus.
- 🔢 The difference quotient is defined as the difference in function values (f(a+h) - f(a)) divided by the difference in x-values (h).
- 📌 The difference quotient serves two purposes: finding the average rate of change between two points and determining the slope of the secant line between those points.
- ➗ As h becomes very small, the secant line approaches the tangent line at a single point on the graph.
- ∞ The limit is used to allow h to become infinitely small, which is crucial for defining the derivative.
- 📚 The derivative is formally defined as the limit of the difference quotient as h approaches zero.
- 🔍 The derivative has two key interpretations: it represents the instantaneous rate of change at a single point and the slope of the tangent line to the graph at that point.
- 📉 The instantaneous rate of change is the rate of change at a specific point on the graph, as opposed to the average rate of change over an interval.
- 🤔 The derivative is used when we are interested in what happens at a single point, not over an interval.
- 📍 When h approaches zero, the secant line becomes a tangent line, touching the graph at exactly one point.
- 🔑 The difference quotient is used for average rate of change and secant line slope, while the derivative is used for instantaneous rate of change and tangent line slope.
- 🚀 The next video will demonstrate how to use the derivative to find the instantaneous rate of change and the slope of a tangent line.
Q & A
What is the difference quotient?
-The difference quotient is defined as the difference between the function values at two points on a graph, divided by the distance between these points on the x-axis. Mathematically, it is expressed as (f(a + h) - f(a)) / h, where 'h' is the small distance between the points 'a' and 'a + h'.
How does the difference quotient relate to the average rate of change?
-The difference quotient is used to calculate the average rate of change between two points on a graph. It measures how much the function value changes, on average, for each unit of change in the x-axis between the two points.
What is the significance of making 'h' very small in the context of derivatives?
-Making 'h' very small in the calculation of the difference quotient is crucial for approaching the concept of a derivative. As 'h' approaches zero, the secant line between the two points becomes the tangent line at a single point, allowing us to examine the instantaneous rate of change at that specific point.
What does the limit of the difference quotient as 'h' approaches zero represent?
-The limit of the difference quotient as 'h' approaches zero defines the derivative of the function at point 'a'. This limit provides the slope of the tangent line at the point, reflecting the instantaneous rate of change of the function at that very point.
Why can't 'h' actually be zero when calculating the derivative?
-If 'h' were zero, it would mean that there is no interval over which to measure the change in function values, leading to a division by zero in the difference quotient. Therefore, we approach zero as closely as possible without actually reaching it, using the concept of a limit.
What is the difference between a secant line and a tangent line in this context?
-A secant line intersects a curve at two distinct points, reflecting the average rate of change between these points. In contrast, a tangent line touches the curve at exactly one point, providing the instantaneous rate of change at that point.
How does the derivative help in understanding the behavior of a function at a single point?
-The derivative, as the slope of the tangent line at a point, tells us the instantaneous rate of change of the function at that point. This helps in understanding how the function behaves and changes very close to that point, offering insights into its properties and trends.
What practical applications can derivatives have based on this explanation?
-Derivatives are extremely useful in various fields such as physics for understanding motion dynamics, in economics for analyzing changes in cost and revenue functions, and in engineering for studying rates of change in systems and materials.
What are the interpretations of the derivative mentioned in the video?
-The derivative has two key interpretations: it represents the instantaneous rate of change at a single point on a graph, and it is used to find the slope of the tangent line to the curve at that point.
What should one expect in the follow-up video mentioned at the end of the transcript?
-The follow-up video is expected to demonstrate practical methods for using the derivative to calculate instantaneous rates of change and to find the slope of tangent lines, likely including examples and problem-solving techniques.
Outlines
📈 Introduction to Derivatives and Difference Quotient
The first paragraph introduces the concept of derivatives in calculus by focusing on the difference quotient, a fundamental building block. The explanation begins with a visual representation of a graph with two points, point A and point A plus h, where h represents a very small distance. The difference quotient is defined as the ratio of the change in function values (f(a+h) - f(a)) to the change in the x-axis (h). It serves two purposes: calculating the average rate of change between two points and determining the slope of the secant line between those points. As h approaches smaller values, the concept of a limit is introduced, which is a method to analyze the behavior of a function as it gets infinitely close to a certain point. The limit of the difference quotient as h approaches zero is defined as the derivative, which is a key concept in understanding the instantaneous rate of change and the slope of a tangent line at a specific point on the graph.
🔍 Instantaneous Rate of Change and Tangent Line Slope
The second paragraph delves deeper into the applications of derivatives by focusing on what happens when the second point on the graph approaches the first point infinitely closely. This situation leads to two significant interpretations of the derivative: the instantaneous rate of change and the slope of the tangent line at a single point on the graph. The instantaneous rate of change is the rate of change at a specific point, which is different from the average rate of change between two points. As the second point coincides with the first, the secant line effectively becomes a tangent line that touches the graph at exactly one point. The derivative in this context provides the slope of this tangent line. The paragraph clarifies the difference between using the difference quotient for average rate of change or secant line slope and using the derivative for instantaneous rate of change or tangent line slope. The video promises to demonstrate how to apply the derivative to find these values in the subsequent video.
Mindmap
Keywords
💡Calculus
💡Derivative
💡Difference quotient
💡Limit
💡Instantaneous rate of change
💡Tangent line
💡Secant line
💡Function value
💡Average rate of change
💡Approaches zero
Highlights
Introduction to the concept of the derivative, one of the most basic concepts of calculus.
Use of the difference quotient, previously learned, to define the derivative.
Explanation of the difference quotient as the difference between function values divided by the difference between points.
Two interpretations of the difference quotient: average rate of change and slope of the secant line.
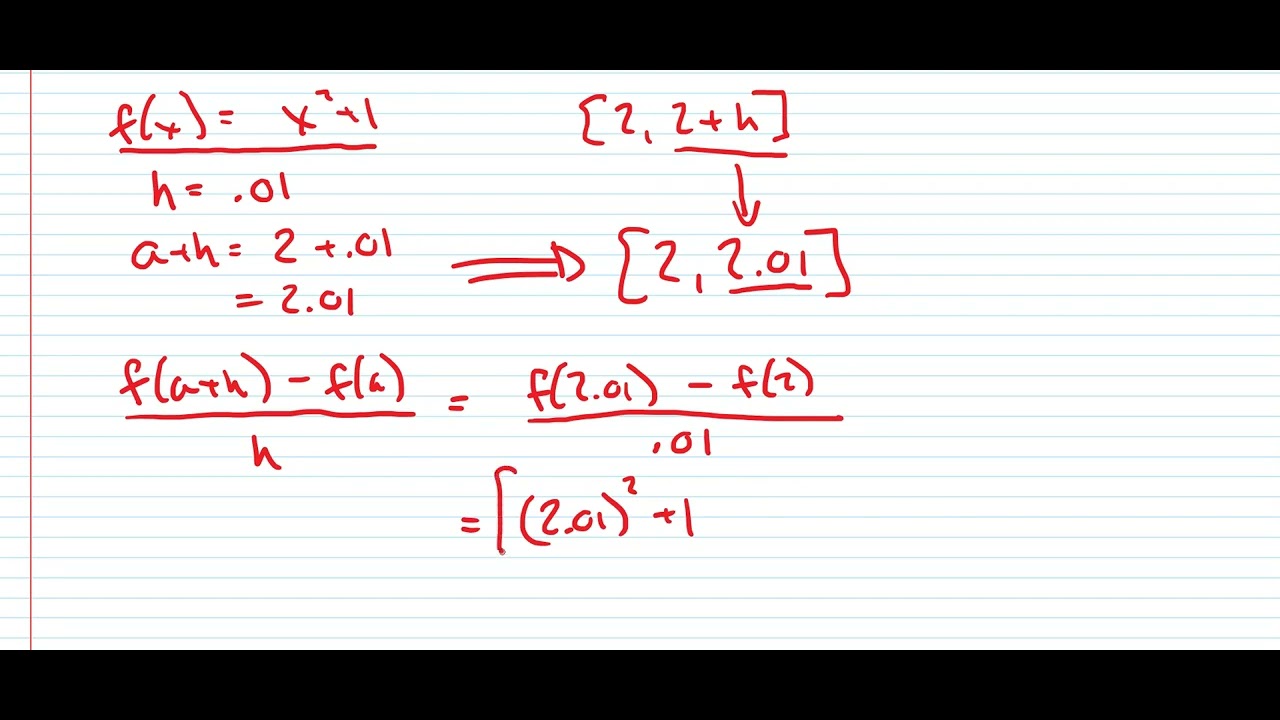
Typically using a small value for h (e.g. 0.1, 0.01, 0.001) to approximate the derivative.
Taking the limit as h approaches zero to define the derivative precisely.
The derivative provides two key interpretations: instantaneous rate of change and slope of the tangent line.
Instantaneous rate of change represents the change at a single point on the graph.
Tangent line touches the graph at just one point, making it distinct from a secant line.
Derivative gives the slope of the tangent line at a specific point.
Difference between using the difference quotient for average rate of change vs. derivative for instantaneous rate.
When to use the difference quotient (average rate, secant line slope) vs. derivative (instantaneous rate, tangent line slope).
Upcoming video will show how to use the derivative to find instantaneous rate of change and slope of a tangent line.
The purpose of introducing the concept of the derivative as the limit of the difference quotient as h approaches zero.
The importance of understanding the concept of limits in calculus.
How the concept of the derivative connects to real-world applications and problem-solving.
The video serves as a foundational introduction to more advanced calculus topics.
Emphasis on the practical applications of derivatives in fields like physics and engineering.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: