Law of Conservation of Charge

Neso Academy
26 Mar 201805:25
EducationalLearning
32 Likes 10 Comments

TLDRThe script discusses the discovery of the amber effect by an ancient Greek philosopher around 1600 BC, where rubbing silk or fur with amber results in electrification. It explains the law of conservation of charge, stating that charge cannot be created or destroyed, only transferred. The mathematical proof using the continuity equation is also mentioned, highlighting the balance between the outflow of charge and the decrease in charge density. The historical development of this law, from its proposal by William Watson and Benjamin Franklin to its proof by Michael Faraday, is briefly outlined.

Takeaways
  • 🌟 The discovery of the amber effect dates back to around 1600 BC by an ancient Greek philosopher, highlighting early understanding of electricity.
  • πŸ’‘ The amber effect occurs when silk or fur rubs against amber, causing a transfer of charge that results in the amber attracting small pieces of thread.
  • πŸ”‹ The term 'electron' originates from the ancient Greeks and is linked to the word for amber, illustrating the historical development of scientific terminology.
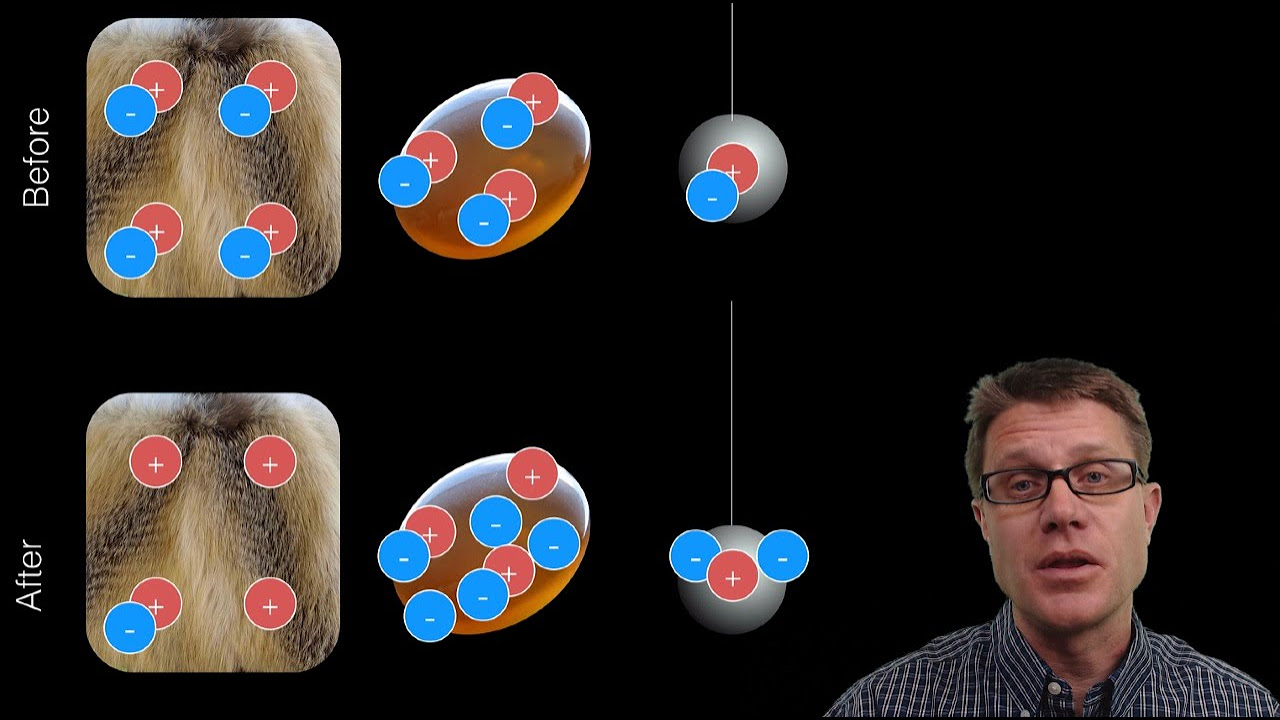
  • πŸ“œ The law of conservation of charge states that charge cannot be created or destroyed, only transferred from one body to another, conserving the total charge in the universe.
  • ♻️ The conservation of charge is reflected in the universe's net charge remaining constant, with positive and negative charges balancing each other out.
  • πŸ“š The mathematical proof of the law of conservation of charge can be found in the electromagnetic theory, specifically through the equation of continuity.
  • 🌊 The divergence operator in the equation of continuity represents the flow of charge through a differential volume, indicating the rate of outflow or inflow of charge.
  • πŸ”„ The left side of the continuity equation (βˆ‡ β€’ J) calculates the rate of outflow of charge, while the right side (- dRho/dt) indicates the rate of decrease in charge density.
  • βš–οΈ The law of conservation of charge is proven by equating the rate of outflow of charge with the rate of decrease in charge density within a differential volume.
  • πŸ† The law was first proposed by William Watson in 1746, and later by Benjamin Franklin in 1747, with Michael Faraday providing the proof in 1843.
  • πŸ“ˆ Understanding the law of conservation of charge and its mathematical proof is crucial for those studying electromagnetic theory and preparing for related interviews or examinations.
Q & A

  • Who is the ancient Greek philosopher mentioned in the script that observed the amber effect?

    -The ancient Greek philosopher mentioned in the script is not specified by name, but the effect was observed around 1600 BC.

  • What is the amber effect?

    -The amber effect refers to the phenomenon where amber, when rubbed with silk or fur, becomes electrically charged and can attract small lightweight objects such as fluff or threads.

  • What is the scientific explanation behind the amber effect?

    -The scientific explanation behind the amber effect is the transfer of electrons between two different materials. When amber and another material like silk or fur are rubbed together, electrons are transferred, leaving the amber with a negative charge and the other material with a positive charge.

  • How did the observation of the amber effect contribute to the understanding of electricity?

    -The observation of the amber effect was one of the earliest known instances of static electricity and laid the foundation for the study of electricity. The term 'electron' was coined by the ancient Greeks, derived from the word for amber, and it signifies the role of electrons in the phenomenon.

  • What is the law of conservation of charge?

    -The law of conservation of charge states that charge can neither be created nor destroyed. It can only be transferred from one body to another, and the total net charge in the universe remains constant.

  • How is the law of conservation of charge mathematically proven?

    -The law of conservation of charge is mathematically proven using the equation of continuity, which is given by βˆ‡ β€’ J = - (d/dt) ρ_V, where βˆ‡ β€’ J represents the divergence of the current density (J), and - (d/dt) ρ_V represents the rate of change of charge density (ρ_V). The equation shows that the outflow of charge from a differential volume is equal to the decrease in charge within that volume, thus conserving the net charge.

  • Who first proposed the law of conservation of charge?

    -The law of conservation of charge was first proposed by William Watson in 1746, and it was later restated by Benjamin Franklin in 1747. The proof was given by Michael Faraday in 1843.

  • What are the units for current density and volume charge density?

    -The unit for current density (J) is amperes per meter square (A/m²), and the unit for volume charge density (ρ_V) is coulombs per meter cube (C/m³).

  • What does the divergence operator (βˆ‡ β€’) represent in the context of the continuity equation?

    -In the context of the continuity equation, the divergence operator (βˆ‡ β€’) represents the flow of a vector field, such as the flow of charge, through a differential volume.

  • What happens when the divergence of the current density (J) is positive?

    -When the divergence of the current density (J) is positive, it indicates that there is a greater than zero flow of charge out of the differential volume.

  • What is the significance of the law of conservation of charge in the study of electromagnetism?

    -The law of conservation of charge is significant in the study of electromagnetism as it is a fundamental principle that governs the behavior of electric fields and currents. It ensures that the total charge in a system remains constant, which is crucial for understanding and solving electromagnetic problems.

Outlines
00:00
πŸ”‹ The Discovery of Electricity and the Law of Conservation of Charge

This paragraph discusses the discovery of electricity dating back to around 1600 BC by an ancient Greek philosopher, who observed that amber, when rubbed with silk or fur, becomes electrified and can attract small lightweight objects. This phenomenon is known as the amber effect. The paragraph further explains the law of conservation of charge, stating that charge cannot be created or destroyed, only transferred from one body to another, and the net quantity of charge in the universe is always conserved. The explanation includes a mathematical proof using the equation of continuity, highlighting the concepts of divergence and charge flow. Historically, the law was first proposed by William Watson in 1746, and the proof was given by Michael Faraday in 1843.

05:02
πŸŽ™οΈ Closing Remarks and Invitation for Questions

The speaker concludes the lecture by inviting the audience to ask any doubts or questions in the comment section. The lecture ends with a sense of closure, accompanied by applause and music, signaling the end of the session and the transition to the next lecture.

Mindmap
Keywords
πŸ’‘Ancient Greek philosopher
The term refers to the scholars from ancient Greece who were engaged in the study of various fields of knowledge, including natural philosophy. In the context of the video, it highlights the historical roots of scientific inquiry and the discovery of the amber effect, which is foundational to the concept of electricity. The mention of the philosopher sets the stage for the discussion on the origins of our understanding of electricity.
πŸ’‘Halley's
Halley's is a misspelling in the script; it should be Thales of Miletus, an ancient Greek philosopher who is credited with the observation that rubbing amber against fur could generate an electric charge. This observation is one of the earliest recorded instances of humans recognizing and documenting the phenomenon of static electricity.
πŸ’‘Amber effect
The amber effect refers to the phenomenon where amber, when rubbed against certain materials like fur or silk, becomes electrically charged and can attract small, light objects. This effect is the earliest known documentation of electricity and is the origin of the word 'electron,' which comes from the Greek word for amber.
πŸ’‘Charge conservation
Charge conservation is a fundamental principle in physics stating that electric charge can neither be created nor destroyed. Instead, it can only be transferred from one object to another, ensuring that the total amount of charge in a closed system remains constant. This concept is central to the understanding of electromagnetic interactions and is a key aspect of the law of conservation of charge.
πŸ’‘Law of conservation of charge
The law of conservation of charge is a fundamental principle in physics that states the total electric charge in an isolated system remains constantβ€”it cannot be created or destroyed, only transferred from one body to another. This law is a cornerstone of the study of electromagnetism and is illustrated in the video through the equation of continuity.
πŸ’‘Equation of continuity
The equation of continuity is a mathematical expression used in electromagnetism that describes the conservation of charge. It is given by βˆ‡ β€’ J = - (dRho/dt), where βˆ‡ β€’ J is the divergence of the current density (J) and dRho/dt is the rate of change of charge density (Rho) with respect to time (t). This equation shows that the rate of outflow of charge from a volume is equal to the rate of decrease of charge within that volume, thereby proving the conservation of charge.
πŸ’‘Divergence
In vector calculus, divergence is a measure of how much a vector field spreads out or diverges from a given point. It is a scalar function that represents the source or sink of a vector field. In the context of the video, the divergence of the current density (J) is used to describe the flow of charge through a small volume, which is essential in proving the law of conservation of charge.
πŸ’‘Current density
Current density, denoted by J, is a physical quantity that describes the amount of electric current per unit area. It is measured in amperes per meter square (A/mΒ²) and is a key component in the study of how electric charges move through conductors. In the video, current density is used to illustrate the flow of charge in relation to the law of conservation of charge.
πŸ’‘Volume charge density
Volume charge density, symbolized by Rho (ρ), is the amount of electric charge per unit volume. It is measured in coulombs per meter cube (C/m³) and is used to describe the distribution of charge within a region of space. In the video, volume charge density is crucial for understanding how the law of conservation of charge is reflected in the rate of change of charge within a given volume.
πŸ’‘William Watson
William Watson was an 18th-century English scientist who, along with Benjamin Franklin and Michael Faraday, contributed to the development of the law of conservation of charge. Watson first proposed the law in 1746, and it was later restated by Franklin and proven by Faraday.
πŸ’‘Benjamin Franklin
Benjamin Franklin was a polymath, one of the Founding Fathers of the United States, and a prominent scientist of his time. He is known for his experiments with electricity, including his famous kite experiment, and for his role in restating the law of conservation of charge in 1747, which furthered the understanding of electrical phenomena.
πŸ’‘Michael Faraday
Michael Faraday was an English scientist known for his significant contributions to the field of electromagnetism and electrochemistry. Faraday provided the experimental proof for the law of conservation of charge in 1843, solidifying the principle's place in physics.
Highlights

Ancient Greek philosopher Halley's discovered the amber effect around 1600 BC.

The amber effect is the electrification of amber when rubbed with silk or fur.

The term 'electron' comes from the Greek word for amber, due to its ability to attract small objects when electrified.

The law of conservation of charge states that charge can neither be created nor destroyed.

Charge can only be transferred from one body to another, maintaining a constant net charge in the universe.

The equation of continuity is used to mathematically prove the law of conservation of charge.

Nabla dot J represents the divergence of the current density J, indicating the flow of charge.

The left side of the continuity equation represents the rate of outflow of current or charge.

The right side of the continuity equation represents the rate of decrease of charge density.

The equality of the left and right sides of the continuity equation demonstrates charge conservation.

The law of conservation of charge was first proposed by William Watson in 1746.

Benjamin Franklin proposed the law again in 1747.

Michael Faraday provided the proof for the law in 1843.

Understanding the law of conservation of charge is crucial for various scientific and engineering applications.

The lecture emphasizes the importance of remembering the statement that charge cannot be created or destroyed.

The method of proving the law of conservation of charge using the continuity equation is highlighted.

The historical development of the law of conservation of charge is discussed, from its proposal to its proof.

The lecture concludes with an invitation for questions and applause, indicating an interactive and engaging presentation.

Transcripts

Browse More Related Video

Electric Charge

What is Electric Charge? (Electrodynamics)

4. Gauss's Law and Application to Conductors and Insulators

Conservation of Electric Charge

Gauss Law Problems, Cylindrical Conductor, Linear & Surface Charge Denisty, Electric Field & Flux,

Physics 37.1 Gauss's Law Understood (4 of 29) Gauss' Law Clarified

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ElectricityHistoryAmberEffectConservationLawPhysicsPrinciplesAncientGreeksElectromagnetismContinuityEquationScientificDiscoveriesEducationalContentLectureSummary