Gauss Law Problems, Cylindrical Conductor, Linear & Surface Charge Denisty, Electric Field & Flux,

The Organic Chemistry Tutor

12 Jan 201713:21

EducationalLearning

32 Likes 10 Comments

TLDRThis video script explores the concept of electric fields and charge distribution around an infinitely long cylindrical conductor with a given surface charge density. It calculates the total charge enclosed by a Gaussian cylinder using the surface charge density and the lateral area formula. Further, it utilizes Gauss's law to determine the electric flux through the cylinder and derives the electric field at a specific distance from the conductor. The script concludes by calculating the linear charge density and its relation to the electric field, providing a foundational understanding of electrostatics.

Takeaways

🌟 The problem involves calculating the total charge and electric properties of a system consisting of an infinitely long cylindrical conductor and a Gaussian cylinder.
🔍 The cylindrical conductor has a uniform surface charge density and is surrounded by a larger Gaussian cylinder used for calculations.
📏 The conductor's radius is given as 30 centimeters, and the Gaussian cylinder has a radius of 1.5 meters and a length of 2 meters.
🔋 The surface charge density (sigma) of the conductor is 15 microcoulombs per square meter.
⚡ The electric field inside a metal conductor is zero because all the charge resides on the surface.
📘 The total charge enclosed by the Gaussian cylinder is calculated using the formula: Charge (Q) = sigma * lateral surface area of the conductor * length of the Gaussian cylinder.
🧲 The lateral surface area of the conductor is 2 * pi * radius * length, which is used in the calculation of the enclosed charge.
📊 The electric flux through the Gaussian cylinder is found using Gauss's law, relating it to the enclosed charge and the permittivity of free space (epsilon naught).
🌐 The electric field at a distance from the conductor is derived from the relationship between charge density, the conductor's radius, and the distance from the conductor.
📐 The linear charge density (lambda) of the conductor is determined by the surface charge density and the conductor's circumference.
🔗 The electric field outside the conductor can be expressed in terms of the linear charge density, resembling the electric field formula for a line of charge.

Q & A

What is the significance of the surface charge density in the context of the problem?
-The surface charge density is crucial as it determines the amount of charge per unit area on the surface of the cylindrical conductor. It is used to calculate the total charge enclosed by the Gaussian cylinder and plays a key role in applying Gauss's law.
Why is the electric field inside a metal conductor zero?
-The electric field inside a metal conductor is zero because the charges in a metal are free to move and will distribute themselves on the surface, creating an internal field configuration that cancels out any internal electric field.
What is the formula for calculating the total charge enclosed by the Gaussian cylinder?
-The total charge enclosed by the Gaussian cylinder is calculated using the formula: Q = σ × 2πR × L, where σ is the surface charge density, R is the radius of the cylindrical conductor, and L is the length of the Gaussian cylinder.
How is the electric flux related to the enclosed charge according to Gauss's law?
-According to Gauss's law, the electric flux through a closed surface is equal to the total charge enclosed by that surface divided by the permittivity of free space, ε₀. The formula is Φ = Q / ε₀.
What is the unit of electric flux?
-The unit of electric flux is newtons times square meters per coulomb (N·m²/C), which is the product of the unit of electric field (newtons per coulomb, N/C) and the unit of area (square meters, m²).
How do you derive the equation for the electric field due to a cylindrical conductor?
-The equation for the electric field due to a cylindrical conductor is derived by equating the electric flux through the Gaussian cylinder to the product of the electric field and the area of the cylinder, and then solving for the electric field in terms of the surface charge density, the radius of the conductor, and the permittivity of free space.
What is the formula for the electric field at a distance from a cylindrical conductor?
-The formula for the electric field at a distance r from a cylindrical conductor with surface charge density σ is E = σ × R / 2π ε₀ × r, where R is the radius of the conductor and ε₀ is the permittivity of free space.
What is the linear charge density of the cylindrical conductor?
-The linear charge density (lambda, λ) of the cylindrical conductor is the charge per unit length and is given by λ = σ × 2πR, where σ is the surface charge density and R is the radius of the conductor.
How can you express the electric field in terms of the linear charge density?
-The electric field can be expressed in terms of the linear charge density as E = λ / 2π ε₀ × r, where λ is the linear charge density, ε₀ is the permittivity of free space, and r is the distance from the conductor.
What is the relationship between the electric field of a cylindrical conductor and that of a line of charge?
-When observing the electric field of a cylindrical conductor from a distance much greater than its radius, the field behaves similarly to that of a line of charge. The electric field formula for a line of charge is E = λ / 2π ε₀ × r, which is the same as the formula for the electric field of a cylindrical conductor when considering large distances.

Outlines

00:00

🔋 Calculating Total Charge Enclosed by a Gaussian Cylinder

The first paragraph introduces the problem of calculating the total charge enclosed by a Gaussian cylinder around an infinitely long cylindrical conductor with a given radius and surface charge density. The conductor has a positive charge uniformly distributed on its surface, and the electric field inside a metal conductor is zero due to the charge being on the surface. The calculation involves using the surface charge density (sigma), the radius of the conductor, and the length of the Gaussian cylinder to find the total charge (Q) using the formula Q = sigma * lateral area of the conductor * length of the Gaussian cylinder. The lateral area is 2 * pi * r * h, where r is the radius and h is the height (or length in this case). The example uses specific values to compute the total charge as 5.65 * 10^-5 Coulombs.

05:00

🌀 Determining Electric Flux Using Gauss's Law

The second paragraph explains how to find the electric flux through the Gaussian cylinder using Gauss's law. The electric flux is calculated by dividing the total charge enclosed by the permittivity of free space (epsilon sub naught). The example provided calculates the electric flux as 6.38 * 10^6 N*m^2/C, which represents the electric field times the area through which it passes. The units for electric field are Newtons per Coulomb, and the area is in square meters, leading to the final units for electric flux.

10:00

📚 Deriving the Electric Field Equation for a Cylindrical Conductor

The third paragraph delves into deriving the equation for the electric field at a point 1.5 meters away from the cylindrical conductor. It starts with Gauss's law, equating the electric flux to the total charge divided by epsilon sub naught, and then relates this to the surface charge density and the lateral area of the conductor. The electric field is then expressed in terms of the surface charge density, the radius of the conductor, and the radius of the Gaussian cylinder. The example shows the calculation of the electric field outside the conductor as 300,389.83 N/C, assuming the Gaussian cylinder's radius is larger than the conductor's radius.

🌐 Calculating Linear Charge Density and Electric Field Relation

The final paragraph discusses how to determine the linear charge density (lambda) of the cylindrical conductor. It explains that lambda is the charge per unit length and can be found by dividing the surface charge density by the circumference of the conductor. The paragraph also relates the linear charge density to the electric field, showing that the electric field can be calculated using lambda, the permittivity of free space, and the radius of the Gaussian cylinder. The example calculates the linear charge density as approximately 0.83 * 10^-5 C/m and then uses it to derive the formula for the electric field in terms of lambda.

Mindmap

Keywords

💡Cylindrical Conductor

A cylindrical conductor is a tube-shaped object that conducts electricity. In the video, it is described as infinitely long with a radius of 30 centimeters, and it carries a uniform positive surface charge. The concept is central to the video as it is the source of the electric field and charge distribution being analyzed.

💡Surface Charge Density

Surface charge density, denoted as sigma in the script, is a measure of the amount of electric charge per unit area on the surface of a conductor. It is given as 15 microcoulombs per square meter in the video and is essential for calculating the total charge enclosed by the Gaussian cylinder.

💡Gaussian Cylinder

A Gaussian cylinder is an imaginary cylindrical surface used in the application of Gauss's law to calculate electric fields. In the video, it has a radius of 1.5 meters and a length of 2 meters, and it is used to determine the total charge enclosed and the electric flux through its surface.

💡Electric Field

The electric field is a vector field that describes the force experienced by a unit charge in the vicinity of other charges. The script discusses how the electric field emanates from the positive charge on the cylindrical conductor and is perpendicular to the lateral area of the Gaussian cylinder.

💡Lateral Area

The lateral area of a cylinder refers to the surface area excluding the top and bottom circles. In the context of the video, the lateral area is used to calculate the total charge enclosed by the Gaussian cylinder, as it represents the path the electric field lines take.

💡Gauss's Law

Gauss's law is a fundamental principle in electromagnetism that relates the electric flux through a closed surface to the total charge enclosed by that surface. The script uses Gauss's law to find the electric flux through the Gaussian cylinder and to derive the electric field around the cylindrical conductor.

💡Electric Flux

Electric flux is a measure of the electric field passing through a given surface and is calculated using Gauss's law. The script calculates the electric flux through the Gaussian cylinder to understand the effect of the enclosed charge on the electric field distribution.

💡Linear Charge Density

Linear charge density, represented by lambda in the script, is the charge per unit length along a conductor. The video derives this concept from the surface charge density and uses it to express the electric field in terms of the linear charge density of the cylindrical conductor.

💡Epsilon Sub Naught (ε₀)

Epsilon sub naught, also known as the vacuum permittivity, is a physical constant related to the electric field created in a vacuum by a unit charge. In the script, it is used in the denominator when applying Gauss's law to find the electric field and flux.

💡Perpendicular

In the context of the video, perpendicular refers to the direction of the electric field lines being at a right angle to the surface of the Gaussian cylinder. This orientation is crucial for the calculation of the electric flux through the cylinder's lateral surface.

💡Metal Conductor

A metal conductor, as mentioned in the script, is a material that allows the free movement of electrons and thus the flow of electric current. The script explains that within a metal conductor, the electric field is zero because all the charge resides on the surface.

Highlights

An infinitely long cylindrical conductor with a radius of 30 centimeters has a surface charge density of 15 microcoulombs per square meter.

A Gaussian cylinder of radius 1.5 meters and length 2 meters is used to calculate the total charge enclosed.

Positive charge is uniformly distributed on the surface of the conductor, with the electric field inside the metal conductor being zero.

The electric field emanates perpendicularly from the charge, affecting the lateral area of the Gaussian cylinder.

The total charge enclosed by the Gaussian cylinder is calculated using the surface charge density and the lateral area of the conductor.

The formula for calculating the total charge is derived, involving the radius of the conductor and the length of the Gaussian cylinder.

The calculation results in a total charge of 5.65 × 10^-5 coulombs enclosed by the Gaussian cylinder.

Gauss's law is applied to find the electric flux passing through the Gaussian cylinder.

The electric flux is calculated as 6.38 × 10^6 newtons times square meters per coulomb.

The equation for the electric field 1.5 meters away from the conductor is derived using Gauss's law.

The electric field outside the conductor is calculated to be 300,389.83 newtons per coulomb.

The linear charge density of the cylindrical conductor is determined to be approximately 0.83 × 10^-5 coulombs per meter.

The relationship between the electric field and the linear charge density is established for a cylindrical conductor.

The electric field formula is simplified using the linear charge density, showing its similarity to the electric field of a line of charge.

The practical application of treating the cylindrical conductor as a line of charge for calculations at a distance is discussed.

Transcripts

Browse More Related Video

4. Gauss's Law and Application to Conductors and Insulators

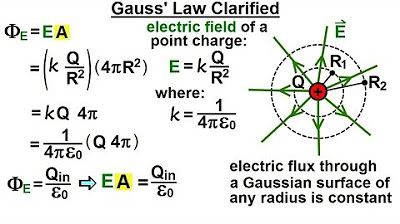
Physics 37.1 Gauss's Law Understood (4 of 29) Gauss' Law Clarified

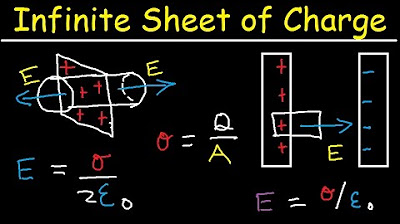
Gauss Law Problems - Infinite Sheet of Charge and Parallel Plate Capacitor - Physics

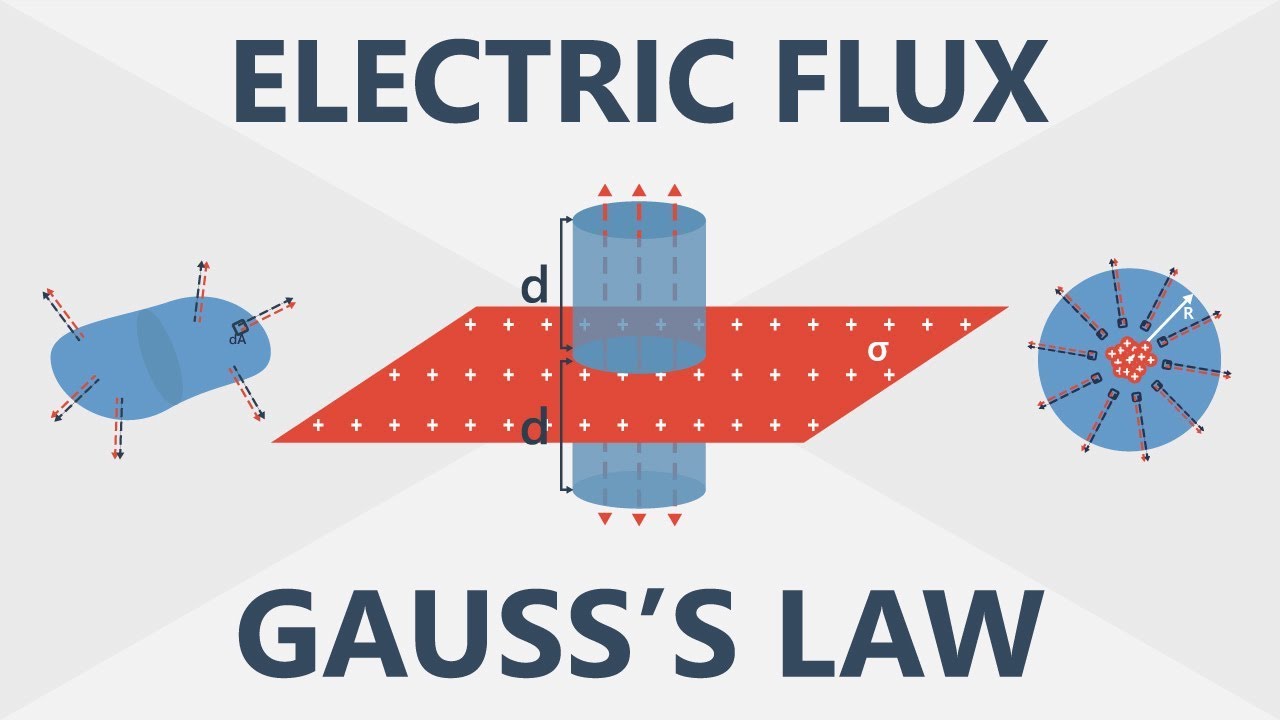
Electric Flux and Gauss’s Law | Electronics Basics #6

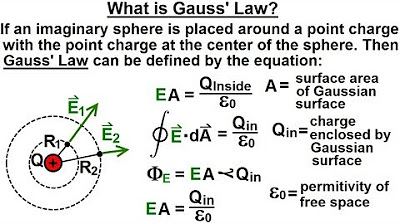
Physics 37.1 Gauss's Law Understood (3 of 29) What is Gauss' Law?

Gauss law of electricity | Electrostatics | Physics | Khan Academy