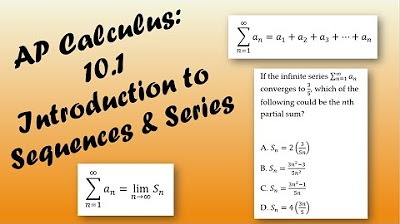
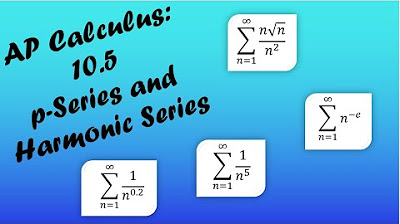
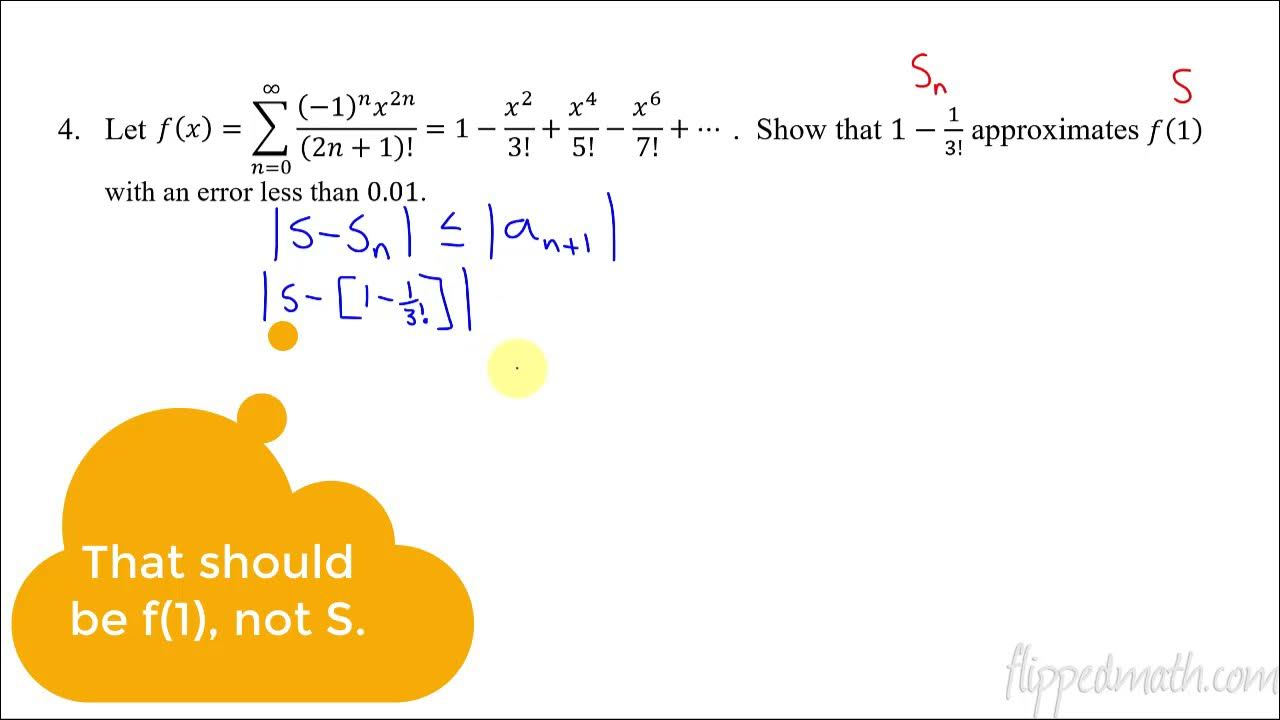
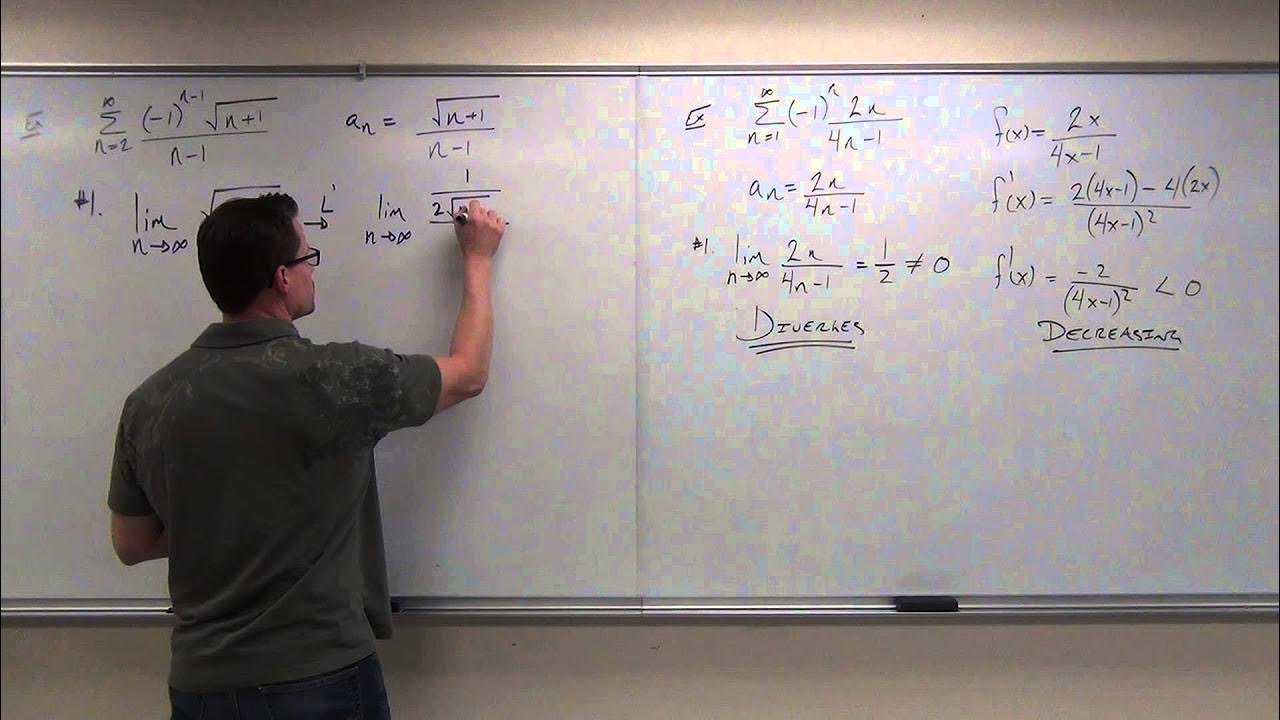700 years of secrets of the Sum of Sums (paradoxical harmonic series)

The video begins with an introduction to the harmonic series, a famous infinite series in mathematics that has been studied for centuries. The speaker aims to discuss some of its lesser-known paradoxical properties, particularly recent discoveries made in the past two months. The video is structured into six chapters, each with its own highlight, and viewers are invited to vote on their favorite at the end. To start, the speaker presents a thought experiment involving a balance scale with weights to illustrate the concept of leverage and to set the stage for discussing the harmonic series.

This paragraph delves into a real-world puzzle involving stacking blocks to create a leaning tower with the maximum possible overhang. The speaker explains how adding and rearranging blocks can incrementally increase the overhang, leading to a discussion on the harmonic series and its relation to the sum of reciprocals of integers. The 'Leaning Tower of Lire' is introduced as a stack that achieves a remarkable overhang with 20 blocks, and the speaker challenges viewers to experiment with this setup themselves. The paragraph also touches on the concept of optimal stacks and introduces the idea of counterweights and bridging weights in achieving maximal overhang.

The speaker continues the discussion on the leaning tower of lire, explaining the complexity of building such a stack due to the need for counterweights and bridging pieces. It's revealed that the leaning tower is not the only configuration to achieve a maximal overhang with one brick per layer, and the speaker alludes to another stack with the same properties. The paragraph also explores the concept of an unlimited supply of blocks and how this relates to the sum of the harmonic series, which is shown to diverge to infinity. A historical note on the proof of the harmonic series' divergence by Nicole Oresme is included, along with a discussion on the implications for the leaning tower paradox.

The speaker discusses the slow divergence of the harmonic series, using visual aids to demonstrate how the series grows and how many terms are needed to reach a partial sum of 100. The calculation of this number by John Wrench Jr. in 1968 is highlighted as a significant achievement. The speaker then explores the idea of finding a formula for the n-th partial sum of the harmonic series, noting that the usual methods for identifying patterns do not apply in this case. The concept of odd-over-even fractions and their non-integer properties are introduced, leading to the conclusion that all partial sums of the harmonic series, except the first, are not integers.

The speaker introduces the Euler-Mascheroni constant, gamma, and its relationship with the harmonic series. A challenge question about representing numbers as sums of reciprocals of distinct positive integers is posed, followed by a discussion on the function 1/x and its visual representation. The natural logarithm's role in approximating the partial sums of the harmonic series is explained, and a formula relating the n-th partial sum to the natural logarithm of n plus one is presented. The speaker also discusses how this formula can be improved by incorporating gamma, resulting in a highly accurate approximation for large n values.

This paragraph focuses on the remarkable formula for the n-th partial sum of the harmonic series, which includes the Euler-Mascheroni constant. The speaker demonstrates the increasing accuracy of this formula as n grows larger and uses it to address the challenge questions posed earlier about the number of terms needed to exceed a sum of 100 and the overhang of a leaning tower with a large number of blocks. The speaker also discusses the concept of sub-series within the harmonic series, noting that every positive number can be represented as a sum of sub-series of the harmonic series. The paragraph concludes with a teaser about the connection between gamma and other mathematical constants.

The final paragraph discusses the harmonic series as a 'sum of sums,' highlighting its inclusion of many important infinite series such as the geometric series and Euler's sum of reciprocals of squares. The speaker explores the idea that every number is a sum of sub-series within the harmonic series and provides a method for finding such sub-series using a greedy algorithm. The paragraph also touches on divergent sub-series within the harmonic series, such as the sum of reciprocals of even, odd, and prime numbers. The speaker then presents a trickier question about the sum of reciprocals of primes, confirming its infinity. The video concludes with a discussion on Kempner's series, which omits certain digits, and the recent discoveries and approximations of sums for related series by Robert Baillie.

The speaker concludes the video with an exploration of Kempner's series, focusing on the surprising result that the sum of the series without the digit 9 is finite, despite the series being very sparse. The speaker shares a personal anecdote about a university homework assignment related to this series and introduces a visual representation, suggested by Tristan, to illustrate the convergence of the series. The video ends with an animated algebraic proof that demonstrates the finiteness of Kempner's series, showing that the sum is less than 80. The speaker reflects on the excitement of discovering new insights into the harmonic series and encourages viewers to participate in a vote to win a book.