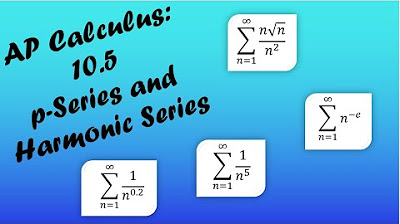
Proof: harmonic series diverges | Series | AP Calculus BC | Khan Academy
TLDRThis video discusses Nicole Oresme, a medieval French philosopher and mathematician known for proving the divergence of the harmonic series. The proof involves constructing a new series with terms less than or equal to those in the harmonic series, demonstrating its divergence by the comparison test. The elegant proof shows that if a smaller series diverges, the original harmonic series must also diverge.
Takeaways
- 🎨 The video discusses a painting of Nicole Oresme, a renowned French philosopher and mathematician from the 1300s.
- 📚 Nicole Oresme is famous for his proof that the harmonic series diverges, which was a significant contribution to mathematics.
- 🔍 The harmonic series is a sequence where each term is the reciprocal of a natural number, starting with 1.
- 🤔 Initially, it's not clear whether the harmonic series converges or diverges due to the terms approaching zero but remaining positive.
- 📉 Oresme's proof involved constructing a new series where each term is less than or equal to the corresponding term in the harmonic series.
- 📝 He replaced each term in the harmonic series with the largest power of 1/2 that is less than or equal to that term.
- 🔑 The constructed series was shown to diverge by demonstrating that it is a sum of fractions that each add up to 1/2, repeated indefinitely.
- 📈 By proving the divergence of the constructed series, Oresme applied the comparison test to conclude that the harmonic series must also diverge.
- 📚 The comparison test is a method in calculus used to determine the convergence of series by comparing them to another series with known behavior.
- 📉 The video script provides a step-by-step explanation of how Oresme's proof works, making it accessible to viewers.
- 🧠 The proof is considered one of the most elegant in mathematics, showcasing Oresme's deep understanding of the harmonic series.
Q & A
Who is Nicole Oresme and what is he famous for?
-Nicole Oresme was a famous French philosopher and mathematician who lived in medieval France during the 1300s. He is renowned for his proof that the harmonic series diverges.
What is the harmonic series in mathematics?
-The harmonic series is the infinite series 1 + 1/2 + 1/3 + 1/4 + 1/5 + ..., where each term is the reciprocal of a natural number.
What does it mean for a series to converge or diverge?
-A series converges if the sum of its terms approaches a finite limit as the number of terms goes to infinity. A series diverges if it does not converge, meaning the sum grows without bound.
How did Nicole Oresme prove that the harmonic series diverges?
-Oresme proved the divergence of the harmonic series by constructing a new series where each term is less than or equal to the corresponding term in the harmonic series, and then showing that this new series diverges.
Can you explain the comparison test mentioned in the script?
-The comparison test is a method used to determine the convergence of an infinite series. If 0 ≤ a_n ≤ b_n for all n, and the series ∑b_n diverges, then the series ∑a_n also diverges.
What is the significance of the largest power of 1/2 less than or equal to a term in the harmonic series?
-Oresme used the largest power of 1/2 less than or equal to each term in the harmonic series to construct a new series with terms that are smaller but still diverge, thus proving the divergence of the original harmonic series.
How did Oresme construct the new series with terms less than or equal to the harmonic series?
-Oresme replaced each term in the harmonic series with the largest power of 1/2 that is less than or equal to that term, such as replacing 1/3 with 1/4, 1/5 with 1/8, and so on.
Why does the constructed series of largest powers of 1/2 also diverge?
-The constructed series diverges because it essentially becomes a series of 1/2's being added repeatedly, which is equivalent to adding 1/2 an infinite number of times, leading to an unbounded sum.
What is the conclusion of Oresme's proof regarding the harmonic series?
-The conclusion of Oresme's proof is that since the constructed series, with terms smaller than the harmonic series, diverges, the harmonic series itself must also diverge.
Why is Oresme's proof considered elegant in mathematics?
-Oresme's proof is considered elegant because it uses a simple and clever comparison method to prove a fundamental property of the harmonic series without complex calculations.
What is the importance of understanding divergence or convergence of series in mathematics?
-Understanding the divergence or convergence of series is important in mathematics as it helps in analyzing the behavior of infinite sums, which are fundamental in various fields such as calculus, probability, and mathematical physics.
Outlines
🎨 Nicole Oresme's Proof of Harmonic Series Divergence
The script introduces Nicole Oresme, a medieval French philosopher and mathematician renowned for his proof that the harmonic series diverges. The harmonic series is a sequence of positive terms, each approaching zero, which initially appears to converge but is proven to diverge by Oresme. His method involves constructing a new series where each term is the largest power of 1/2 less than or equal to the corresponding term in the harmonic series. This comparison series is shown to diverge by summing up to a series of 1/2 increments, thereby proving the divergence of the harmonic series through the comparison test.
📚 Demonstrating the Divergence of the Harmonic Series
This paragraph delves deeper into the proof of the harmonic series' divergence. It explains the step-by-step construction of the comparison series, where each term in the harmonic series is replaced with a smaller term, specifically the largest power of 1/2 that is less than or equal to the original term. The script illustrates this by replacing fractions like 1/3 with 1/4, and 1/5 with 1/8, and so on. The constructed series is then summed, revealing a pattern of adding up to 1/2 repeatedly. This pattern indicates that the series is unbounded and diverges to infinity, which by the comparison test, confirms the divergence of the original harmonic series.
Mindmap
Keywords
💡Nicole Oresme
💡Phonetic spelling
💡Harmonic series
💡Divergence
💡Comparison test
💡Powers of 1/2
💡Non-negative terms
💡Infinite sum
💡Unbounded
💡Elegant proofs
Highlights
Introduction to Nicole Oresme, a famous French philosopher and mathematician from the 1300s.
Nicole Oresme is known for his proof that the harmonic series diverges.
Review of the harmonic series: 1 + 1/2 + 1/3 + 1/4 + 1/5 and the uncertainty of its convergence or divergence.
Oresme's elegant proof method by replacing terms in the harmonic series with smaller equivalents.
Explanation of constructing a new series by replacing each term with the largest power of 1/2 less than or equal to that term.
Replacing 1/3 with 1/4 as an example of the construction process.
The largest power of 1/2 less than or equal to 1/5 is 1/8, illustrating the replacement process.
Continuing the pattern to replace fractions with corresponding powers of 1/2.
Verification of the comparison test's applicability to the constructed series.
Observation that each term in the new series is smaller than the corresponding term in the harmonic series.
The constructed series S is shown to be an infinite sum of 1/2, indicating divergence.
Demonstration that if S diverges, then the harmonic series must also diverge by the comparison test.
The constructed series S is unbounded and approaches infinity, implying the divergence of the harmonic series.
The impossibility of the harmonic series converging given the divergence of the constructed series with smaller terms.
The interesting nature of the proof and its impact on understanding series convergence.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: