AP Calculus BC Lesson 10.5

Elizabeth Fein

8 Jan 202312:34

EducationalLearning

32 Likes 10 Comments

TLDRThis video script delves into the concepts of p-series and harmonic series, explaining their convergence and divergence based on the value of p. It clarifies that a p-series converges if p > 1 and diverges if p ≤ 1, using examples to illustrate the integral and series tests. The script also distinguishes between p-series and harmonic series, highlighting the divergence of the latter despite p being equal to 1. Additionally, it addresses the convergence of geometric series and applies the nth term and integral tests to various series, providing a comprehensive understanding of infinite series behavior.

Takeaways

📚 The lesson focuses on P-series and harmonic series, which are specific types of infinite series.
🔢 A P-series is an infinite series of the form 1/n^P, where P is a positive number.
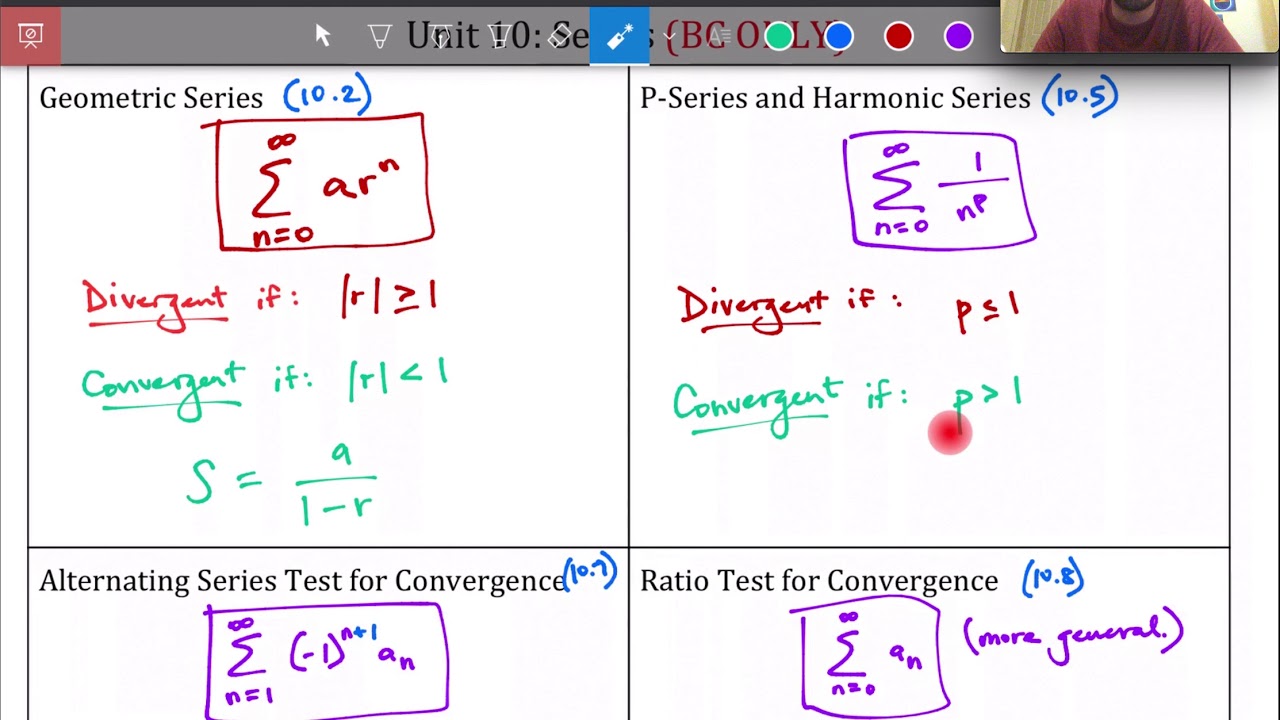
📈 The convergence or divergence of a P-series is determined by the value of P: it converges if P > 1 and diverges if P ≤ 1, based on the integral test.
🌐 The integral test implies that the behavior of a P-series is analogous to that of the corresponding improper integral.
🌰 Examples are provided to illustrate the convergence or divergence of series with different values of P, such as 1/n^5 (converges) and 1/n^0.2 (diverges).
🔍 The concept of a harmonic series is introduced as a special case of a P-series where P = 1, and it is shown to diverge.
📊 The script includes a detailed analysis of various series, applying the nth term test, geometric series rules, and the integral test to determine convergence.
🤔 The nth term test is inconclusive for certain series, requiring additional tests like the integral test for a definitive conclusion.
📂 The integral test is used to compare the series to an integral that converges, thus confirming the convergence of the series if the integral converges.
🔑 The values of P that allow for the convergence of both the P-series and the harmonic series are explored, with the conclusion that P must be greater than 1/2 but less than 4.
📝 The lesson concludes with a clear set of conditions for P that ensure the convergence of the given series.

Q & A

What is a P-series in the context of infinite series?
-A P-series is a specific type of infinite series with the format Σ from n=1 to Infinity of 1/(n^p), where p is a positive number.
How does the Integral Test relate to the convergence of a P-series?
-The Integral Test states that an integral and a series will converge or diverge together. Since the integral of 1/x^p converges for p > 1 and diverges for p ≤ 1, the same behavior applies to the P-series.
What is the condition for a P-series to converge?
-A P-series converges if p, the exponent, is greater than 1. If p is less than or equal to 1, the series diverges.
What is a harmonic series and how does it behave?
-A harmonic series is a special type of P-series where p equals 1. It diverges because, according to the convergence condition, a series diverges when p is less than or equal to 1.
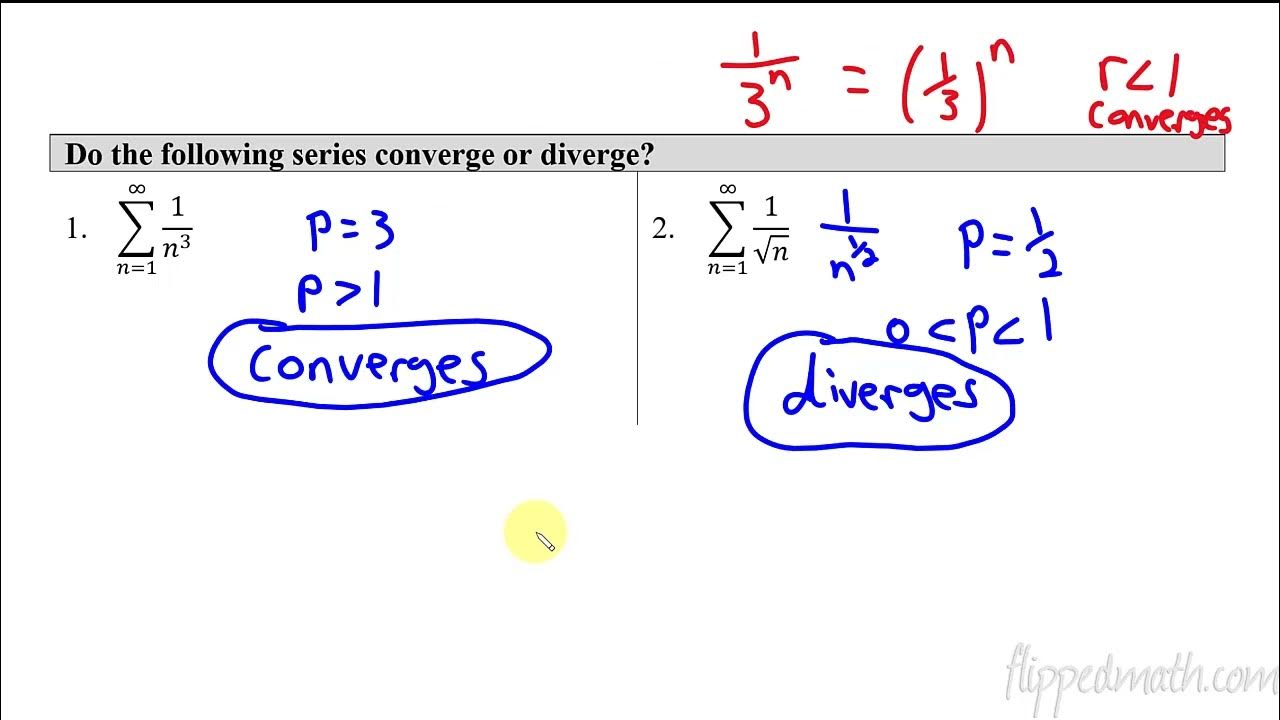
How can you determine the convergence or divergence of the series Σ from n=1 to Infinity of 1/n^5?
-Since p equals 5 in this series, which is greater than 1, the series converges according to the P-series convergence condition.
What is the behavior of the series Σ from n=1 to Infinity of 1/n^0.2?
-The series diverges because p equals 0.2, which is less than or equal to 1, indicating divergence based on the P-series convergence rule.
What happens with the series Σ from n=1 to Infinity of 1/(n^(1/3))?
-This series diverges because p equals 1/3, which is less than or equal to 1, leading to divergence according to the P-series convergence criteria.
How does the nth term test work for determining the convergence of a series?
-The nth term test checks if the limit of the nth term of the series approaches Infinity as n also approaches Infinity. If the limit is zero, the test is inconclusive; it does not confirm convergence or divergence.
What is the condition for the convergence of the geometric series Σ from n=1 to Infinity of (e^n)/(2^n)?
-The geometric series converges if the absolute value of the common ratio r is less than 1. In this case, r is e/2, and since e is approximately 2.7, the series converges because e/2 is less than 1.
How can you determine if the series Σ from n=1 to Infinity of n^(-e) converges?
-Since e is approximately 2.7, the series can be rewritten as 1/n^e. The series converges because e is greater than 1, ensuring that the terms of the series approach zero as n increases.
What values of P make both the series Σ from n=1 to Infinity of P^n/(4^n) and the series Σ from n=1 to Infinity of 1/(n^(2P))) converge?
-For both series to converge, P must be greater than 1/2 but less than 4. This is because the exponent 2P in the first series must be greater than 1 for convergence, and for the second series, P must be less than 4 to ensure the absolute value of the term 1/(4^n) is less than 1.

Outlines

00:00

📚 Introduction to P-Series and Harmonic Series

This paragraph introduces the concept of P-series and harmonic series in the context of infinite series. A P-series is defined as an infinite series of the form ∑ from n=1 to ∞ of 1/n^p, where p is a positive number. The convergence or divergence of these series is linked to the value of p, with the series converging if p > 1 and diverging if p ≤ 1. This is based on the integral test, which was discussed in a previous lesson. The paragraph provides examples to illustrate the convergence and divergence of P-series based on the value of p, such as a series with p=5 (convergent) and p=0.2 (divergent). It also introduces the concept of a harmonic series, which is a special case of a P-series with p=1 and is known to diverge.

05:02

🔍 Analyzing Convergence and Divergence of Infinite Series

This paragraph delves into the analysis of whether various infinite series converge or diverge. It discusses the convergence of a series with a cubic term (1/n^3) and the divergence of the harmonic series (1/n). The paragraph also addresses a series with a negative exponent (1/n^(-negative e)) and explains that it converges because the exponent e is greater than 1. The discussion continues with the identification of a convergent geometric series (1/3^n) and the application of the nth term test and integral test to determine the convergence of other series, such as 1/(n+2)^2 and e^n/(2^n). The paragraph concludes with the determination that only the first series (1/(n+2)^2) converges.

10:03

🔢 Finding Values of P for Convergent Series

The focus of this paragraph is on identifying the values of P for which two given series converge. The first series is a P-series of the form P^n/(4^n * (n+1)^p), and the second is a harmonic series modified by a factor of 1/(n^2 * P). To ensure convergence for the P-series, the exponent 2p must be greater than 1, leading to the conclusion that P must be greater than 0.5. For the harmonic series to converge, the value of P must be between -4 and 4, but since P must also be greater than 0.5, the final condition is that P must be between 0.5 and 4. The paragraph concludes that the correct answer is Choice A, which states P is between 0.5 and 4.

Mindmap

Keywords

💡P series

A P series, or power series, is an infinite series of the form 1/n^p where n starts from 1 and goes to infinity, and p is a positive number. The convergence or divergence of a P series is determined by the value of p. If p is greater than 1, the series converges; if p is less than or equal to 1, the series diverges. This concept is central to the video, as it is used to analyze various infinite series and determine their convergence or divergence.

💡Harmonic series

A harmonic series is a specific type of P series where p equals 1. The general form is the sum from n equals 1 to infinity of 1/n. It is known that a harmonic series diverges, meaning it does not approach a finite value as the number of terms increases. This is a key concept in the video, used to illustrate the divergence of a particular type of series.

💡Convergence

In mathematics, convergence refers to the property of a sequence or series to approach a certain value as the number of terms increases indefinitely. A series is said to converge if the sequence of its partial sums approaches a finite limit. In the context of the video, determining whether a series converges or diverges is a primary objective.

💡Divergence

Divergence, in the context of mathematical series, indicates that the series does not approach a finite value as the number of terms increases. A series is said to diverge if the sequence of its partial sums does not have a limit or if the limit is infinite. The video uses this concept to analyze and classify different types of series.

💡Integral test

The integral test is a method used to determine the convergence or divergence of an infinite series by comparing it to an improper integral. If the integral converges, so does the series, and if the integral diverges, the series also diverges. The test is based on the fact that the integral and the series should behave similarly, as suggested by the integral test covered in the video.

💡Improper integrals

Improper integrals are a type of integral that have one or more infinite limits or involve functions that are unbounded at one or more points within the interval of integration. The concept is important in the video because it is used to prove the convergence or divergence of certain series, particularly P series, through comparison with improper integrals.

💡Geometric series

A geometric series is an infinite series where each term is a constant ratio multiplied by the previous term. The general form is a_n = ar^(n-1), where a is the first term and r is the common ratio. The series converges if the absolute value of r is less than 1. This concept is used in the video to analyze series where each term is a power of a constant, such as 1/n^3.

💡Nth term test

The nth term test is a method to determine the convergence or divergence of an infinite series by examining the limit of the sequence of the terms as n approaches infinity. If the limit is zero, the series may converge; if the limit is a non-zero value, the series diverges. However, if the limit is zero, the test is inconclusive and another method must be used to determine convergence.

💡Limit

In mathematics, a limit is the value that a function or sequence approaches as the input (or index) approaches some value. The concept of a limit is crucial in calculus and analysis, and it is used in the video to discuss the behavior of series as the number of terms approaches infinity.

💡Derivative

A derivative is a concept in calculus that represents the rate of change of a function with respect to its input variable. It is used to analyze the behavior of functions, particularly in determining monotonicity, which is crucial for applying the integral test in the video.

💡Monotonicity

Monotonicity refers to the consistency of increase or decrease in a function's values over its domain. A function is said to be monotonic if it is either always increasing or always decreasing. In the context of the video, monotonicity is important for the integral test, which requires the function to be decreasing for the test to be applicable.

Highlights

Exploration of P series and harmonic series in lesson 10.5.

P series is an infinite series of the format 1/n^P, where P is a positive number.

The convergence of P series is related to improper integrals, as covered in lesson 6.13.

The integral test indicates that P series converges if P > 1 and diverges if P ≤ 1.

Examples provided to determine the convergence or divergence of various P series.

The series 1/n^5 converges because P (5) is greater than one.

The series 1/n^0.2 diverges as P (0.2) is less than or equal to one.

The series 1/(n^(1/3)) diverges because P (1/3) is less than or equal to one.

The series 1/(n^(3/2)) diverges as it simplifies to 1/√n, with P (1/2) being less than or equal to one.

The series 1/n^e converges because P (e) is greater than one.

The harmonic series, a special P series with P = 1, always diverges.

The convergence or divergence of infinite series is determined by specific tests, such as the P series test and the integral test.

The series 1/n^3 converges, as P (3) is greater than one.

The series 1/n diverges as it is the harmonic series with P = 1.

The series 1/n^(-π) converges because P (-π) is greater than one.

The series 1/(3^n) is a convergent geometric series as the absolute value of its common ratio (1/3) is less than one.

The series 1/(n + 2)^2 does not converge based on the nth term test, but the integral test can be applied for further analysis.

The series 1/(n^(1/4)) does not converge as P (1/4) is less than or equal to one.

For the series 1/(n^P) and 1/(n^(2P)), both converge if P > 1/2 and P < 4.

The value of P for convergence in the given series is between 1/2 and 4, exclusive.

Transcripts

Browse More Related Video

Calculus BC – 10.5 Harmonic Series and p-series

Proof of p-series convergence criteria | Series | AP Calculus BC | Khan Academy

Integral Test and p-Series

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

Tests for Convergence

Unit 10 Study Guide - AP Calculus (BC Only)

AP Calculus BC Lesson 10.5

Takeaways

Q & A

What is a P-series in the context of infinite series?

How does the Integral Test relate to the convergence of a P-series?

What is the condition for a P-series to converge?

What is a harmonic series and how does it behave?

How can you determine the convergence or divergence of the series Σ from n=1 to Infinity of 1/n^5?

What is the behavior of the series Σ from n=1 to Infinity of 1/n^0.2?

What happens with the series Σ from n=1 to Infinity of 1/(n^(1/3))?

How does the nth term test work for determining the convergence of a series?

What is the condition for the convergence of the geometric series Σ from n=1 to Infinity of (e^n)/(2^n)?

How can you determine if the series Σ from n=1 to Infinity of n^(-e) converges?

What values of P make both the series Σ from n=1 to Infinity of P^n/(4^n) and the series Σ from n=1 to Infinity of 1/(n^(2P))) converge?