The Definite Integral Part II: Using Summation Notation to Define the Definite Integral
TLDRThe video script introduces the concept of summation notation, denoted by the capital Greek letter Sigma, which is a shorthand for adding a sequence of terms. It explains how this notation simplifies the representation of long sums, such as when n equals a hundred, and how it can be applied to various functions, including a simple example where X_i equals 2i. The script then transitions into discussing the area problem under the curve of x squared, using the summation notation to represent the area as the sum of rectangles' areas, each with a height of X_i squared and a width of 0.2. The X_i values are determined based on the function's evaluation at specific points. The script further explains the process of finding the area under a curve by dividing the interval into subintervals, choosing points within each, and forming a rectangle approximation. The limit of this approximation as the number of subintervals approaches infinity is defined as the definite integral, symbolized by the integral sign with limits a and b. This concept is fundamental to calculus, and the script emphasizes the importance of understanding the summation and limit processes in calculating areas and developing integral calculus.
Takeaways
- 📚 **Summation Notation**: The capital sigma (Σ) is used to represent the sum of a sequence of terms, where the subscript denotes the starting index and the superscript denotes the ending index.
- 🔢 **Generic Summation**: Summation notation can be used generically with a formula to represent the sum, such as \( \sum_{i=1}^{n} X_i = 2i \), which sums the first n natural numbers multiplied by two.
- 📈 **Customizing Summation**: The start and end values, as well as the formula for the sequence, can be customized, allowing for flexibility in representing various summations.
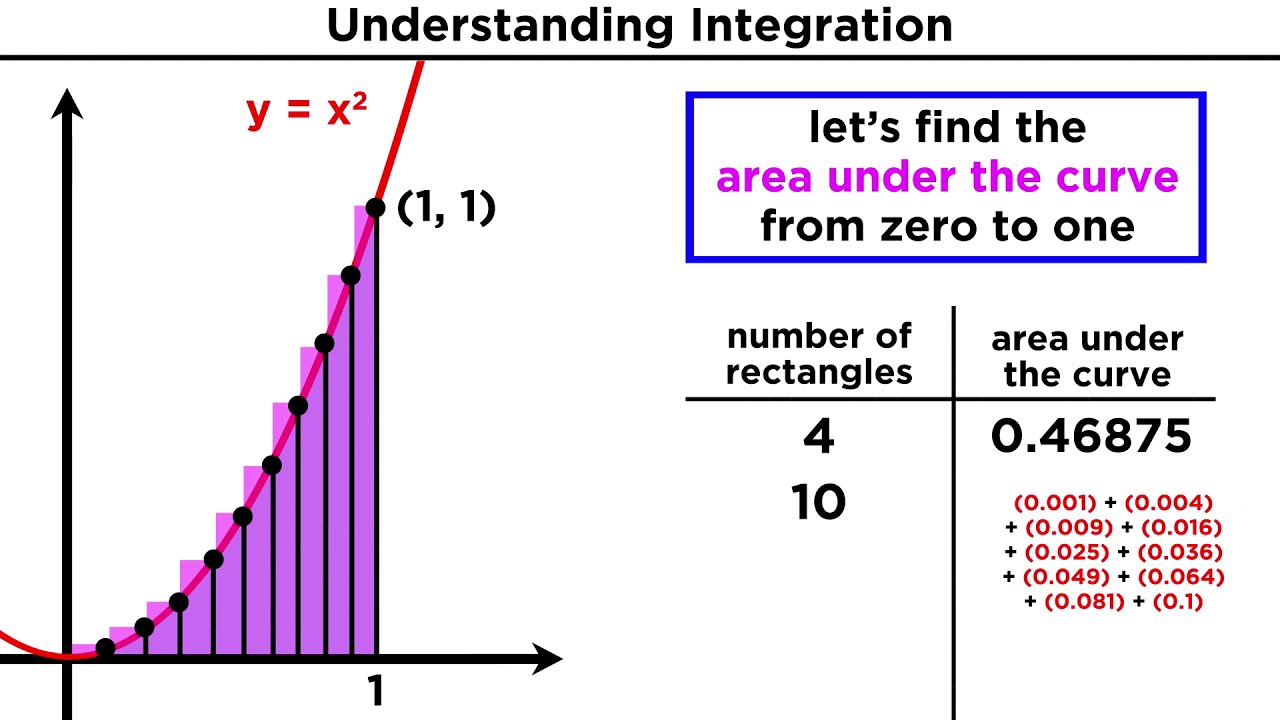
- 🏗️ **Area Under the Curve**: The area under a curve can be approximated using rectangles, where the height of each rectangle corresponds to the function value at a point and the width is constant.
- 📏 **Rectangle Approximation**: The area under the curve is calculated by summing the areas of rectangles, which is a method used before the concept of integration was formalized.
- 📉 **Summation in Area Calculation**: The summation notation can be applied to express the process of summing the areas of rectangles to approximate the area under a curve.
- 🔑 **Notational Efficiency**: Using summation notation makes it easier to represent complex sums, especially when dealing with large numbers of terms.
- 🚀 **Limit Concept**: The actual area under the curve is found by taking the limit of the rectangle approximation as the number of rectangles (n) approaches infinity.
- 💡 **Definite Integral**: The definite integral is a formalization of the limit of the rectangle approximation, represented with the integral symbol ∫ and bounds a and b.
- 📌 **Interval Division**: To calculate a definite integral, the interval is divided into n subintervals of width dx, each with a chosen point xi within it.
- ∞ **Infinite Sum**: The integral sign represents taking an infinite sum in the limit as the width of the rectangles (dx) approaches zero.
Q & A
What is summation notation?
-Summation notation is a mathematical notation that represents the sum of a sequence of terms. It is denoted by the capital Greek letter sigma (Σ) and is used to compactly write long sums, especially when the number of terms is large.
How does the summation notation work?
-The summation notation works by placing the capital sigma symbol (Σ), followed by the index variable (usually i), the starting value (often 1), and the ending value (often n) underneath the sigma, and the expression to be summed above it. It represents the sum of the expression for the index variable starting from the initial value up to the final value.
What is the purpose of using summation notation in the context of the script?
-In the script, summation notation is used to simplify the representation of the area under a curve by approximating it with the sum of areas of rectangles. This is particularly useful when dealing with a large number of rectangles, as it avoids the need to list each term individually.
What is the formula for the sum of the first n natural numbers squared?
-The formula for the sum of the first n natural numbers squared is given by the expression 1^2 + 2^2 + 3^2 + ... + n^2, which can be represented in summation notation as Σ(i^2) from i=1 to n.
How does the script relate summation notation to the area under a curve?
-The script relates summation notation to the area under a curve by using the concept of rectangle approximation. It shows that the area under a curve can be approximated by summing the areas of rectangles, each with a height corresponding to the function value at a chosen point and a width corresponding to the interval size. This sum is then represented using summation notation.
What is the significance of the width DX in the context of the definite integral?
-In the context of the definite integral, DX represents an infinitesimally small change in the x-axis, which is used to form the rectangles in the limit as the number of rectangles (n) goes to infinity. It is a symbolic representation of the width of each subinterval when approximating the area under a curve.
How does the choice of the point XI within each subinterval affect the approximation of the area under a curve?
-The choice of the point XI within each subinterval can affect the accuracy of the area approximation under a curve. Different methods, such as choosing the left endpoint, right endpoint, midpoint, maximum, or minimum value of the function within the interval, can yield different approximations. The right endpoint is commonly chosen for simplicity, but the choice can be varied to improve the approximation.
What is the final step in finding the area under a curve using the rectangle approximation method?
-The final step in finding the area under a curve using the rectangle approximation method is to take the limit of the sum of the areas of the rectangles as the number of rectangles (n) approaches infinity. This is represented by the definite integral symbol and gives an exact value for the area under the curve.
What is the definite integral symbol and how is it used?
-The definite integral symbol, represented by the letter 'S' with a horizontal line, is used to denote the exact area under a curve between two points, a and b. It is written as ∫ from a to b of f(X) dX, where f(X) is the function, and dX represents an infinitesimally small width of the rectangles used in the approximation.
Why is the concept of taking the limit as n goes to infinity important in calculus?
-The concept of taking the limit as n goes to infinity is fundamental in calculus because it allows for the transition from discrete sums to continuous areas. It is the basis for defining derivatives and integrals, which are key concepts in calculus for analyzing rates of change and accumulation, respectively.
What is the relationship between the summation notation and the definite integral in the context of the area under a curve?
-The summation notation is used to represent the discrete sum of areas of rectangles that approximate the area under a curve, while the definite integral symbolizes the limit of this sum as the number of rectangles approaches infinity, thus providing an exact measure of the area under the curve.
Outlines
📚 Summation Notation and Area Approximation
The first paragraph introduces summation notation, denoted by the capital Greek letter Sigma (Σ). It is used to represent the sum of a sequence of terms. The paragraph explains how to use this notation to simplify the representation of a sum, such as the sum from 1 to n of a generic symbol Xi. The concept is illustrated with examples, including a simple sequence where Xi equals 2i and a more complex one where Xi equals i squared. The paragraph then transitions into discussing the application of summation notation to the problem of finding the area under a curve, specifically the curve of x squared. The area is approximated using rectangles, and the summation notation is used to express the sum of the areas of these rectangles. The paragraph concludes by emphasizing the notational efficiency of summation notation, especially when dealing with large numbers of terms.
📈 The Definite Integral and Limit of Rectangle Approximation
The second paragraph delves into the concept of the definite integral, which is a fundamental part of calculus. It starts by discussing the process of finding the area under a curve between two points. The steps include dividing the interval into n subintervals of width Δx, choosing a point xi within each subinterval, and forming a rectangle approximation by summing the areas of these rectangles. The paragraph explains that the goal is to take the limit of this sum as n approaches infinity, which leads to the actual area under the curve. The definite integral is then defined with a new set of symbols, and the paragraph clarifies the relationship between Δx and dx, emphasizing the philosophical difference between them. The integral symbol is described as representing an infinite sum in the limit as the rectangles become infinitesimally small. The paragraph concludes by highlighting the importance of the definite integral in the development of calculus.
Mindmap
Keywords
💡Summation Notation
💡Rectangle Approximation
💡Definite Integral
💡Limit
💡Function Value
💡Interval
💡Subintervals
💡Width (Δx or dx)
💡Generic Symbol
💡Integration
💡Notational Efficiency
Highlights
Introduction to summation notation, denoted by the capital Sigma symbol.
Summation notation simplifies the expression of adding a sequence of terms, such as x1 + x2 + ... + xn.
A specific formula for X can be provided within the summation notation, for example, X_i = 2*i.
The flexibility of summation notation allows for different starting points and end points in the summation.
An example provided where the sum starts at 3 and ends at 7, with X_i = i^2.
Summation notation is a shorthand that is particularly useful for large numbers of terms.
The area problem under the curve of x^2 is approached using rectangle approximation.
The right rectangle approximation method involves summing the areas of rectangles along the curve.
The summation notation is used to represent the area under the curve as a sum of areas of rectangles.
X_i in the context of the area problem corresponds to specific values obtained from the function.
A generic formula for X is derived as X = 0.2 * I, where I is an index.
The area formula is simplified using the derived formula for X and summation notation.
The concept of summation is key for notational efficiency, especially when dealing with a large number of terms.
The process of dividing the interval into subintervals and choosing points within each for approximation is explained.
Different methods of choosing points within subintervals are discussed, such as endpoints, midpoints, or function extreme values.
The rectangle approximation formula is derived using the chosen points and the width of subintervals.
The limit of the rectangle approximation as the number of rectangles goes to infinity is taken to find the actual area.
The definite integral is introduced as a symbolic representation of the limit of the rectangle approximation.
The definite integral symbol includes the bounds a and b, and the function to be integrated, with dx representing an infinitesimally small width.
The philosophical difference between ΔX and dx is highlighted, with dx representing an infinitesimally small change.
The integral sign is a comprehensive symbol representing the sum of an infinite number of rectangles in the limit.
Transcripts
Browse More Related Video

The Definite Integral Part III: Evaluating From The Definition
AP Calculus AB: Lesson 6.2 Part 2 (Limit Definition of Definite Integral)

Riemann sums in summation notation | Accumulation and Riemann sums | AP Calculus AB | Khan Academy
What is Integration? Finding the Area Under a Curve

Math 1325 Lecture 13 2

What Is an Integral?
5.0 / 5 (0 votes)
Thanks for rating: