Fundamental Theorem of Calculus - Part I

Prime Newtons
25 Apr 202305:52
EducationalLearning
32 Likes 10 Comments

TLDRThis video script provides an insightful explanation of the Fundamental Theorem of Calculus, which bridges the gap between differentiation and integration. The presenter clarifies that while differentiation deals with instantaneous change, integration is about accumulating those changes. The script introduces the two parts of the theorem, emphasizing the importance of understanding the relationship between the derivative of an integral and the integral itself. The video uses a specific example to illustrate how to apply the theorem, replacing the variable 't' with a function of 'x' and then multiplying by the derivative of that function. The presenter also advises against attempting to integrate before differentiating when dealing with complex functions, as it may require advanced skills beyond the viewer's current level. The video concludes by encouraging continuous learning in the field of calculus.

Takeaways
  • 📚 The video introduces the Fundamental Theorem of Calculus, which is a key concept in calculus that connects differentiation and integration.
  • 🔗 Differentiation is about instantaneous change, while integration is about accumulating changes over an interval.
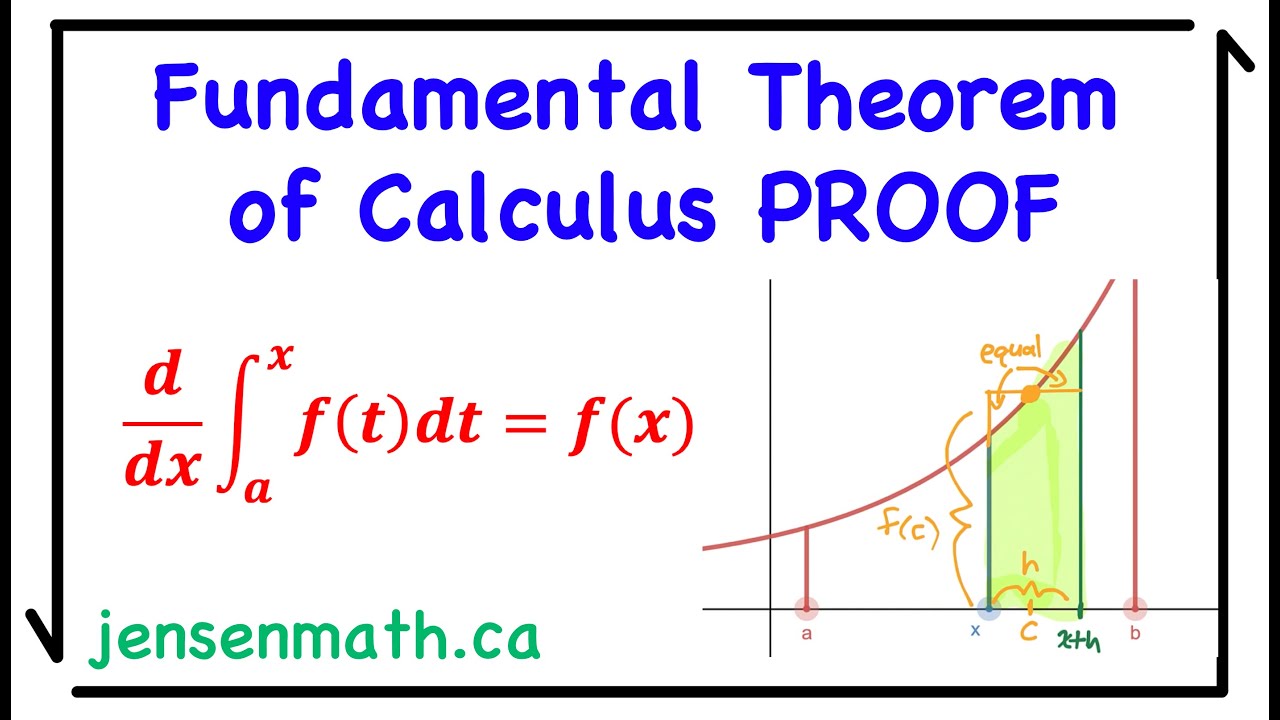
  • 🧩 Part one of the Fundamental Theorem of Calculus states that the derivative of the integral of a function is the function itself, provided certain conditions are met.
  • 📌 When differentiating an integral, it's important to multiply by the derivative of the upper boundary function, not just the constant.
  • 🔑 Students often overlook the need to multiply by the derivative of the upper boundary function when differentiating an integral.
  • ❌ The video clarifies that not all functions can be integrated before differentiation; some functions do not have elementary integrals.
  • 🔄 The process demonstrated involves replacing the variable 't' with a function of 'x', and then multiplying by the derivative of that function.
  • 📐 An example in the video shows how to apply the theorem by integrating and differentiating a function involving sine and a polynomial.
  • 🤔 The video challenges the viewer to consider whether they could integrate before differentiating and suggests that it's not always possible with non-elementary functions.
  • 📈 The final answer in the example provided is a function of 'x' involving a sine term, demonstrating the application of the theorem.
  • 💡 The video encourages continuous learning in calculus, as understanding these concepts is crucial for further progress in the subject.
  • 👋 The presenter signs off with a reminder that those who stop learning have stopped living, emphasizing the importance of ongoing education.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the Fundamental Theorem of Calculus, which establishes the connection between differentiation and integration.

  • What is the first part of the Fundamental Theorem of Calculus?

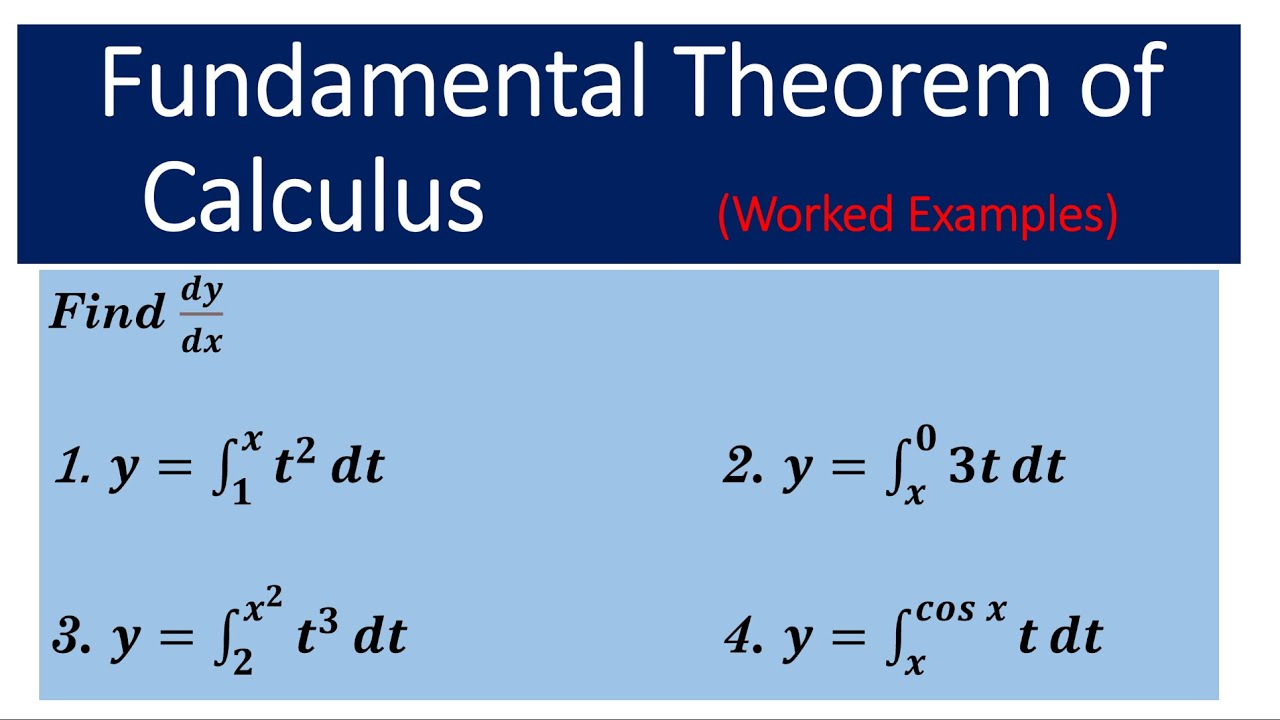
    -The first part of the Fundamental Theorem of Calculus states that if you have a function F(t) and you integrate it with respect to t from a constant 'a' to a function of X, G(X), then the derivative of this integral with respect to X is F(G(X)) multiplied by the derivative of G(X).

  • What is the role of the constant 'a' and the function G(X) in the theorem?

    -In the theorem, 'a' is a constant and serves as the lower boundary of integration, while G(X) is a function of X and serves as the upper boundary. The integral is taken from 'a' to G(X).

  • Why do students often make a mistake when differentiating the integral?

    -Students often make a mistake by not realizing that they need to multiply the differentiated function by the derivative of the upper boundary function (G(X)) when differentiating the integral.

  • Can you integrate and then differentiate the same function to get back to the original function?

    -In general, no, because not all functions are integrable in a way that their integral can be differentiated back into the original function. The process is not always reversible, especially when dealing with non-elementary functions.

  • What is the significance of the derivative of G(X) in the differentiation of the integral?

    -The derivative of G(X) is significant because it is the factor by which the function F(G(X)) is multiplied when differentiating the integral with respect to X.

  • What is the second part of the Fundamental Theorem of Calculus?

    -The second part of the Fundamental Theorem of Calculus is a direct consequence of the first part and is used to evaluate definite integrals by using antiderivatives (indefinite integrals).

  • Why is the function F(t) replaced by f(x) when differentiating the integral with respect to X?

    -The function F(t) is replaced by f(x) because when the integral is with respect to t and the upper boundary is a function of X, G(X), the variable t is effectively replaced by the expression G(X), and thus F(t) becomes F(G(X)) or f(x) when G(X) is simplified to x.

  • What is the process of differentiating the integral of F(t) from a constant to X with respect to X?

    -The process involves replacing t with the upper boundary function G(X), multiplying by the derivative of G(X), and then applying the Fundamental Theorem of Calculus to find the derivative of the integral with respect to X.

  • Why is it important to understand the Fundamental Theorem of Calculus?

    -Understanding the Fundamental Theorem of Calculus is important because it provides a bridge between differential and integral calculus, allowing for the manipulation and solution of problems involving rates of change and accumulation of quantities.

  • What does the video suggest about the learning process in calculus?

    -The video suggests that learning in calculus is a continuous process, emphasizing that one should never stop learning as it is a vital part of intellectual growth and understanding more complex mathematical concepts.

Outlines
00:00
📚 Introduction to the Fundamental Theorem of Calculus

This paragraph introduces the audience to the concept of the Fundamental Theorem of Calculus, which bridges the gap between differentiation and integration. The speaker clarifies that differentiation deals with instantaneous change, while integration accumulates those changes. The first part of the theorem is explained, which states that the derivative of the integral of a function F(t) from a constant to a function G(x) is simply F(G(x)) multiplied by the derivative of G(x). The explanation is illustrated with an example involving the sine function and a polynomial, emphasizing the importance of multiplying by the derivative of the upper boundary function in the integral expression.

05:03
🚫 Incorrect Approach: Trying to Integrate Before Differentiating

The speaker advises against attempting to integrate before differentiating when dealing with the Fundamental Theorem of Calculus, especially if the integral does not correspond to the derivative of a known elementary function. The paragraph highlights that the theorem is designed to be used in the order presented: to differentiate the integral, not the other way around. The video aims to correct a common misunderstanding and encourages continuous learning in calculus, with a reminder that those who cease to learn have ceased to live.

Mindmap
Keywords
💡Differentiation
Differentiation is a mathematical process that involves finding the derivative of a function, which represents the rate of change of the function with respect to its variable. In the video, differentiation is discussed in the context of the Fundamental Theorem of Calculus, which connects differentiation with integration. An example from the script is when the instructor explains that differentiation 'undoes' integration, highlighting the inverse relationship between the two processes.
💡Integration
Integration is the mathematical operation that is the reverse of differentiation. It involves finding the accumulated sum of an infinite number of infinitesimally small quantities of a function over an interval. The video emphasizes the connection between integration and differentiation, and how the Fundamental Theorem of Calculus bridges these two concepts. An example used in the script is the integral from a constant to a function of X, which is a key part of the theorem's application.
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a central theorem that links the concepts of differentiation and integration. It is divided into two parts, with the first part stating that the integral of a derivative is the original function (up to a constant), and the second part stating that the derivative of an integral evaluates to the original function. The theorem is crucial for solving problems that involve both differentiation and integration, as demonstrated in the video.
💡Instantaneous Change
Instantaneous change refers to the concept of finding the rate of change of a function at a specific point in time, which is the essence of differentiation. The video mentions this concept when discussing how differentiation deals with the change at a single moment, as opposed to integration, which accumulates changes over a period. An example from the script is the differentiation of a function with respect to time, which gives the instantaneous rate of change.
💡Accumulation
Accumulation in the context of calculus refers to the process of summing up or integrating a quantity over a given interval. It is used to describe the concept of integration, which is about adding up all the small changes to find a total amount. The video script uses the term to contrast with differentiation, where accumulation is about adding up changes over time, whereas differentiation focuses on the change at a specific instant.
💡Derivative
A derivative is a measure of how a function changes as its input changes. It is a fundamental concept in calculus and is used to find the rate at which a quantity is changing at any given point. In the video, the derivative is discussed in relation to the Fundamental Theorem of Calculus, where taking the derivative of an integral results in the original function. An example from the script is the derivative of the integral from a to G(x), which simplifies to f(G(x)) * G'(x).
💡Boundary
In calculus, a boundary refers to the limits within which an integral is evaluated. The video script discusses how boundaries are crucial in integral calculus, as they define the interval over which the function is integrated. An example from the script is the integral of F(t) with respect to t from a constant 'a' to a function of X, G(X), where 'a' and G(X) serve as the lower and upper boundaries, respectively.
💡Constant
A constant is a value that does not change within the context it is being used. In the context of calculus, constants are often used to define the boundaries of an integral or to simplify expressions. The video emphasizes the importance of correctly placing constants in integral expressions, as they cannot be the upper boundary of an integral. An example from the script is the integral from a constant 'a' to a function of X, where 'a' is the lower boundary.
💡Function of X
A function of X is an expression that assigns a unique value to each value of X within its domain. In the video, functions of X are used to represent the upper boundary of an integral and to illustrate how the Fundamental Theorem of Calculus is applied. An example from the script is G(X), which could be X, X squared, or X minus 5, and is used to show how the derivative of the integral is computed.
💡Special Skill
In the context of the video, a special skill refers to advanced mathematical techniques or knowledge that may be beyond the current level of understanding of the viewer. The term is used to caution against attempting to integrate certain complex functions before differentiating, as it may require a deeper understanding of calculus. An example from the script is the suggestion that integrating the given function before differentiating would require a 'special skill' that is not covered in the video.
💡Elementary Function
An elementary function is a function that can be expressed using a finite combination of basic mathematical operations, exponentials, logarithms, constants, and trigonometric functions. The video mentions that the given function in the problem is not the derivative of any elementary function, indicating that it cannot be integrated using basic integration techniques. This highlights the complexity of the problem and the need to apply the Fundamental Theorem of Calculus.
Highlights

Differentiation and integration are two fundamental operations in calculus that are often seen as opposites, but they are actually connected.

The Fundamental Theorem of Calculus is introduced, which bridges the gap between differentiation and integration.

Differentiation is concerned with instantaneous change, while integration is about accumulating changes over a period.

The first part of the Fundamental Theorem of Calculus is explained, which involves integrating a function and then differentiating the result.

The importance of understanding the boundaries in integration and how they interact with differentiation is emphasized.

The derivative of an integral with respect to a variable is shown to be the original function, multiplied by the derivative of the boundary function.

Students often overlook the need to multiply by the derivative of the boundary function when differentiating an integral.

An example is provided to illustrate the process of differentiating an integral with a boundary function of X.

The concept that the derivative of X is one is clarified, which is a common point of confusion for students.

The video demonstrates how to apply the Fundamental Theorem of Calculus to a specific problem involving a sine function and a boundary function.

The process of substituting the boundary function into the integral and then differentiating is shown step by step.

The final answer to the example problem is derived, showcasing the application of the theorem.

The video addresses the question of whether it's possible to integrate before differentiating and explains why it's not advisable in this context.

The limitations of integrating certain functions that are not derivatives of elementary functions are discussed.

The importance of using the Fundamental Theorem of Calculus to solve the problem is emphasized, rather than attempting to integrate first.

The video concludes with a reminder to never stop learning, as those who stop learning have essentially stopped living.

Transcripts

Browse More Related Video

Calculus (Version #2) - 9.1 The Second Fundamental Theorem of Calculus

Fundamental Theorem of Calculus Parts 1&2

1st Fundamental Theorem of Calculus PROOF | Calculus 1 | jensenmath.ca

Calculus 1 - Integration: Proof of the Fundamental Theorem of Calculus

Derivatives of Integrals (w/ Chain Rule)

Calculus - Lesson 15 | Relation between Differentiation and Integration | Don't Memorise

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusDifferentiationIntegrationFundamental TheoremEducational ContentMathematicsInstantaneous ChangeAccumulationDerivativeIntegralLearningTheoretical Math