2.2 Kinematics in One Dimension | General Physics

Chad's Prep

6 Sept 202329:30

EducationalLearning

32 Likes 10 Comments

TLDRIn this educational video, Chad explores kinematics in one dimension, focusing on problem-solving techniques for scenarios with constant velocity and uniform acceleration. He emphasizes understanding the concepts' relationships and provides equations for calculating displacement, velocity, and acceleration. Through examples, Chad demonstrates how to apply these principles, clarifying that the average velocity and time factors play crucial roles in determining the overall motion and distance traveled.

Takeaways

📚 Kinematics in one dimension is the focus of the lesson, aiming to develop problem-solving skills and an intuitive understanding of displacement, velocity, and acceleration.
🔢 There are two primary scenarios in one-dimensional kinematics: constant velocity (no acceleration) and uniform acceleration (constant acceleration).
🚗 For constant velocity, the equation Δx = v * Δt applies, where Δx is displacement, v is velocity, and Δt is the change in time.
🏃‍♂️ In the case of uniform acceleration, the average velocity is used, and the displacement is calculated using the equation Δx = (v_initial + v_final) / 2 * t.
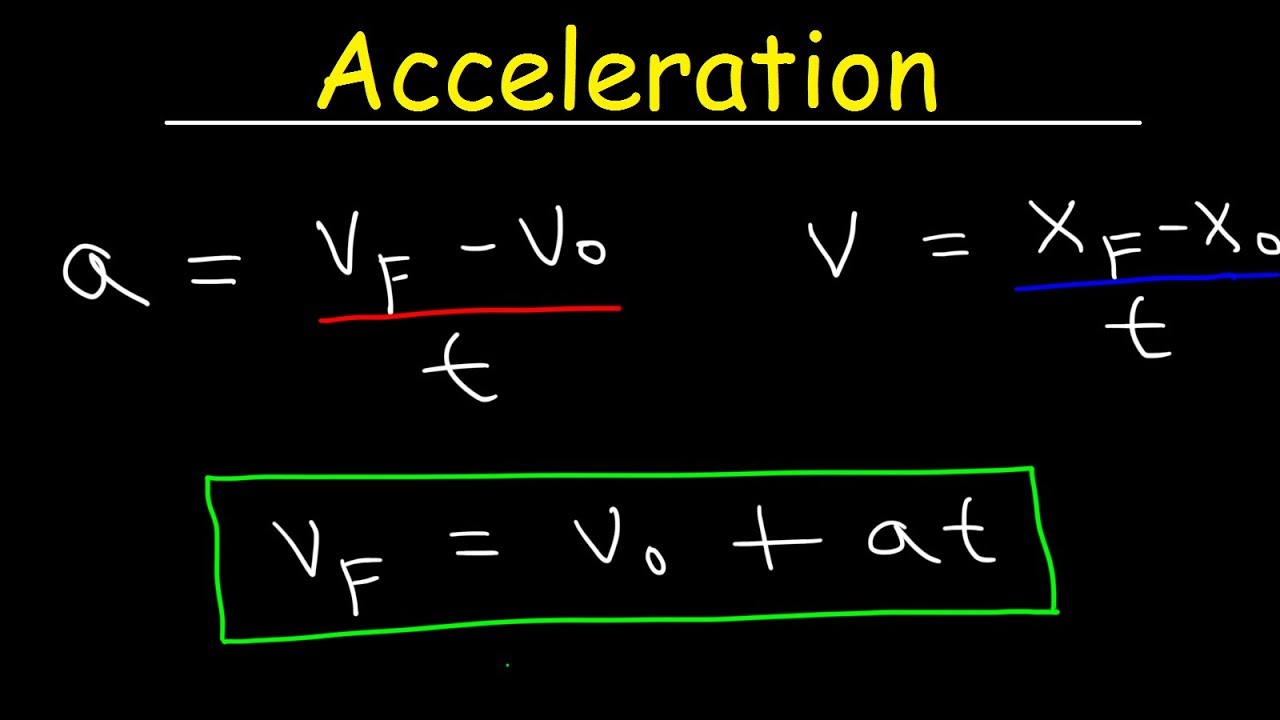
📈 The concept of acceleration is key, defined as the change in velocity over the change in time, and can be represented as a positive or negative value depending on the direction of change.
🔄 When dealing with uniform acceleration, it's important to consider the square terms, as they are commonly forgotten and can lead to errors in calculations.
📊 The lesson emphasizes the importance of systematically working through the available equations to solve kinematics problems, even if the initial conditions are not immediately clear.
🧠 Developing an intuitive feel for the relationships between displacement, velocity, and acceleration is crucial for demystifying kinematics problems.
📝 For a round-trip journey with different average speeds for each leg, the overall average speed is influenced by the time spent at each speed, and it may not be the simple average of the two speeds.
🛣️ In problems involving travel over a distance with varying speeds, it's essential to calculate the time spent at each speed to determine the overall average speed correctly.
📊 The lesson provides examples and practical applications of kinematics equations, highlighting the importance of understanding the underlying concepts and being able to apply them to real-world scenarios.

Q & A

What is the main topic of the lesson?
-The main topic of the lesson is kinematics in one dimension, focusing on problem-solving in the area of kinematics with an emphasis on understanding displacement, velocity, and acceleration.
What are the two scenarios that will be dealt with in this lesson?
-The two scenarios are constant velocity (no acceleration) and uniform acceleration (constant acceleration).
What is the single equation used for kinematics calculations when there is no acceleration?
-The single equation used when there is no acceleration is Delta X (displacement) equals velocity times time (ΔX = V × ΔT).
How is the average velocity calculated for uniform acceleration?
-The average velocity for uniform acceleration is calculated as the average of the initial and final velocities (V_avg = (V_initial + V_final) / 2).
What is the significance of the equation ΔX = V_avgT for uniform acceleration?
-The equation ΔX = V_avgT is significant for uniform acceleration because it allows us to calculate the displacement when the velocity is changing at a constant rate over time.
Why might students struggle with kinematics problems involving varying acceleration?
-Students might struggle with varying acceleration because it requires more complex calculations, potentially involving calculus, which can be challenging for those taking an algebra-based physics class.
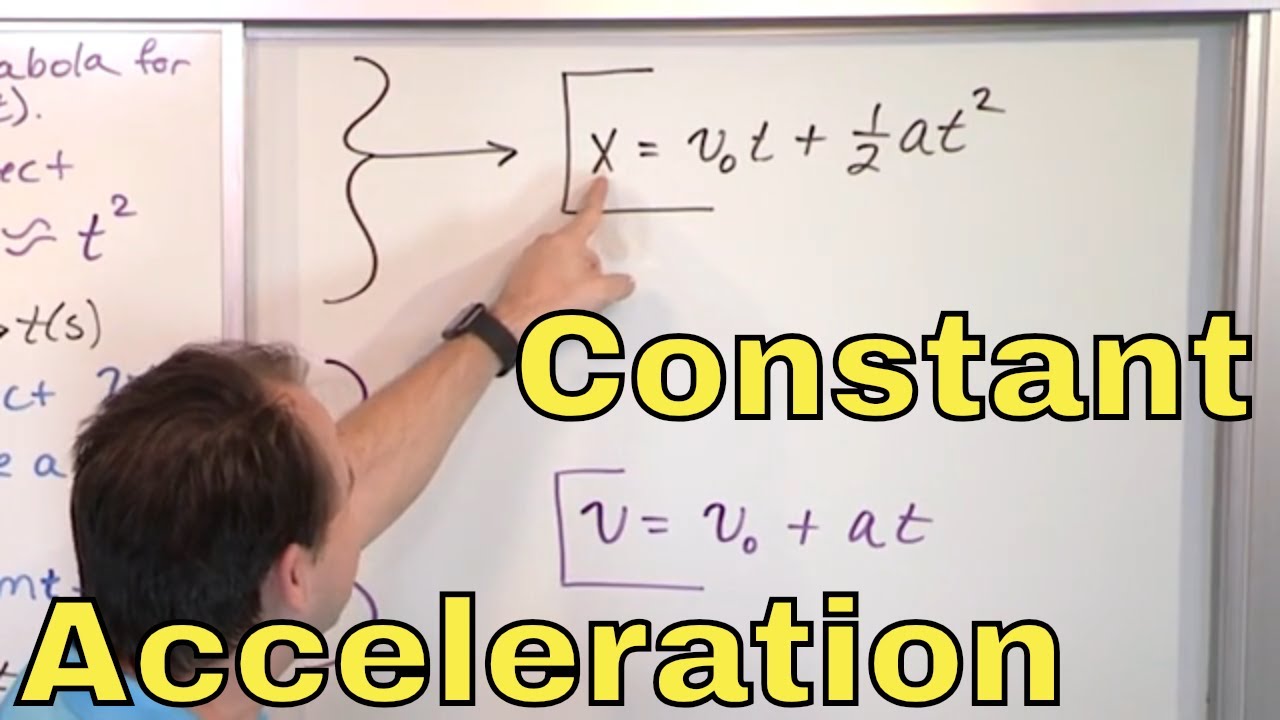
What is the role of the equation ΔX = V_initialT + (1/2)aT^2 in the context of uniform acceleration?
-This equation accounts for the change in velocity due to constant acceleration, where 'a' is the acceleration and 'T' is the time. It helps calculate the displacement by considering the initial velocity, the time during which the object is moving, and the effect of acceleration on the displacement.
How does the instructor approach the concept of acceleration in the lesson?
-The instructor approaches the concept of acceleration by explaining it as the change in velocity per second and emphasizes understanding its intuitive meaning to solve problems more effectively.
What is the average speed of a round trip if a person travels 60 miles to work at an average speed of 40 miles per hour and 60 miles back home at an average speed of 60 miles per hour?
-The average speed of the round trip is not simply the average of the two speeds (40 mph and 60 mph), but rather it is closer to 40 mph due to the longer time spent traveling at the lower speed.
How does the instructor demonstrate the concept of uniform acceleration over time?
-The instructor demonstrates the concept by using a car accelerating from rest with an acceleration of 10 meters per second squared, and showing how the velocity increases by 10 m/s every second, leading to increasing displacement with each successive second.
What is the displacement of the car in the first second of its uniform acceleration from rest?
-The displacement in the first second is calculated by using the average velocity for that interval, which is half of the initial velocity (0 m/s) and the final velocity (10 m/s), resulting in an average velocity of 5 m/s and a displacement of 5 meters.

Outlines

00:00

📚 Introduction to Kinematics in One Dimension

The paragraph introduces the topic of kinematics in one dimension, emphasizing the challenges students may face in problem-solving within this area. The speaker, Chad, outlines his goal to provide an intuitive understanding of the material and a systematic approach to problem-solving. He welcomes viewers to his educational platform, which offers comprehensive resources for various science subjects. Chad reviews the concepts of displacement, velocity, and acceleration from the previous lesson and hints at the focus on constant velocity and uniform acceleration scenarios, clarifying that varying acceleration will not be covered in this algebra-based physics class.

05:00

🚀 Understanding Uniform Acceleration and Displacement

This paragraph delves into the specifics of uniform acceleration, explaining how it differs from constant velocity. Chad discusses the use of average velocity in calculations when dealing with uniform acceleration and highlights the importance of understanding the relationship between initial and final velocities. He critiques the common practice of presenting a fifth equation in textbooks, arguing that it adds unnecessary confusion for students. Chad's approach is to simplify the concept by focusing on the four main equations relevant to uniform acceleration, making the subject more accessible and less intimidating.

10:04

🧠 Developing Intuition for Acceleration and Time

Chad continues to build on the concept of uniform acceleration by discussing the least favorite equation involving squares, which he finds difficult for mental calculations. He emphasizes the importance of recognizing that not all equations are necessary for solving problems, especially when an intuitive understanding of the concepts can be applied. Chad then introduces a problem-solving scenario involving a car's displacement and average speed, illustrating how to apply the concepts of velocity and time to find the solution.

15:08

🛣️ Calculating Displacement and Average Speed in Real-World Scenarios

The paragraph presents a real-world scenario of a man commuting to work and back home, highlighting the misconception that the average speed of a round trip is the simple average of the individual average speeds. Chad explains why this is incorrect and provides a detailed calculation to find the true average speed for the entire round trip. He emphasizes the importance of considering the time spent at each average speed and how it affects the overall average velocity.

20:09

🏎️ Detailed Analysis of Uniform Acceleration Over Time

Chad tackles a multi-part question involving a car accelerating uniformly from rest. He explains how to calculate the velocity after a certain time and the displacement during each successive second. Chad uses both intuitive reasoning and formal equations to demonstrate how the car's displacement increases with each second due to the constant acceleration. He emphasizes the importance of understanding acceleration and its impact on velocity and displacement over time.

25:15

📐 Multiple Methods for Calculating Displacement During Uniform Acceleration

In this paragraph, Chad explores different methods for calculating the displacement of an object undergoing uniform acceleration. He uses the example of an object moving from rest and加速 uniformly, discussing various equations that can be applied to find the displacement during different time intervals. Chad highlights the flexibility in choosing the most straightforward method for problem-solving, depending on the given information and the learner's preference. He reinforces the concept by using different approaches to arrive at the same result, showcasing the interconnectedness of the equations and their applications.

Mindmap

Keywords

💡Kinematics

Kinematics is the branch of physics that deals with the motion of objects without considering the forces that cause the motion. In the video, the topic of kinematics, specifically in one dimension, is the focus of the lesson, aiming to help students understand and solve problems related to the motion of objects along a straight line.

💡Displacement

Displacement is a vector quantity that represents the change in position of an object. In the context of the video, displacement is calculated as the product of velocity and time. It is used to determine the total distance an object has moved from its initial position to its final position, and is a key concept in solving one-dimensional kinematics problems.

💡Velocity

Velocity is a physical quantity that describes the rate of change of an object's position with respect to time, and it is a vector quantity that includes both magnitude (speed) and direction. In the video, the definition of velocity is used to derive equations for calculating displacement, and understanding velocity is crucial for solving problems involving constant speed as well as uniform acceleration.

💡Acceleration

Acceleration is the rate of change of velocity of an object with respect to time, and it is typically measured in meters per second squared (m/s^2). In the video, the concept of uniform acceleration, where an object's speed changes at a constant rate, is discussed. This is a fundamental concept for solving kinematics problems involving changing speeds.

💡Uniform Acceleration

Uniform acceleration refers to a type of motion where an object's acceleration remains constant throughout the motion. In the video, the instructor explains how to calculate displacement and velocity for objects undergoing uniform acceleration, emphasizing the use of specific kinematic equations that account for this constant rate of change in speed.

💡Problem Solving

Problem solving in the context of the video involves applying kinematic principles and equations to determine unknown quantities such as displacement, velocity, and acceleration. The instructor aims to develop an intuitive understanding and a systematic approach for students to tackle kinematics problems, both with constant velocity and uniform acceleration scenarios.

💡Average Velocity

Average velocity is defined as the mean speed of an object over a certain distance or time interval. In the video, the concept of average velocity is used to derive equations for calculating displacement when an object is undergoing uniform acceleration. It is particularly useful when the velocity is changing and cannot be simply equated with the initial or final velocity alone.

💡Initial and Final Velocities

Initial and final velocities are the velocities of an object at the beginning and end of a specified time interval or displacement. In the video, these velocities are crucial for calculating the average velocity and for using in kinematic equations to find other unknowns, such as displacement and acceleration, especially in scenarios with uniform acceleration.

💡Calculus-based Physics

Calculus-based physics is a more advanced approach to physics that incorporates calculus to deal with problems involving rates of change, such as acceleration and varying acceleration. In the video, it is mentioned that while calculus-based physics might cover varying acceleration, the algebra-based class being discussed will focus on constant velocity and uniform acceleration scenarios.

💡Master Course

The term 'Master Course' refers to comprehensive, in-depth educational programs designed to thoroughly cover a subject area. In the video, the instructor mentions that for those joining his master course, the problems discussed are already typed out in study guides, suggesting a structured learning path with additional resources for deeper understanding of the material.

Highlights

The lesson focuses on kinematics in one dimension, specifically problem-solving in the area of kinematics with an emphasis on developing an intuitive feel for the material.

Two main scenarios are discussed: constant velocity (no acceleration) and uniform acceleration (constant acceleration).

For constant velocity, the equation Δx = velocity × time is used, derived from the definition of velocity (Δx/ΔT).

In the case of uniform acceleration, the average velocity is used in the equation Δx = (initial velocity + final velocity) / 2 × time.

The lesson aims to demystify kinematics problems and provide a systematic foundation for approaching them.

Displacement, velocity, and acceleration are the key quantities analyzed in the lesson, with their interrelationships being crucial for solving problems.

For uniform acceleration, the equation Δx = initial velocity × time + 0.5 × acceleration × time² is introduced, accounting for the changing velocity.

The lesson clarifies that in algebra-based physics classes, varying acceleration is not typically dealt with, unlike in calculus-based physics classes.

The concept of average velocity is crucial for solving problems involving uniform acceleration, where the speed is not constant and changes at a constant rate.

The lesson provides a method for determining the total displacement when given constant speed and time, using the equation Δx = velocity × time.

A man's round trip average speed is discussed, highlighting that it's not simply the average of the two speeds involved, but depends on the time spent at each speed.

The lesson explains how to calculate the average speed for the entire round trip by considering the time spent at each average speed.

A multi-part question involving a car accelerating uniformly from rest is used to illustrate the concepts of velocity and displacement over time intervals.

The velocity after a certain time period is calculated by adding the product of acceleration and time to the initial velocity.

The lesson demonstrates that the displacement in each successive second increases due to the constant acceleration, using both intuitive reasoning and equations.

Different methods for calculating displacement in each second are presented, including using the average velocity and the formula Δx = initial velocity × time + 0.5 × acceleration × time².

The lesson concludes by showing that various equations can be used to solve for displacement, but some methods are more intuitive or easier depending on the scenario.

Transcripts

Browse More Related Video

Kinematics Part 1: Horizontal Motion

Physics - Acceleration & Velocity - One Dimensional Motion

How to Cram Kinematics in 1 hour for AP Physics 1

Rotational Kinematics Physics Problems, Basic Introduction, Equations & Formulas

01 - Motion with Constant Acceleration in Physics (Constant Acceleration Equations)

AP Calculus AB: Lesson 6.4 Rectilinear Motion

Related Tags

PhysicsEducation Kinematics ProblemSolving OneDimension ChadWise Velocity Acceleration Displacement AlgebraBased IntuitiveUnderstanding