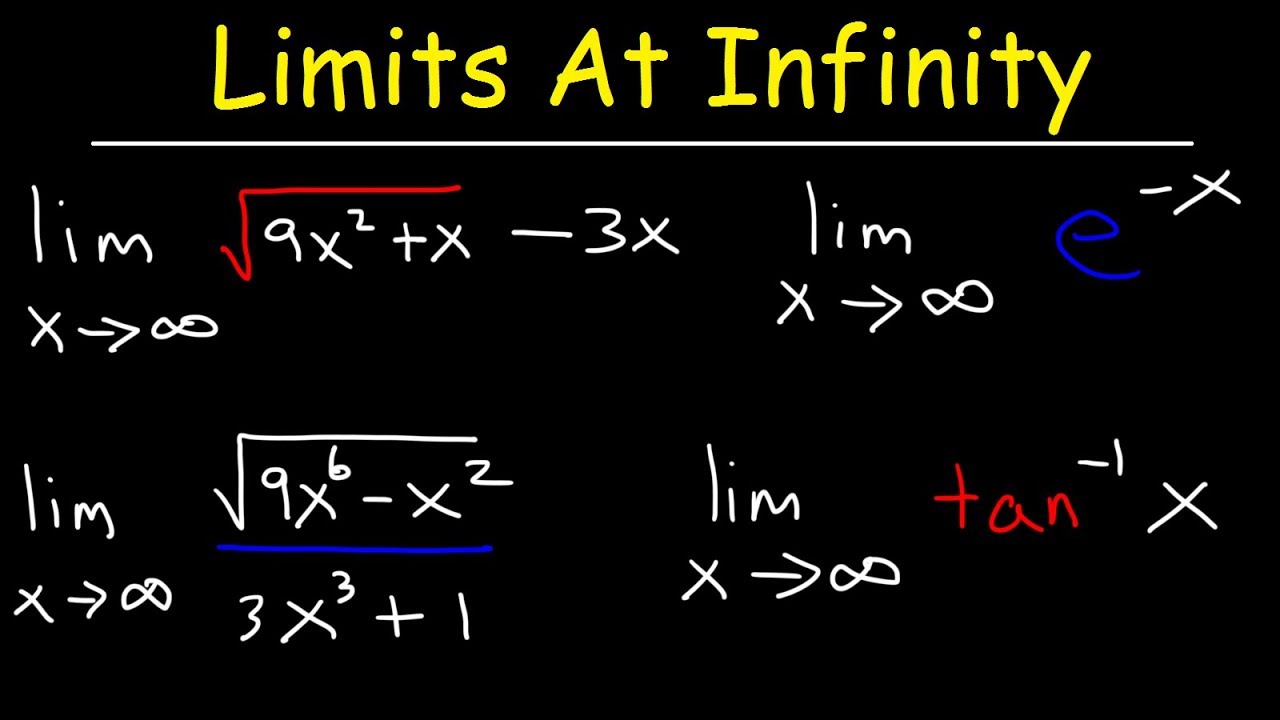How to Find the Limit at Infinity (NancyPi)
TLDRThe video script provides a comprehensive guide on calculating limits at infinity and negative infinity. It covers various types of mathematical expressions, including polynomials, constants, rational expressions, and exponential functions. The key concepts are explained with examples, highlighting shortcut methods for rational expressions and properties for trigonometric functions. The script emphasizes understanding the behavior of functions as x approaches very large or very small values, and offers algebraic techniques to find limits, ultimately aiming to demystify the complex topic of limits.
Takeaways
- 📚 Limits at infinity and negative infinity are important topics in calculus, often approached with various methods depending on the type of expression involved.
- 🔢 For polynomials and constants, as x approaches infinity or negative infinity, the limit behavior depends on the highest power of x present in the expression.
- 🚀 When dealing with polynomials, higher degree terms dominate the limit as x becomes very large, making lower degree terms insignificant.
- 🔄 For even powers of x, negative values of x lead to positive results when squared, thus affecting the limit at negative infinity.
- 🔢 In rational expressions, comparing the degrees of the numerator and the denominator provides a shortcut for finding limits at infinity.
- 📈 If the degree of the numerator is less than the denominator, the limit approaches 0, as the higher power is in the denominator, making the expression approach 0.
- 🎯 When the degrees of the numerator and the denominator are equal, the limit is the ratio of the leading coefficients, disregarding lower degree terms.
- 🌟 If the numerator's degree is greater than the denominator's, the limit is either positive or negative infinity, depending on the leading terms.
- 📊 For expressions of the form 1/x or 1/x^n (where n is a positive integer), the limit as x approaches infinity or negative infinity is 0.
- 🥂 The property that the limit of (sin x)/x as x approaches infinity or negative infinity equals 0 is useful for simplifying such expressions.
- 🧬 Exponential expressions with negative exponents can be rewritten with positive exponents by taking the reciprocal, which simplifies the process of finding limits at infinity.
Q & A
What is the main topic of the video?
-The main topic of the video is finding the limit at infinity or negative infinity for different types of mathematical expressions.
How does the behavior of a polynomial expression change as x approaches infinity?
-As x approaches infinity, polynomial expressions with positive leading terms will also approach infinity, while those with negative leading terms will approach negative infinity.
What is the degree of a polynomial?
-The degree of a polynomial is the highest power of x that appears in the polynomial expression.
How can you quickly find the limit of a rational expression as x approaches infinity?
-You can quickly find the limit of a rational expression by comparing the degrees of the numerator and the denominator. If the degree of the numerator is less than the denominator, the limit is 0. If they are equal, the limit is the ratio of the leading coefficients. If the numerator's degree is greater, the limit is infinity or negative infinity, depending on the leading terms.
What happens to the limit of a constant expression as x approaches infinity?
-The limit of a constant expression as x approaches infinity remains the constant value itself, as the constant does not change regardless of the value of x.
How does the concept of infinity help in understanding limits?
-The concept of infinity helps in understanding limits by providing a way to visualize the behavior of expressions as they grow without bound. It is not meant to be a literal value that can be substituted, but rather a conceptual tool for comprehension.
What is the limit of 1/x as x approaches infinity or negative infinity?
-The limit of 1/x as x approaches either infinity or negative infinity is 0, because any non-zero number divided by an infinitely large number tends toward 0.
What property is used to find the limit of (sin x)/x as x approaches infinity or negative infinity?
-The property states that the limit of (sin x)/x as x approaches infinity or negative infinity is 0, regardless of the specific form of the expression.
How can you find the limit of an exponential expression with a negative exponent as x approaches infinity?
-To find the limit of an exponential expression with a negative exponent as x approaches infinity, you can rewrite the expression as 1 over the exponential expression with a positive exponent. Then, as x approaches infinity, the expression will tend toward 0 because you are dividing by an infinitely large number.
What is the general rule for finding the limit of 1/x^n as x approaches infinity or negative infinity?
-The general rule is that the limit of 1/x^n as x approaches infinity or negative infinity is 0, provided that n is a positive integer. This is because dividing by an increasingly large number (x^n) tends toward 0.
How does the video suggest approaching limits at infinity for different types of expressions?
-The video suggests using specific strategies for different types of expressions: comparing degrees for polynomials, using the ratio of leading coefficients for rational expressions, and applying specific properties for forms like (sin x)/x and exponential expressions with negative exponents.
Outlines
📚 Introduction to Limits at Infinity
The video begins with Nancy introducing the concept of limits at infinity and negative infinity. She explains that limits are a complex topic and mentions other videos for different types of limits. The focus of this video is on polynomials, constants, and rational expressions as x approaches infinity or negative infinity. Nancy also provides a shortcut for finding limits in the form of rational expressions based on the degrees of the numerator and denominator.
📈 Limits of Polynomials and Constants
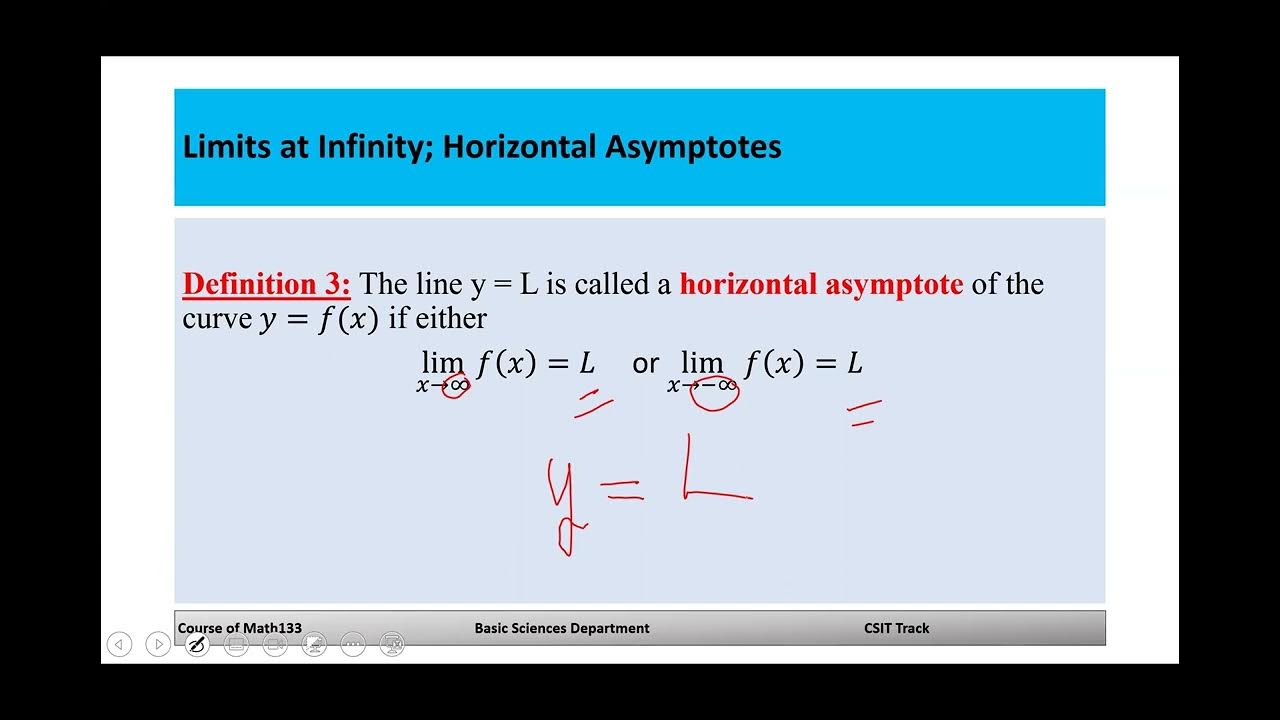
Nancy discusses the behavior of polynomials and constants at infinity. She explains that terms with higher powers grow larger as x approaches infinity and that lower degree terms become negligible. For polynomials with a positive leading term, the limit is infinity, and for those with a negative leading term, the limit is negative infinity. Constants, regardless of the value of x, have a limit equal to themselves, as they do not change.
📊 Shortcut for Rational Expressions
The video segment focuses on a shortcut method for finding limits of rational expressions as x approaches infinity or negative infinity. Nancy explains that by comparing the degrees of the numerator and denominator, one can quickly determine the limit. She outlines three cases: if the degree of the numerator is less than the denominator, the limit is 0; if they are equal, the limit is the ratio of the leading coefficients; and if the numerator's degree is greater, the limit is infinity or negative infinity depending on the leading terms.
🔢 Formal Method for Rational Expressions
Nancy presents the formal algebraic method for finding limits of rational expressions. She explains that by dividing each term by the highest power of x in the denominator, one can simplify the expression and find the limit. This method confirms the shortcut rules mentioned earlier and is useful for understanding the underlying algebraic principles.
📉 Limits of 1/x and (sin x)/x
This part of the video addresses the limits of 1/x and (sin x)/x as x approaches infinity or negative infinity. Nancy explains that the limit of 1/x, regardless of the power of x in the denominator, is 0. She also clarifies that the limit of (sin x)/x is 0 for both positive and negative infinity, using the property that sine of an expression over the same expression equals 0.
📚 Exponential Limits
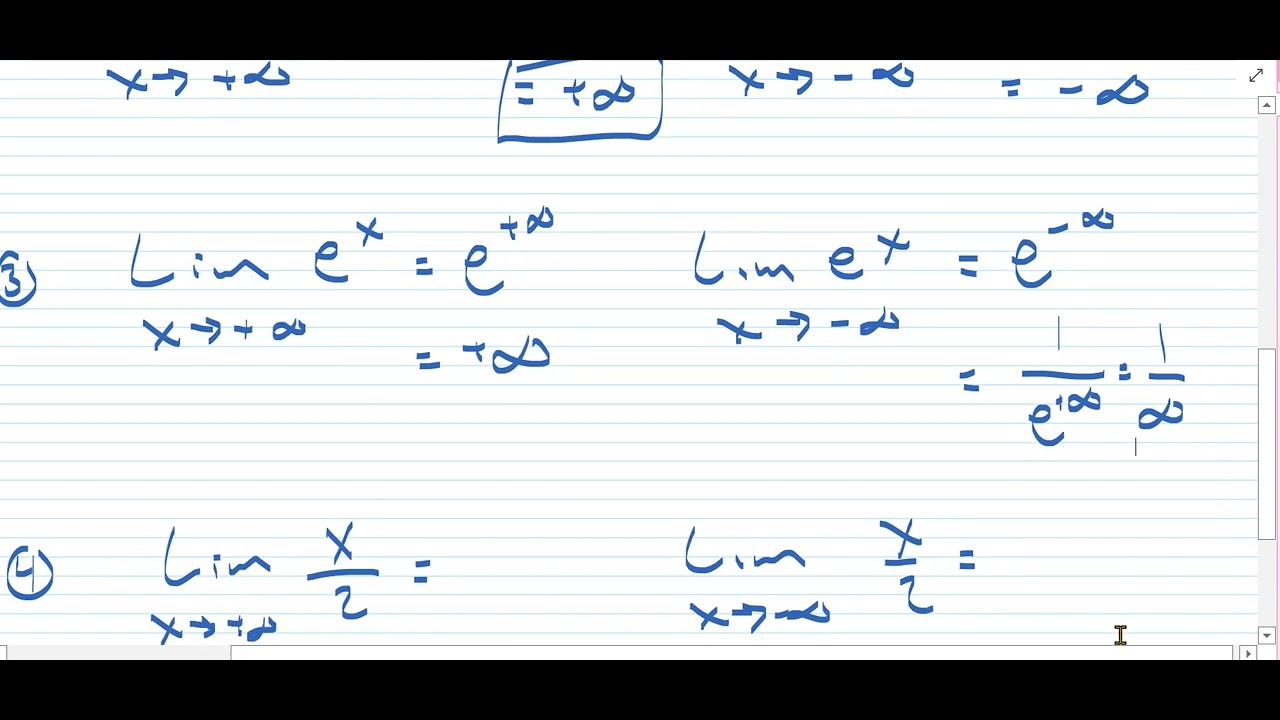
Nancy concludes the video by discussing exponential limits. She explains how to handle expressions with negative exponents by rewriting them with positive exponents and then applying the rules for limits at infinity. For e^(-2x) as x approaches infinity, the limit is 0, while for e^(2x) as x approaches negative infinity, the limit is also 0. This segment wraps up the various methods for finding limits at infinity.
🎉 Video Conclusion and Encouragement
In the final part of the video, Nancy wraps up the discussion on limits at infinity and encourages viewers to like and subscribe if they found the content helpful. She acknowledges that limits can be a challenging topic but emphasizes the importance of understanding the concepts presented.
Mindmap
Keywords
💡Limit
💡Polynomial
💡Rational Expression
💡Degree
💡Infinity
💡Negative Infinity
💡Leading Term
💡Sine Function
💡Exponential Function
💡Shortcut
Highlights
Introduction to limits at infinity and negative infinity, providing an overview of the topic and directing viewers to other related videos.
Explanation of how to handle polynomials or constants in limits as x approaches infinity or negative infinity, emphasizing the direct variation with x.
Illustration of the concept of degree in polynomials, explaining its relevance in determining the limit of rational expressions.
Shortcut method for finding limits of rational expressions based on comparing the degrees of the numerator and the denominator.
Three main cases for finding limits using the shortcut method, providing clear rules for each scenario.
Detailed explanation of how to apply the shortcut method for rational expressions with equal degrees in the numerator and denominator.
Discussion on the behavior of odd and even powers of x in polynomials when taking limits at infinity and negative infinity.
Explanation of the limit of constants, emphasizing that the limit is simply the constant value regardless of the value of x.
Formal algebraic method for finding the limit of rational expressions, providing a deeper understanding of the shortcut's basis.
Clarification on the limit of 1/x and 1/x^n as x approaches infinity or negative infinity, highlighting the tendency towards zero.
Property of (sin x)/x approaching zero as x goes to infinity or negative infinity, with examples of how to apply this property.
Explanation of how to handle exponential expressions with negative exponents when taking limits at infinity.
Strategy for finding the limit of e^(2x) as x approaches negative infinity, using the concept of negative powers.
Summary of the main points and methods discussed in the video, reinforcing the understanding of limits at infinity and negative infinity.
Encouragement for viewers to like and subscribe to the channel for more educational content on similar topics.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: