Calculus AB Homework 1.4 Limits Involving Infinity
TLDRThis educational video script covers the evaluation of limits involving infinity for 19 homework problems. The presenter demonstrates how to find limits for various expressions, including rational functions and exponentials, by using both algebraic manipulation and shortcuts based on leading terms. The script also explains how these limits relate to the existence of vertical and horizontal asymptotes, providing insights into the behavior of functions as they approach infinity or specific values.
Takeaways
- 📈 Evaluate the limit as X approaches infinity or negative infinity for rational functions.
- ✏️ Divide each term by the highest power of X to simplify rational expressions.
- 🧮 Use substitution to evaluate limits once the expression is simplified.
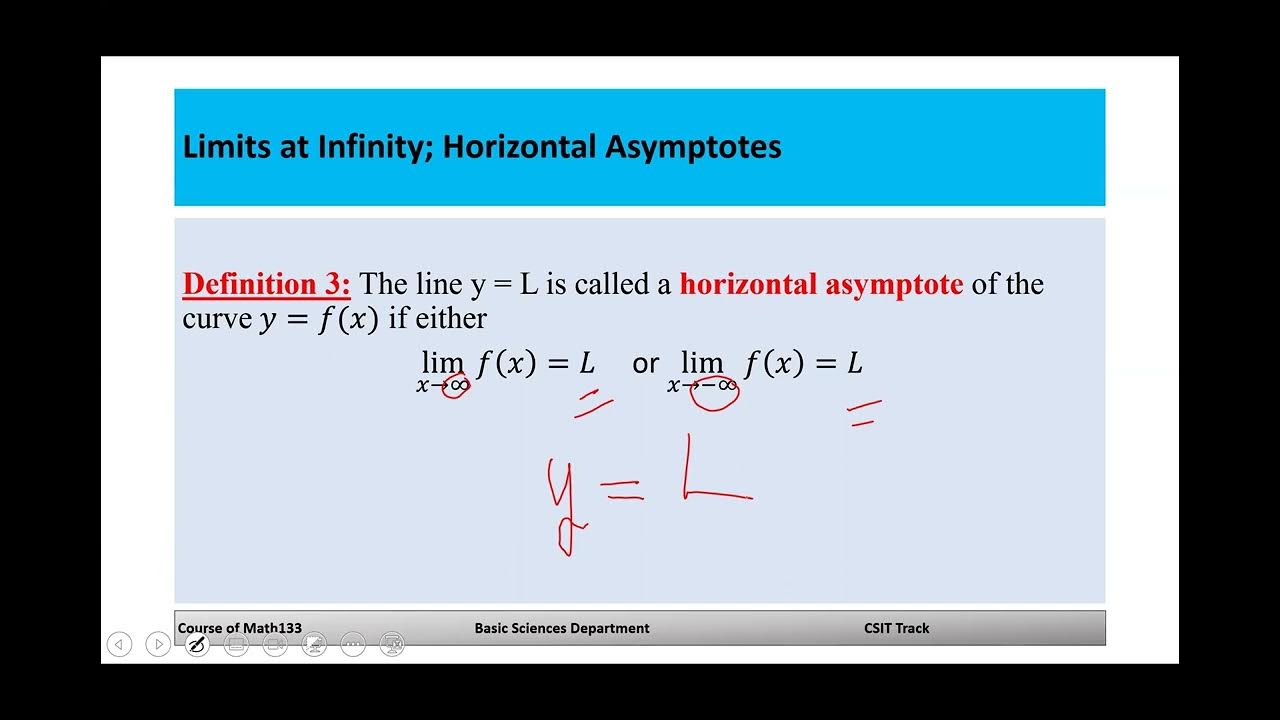
- 📉 Determine if a function has a horizontal asymptote by analyzing the limit as X approaches infinity.
- 🔗 Use the ratio of leading coefficients for rational functions to find limits as X approaches infinity.
- 🔄 A limit resulting in an infinite value indicates a vertical asymptote.
- 📊 Compare the degrees of the leading terms in the numerator and denominator to use shortcuts.
- 📝 Always check for the sign of the result when X approaches negative infinity.
- 📐 Limits approaching zero indicate the presence of a horizontal asymptote at Y=0.
- 🚫 Limits that do not yield finite values signify no horizontal asymptote.
Q & A
What is the main topic of the video?
-The main topic of the video is to work through unit 1 homework problems 27 through 46, which involve limits and their relation to vertical or horizontal asymptotes.
What is the first problem discussed in the video?
-The first problem discussed is finding the limit as X approaches infinity of the expression (1 - X) / (X - 2).
What is the long method used to evaluate the limit of the first problem?
-The long method involves rewriting the rational expression by dividing each term by the highest power of X and then evaluating the limit using substitution.
What is the shortcut method mentioned for evaluating limits as X approaches infinity or negative infinity?
-The shortcut method involves looking at the degree of the leading terms in the numerator and the denominator. If they have the same degree, the ratio of the leading coefficients determines the limit.
What does the limit of the first problem indicate about the graph of the function?
-The limit of the first problem indicates that there is a horizontal asymptote at y equals negative one, as the Y values approach negative one as X approaches infinity.
What is the limit of the function as X approaches negative infinity for problem 28?
-The limit of the function as X approaches negative infinity for problem 28 is zero, indicating a horizontal asymptote at y equals zero.
Why does the limit not exist for problem 29 as X approaches negative infinity?
-The limit does not exist for problem 29 because the function is unbounded, with the numerator growing at a faster rate than the denominator, resulting in the function approaching negative infinity.
What does the limit as X approaches 2 from the left-hand side in problem 30 indicate?
-The limit as X approaches 2 from the left-hand side indicates a vertical asymptote at x equals 2, as the function approaches negative infinity and does not have a finite limit.
What is the limit of the natural log of X plus 1 as X approaches infinity for problem 31?
-The limit of the natural log of X plus 1 as X approaches infinity is infinity, indicating that there is no horizontal asymptote for this function.
What is the significance of evaluating the limit from both the left and right-hand sides when determining the existence of vertical asymptotes?
-Evaluating the limit from both sides is significant because it provides information about the behavior of the function as it approaches a certain value from different directions, which helps in determining the existence and location of vertical asymptotes.
How does the video script handle the limit of exponential functions as X approaches infinity or negative infinity?
-The script handles the limit of exponential functions by considering the base of the exponent and its relation to 1. If the base is greater than 1, the function approaches infinity; if less than 1, it approaches 0. The sign of the exponent is also considered to determine the final limit value.
Outlines
📚 Calculus Limits and Asymptotes Introduction
The video begins with an introduction to solving calculus problems involving limits that approach infinity, focusing on determining the existence of vertical or horizontal asymptotes. The first problem involves finding the limit as X approaches infinity for the expression 1 - x / (X - 2). The presenter demonstrates both a detailed method of dividing by the highest power of X and a shortcut by examining the leading terms' coefficients, concluding with a horizontal asymptote at y = -1.
🔍 Analyzing Limits and Asymptotes Algebraically
The presenter continues with problem 28, which involves the limit as X approaches negative infinity for the expression X + 1 / (X^2 + 1). By dividing through by X^2 and substituting negative infinity, the limit is found to be zero, indicating a horizontal asymptote at y = 0. The explanation includes a shortcut based on the degrees of the leading terms, reinforcing the concept that a higher degree in the denominator results in a limit approaching zero.
🚫 Identifying Non-Existent Limits and Asymptotes
In problem 29, the limit as X approaches negative infinity for the expression X^3 + 1 / (3X^2 + 1) is explored. The presenter uses substitution and the highest power of X to show that the limit does not exist, as it approaches negative infinity, indicating no horizontal asymptote. A shortcut is mentioned, emphasizing the importance of the degrees of the leading terms in determining the behavior of the function at infinity.
📉 Limits Involving Finite Approaches and Vertical Asymptotes
Problem 30 discusses the limit as X approaches 2 from the left side for the expression 2 / (2 - X). The substitution of 2 for X results in an undefined expression, indicating a vertical asymptote at x = 2. The explanation clarifies that approaching a finite value with an infinite limit signifies a vertical asymptote.
📈 Limits of Logarithmic Functions and Their Behavior
The presenter tackles limits involving the natural logarithm function, such as the limit as X approaches infinity for ln(X) + 1, which is shown to be infinite with no horizontal asymptote. For X approaching 0, ln(X) + 1 results in a finite limit of 0, not indicating any asymptote. The importance of evaluating limits from both sides for horizontal asymptotes is highlighted, with a correction made for prematurely concluding the absence of a horizontal asymptote in a previous example.
📉 Further Exploration of Vertical Asymptotes in Logarithmic Functions
Problems involving the natural logarithm approaching specific values from the right-hand side are discussed, such as the limit as X approaches -1 for ln(-1) + 1, which is undefined due to the domain restriction of the ln function. The approach from the right-hand side results in values approaching negative infinity, indicating a vertical asymptote at x = -1.
🔢 Limits of Exponential and Polynomial Functions Near Infinity
The video script explores limits of exponential and polynomial functions as X approaches infinity and negative infinity, such as 3X - 2 / √(2X^2 + 1). The presenter uses leading coefficients to determine the limit, which is 3 / √2 for positive infinity and -3 / √2 for negative infinity, highlighting the importance of considering the rate of change and the signs of the numerator and denominator.
📚 Comprehensive Limits Analysis for Various Functions
The script concludes with a detailed analysis of several limit problems involving different types of functions, including exponential, logarithmic, and polynomial expressions. Each problem is approached with substitution and consideration of the leading terms' coefficients to determine the limit's behavior, whether it be finite, infinite, or undefined, and the implications for the existence of asymptotes.
Mindmap
Keywords
💡Limit
💡Infinity
💡Asymptote
💡Rational Expression
💡Leading Term
💡Degree of a Polynomial
💡Substitution
💡Indeterminate Form
💡Natural Logarithm
💡Exponential Function
Highlights
Introduction to solving limits involving infinity for unit 1 homework problems 27 to 46.
Explanation of how to evaluate limits and determine the existence of vertical or horizontal asymptotes.
Detailed walkthrough of problem 27, showcasing the method of dividing by the highest power of X.
Demonstration of the shortcut for rational expressions involving infinity by comparing leading terms.
Graphical interpretation of horizontal asymptotes and their mathematical representation.
Solution to problem 28 using algebraic division and the concept of leading term degrees.
Shortcut explanation for rational functions at infinity, emphasizing the importance of leading term degrees.
Analysis of problem 29, illustrating the absence of a horizontal asymptote due to the numerator's higher degree.
Problem 30's approach, discussing the concept of vertical asymptotes and their identification.
Problem 31's solution, emphasizing the natural log function's behavior as X approaches infinity.
Problem 33's walkthrough, identifying a vertical asymptote at x equals negative 1.
Problem 34's solution, involving the concept of approaching a finite value from the right-hand side.
Problem 35's approach, discussing indeterminate forms and the comparison of leading coefficients.
Problem 36's solution, applying the concept of approaching negative infinity and its effect on limits.
Problem 37's analysis, explaining the behavior of functions with degree 2 in the numerator and degree 1 in the denominator.
Problem 38's solution, discussing the impact of approaching negative infinity on the limit's existence.
Problem 39's walkthrough, illustrating the use of reciprocals for negative exponents and their limits.
Problem 40's solution, contrasting the behavior of the function at positive versus negative infinity.
Problem 41's approach, using substitution to evaluate limits with exponential functions.
Problem 42's solution, demonstrating the impact of adding constants to exponential functions at infinity.
Problem 43's walkthrough, showing the finite limit achieved by adding constants to exponential functions.
Problem 44's solution, discussing the behavior of e to the power of negative infinity.
Problem 45's approach, evaluating limits with exponential functions and their reciprocals.
Problem 46's solution, illustrating the limit of a function involving 1/2 raised to a power.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: