Estimating Function Values Using Differentials and Local Linearization | Calculus

The Organic Chemistry Tutor
7 Mar 201811:05
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the method of approximating mathematical expressions without a calculator using tangent line equations. It illustrates the process with examples of estimating 2.99 to the fourth power and the square root of 9.1. The script explains how to identify the function, calculate the first derivative to find the slope, and use the point-slope formula to approximate the values. The approximations are then compared with exact answers obtained from a calculator, demonstrating the effectiveness of the method.

Takeaways
  • 📈 The problem discussed is estimating 2.99 to the fourth power without a calculator.
  • 🔢 The expression is associated with the function f(x) = x to the fourth power.
  • 👉 A value close to 2.99, in this case, 3, is chosen for better approximation.
  • 🤝 The tangent line equation is used to approximate the value of the function at 2.99.
  • 📚 The point-slope form of the tangent line equation is y - y1 = m(x - x1).
  • 🧮 Calculation of y1 involves substituting the chosen value into the function, resulting in 81 for f(3).
  • 🔄 The slope m is found using the first derivative, which for f(x) = x^4 is 4x^3.
  • 🏁 The slope at x=3 is calculated to be 108 by substituting into the derivative.
  • 🔧 Plugging in 2.99 into the tangent line equation yields an approximation of 79.92.
  • 📊 The approximation is verified to be close to the exact answer of 79.9253.
  • 🌐 The method is also applied to estimate the square root of 9.1 and the natural log of 1.1.
  • 📈 The process involves identifying the function, finding the first derivative, and using the tangent line approximation for estimation.
  • 👌 The approximations for the square root of 9.1 and the natural log of 1.1 are also shown to be close to their exact values.
Q & A

  • What is the problem presented in the transcript?

    -The problem presented is to estimate the value of 2.99 raised to the fourth power without using a calculator.

  • How does the transcript suggest to approximate the value?

    -The transcript suggests using the tangent line equation at a point close to the value of interest, in this case, x=3, to approximate the value of 2.99 to the fourth power.

  • What is the function associated with the expression 2.99 to the fourth power?

    -The function associated with the expression is f(x) = x to the fourth power, where x is replaced by 2.99.

  • How is the slope of the tangent line calculated?

    -The slope of the tangent line is calculated using the first derivative of the function f(x) at the point x=3, which is 4x^3. The derivative at x=3 is found to be 108.

  • What is the estimated value of 2.99 to the fourth power as per the transcript?

    -The estimated value of 2.99 to the fourth power is approximately 79.92.

  • How close is the estimated value to the actual value?

    -The actual value is 79.9253, so the estimated value is very close to the exact answer.

  • What is the second problem discussed in the transcript?

    -The second problem is to estimate the square root of 9.1 without using a calculator.

  • What function and derivative are used for the second problem?

    -The function used is the square root of x, and the first derivative used is 1 over 2 times the square root of x.

  • What is the estimated value of the square root of 9.1?

    -The estimated value of the square root of 9.1 is approximately 3.016 repeating.

  • What is the actual value of the square root of 9.1?

    -The actual value of the square root of 9.1 is 3.016662.

  • How does the transcript suggest estimating the natural log of 1.1 without a calculator?

    -The transcript suggests using the tangent line approximation with the natural log function, where the function is ln(x) and the first derivative is 1/x. The point of tangency is chosen to be x=1, which simplifies the calculation.

  • What is the estimated value of the natural log of 1.1?

    -The estimated value of the natural log of 1.1 is approximately 0.1.

  • How close is the estimated value of the natural log of 1.1 to the actual value?

    -The actual value is 0.0953, which is quite close to the estimated value of 0.1.

Outlines
00:00
🔢 Estimating Powers and Roots without a Calculator

This paragraph introduces a method for approximating the result of raising a number to a power or finding the square root without using a calculator. The focus is on estimating 2.99 to the fourth power, which is less than 3 to the fourth power (81). The process involves defining a function, f(x) = x to the fourth power, and using the tangent line equation at a value close to the one we're interested in (x = 3). By calculating the first derivative to find the slope and using the point-slope formula, we can approximate the value of the function at x = 2.99, resulting in an estimate of 79.92. The method is then applied to estimate the square root of 9.1, using a similar approach with the square root function and its derivative. The paragraph emphasizes the usefulness of the tangent line approximation for these types of calculations.

05:02
📈 Tangent Line Approximation for Square Roots

This paragraph delves deeper into the application of tangent line approximation for estimating square roots, specifically focusing on the square root of 9.1. It begins by identifying the function f(x) as the square root of x and calculating the first derivative to find the slope at x = 9. Using the point-slope formula, the tangent line equation is derived with x1 = 9, y1 = 3 (the square root of 9), and m = 1/6 (the slope). The equation is then used to estimate the square root of 9.1 by plugging in x = 9.1 and simplifying the result to get an approximation of 3.016 repeating. The paragraph concludes by comparing this approximation to the exact value obtained using a calculator, demonstrating the close accuracy of the method.

10:05
📊 Approximating Natural Logarithms without a Calculator

The final paragraph of the script focuses on estimating the natural logarithm of 1.1 without a calculator. The natural logarithm function, ln(x), and its first derivative, 1/x, are introduced. The best value to use for x is 1, as the natural log of 1 is zero, making y1 = 0. With the slope m equal to 1 (since the derivative at x = 1 is 1/1), the tangent line approximation simplifies to x - 1. By substituting x = 1.1 into this approximation, the natural logarithm of 1.1 is estimated to be approximately 0.1. The paragraph ends by comparing this estimate to the actual value calculated using a calculator, which is 0.0953, showing that the approximation is reasonably close and highlighting the practicality of the method for quick estimations.

Mindmap
Keywords
💡Estimation
Estimation refers to the process of approximating a value or quantity without exact calculation. In the context of the video, estimation is used to find the approximate values of mathematical expressions like 2.99 raised to the fourth power and the natural log of 1.1 without using a calculator. It is a valuable skill that allows for quick calculations and a general understanding of numerical results.
💡Function
A function in mathematics is a relation that assigns a single output value to each input value. In the video, functions are used to describe the mathematical relationships between variables, such as f(x) = x^4 for the fourth power and ln(x) for the natural logarithm. Functions are essential for understanding the behavior of these mathematical expressions and for performing estimations.
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point and has the same slope as the curve at that point. In the video, the tangent line is used as an approximation tool to find the value of a function near a specific point. By calculating the slope of the function at a point and using the point-slope form of a line, one can estimate the value of the function at nearby points.
💡First Derivative
The first derivative of a function represents the rate of change or the slope of the function at a specific point. It is a fundamental concept in calculus and is used in the video to find the slope of the tangent line for estimation purposes. The first derivative provides insight into how a function behaves and changes as its input varies.
💡Point-Slope Form
The point-slope form is a linear equation that describes the relationship between the slope of a line and a point on that line. It is given by the formula y - y1 = m(x - x1), where m is the slope, and (x1, y1) is a known point on the line. In the video, the point-slope form is used to create the tangent line at specific points to approximate the values of functions.
💡Power Rule
The power rule is a fundamental calculus formula that allows the differentiation of functions involving exponents. It states that the derivative of x^n, where n is a constant, is nx^(n-1). This rule is essential for finding the first derivative of functions like x^4 or x^3, as demonstrated in the video.
💡Natural Logarithm
The natural logarithm, denoted as ln(x), is the logarithm to the base e (approximately 2.71828) of x. It is a key concept in mathematics, particularly in calculus, and is used to model various natural phenomena and mathematical relationships. In the video, the natural logarithm is used to estimate the value of ln(1.1) without a calculator.
💡Slope
The slope of a line represents the rate of change of a function with respect to its independent variable. It indicates how steep or gradual a line is and is a fundamental concept in understanding linear relationships. In the video, the slope is calculated for the tangent line at specific points to estimate the values of functions like x^4 and the square root of x.
💡Approximation
Approximation is the process of finding a value that is close to, but not exactly, the true value of a mathematical expression. It is often used when exact calculations are not feasible or when a quick estimate is needed. In the video, approximation techniques are used to estimate the values of various mathematical expressions without the use of a calculator.
💡Calculus
Calculus is a branch of mathematics that deals with the study of change and motion, primarily through the use of limits, derivatives, and integrals. It is a powerful tool for analyzing and solving problems in various fields, including physics, engineering, and economics. In the video, calculus concepts like derivatives and tangent lines are used to approximate values of functions.
💡Exact Value
The exact value refers to the precise and accurate numerical result of a mathematical expression or calculation. In contrast to approximations, exact values do not involve any estimation or rounding. In the video, exact values are obtained using a calculator to validate the approximations made without it.
Highlights

Estimating 2.99 raised to the fourth power without a calculator using the tangent line approximation method.

The process begins by identifying the function associated with the expression, which in this case is f(x) = x to the fourth power.

Choosing a value for x close to 2.99, which is 3, to simplify the approximation.

Using the point-slope formula to write the tangent line equation at x equals three.

Calculating y1 as 81, which is 3 to the fourth power.

Finding the slope (m) using the first derivative, which is 4 x to the third power, resulting in m = 108 when x is 3.

Plugging the values into the tangent line equation to get an approximation of 2.99 to the fourth power.

The estimated value of 2.99 to the fourth power is approximately 79.92.

Comparing the estimated value with the exact answer (79.9253) to demonstrate the accuracy of the approximation method.

Estimating the square root of 9.1 using a similar tangent line approximation method.

Identifying the function as the square root of x and finding its first derivative.

Choosing x = 9 for the approximation of the square root of 9.1.

Calculating the slope (m) as 1/6 when x is 9.

Using the tangent line equation to estimate the square root of 9.1, resulting in an approximation of 3.016 repeating.

Comparing the estimated value (3.016 repeating) with the exact answer (3.016662) to show the effectiveness of the method.

Estimating the natural log of 1.1 without a calculator by using the tangent line approximation.

Identifying the function as ln(x) and its first derivative as 1/x.

Choosing x = 1 for the approximation of ln(1.1) due to its proximity to 1.

Calculating the slope (m) as 1 since the first derivative of ln(x) when x is 1 is 1.

Using the tangent line approximation to estimate ln(1.1) as approximately 0.1.

Comparing the estimated value (0.1) with the exact answer (0.0953) to illustrate the close approximation.

Transcripts

Browse More Related Video

Math

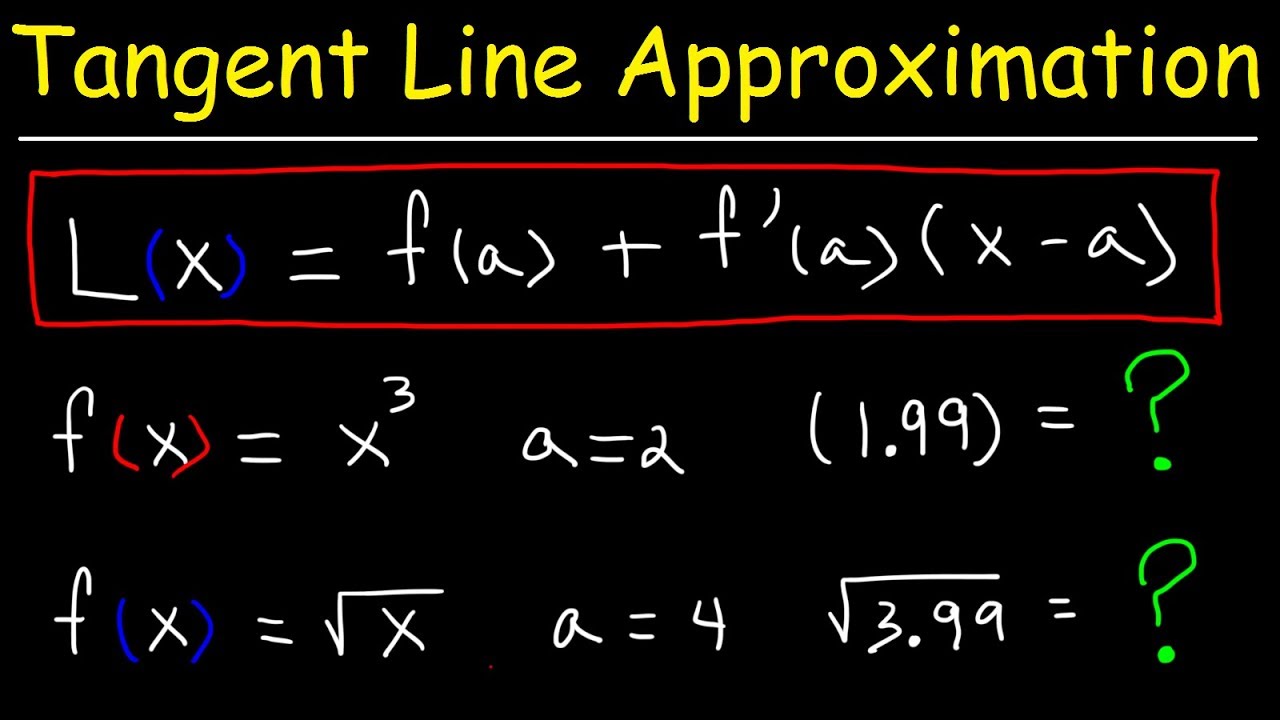
Finding The Linearization of a Function Using Tangent Line Approximations

Linear Approximations | Using Tangent Lines to Approximate Functions

Euler's Method (introduction & example)

Calculus - Finding the derivative at a point using a Ti-83 or 84 calcululator

How To Find The Equation of a Tangent Line Using Derivatives - Calculus 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Math ApproximationNo CalculatorReal World ExamplesProblem SolvingFunctions UnderstandingDerivatives ApplicationEducational ContentCritical ThinkingMathematical ConceptsAnalytical Skills