How To Find The Equation of a Tangent Line Using Derivatives - Calculus 1
TLDRThis video tutorial explains the process of finding the equation of a tangent line to a curve at a specific point. It emphasizes the need for the curve's point of tangency (x, y coordinates) and the slope of the tangent line, which is derived from the function's first derivative. The video demonstrates the application of the point-slope formula and the conversion from point-slope to slope-intercept form. It also reviews basic calculus rules, such as the power rule and the product rule, to calculate derivatives. The process is illustrated with two examples, providing a clear guide for viewers to understand how to find tangent line equations.
Takeaways
- 📌 A tangent line is a line that touches a curve at exactly one point.
- 🔍 To find the equation of a tangent line, you need the point of tangency (x, y coordinates) and the slope of the tangent line.
- 📈 The slope of the tangent line can be found by taking the first derivative of the function and evaluating it at the x coordinate of the tangency point.
- 📝 The general formula for the equation of a tangent line is given by the point-slope formula: y - y1 = m(x - x1), where m is the slope and (x1, y1) is the point of tangency.
- 🌟 For the given example, the point of tangency is (1, 12) and the slope is calculated to be 37 using the first derivative of the function f(x) = 4x^3 + 5x^2 + 9x.
- 🔄 The equation of the tangent line can be rewritten in slope-intercept form (y = mx + b) by isolating the y variable.
- 📊 For the second example involving f(x) = x^3 * ln(x), the point of tangency is (1, 0) with a slope of 1, obtained by applying the product rule for derivatives.
- 👉 The equation of the tangent line for the second example simplifies to y = x - 1 using the point-slope formula.
- 💡 Understanding the power rule and product rule for derivatives is crucial for calculating the slope of tangent lines.
- 📚 Reviewing basic derivative techniques such as the natural log function, product rule, quotient rule, and chain rule can be helpful in solving these problems.
Q & A
What is a tangent line in the context of the video?
-A tangent line is a line that touches a curve at exactly one point, representing the slope of the curve at that specific point.
What are the two key components needed to find the equation of a tangent line?
-The two key components needed are the x and y coordinates of the point where the tangent line touches the curve, and the slope of the tangent line.
How is the slope of a tangent line found?
-The slope of a tangent line is found by taking the first derivative of the function and evaluating it at the x coordinate of the point that touches the curve.
What is the power rule for derivatives mentioned in the video?
-The power rule states that the derivative of a variable raised to a constant is the constant multiplied by the variable raised to the power of (n-1), where n is the original exponent.
What is the point-slope formula used to write the equation of a tangent line?
-The point-slope formula is y - y1 = m(x - x1), where m is the slope of the line, and (x1, y1) are the coordinates of the point where the tangent line touches the curve.
How do you convert the point-slope form of a line to the slope-intercept form?
-To convert the point-slope form to the slope-intercept form, isolate the y variable by distributing the slope (m) and then adding the y-intercept (b), which is calculated by rearranging the equation.
What is the function given in the second example of the video?
-The function given in the second example is f(x) = x^3 * ln(x), where x is the independent variable and ln(x) represents the natural logarithm of x.
How is the y-coordinate (y1) of the tangent line's point found?
-The y-coordinate (y1) is found by evaluating the original function f(x) at the given x-coordinate (x1).
What derivative technique is used to find the slope of the tangent line in the second example?
-The product rule is used to find the derivative of the function f(x) = x^3 * ln(x), which helps in determining the slope of the tangent line at the given x-coordinate.
What is the final equation of the tangent line in slope-intercept form for the second example?
-The final equation of the tangent line in slope-intercept form for the second example is y = x - 1.
How can one review derivative techniques such as natural log functions and the product rule?
-One can review derivative techniques by checking out the links in the description section of the video or watching other videos posted by the creator that cover these topics in more detail.
Outlines
📚 Introduction to Tangent Lines and Derivatives
This paragraph introduces the concept of a tangent line, which is a line that touches a curve at exactly one point. It emphasizes the need for two key pieces of information to find the equation of a tangent line: the point of tangency (x and y coordinates) and the slope of the tangent line. The slope is determined by taking the first derivative of the function and evaluating it at the x coordinate of the tangency point. The paragraph also reviews basic derivative rules, such as the power rule for variables raised to a constant, and applies these rules to find the derivative of a given function. The process of calculating the slope of the tangent line by plugging in the x value of the point of tangency is explained, culminating in the determination of the tangent line's equation using the point-slope formula.
📈 Converting to Slope Intercept Form
This paragraph focuses on converting the equation of a tangent line from point-slope form to slope intercept form (y = mx + b). It explains the process of isolating the y variable in the equation, which involves distributing the slope value and adding the y-intercept to both sides of the equation. The paragraph then applies this process to find the equation of a tangent line for a given function at a specific x value. It also encourages viewers to practice the problem and provides additional resources for reviewing derivative techniques such as the product rule, chain rule, and natural log functions.
🔢 Solving for Tangent Line Equation with Product Rule
The final paragraph delves into solving for the equation of a tangent line using the product rule for derivatives. It demonstrates how to calculate the y-coordinate of the point of tangency by evaluating the original function at the given x coordinate. The paragraph then explains the process of finding the derivative of a function that involves a product of two terms, highlighting the use of the product rule. The calculation of the slope of the tangent line is detailed, with the natural log function's derivative being a key component. The paragraph concludes by applying the point-slope formula to derive the equation of the tangent line, simplifying it to its final form, and summarizing the overall process for finding the equation of a tangent line.
Mindmap
Keywords
💡Tangent Line
💡First Derivative
💡Point-Slope Formula
💡Slope Intercept Form
💡Product Rule
💡Natural Logarithm
💡Power Rule
💡Constant Multiple Rule
💡Derivative Techniques
💡Curve
💡Point of Tangency
Highlights
The video discusses the method for finding the equation of a tangent line to a curve.
A tangent line is defined as a line that touches a curve at exactly one point.
To find the equation of a tangent line, you need the point of tangency (x, y coordinates) and the slope of the tangent line.
The slope of the tangent line can be found by taking the first derivative of the function and evaluating it at the x coordinate of the tangency point.
The point-slope formula is used to write the equation of the tangent line once you have the slope and the point of tangency.
The derivative of x to the n (where n is a constant) is given by the power rule: n * x to the (n-1).
The derivative of x raised to the fourth power is 4 * x cubed.
The derivative of x cubed is 3 * x squared.
The derivative of x squared is 2 * x.
The derivative of a constant is zero.
The first derivative of the given function is calculated to find the slope of the tangent line.
The slope of the tangent line in the example is found to be 37 by plugging in the x value of the tangency point.
The equation of the tangent line can be written in point-slope form or slope-intercept form (y = mx + b).
The process for finding the equation of a tangent line is demonstrated with a specific example where the function is f(x) = x^4 + 5x^3 + 9x^2.
For the second problem, the function is f(x) = x^3 * ln(x) and the tangent line is found at x = 1.
The y-coordinate of the tangency point for the second problem is found by evaluating the original function at x = 1, resulting in y1 = 0.
The derivative for the second problem is found using the product rule: f'(x) = 3x^2 * ln(x) + x^3 * (1/x).
The slope of the tangent line for the second problem is calculated to be 1 by evaluating the derivative at x = 1.
The final equation of the tangent line for the second problem is simplified to y = x - 1 using the point-slope formula.
The video provides a comprehensive guide on how to find the equation of a tangent line, including the necessary calculus concepts and steps.
Transcripts
Browse More Related Video
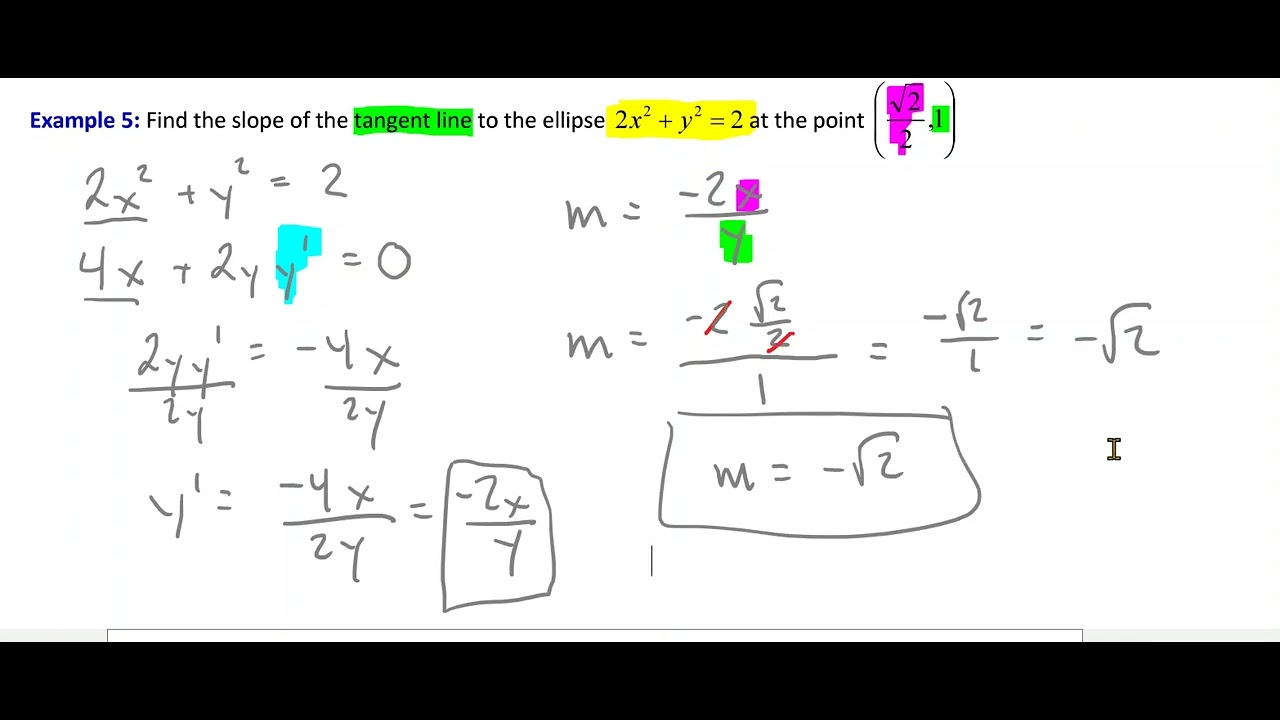
Implicit Differentiation - Finding Equation of Tangent Line
How To Find The Equation of The Tangent Line With Derivatives
How To Find The Equation of the Normal Line
Tangent Line to a Polynomial | MIT 18.01SC Single Variable Calculus, Fall 2010

How to Find the Equation of a Tangent Line
Equation of a normal line [IB Maths AI SL/HL]
5.0 / 5 (0 votes)
Thanks for rating: