L'hopital's rule

The Organic Chemistry Tutor
5 Mar 201813:09
EducationalLearning
32 Likes 10 Comments

TLDRThe transcript discusses the application of L'Hopital's Rule in evaluating limits of indeterminate forms, particularly as x approaches infinity or 0. It explains the process of differentiating the numerator and denominator to simplify expressions and find limits. Examples are provided to illustrate how direct substitution can lead to indeterminate forms like 0/0 or โˆž/โˆž, and how L'Hopital's Rule, along with natural logarithms, can be effectively used to find the actual limits, demonstrating the mathematical concepts with clarity and precision.

Takeaways
  • ๐Ÿ“š The concept of limits and how to evaluate them using direct substitution and L'Hopital's Rule is discussed.
  • ๐Ÿ”ข When direct substitution results in an indeterminate form (e.g., infinity/infinity or 0/0), L'Hopital's Rule can be applied.
  • ๐Ÿ“ˆ L'Hopital's Rule states that the limit of a ratio of two functions can be found by taking the limit of the ratio of their derivatives, provided the limit exists.
  • ๐Ÿค” The video provides examples of evaluating limits as x approaches infinity and as x approaches 0, showcasing different scenarios.
  • ๐ŸŒŸ The first example demonstrates evaluating the limit of x^2/e^x as x approaches infinity, resulting in 0 using L'Hopital's Rule.
  • ๐Ÿ“Š The second example involves the limit of ln(x)/x as x approaches infinity, which also results in 0 by applying L'Hopital's Rule.
  • ๐Ÿ‘‰ The third example shows how to evaluate the limit of sin(7x)/sin(4x) as x approaches 0, leading to a final answer of 7/4.
  • ๐Ÿงฎ The fourth example calculates the limit of sin(8x)/3x as x approaches 0, yielding a result of 8/3.
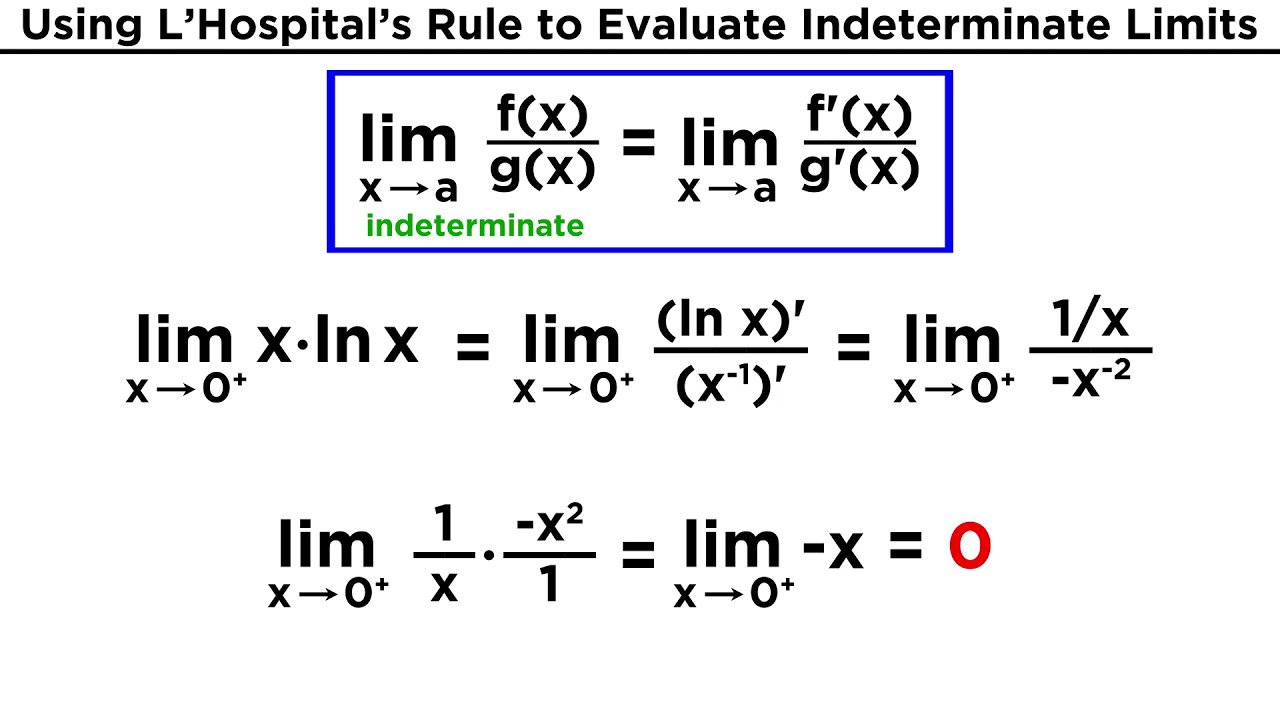
  • ๐ŸŒ The video also addresses the limit of x*ln(x) as x approaches infinity, which is found to be infinity.
  • ๐Ÿ”„ A special case is presented where the original function is rewritten (e.g., x^(1/x)) and the natural log transformation is used to find the limit as x approaches infinity.
  • ๐Ÿ”ง The use of L'Hopital's Rule is demonstrated with clear steps, including differentiating the numerator and denominator, applying the rule, and using direct substitution to find the limit.
Q & A

  • What is the indeterminate form when approaching infinity by direct substitution in the given script?

    -The indeterminate form in the script is when the limit of a function as x approaches infinity results in infinity divided by infinity, which does not provide a clear value.

  • What is the basic idea of L'Hopital's Rule mentioned in the transcript?

    -The basic idea of L'Hopital's Rule is that the limit of a fraction as x approaches a certain value can be found by taking the limit of the ratio of the derivatives of the numerator and the denominator functions.

  • How does the derivative of x squared change when using L'Hopital's Rule in the first example of the script?

    -The derivative of x squared changes to 2x when using L'Hopital's Rule in the first example. This is because the derivative of x^n is n*x^(n-1), so for x^2, it becomes 2*x^(2-1) or 2x.

  • What is the result of the limit as x approaches infinity for the expression ln(x)/x as explained in the script?

    -The result of the limit as x approaches infinity for the expression ln(x)/x is 0. This is because the derivative of ln(x) is 1/x, and the derivative of x is 1, leading to an expression of 1/infinity which evaluates to 0.

  • How does the script demonstrate the use of L'Hopital's Rule for the limit as x approaches 0 of sine(7x)/sine(4x)?

    -The script demonstrates the use of L'Hopital's Rule by finding the derivatives of the numerator and the denominator, which are cosine(7x) and cosine(4x) multiplied by 4, respectively. After applying the rule, the expression simplifies to (cosine(0) * 7) / (cosine(0) * 4), which evaluates to 7/4.

  • What is the limit as x approaches 0 of (1 - 2x)^(1/x) in the context of the script?

    -The limit as x approaches 0 of (1 - 2x)^(1/x) is e^(-2), which can also be written as 1/e^2. This is found by applying L'Hopital's Rule after taking the natural logarithm of both sides and simplifying the expression.

  • How does the script explain the evaluation of the limit as x approaches infinity for the expression x*ln(x)?

    -The script explains that the limit as x approaches infinity for the expression x*ln(x) is infinity, because both x and ln(x) approach infinity, and the product of two large numbers remains infinity.

  • What is the process used in the script to evaluate the limit of x^(1/x) as x approaches infinity?

    -The script uses a combination of setting the expression equal to y, taking the natural logarithm of both sides, and then applying L'Hopital's Rule to the transformed expression. The limit is then found by evaluating the natural logarithm of y, which simplifies to 1, and thus y equals e^0, which is 1.

  • How does the script illustrate the concept of direct substitution in evaluating limits?

    -The script illustrates the concept of direct substitution by showing that when plugging in values directly into the function, one can find the limit in certain cases, such as when a fixed number is divided by infinity, which results in 0.

  • What is the significance of the table of values presented in the script for the expression x^(1/x) as x approaches infinity?

    -The table of values in the script demonstrates the behavior of the expression x^(1/x) as x becomes larger, approaching infinity. It shows that the value of y, which represents the expression, gets closer to 1 as x increases, confirming the limit is indeed 1.

  • How does the script handle the evaluation of the limit as x approaches 0 for the function 1 - (2x)^(1/x)?

    -The script handles this by first setting the function equal to y, taking the natural logarithm of both sides, and then applying L'Hopital's Rule to the transformed expression. The limit is found by evaluating the expression after simplification, which results in ln(y) being equal to -2, and thus y equals e^(-2).

Outlines
00:00
๐Ÿ“š Introduction to Evaluating Limits with Indeterminate Forms

This paragraph introduces the concept of evaluating limits as x approaches infinity for certain mathematical expressions. It presents a scenario where direct substitution results in an indeterminate form, such as infinity divided by infinity. To resolve this, the paragraph explains the use of L'Hopital's Rule, which involves taking the derivatives of the numerator and the denominator separately. The process is demonstrated through the example of the expression x^2 / e^x, where after applying L'Hopital's Rule and direct substitution, the limit is found to be zero. The explanation is clear and provides a solid foundation for understanding how to deal with indeterminate forms in limit calculations.

05:01
๐Ÿ”ข Applying L'Hopital's Rule to Different Scenarios

This paragraph delves into applying L'Hopital's Rule to various limit scenarios. It begins with evaluating the limit of ln(x)/x as x approaches infinity, which also results in an indeterminate form. After differentiating the numerator and the denominator, the limit is found to be zero. The paragraph then moves on to evaluate the limit of sine(7x)/sine(4x) as x approaches zero, using L'Hopital's Rule to find the derivative of the functions and substituting to get a final answer of 7/4. The explanation is methodical and emphasizes the importance of L'Hopital's Rule in dealing with indeterminate forms, providing a clear understanding of the process and its application to different types of limits.

10:02
๐ŸŒŸ Advanced Techniques for Evaluating Limits

This paragraph explores more advanced techniques for evaluating limits, particularly when dealing with indeterminate forms. It starts with the limit of x * ln(x) as x approaches infinity, where direct substitution confirms the result as infinity. The paragraph then addresses a more complex example involving x^(1/x) as x approaches infinity, which requires a different approach. By setting the expression equal to y and taking the natural log of both sides, the paragraph applies L'Hopital's Rule and direct substitution to find that the limit is 1. The explanation is detailed and shows how to adapt the process for different types of limit problems, reinforcing the versatility of L'Hopital's Rule and the importance of understanding the underlying concepts.

๐Ÿง  Solving Limits with Natural Logarithms and Substitution

The final paragraph focuses on solving limits that involve natural logarithms and substitution. It presents the problem of finding the limit of (1 - 2x)^(1/x) as x approaches 0. By setting the expression equal to y and applying the natural log, the paragraph uses L'Hopital's Rule to find the derivative of the inside function and solve for y. The process is shown to yield the result of e^(-2), which can also be written as 1/e^2. The explanation is thorough and demonstrates the application of substitution and logarithmic properties in evaluating limits, providing a comprehensive understanding of the techniques involved.

Mindmap
Keywords
๐Ÿ’กLimit
In the context of the video, a limit refers to the value that a function approaches as the input (x) gets arbitrarily close to a certain point (either a specific number or infinity). It is a fundamental concept in calculus for understanding the behavior of functions. For example, when evaluating the limit as x approaches infinity for the expression x squared over e^x, the video explains that direct substitution results in an indeterminate form, prompting the use of more advanced techniques like L'Hopital's rule.
๐Ÿ’กDirect Substitution
Direct substitution is a method used in calculus where one simply plugs the value of the input (x) into the function to find the output. However, it may not always work, especially when dealing with limits at infinity or zero, resulting in indeterminate forms. The video illustrates this by attempting direct substitution for various limit problems, which often leads to expressions like infinity over infinity or zero over zero.
๐Ÿ’กIndeterminate Form
An indeterminate form occurs when the direct substitution of values into a function results in an undefined expression, such as 0/0 or infinity/infinity. These forms indicate that the function is not well-behaved at the point of interest, and alternative methods, like L'Hopital's rule, must be used to evaluate the limit.
๐Ÿ’กL'Hopital's Rule
L'Hopital's rule is a powerful tool in calculus that helps to evaluate limits when direct substitution results in an indeterminate form. It states that if the limit of a ratio of two functions is of the form 0/0 or infinity/infinity, then the limit of that ratio is equal to the limit of the ratio of their derivatives. This rule is used to simplify the expression and find the actual limit.
๐Ÿ’กDerivative
A derivative in calculus represents the rate of change or the slope of a function at a particular point. It is used to find the tangent line to a curve at a given point and is a crucial concept when applying L'Hopital's rule, as it involves taking the derivative of the numerator and denominator of a function to evaluate limits.
๐Ÿ’กInfinity
Infinity is a concept that represents an unbounded quantity, larger than any real number. In calculus, infinity is often used when describing the behavior of functions as their input approaches extremely large or small values. Evaluating limits involving infinity requires special techniques, such as L'Hopital's rule, to handle the resulting indeterminate forms.
๐Ÿ’กNatural Logarithm (ln)
The natural logarithm, denoted as ln, is the logarithm to the base e (where e is a mathematical constant approximately equal to 2.71828). It is a key function in calculus and is used to model various natural phenomena. In the video, the natural logarithm is used in multiple examples to evaluate limits as x approaches either zero or infinity.
๐Ÿ’กChain Rule
The chain rule is a fundamental calculus technique used to find the derivative of a composite function. It states that the derivative of a function composed of multiple functions is the derivative of the outer function times the derivative of the inner function. The chain rule is particularly useful when dealing with more complex functions involving multiple operations or functions nested within each other.
๐Ÿ’กZero
Zero is a unique value in mathematics; it is the absence of quantity and serves as the additive identity. In the context of limits, functions approaching zero can exhibit interesting behaviors, such as approaching zero themselves or becoming undefined. Zero is also used as a reference point for evaluating limits as x approaches it from the right or left.
๐Ÿ’กSine Function
The sine function is a trigonometric function that models the ratio of the opposite side to the hypotenuse in a right-angled triangle or the y-coordinate of a point on the unit circle. It oscillates between -1 and 1 and is periodic with a period of 2ฯ€. In the video, the sine function is used in examples to evaluate limits as x approaches specific values, often resulting in indeterminate forms that require further analysis.
๐Ÿ’กCosine Function
The cosine function is a trigonometric function that models the ratio of the adjacent side to the hypotenuse in a right-angled triangle or the x-coordinate of a point on the unit circle. It is also periodic with a period of 2ฯ€ and oscillates between -1 and 1. The cosine function is used in the video to find the derivatives of the sine function in limit problems, as the derivative of sine is the cosine.
Highlights

The concept of evaluating limits as x approaches infinity using different methods, such as direct substitution and L'Hopital's rule.

The indeterminate form of infinity over infinity and its evaluation using L'Hopital's rule.

The process of differentiating the numerator and denominator separately to simplify limits that appear indeterminate.

The application of L'Hopital's rule to the function f(x) = x^2 / e^x, resulting in a limit of zero.

The evaluation of the limit for the function g(x) = ln(x) / x using L'Hopital's rule, which also results in a limit of zero.

The use of L'Hopital's rule to evaluate the limit of sine 7x / sine 4x as x approaches 0, yielding a result of 7/4.

The method of applying L'Hopital's rule to the function h(x) = sine(8x) / 3x, leading to a limit of 8/3.

The evaluation of the limit for the function i(x) = x * ln(x) as x approaches infinity, resulting in infinity.

The unique approach of using L'Hopital's rule for the function j(x) = x^(1/x) by first taking the natural log of both sides.

The process of finding the limit for j(x) by taking the natural log, applying L'Hopital's rule, and then exponentiating the result to find the limit is 1.

The verification of the limit for j(x) by creating a table with values approaching infinity and observing the result approaches 1.

The application of L'Hopital's rule to the function k(x) = (1 - 2x)^(1/x) as x approaches 0, resulting in a limit of e^(-2).

The step-by-step process of using L'Hopital's rule, including setting the function equal to y, taking natural logs, and differentiating to find the limit.

The demonstration of how L'Hopital's rule can be applied to various types of indeterminate forms to find the actual limits.

The practical application of L'Hopital's rule in evaluating limits, showcasing its importance in calculus.

Transcripts

Browse More Related Video

Introduction to l'Hรดpital's rule | Derivative applications | Differential Calculus | Khan Academy

Understanding Limits and L'Hospital's Rule

Applying L'Hopital's Rule to Exponential Indeterminate Forms

Limits and Absolute Value

L'Hรดpital's Rule

Limit examples (part 3) | Limits | Differential Calculus | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus TechniquesLimits EvaluationL'Hopital's RuleDirect SubstitutionIndeterminate FormsInfinite LimitsDerivative ApplicationsMathematical AnalysisAdvanced MathEducational Content