Limit examples (part 3) | Limits | Differential Calculus | Khan Academy
TLDRThe video script discusses the concept of limits in calculus, demonstrating how to evaluate limits as x approaches specific values. It emphasizes the importance of simplifying expressions to avoid indeterminate forms like 0/0 and illustrates this with examples. The script also explores the behavior of limits as x approaches infinity, highlighting that the limit is determined by the fastest-growing terms in the numerator and denominator. The presentation aims to provide intuitive understanding and encourages viewers to use graphing calculators for further exploration.
Takeaways
- 📝 When dealing with limits, substitution of the limit value into the function is a good starting point.
- 🔍 If substitution results in 0/0, look for ways to simplify the expression by factoring and canceling out common terms.
- 🌟 Factoring is a powerful technique to use in limits when dealing with polynomials, as it may reveal common factors to cancel.
- ⚠️ It's important to remember that while substitution can give you the value of the limit, it doesn't define the function at the point of discontinuity.
- 📈 Visualizing limits can be aided by graphing functions to see how the function behaves as it approaches the limit value.
- 🤔 When faced with 0/0 after simplification, it's an indication that the limit exists, but the function is undefined at that point.
- 🔢 For limits involving infinity, consider the fastest-growing terms in the numerator and denominator to determine the behavior of the expression.
- 💡 As x approaches infinity, the behavior of a rational function is dictated by the highest power of x in both the numerator and the denominator.
- 📊 Large values of x in limit problems can make smaller terms insignificant, focusing only on the fastest-growing terms.
- 🎯 When the fastest-growing terms in the numerator and denominator are different, the limit is the ratio of the coefficients of those terms.
Q & A
What is the first step the speaker suggests when solving limit problems?
-The first step the speaker suggests is to substitute the number into the function and see if it yields a sensible result, which would indicate that the function is continuous at that point.
What happens when the speaker substitutes 3 into the given function?
-When the speaker substitutes 3 into the numerator, they get 3 squared minus 6 times 3 plus 9, which equals 0. The denominator also becomes 0, resulting in an indeterminate form of 0/0.
How does the speaker suggest simplifying the expression to deal with the 0/0 indeterminate form?
-The speaker suggests factoring the numerator and the denominator to find a common factor that can be canceled out, simplifying the expression and making it easier to evaluate the limit.
What is the limit of the simplified expression when x approaches 3?
-After simplifying the expression and canceling out the common factors, the speaker finds that the limit as x approaches 3 is 0, since the simplified expression becomes 0/6, which equals 0.
Why is it important to remember that the expression is not defined at x equals 3?
-It's important because even though the limit exists and is equal to 0 when x approaches 3, the function itself is undefined at that point, meaning it does not have a value there.
What does the speaker suggest as a method for evaluating limits as x approaches infinity?
-The speaker suggests thinking about what happens when x takes on very large values and considering the fastest-growing terms in the numerator and denominator to determine the limit as x approaches infinity.
How does the speaker determine the limit of x squared plus 3 over x to the third as x approaches infinity?
-The speaker determines that the limit approaches 0 because the fastest-growing term in the denominator, x to the third, grows much faster than the x squared term in the numerator, resulting in the fraction becoming smaller as x increases.
What is the limit of the expression x squared plus x over 4x squared minus 5 as x approaches infinity?
-The limit is 3/4. The speaker explains that the fastest-growing terms in the numerator and the denominator are the x terms, which grow at the same rate, leaving the ratio 3/4 as the limit.
What does the speaker advise for further understanding of limit problems?
-The speaker advises using a graphing calculator to visualize the functions and their limits, as well as creating and solving their own problems to reinforce understanding and prove the concepts to themselves.
How does the speaker describe the process of solving limit problems involving 0/0 indeterminate forms?
-The speaker describes the process as nonrigorous but effective, emphasizing the importance of factoring and simplifying expressions to find the limit, even if the function is undefined at the point of interest.
What is the main takeaway from the speaker's approach to solving limit problems?
-The main takeaway is that by factoring and simplifying expressions, one can often find the limit even when initially faced with an indeterminate form like 0/0, and that understanding the behavior of the fastest-growing terms is key to solving limits as x approaches infinity.
Outlines
📚 Understanding Limits through Simplification
This paragraph delves into the concept of limits in calculus, specifically focusing on how to handle indeterminate forms such as 0/0. The speaker illustrates this by substituting the value of x directly into the function to see if it yields a sensible result. The example given involves a limit as x approaches 3 of the function (x^2 - 6x + 9)/(x^2 - 9). Upon substitution, the speaker finds the result to be 0/0, which is an indeterminate form. To resolve this, the speaker suggests factoring the numerator and denominator to cancel out common terms, leading to a simplified expression that can be evaluated at x equals 3. The key takeaway is that while the function is undefined at x=3, its limit as x approaches 3 is 0, which is demonstrated through simplification and understanding the behavior of the function around the point of discontinuity.
🔢 Approaching Limits with Factoring and Infinite Values
The second paragraph continues the discussion on limits, emphasizing the importance of factoring in solving limit problems. The speaker explains that if the numerator and denominator share a common factor, it can be canceled out, simplifying the expression and allowing for a limit to be determined. Two examples are provided: the first involves a limit as x approaches 1 of the function (x^2 + x - 2)/(x - 1), which simplifies to x + 2, and the limit is 3 when x equals 1. The second example explores the limit as x approaches infinity of the function (x^2 + 3)/(x^3). The speaker uses intuitive reasoning and the concept of dominant terms to conclude that the limit approaches 0 since the denominator grows faster than the numerator. The paragraph concludes with another example, the limit as x approaches infinity of the function (3x^2 + x)/(4x^2 - 5), which simplifies to the constant ratio 3/4. The main theme here is the strategy of simplifying complex limit problems through factoring and analyzing the behavior of the function for large values of x.
Mindmap
Keywords
💡Limit
💡Continuous Function
💡Polynomial
💡Factoring
💡Indeterminate Form
💡Graphing
💡Infinity
💡Growth Rates
💡Rational Expression
💡Simplification
Highlights
The method of substituting the number into the limit function to see if it yields a sensible result.
The issue with getting a 0/0 result in limits and the need for simplification.
The strategy of factoring polynomials in limits to cancel out terms and simplify the expression.
The concept of canceling out terms in the numerator and denominator when they are zero to simplify limits.
The importance of understanding that a function may not be defined at a certain point but the limit can still be found.
The method of evaluating limits by plugging in the value of x and observing the result.
The application of the concept of limits to approach a value as x gets closer and closer to a certain number.
The approach to find limits as x approaches infinity by considering the fastest-growing terms in the numerator and denominator.
The insight that the limit of a function as x approaches infinity can be found by simplifying the expression and examining the dominant terms.
The strategy of using large values for x to estimate the behavior of a function and its limit.
The example of simplifying a limit expression by canceling out common factors in the numerator and denominator.
The explanation of how to deal with limits that result in 0/0 by factoring and simplifying.
The encouragement to use graphing calculators to visually confirm the results of limit calculations.
The approach to solving limits as x approaches a value by factoring and canceling out common terms.
The example of finding the limit as x approaches infinity by comparing the growth rates of terms in the numerator and denominator.
The method of simplifying complex limit expressions by identifying and focusing on the fastest-growing terms.
Transcripts
Browse More Related Video

Limits at infinity of quotients (Part 2) | Limits and continuity | AP Calculus AB | Khan Academy
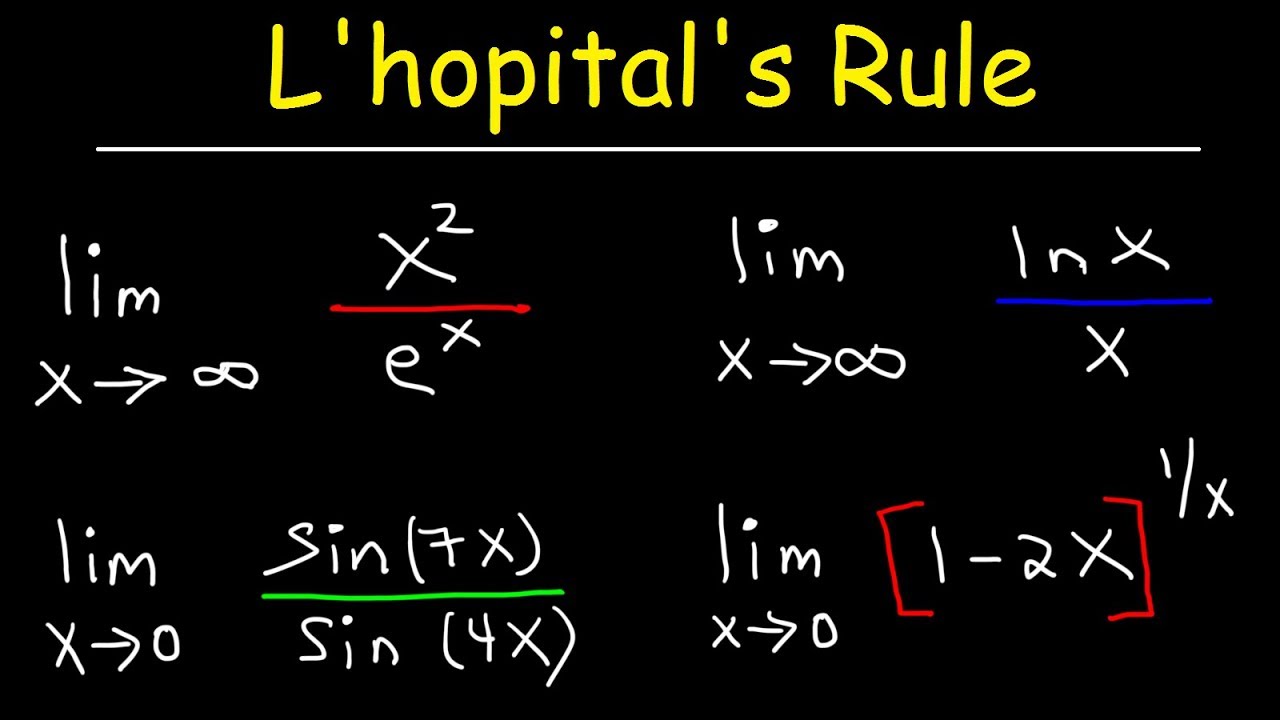
L'hopital's rule

Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan Academy
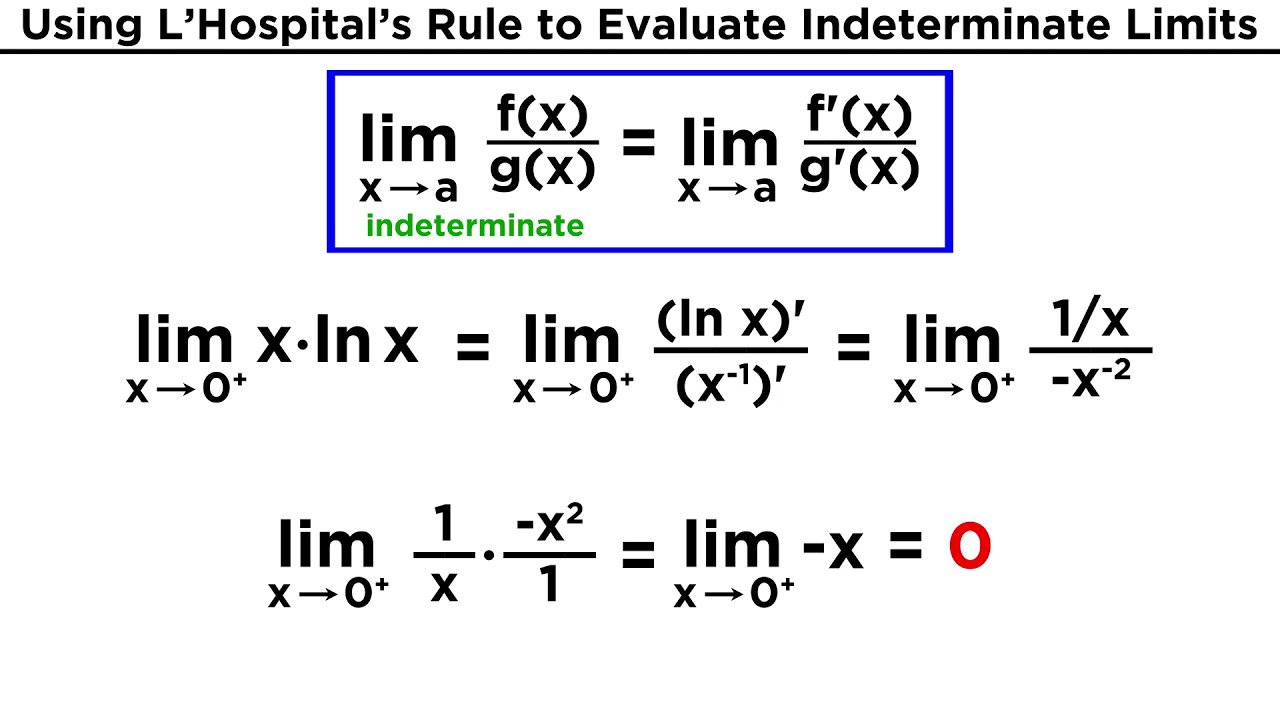
Understanding Limits and L'Hospital's Rule
Calculus: Limits at Infinity-Algebraic
Limits by rationalizing | Limits and continuity | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: