Introduction to l'Hôpital's rule | Derivative applications | Differential Calculus | Khan Academy
TLDRThis video script introduces the concept of using derivatives to evaluate limits, particularly those that result in indeterminate forms such as 0/0 or infinity/infinity. It explains the application of L'Hopital's rule in such scenarios, providing a clear and straightforward method to find the limits when direct substitution leads to undefined expressions. The video includes an example demonstrating how to apply L'Hopital's rule to a limit involving the sine function and x as x approaches zero, resulting in a determinate answer.
Takeaways
- 📚 The foundational concept of using limits to define derivatives in calculus is introduced.
- 🔄 This video reverses the process, aiming to find limits using derivatives, particularly for indeterminate forms.
- 🚫 Indeterminate forms occur when taking a limit results in expressions like 0/0, ∞/∞, -∞/∞, or ∞/-∞.
- 📝 L'Hopital's Rule is a method to address indeterminate forms by examining the derivatives of the functions involved.
- 🏆 The utility of L'Hopital's Rule is highlighted, noting its common use in math competitions for challenging limits.
- 🌟 The abstract form of L'Hopital's Rule is presented, requiring certain conditions to be met for its application.
- 🎯 The first case of L'Hopital's Rule is when both functions approach 0 simultaneously, allowing the limit of their derivatives' ratio to be considered.
- 🌈 An example is provided to illustrate the application of L'Hopital's Rule: finding the limit of sin(x)/x as x approaches 0.
- 🔢 The derivatives of the functions in the example are identified (cosine of x for sin(x), and 1 for x), and used to find the limit.
- 🥇 The result of the example demonstrates that the limit of sin(x)/x as x approaches 0 is equal to 1, satisfying the conditions of L'Hopital's Rule.
Q & A
What is the primary use of limits in calculus?
-The primary use of limits in calculus is to determine the derivatives of functions, as it helps in understanding the slope around a specific point on a graph.
What is the definition of a derivative based on?
-The definition of a derivative is based on the notion of a limit, specifically the limit of points approaching the point in question to find the slope.
What is an indeterminate form in limits?
-An indeterminate form in limits occurs when evaluating a limit results in an undefined expression, such as 0/0, infinity/infinity, negative infinity/infinity, or positive infinity/negative infinity.
What is l'Hopital's rule and what does it help to solve?
-L'Hopital's rule is a method used to evaluate limits that result in indeterminate forms. It allows us to find the limit by taking the derivatives of the functions involved and evaluating the limit of their ratio.
What are the conditions for applying l'Hopital's rule for an indeterminate form of 0/0?
-The conditions for applying l'Hopital's rule for a 0/0 indeterminate form are: the limit of f(x) as x approaches c is 0, the limit of g(x) as x approaches c is 0, and the limit of the ratio of their derivatives, f'(x)/g'(x), exists and equals some value L.
How does l'Hopital's rule work for an indeterminate form of infinity/infinity?
-For an indeterminate form of infinity/infinity, l'Hopital's rule works similarly by checking if the limits of f(x) and g(x) as x approaches c are both positive or negative infinity, and if the limit of the ratio of their derivatives exists and equals some value L.
What is the example provided in the script to illustrate l'Hopital's rule?
-The example provided is the limit of sine(x)/x as x approaches 0, which results in an indeterminate form of 0/0. By applying l'Hopital's rule, we find that the limit equals 1.
What are the derivatives of the functions f(x) = sine(x) and g(x) = x in the provided example?
-In the provided example, the derivative of f(x) = sine(x) is f'(x) = cosine(x), and the derivative of g(x) = x is g'(x) = 1.
What is the limit of the ratio of the derivatives in the example, as x approaches 0?
-In the example, the limit of the ratio of the derivatives, cosine(x)/1, as x approaches 0, is equal to 1.
How does the example demonstrate the application of l'Hopital's rule?
-The example demonstrates that by applying l'Hopital's rule, we can evaluate the limit of an indeterminate form (0/0) by finding the limit of the ratio of the derivatives (cosine(x)/1), which equals 1 as x approaches 0.
What is the significance of l'Hopital's rule in mathematical problem-solving?
-L'Hopital's rule is significant in mathematical problem-solving as it provides a method to evaluate limits that would otherwise be undefined or indeterminate, making it a valuable tool in calculus and mathematical competitions.
Outlines
📚 Introduction to L'Hopital's Rule and Indeterminate Forms
This paragraph introduces the concept of using derivatives to evaluate limits, particularly those that result in indeterminate forms such as 0/0 or infinity/infinity. It explains that the traditional method of learning calculus involves understanding limits and their role in defining derivatives. However, this video will focus on the reverse process, using the concept of limits to understand derivatives, especially in situations where the direct calculation leads to indeterminate forms. The video mentions L'Hopital's rule as a key tool for handling such indeterminate forms and hints at its potential use in math competitions. The explanation sets the stage for a deeper dive into the application of L'Hopital's rule through examples.
📈 Application of L'Hopital's Rule: An Example
This paragraph delves into the practical application of L'Hopital's rule by providing a specific example. It demonstrates how to use the rule to evaluate the limit of the function sine(x)/x as x approaches 0, which results in an indeterminate form of 0/0. The explanation outlines the conditions that must be met to apply L'Hopital's rule, emphasizing that both the numerator and denominator must approach zero or infinity for the rule to be applicable. The video then shows the process of finding the derivatives of the numerator (sine(x)) and the denominator (x), which are cosine(x) and 1, respectively. It concludes by calculating the limit of the ratio of these derivatives as x approaches 0, which results in the value of 1. This example illustrates the process of using L'Hopital's rule to find the limit of a function when it initially appears to be indeterminate.
Mindmap
Keywords
💡Calculus
💡Limits
💡Derivatives
💡Indeterminate Forms
💡l'Hopital's Rule
💡Slope
💡Infinity
💡Quotient
💡Existence
💡Cosine Function
💡Trigonometric Functions
Highlights
Calculus initially involves understanding limits and using them to determine derivatives of functions.
The definition of a derivative is based on the concept of a limit, representing the slope of a function at a particular point.
This video introduces a unique approach of using derivatives to evaluate certain types of limits, especially those that result in indeterminate forms.
Indeterminate forms include scenarios like 0/0, infinity/infinity, negative infinity/infinity, and positive infinity/negative infinity.
L'Hopital's rule is a valuable mathematical tool used to address indeterminate forms by applying derivatives.
The video provides a straightforward explanation and application of L'Hopital's rule without delving into its proof.
L'Hopital's rule is often a focus in math competitions, especially for evaluating complex limits.
The rule states that if the limit of a function f(x) as x approaches c results in 0 and the same for g(x), and the limit of the ratio of their derivatives exists and equals L, then the limit of the ratio f(x)/g(x) also equals L.
A similar application of the rule applies when f(x) and g(x) approach positive or negative infinity.
The video uses the example of the limit of sine(x)/x as x approaches 0 to illustrate the application of L'Hopital's rule.
Sine(0) is 0 and the limit of x as x approaches 0 is also 0, resulting in an indeterminate form of 0/0.
By applying L'Hopital's rule, taking the derivative of sine(x) which is cosine(x) and the derivative of x which is 1, the limit can be evaluated.
The limit of cosine(x)/1 as x approaches 0 is 1, demonstrating that L'Hopital's rule can resolve indeterminate forms.
The video concludes that the limit of sine(x)/x as x approaches 0 is equal to 1, effectively using L'Hopital's rule to find the value.
The video promises further examples in subsequent lessons to solidify the understanding of L'Hopital's rule.
Transcripts
Browse More Related Video
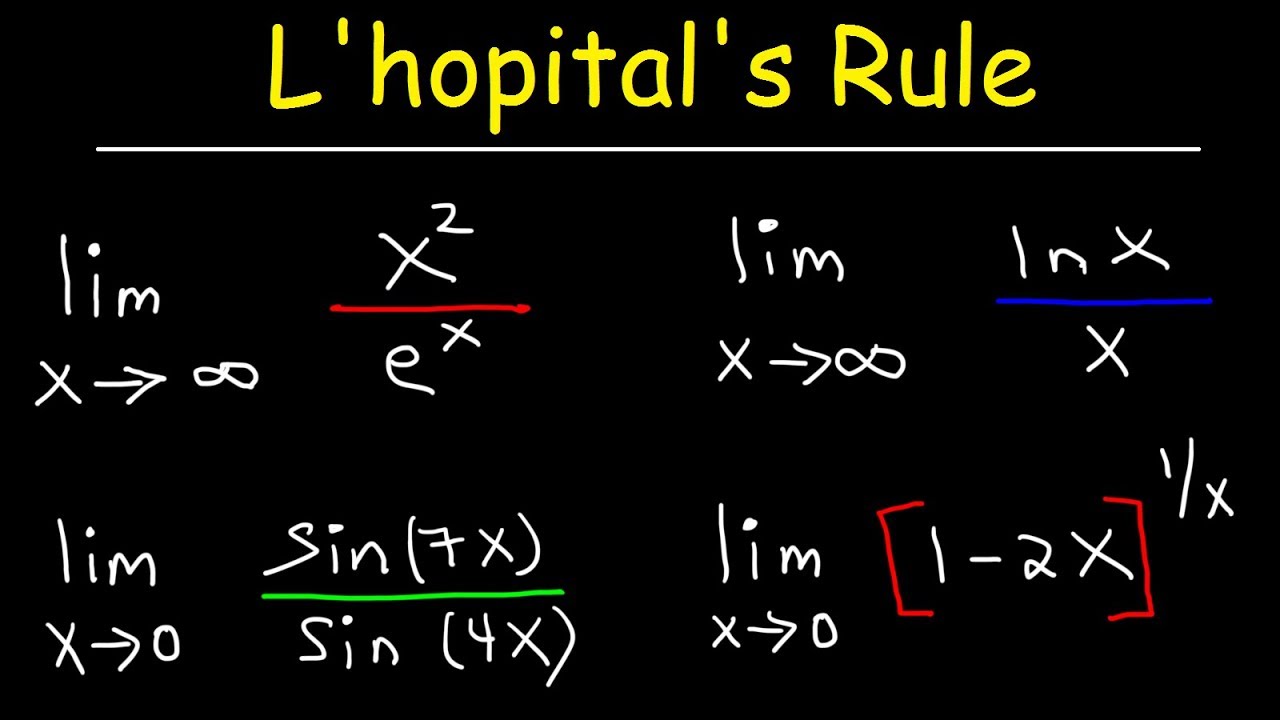
L'hopital's rule

L'Hôpital's rule example 1 | Derivative applications | Differential Calculus | Khan Academy
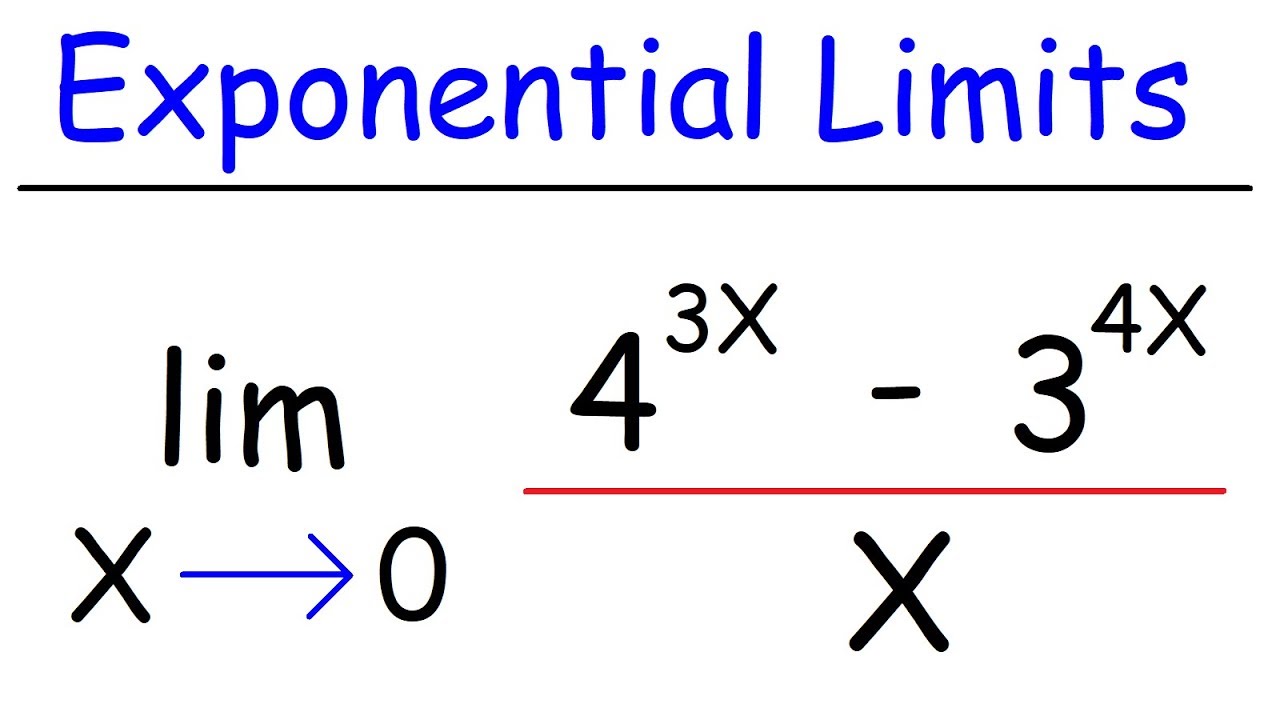
Limits of Exponential Functions | Calculus
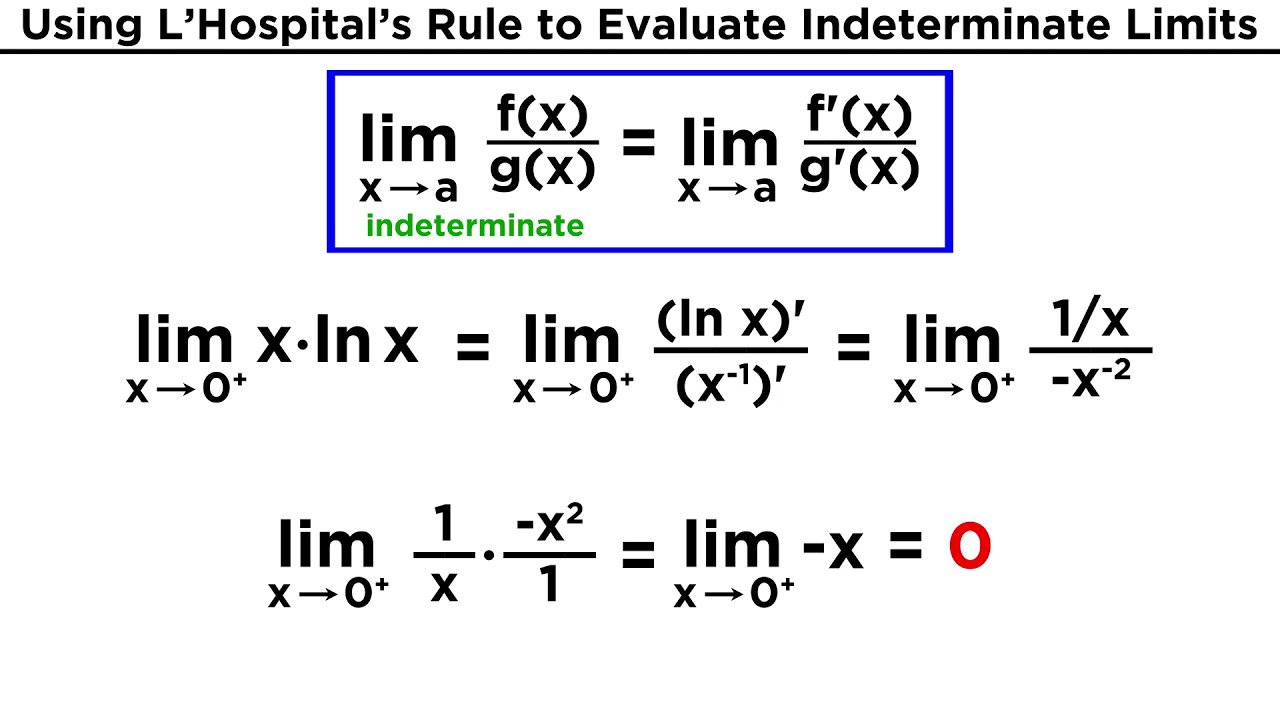
Understanding Limits and L'Hospital's Rule

Using L'Hopital's Rule to show that exponentials dominate polynomials

Limits of Logarithmic Functions | Calculus
5.0 / 5 (0 votes)
Thanks for rating: