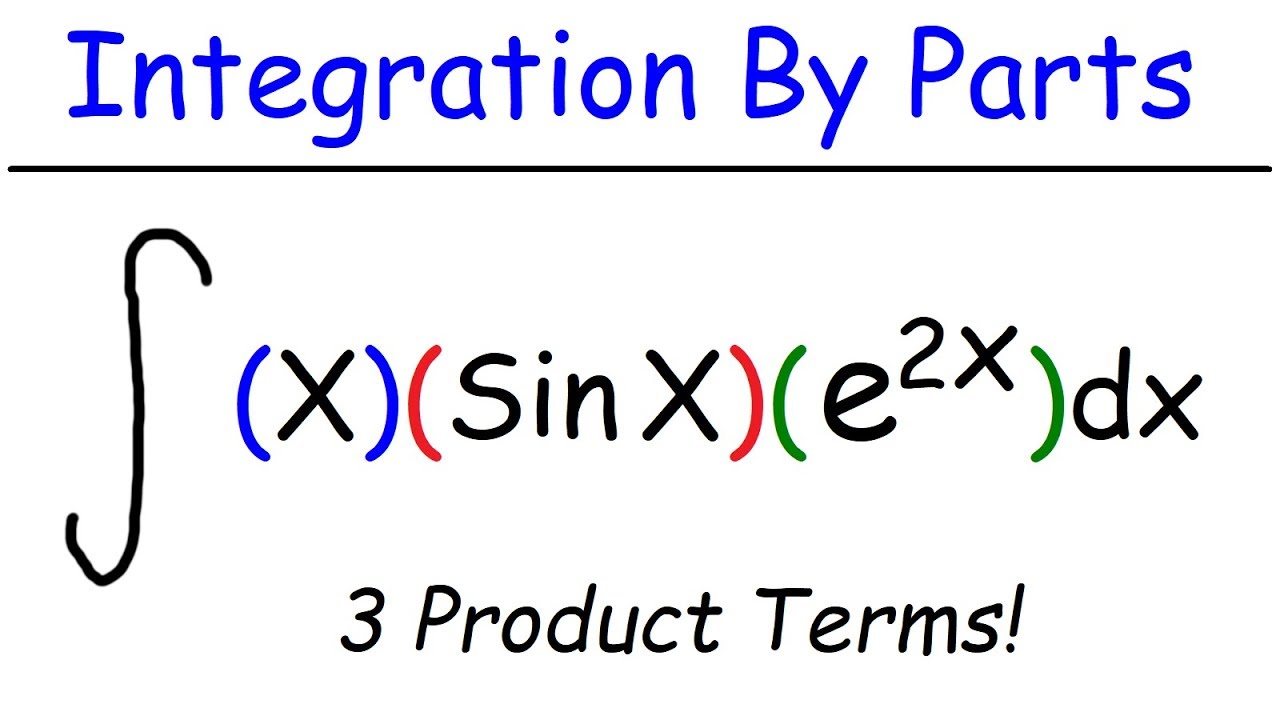
Product Rule With 3 Functions - Derivatives | Calculus

The Organic Chemistry Tutor
19 Dec 201905:08
EducationalLearning
32 Likes 10 Comments

TLDRThe video script offers a comprehensive guide on how to calculate the derivative of a complex function involving three components: x squared, sine x, and the natural log of x. It introduces the triple product rule as an extension of the standard product rule for derivatives and provides a step-by-step solution to the problem. The script also explains the derivatives of the individual functions and suggests simplifications to obtain a more concise final answer. The video encourages viewers to review related topics and explore additional resources for a deeper understanding of derivatives.

Takeaways
  • 📝 The problem involves finding the derivative of a product of three functions, which requires an extension of the product rule.
  • 🔍 The standard product rule applies to the product of two functions, but the situation here involves three functions, necessitating a modification of the rule.
  • 📌 To find the derivative of f(x) * g(x) * h(x), the method involves taking the derivative of the first function and multiplying it by the other two, then taking the derivative of the second function and multiplying by the first and third, and finally differentiating the third function while keeping the first two unchanged.
  • 👉 Identify the functions: f(x) = x^2, g(x) = sin(x), and h(x) = ln(x) to apply the modified product rule.
  • 🧮 Calculate the derivatives: f'(x) = 2x, g'(x) = cos(x), and h'(x) = 1/x.
  • 🔧 Plug the derivatives into the formula: the derivative of the function is 2x * sin(x) * ln(x) + x^2 * cos(x) * ln(x) + x * sin(x).
  • 📈 Simplify the expression by reducing x^2 * 1/x to x and factoring out x from each term.
  • 🎯 The simplified answer is x * (2 * sin(x) * ln(x) + cos(x) * ln(x) + sin(x)).
  • 💡 The video provides a comprehensive guide on using the triple product rule for more complex derivative problems.
  • 🔗 The video description contains links for further review and practice on various derivative topics, including exponential and logarithmic functions, as well as additional differentiation rules.
  • 👍 The video encourages viewers to subscribe to the channel for more content and to check the description for additional resources.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is how to find the derivative of a function that involves three components multiplied together, specifically x squared times sine x times the natural log of x.

  • What is the product rule mentioned in the video?

    -The product rule mentioned in the video is a fundamental calculus rule that states the derivative of a product of two functions is the derivative of the first function times the second function plus the first function times the derivative of the second function.

  • How does the product rule change when dealing with three functions multiplied together?

    -When dealing with three functions multiplied together, the product rule is extended. The derivative of the first function times the second and third functions is the first function's derivative times the second and third functions, plus the first function times the second function's derivative times the third function, and finally the first and second functions times the third function's derivative.

  • What are the individual functions (f, g, h) in the given problem?

    -In the given problem, f is x squared, g is sine x, and h is the natural log of x.

  • What are the derivatives of the individual functions (f, g, h)?

    -The derivative of f (x squared) is 2x, the derivative of g (sine x) is cosine x, and the derivative of h (natural log of x) is 1 over x.

  • What is the full derivative expression for x squared times sine x times the natural log of x?

    -The full derivative expression is 2x times sine x times ln(x) + x squared times cosine x times ln(x) + x times sine x.

  • How can the final derivative expression be simplified?

    -The final derivative expression can be simplified by factoring out common terms. In this case, x can be factored out, resulting in x times (2 sine x ln(x) + cosine x ln(x) + sine x).

  • What additional resources are offered in the video description?

    -The video description offers links for further review and practice on derivatives of trigonometric functions, natural logs, the power rule, as well as additional calculus topics like the product rule, chain rule, and quotient rule.

  • What is the significance of the triple product rule in calculus?

    -The triple product rule is significant in calculus as it extends the product rule to handle more complex functions involving multiple components. This allows for the differentiation of more intricate expressions and is a fundamental tool for solving problems in advanced calculus.

  • How does the video encourage further learning and practice?

    -The video encourages further learning and practice by suggesting that viewers review the provided links in the description for additional problems on derivatives of exponential functions, logarithmic differentiation, and other related calculus topics.

Outlines
00:00
📚 Derivative of a Complex Function using the Triple Product Rule

This paragraph introduces a mathematical concept on how to find the derivative of a complex function, specifically x squared times sine x times the natural log of x. It explains the need for familiarity with the product rule and extends it to a triple product rule for this problem. The paragraph details the process of identifying the functions f, g, and h and calculating their derivatives (f'=2x, g'=cosine, h'=1/x). It then applies the triple product rule to find the derivative of the given function, leading to a simplified result of x(2 sine x ln x + cosine x ln x + sine x). The paragraph concludes by encouraging viewers to review derivative concepts if needed and provides a link for further study materials.

05:00
📖 Additional Resources and Encouragement for Further Learning

The second paragraph serves as a call to action for viewers to utilize additional resources related to the topic of derivatives. It invites the audience to check the description section for links to further study materials on various types of derivatives, such as exponential functions, logarithmic differentiation, product rule, chain rule, quotient rule, and more. The paragraph ends with a reminder to subscribe to the channel for more educational content and an expression of gratitude for watching the video.

Mindmap
Keywords
💡derivative
The derivative is a fundamental concept in calculus that represents the rate of change of a function with respect to its variable. In the context of the video, the derivative is used to find the rate at which one quantity changes in response to changes in another quantity, specifically the function x squared times sine x times ln x. The process of finding the derivative involves applying rules such as the product rule, which is discussed in detail in the video.
💡product rule
The product rule is a vital calculus formula used to find the derivative of a product of two functions. According to the rule, the derivative of a product of functions f and g is f' times g plus f times g'. In the video, the product rule is applied to the function involving three components, x^2, sin(x), and ln(x), where the rule is extended to handle more than two functions.
💡trigonometric functions
Trigonometric functions are mathematical functions that relate angles to real numbers. The most common trigonometric functions are sine, cosine, and tangent. In the video, the derivative of the sine function, which is cosine, is used as part of the process to find the derivative of the given function. Understanding the properties and derivatives of trigonometric functions is crucial for solving calculus problems involving them.
💡natural log
The natural log, or logarithm with base e (where e is the mathematical constant approximately equal to 2.71828), is a key concept in calculus and mathematics in general. It is the inverse function of the exponential function and is denoted as ln(x). In the video, the derivative of the natural log of x, which is 1/x, is calculated as part of the process to find the derivative of the given function.
💡power rule
The power rule is a basic rule in calculus that allows for the differentiation of expressions where the variable is raised to a power. It states that the derivative of x^n, where n is a constant, is n*x^(n-1). Although not explicitly mentioned in the video, the power rule is an essential tool in differentiating functions like x^2, which is part of the given function.
💡chain rule
The chain rule is a fundamental calculus concept used to find the derivative of a composite function. It states that the derivative of a function composed of other functions is the derivative of the outer function evaluated at the inner function, multiplied by the derivative of the inner function. While the chain rule is not directly discussed in the video, it is an essential concept for understanding more complex differentiation problems.
💡differentiation
Differentiation is the process of finding the derivative of a function, which helps in understanding how a function changes with respect to its variable. It is a core operation in calculus and is the focus of the video, where the differentiation of the function x^2 * sin(x) * ln(x) is demonstrated using the product rule.
💡exponential functions
Exponential functions are mathematical functions of the form f(x) = a^x, where 'a' is a constant and 'x' is the variable. These functions are important in many areas of mathematics and science. The video script mentions that additional resources for learning about exponential functions and their derivatives will be provided in the description section.
💡ln(x)
The notation ln(x) represents the natural logarithm of x, which is a fundamental concept in calculus. It is the inverse operation of exponentiation with the base of e, and it is used to solve various mathematical problems, including those involving growth and decay processes. In the video, the derivative of ln(x) is used as part of the process to find the derivative of the given function.
💡review
In the context of the video, a review refers to the process of revisiting and reinforcing previously learned material to ensure understanding and retention. The video script mentions that a link will be provided for a review of derivatives, trigonometric functions, and the power rule, which are essential for understanding the differentiation of the given function.
💡simplify
Simplification in mathematics refers to the process of making a mathematical expression or equation more straightforward or easier to understand. In the video, the derivative of the given function is simplified by combining like terms and factoring out common factors, which helps in presenting the final answer in a more concise form.
Highlights

The problem involves finding the derivative of a complex function, x^2 * sin(x) * ln(x).

The solution requires understanding the product rule for derivatives.

The standard product rule applies to two functions multiplied together, but this problem has three.

The triple product rule is introduced to handle the three-component function.

The function is broken down into three parts: f as x^2, g as sin(x), and h as ln(x).

The derivative of x^2 is found to be 2x.

The derivative of sin(x) is identified as cosine x.

The derivative of ln(x) is given as 1/x.

The derivative calculation involves multiplying the derivative of the first part by the other two parts, and vice versa for the other parts.

The derivative of the function is expressed as a sum of three terms, using the triple product rule.

The term x^2 * ln(x)/x simplifies to x * ln(x).

The final answer is expressed as x * (2 * sin(x) * ln(x) + cos(x) * ln(x)) + sin(x).

The video provides a link in the description for a review of derivatives and related rules.

The video ends with an encouragement to subscribe to the channel and explore more problems related to derivatives in the description.

The video covers the use of the triple product rule for derivative problems with three components.

Transcripts

Browse More Related Video

Product Rule With 4 Functions - Derivatives | Calculus

Introduction to Logarithmic Differentiation

Finding Derivatives Using Logarithms - Differential Calculus

Product Rule For Derivatives

Derivative of sin(ln(x_)) | Advanced derivatives | AP Calculus AB | Khan Academy

Calculus - Integration By Parts

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativesTriple Product RuleMathematicsCalculusTutorialEducational ContentTrigonometric FunctionsLogarithmsDifferential EquationsMath Teaching