CH403 8 Monoprotic Acid-Base Equilibria
TLDRThis transcript delves into the intricacies of monoprotic acid-base equilibria, exploring the calculation of pH for strong acids and bases, as well as weak acids and bases. It explains the concepts of Ka and Kb constants, the Henderson-Hasselbalch equation, and the importance of buffers in maintaining pH stability. The script also discusses the impact of ionic strength and activity coefficients on pH calculations, and how these factors can lead to deviations from predicted pH values. The discussion includes examples and calculations that demonstrate the application of these principles in real-world scenarios.
Takeaways
- 📚 The pH of a strong acid solution can be calculated using the formula pH = -log[H+], where [H+] is the hydrogen ion concentration.
- 🧪 For strong bases like potassium hydroxide, pH is determined by the concentration of hydroxide ions, with pH = 14 - pOH, where pOH = -log[OH-].
- 🔄 The autoionization of water is a crucial factor to consider when dealing with very dilute solutions of acids or bases, affecting the pH calculation.
- 🔧 The systematic treatment of equilibrium is required to solve for pH when the concentration of hydrogen or hydroxide ions is low due to the autoionization of water.
- 📈 The Henderson-Hasselbalch equation (pH = pKa + log([A-]/[HA])) is used to calculate the pH of a buffer solution, which is an approximation unless activity coefficients are included.
- 🧬 Buffer capacity, or β, measures a solution's resistance to pH changes upon addition of strong acids or bases, and is highest near the pKa value.
- 🌡️ The pKa of an acid can change with temperature, affecting the pH of a buffer solution if the temperature varies.
- 💧 The presence of other ions, such as sodium ions, can influence the pH calculation, especially when dealing with salts of weak acids and bases.
- 🔢 In practice, preparing a buffer solution of a specific pH involves adjusting the ratio of acid to base and monitoring the pH until the desired value is reached.
- 🌟 The effectiveness of a buffer is influenced by the concentrations of the acid and its conjugate base, as well as the ionic strength of the solution.
- 🛠️ When calculating pH, it's important to consider the impact of ionic strength and activity coefficients, especially in solutions with low concentrations of hydrogen or hydroxide ions.
Q & A
What is the pH of a 0.1 M hydrobromic acid solution?
-The pH of a 0.1 M hydrobromic acid solution is 1.00, calculated using the formula pH = -log[H+], where [H+] is the concentration of hydrogen ions, which in this case is 0.1 M.
How can activity coefficients affect the pH calculation of a solution?
-Activity coefficients account for the interactions between ions in a solution. They can affect the pH calculation by adjusting the effective concentration of ions, leading to a more accurate pH value. For example, in a 0.1 M hydrobromic acid solution, considering activity coefficients, the pH is 1.08 instead of 1.00.
What is the relationship between the concentration of hydrogen ions and hydroxide ions in a solution of a strong base like potassium hydroxide?
-In a solution of a strong base like potassium hydroxide, the concentration of hydrogen ions is related to the hydroxide ion concentration through the ion product of water (Kw). The hydrogen ion concentration is equal to Kw divided by the hydroxide ion concentration.
Why does the pH calculation for a dilute solution of potassium hydroxide (KOH) require considering the autoionization of water?
-The pH calculation for a dilute KOH solution requires considering the autoionization of water because, at low concentrations, the hydroxide ions from water's autoionization can significantly contribute to the total hydroxide ion concentration, affecting the pH of the solution.
What is the Henderson-Hasselbalch equation and how is it used in buffer solutions?
-The Henderson-Hasselbalch equation is a mathematical relationship that describes the pH of a buffer solution based on the ratio of the concentrations of the base (conjugate base) to the acid (conjugate acid). It is used to calculate the pH of a buffer solution when the concentrations of the weak acid and its conjugate base are known.
How does the buffer capacity (β) of a solution change with pH?
-The buffer capacity (β) of a solution is highest near the pKa value of the weak acid in the buffer. It indicates how effectively a buffer resists changes in pH when a strong acid or base is added. The capacity decreases as the pH moves further away from the pKa value.
What factors can cause the observed pH of a buffer to differ from the calculated pH?
-Several factors can cause the observed pH of a buffer to differ from the calculated pH, including the ionic strength of the solution affecting activity coefficients, the temperature affecting the pKa value, and the presence of other ions in the solution that can interact with the buffer components.
What is the significance of the pKa value in the context of buffer solutions?
-The pKa value is the negative logarithm of the acid dissociation constant (Ka) and represents the pH at which a weak acid is half dissociated. In buffer solutions, the pH is ideally close to the pKa value of the weak acid, where the buffer is most effective at resisting changes in pH.
How can the autoionization of water affect the pH of a very dilute solution?
-In very dilute solutions, the autoionization of water can become more significant and affect the pH. At very low concentrations, the hydrogen and hydroxide ions from water's autoionization can contribute significantly to the total ion concentration, thus influencing the pH of the solution.
What is the relationship between the fraction of dissociation (α) and the concentrations of a weak acid and its conjugate base?
-The fraction of dissociation (α) is the ratio of the concentration of the deprotonated form of the weak acid to the total concentration of the acid (both protonated and deprotonated forms). It represents the proportion of the weak acid that has dissociated into its conjugate base in solution.
How can the charge balance and mass balance equations be used to calculate the pH of a solution containing a weak acid and its conjugate base?
-The charge balance equation ensures that the total positive charge from cations equals the total negative charge from anions, while the mass balance equation accounts for the conservation of matter. By solving these two equations simultaneously, along with the equilibrium expression for the weak acid dissociation, one can determine the concentrations of the weak acid, its conjugate base, and the hydrogen and hydroxide ions, which allows for the calculation of the pH.
Outlines
📚 Introduction to Acid-Base Equilibria
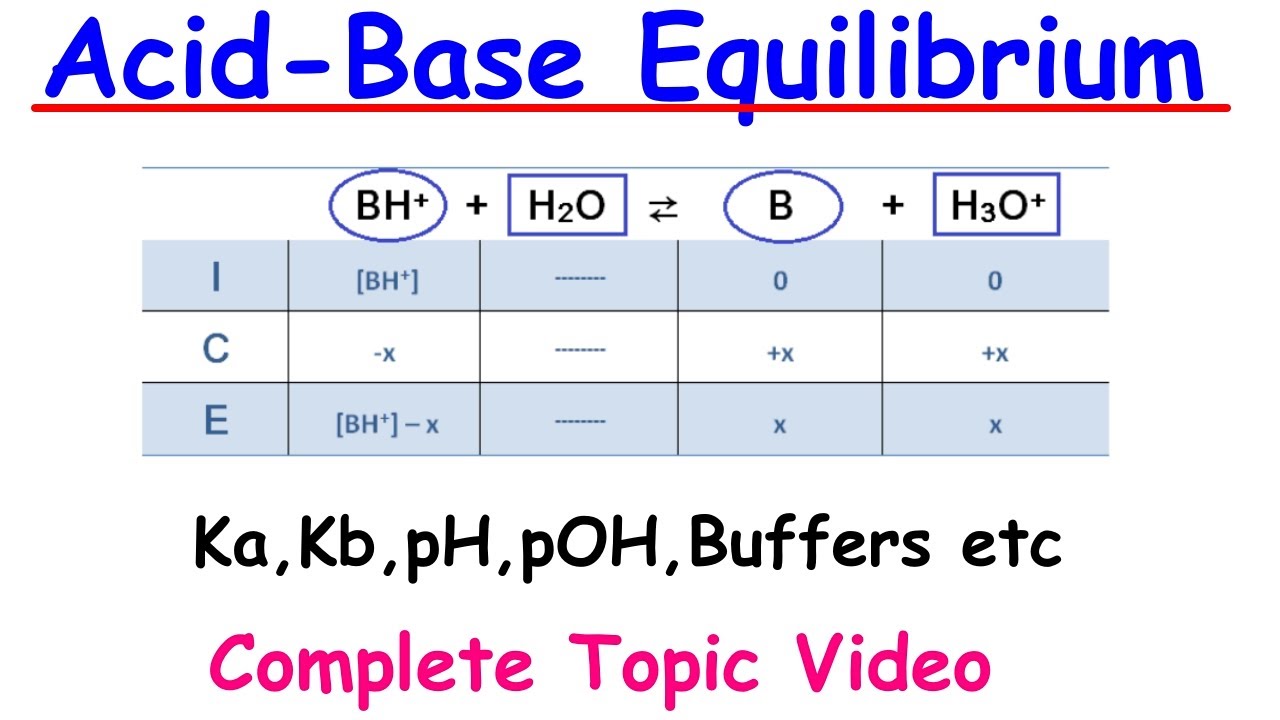
This paragraph introduces the concept of acid-base equilibria, focusing on monoprotic acids and bases. It begins with a discussion on calculating the pH of a strong acid like hydrobromic acid and a strong base like potassium hydroxide. The importance of considering activity coefficients and the autoionization of water is highlighted when dealing with more dilute solutions. The paragraph also introduces the method of equilibrium to solve for pH in cases where the concentration of hydrogen ions or hydroxide ions is low.
📈 Understanding Weak Acids and Bases
This section delves into the behavior of weak acids and bases, explaining how they do not dissociate completely in solution. The paragraph introduces the acid dissociation constant (Ka) and base dissociation constant (Kb), and how they relate to the pH and pOH of a solution. It also explains the concept of conjugate acids and bases, and provides examples using formic acid and benzoic acid. The relationship between Ka and Kb with the ion product of water (Kw) is also discussed.
🧪 Calculation of pH for Weak Acids
This paragraph focuses on the calculation of pH for weak acids, taking into account the autoionization of water and the mass and charge balances in the solution. It simplifies the complex equations into a more manageable quadratic equation by making assumptions based on the relative concentrations of the species involved. The concept of the fraction of dissociation (alpha) for an acid is introduced, and its mathematical expression is provided.
🧬 Buffer Solutions and Their Importance
This section discusses the concept of buffer solutions, which are mixtures of a weak acid and its conjugate base. It explains the importance of buffers in maintaining a relatively constant pH, especially in biological systems. The paragraph introduces the Henderson-Hasselbalch equation, which is used to calculate the pH of a buffer solution. The effect of adding strong acids or bases to a buffer solution is also explored, demonstrating the buffering capacity.
🥼 Practical Buffer Preparation and the Buffer Capacity
This paragraph covers the practical aspects of preparing buffer solutions and the concept of buffer capacity, which measures a solution's resistance to pH changes. It explains the factors that can cause deviations between calculated and observed pH values, such as ionic strength, activity coefficients, and temperature. The paragraph also provides a method for preparing a buffer solution with a specific pH and discusses the limitations of the Henderson-Hasselbalch equation in certain conditions.
🔬 Advanced Acid-Base Calculations
The final paragraph presents a more advanced treatment of acid-base equilibria, considering scenarios where the approximations made in previous calculations may not be valid. It discusses the impact of formal concentrations on the accuracy of pH calculations, particularly when dealing with small concentrations of hydrogen ions or hydroxide ions. The paragraph concludes with a detailed example calculation that illustrates the nuances of acid-base equilibria.
Mindmap
Keywords
💡Monoprotic acid
💡pH
💡Equilibria
💡Kw (Ionic Product of Water)
💡Strong base
💡Dissociation
💡Autoionization of water
💡Buffer solution
💡Henderson-Hasselbalch equation
💡pKa
💡Conjugate acid-base pair
💡Ionic strength
💡Activity coefficients
Highlights
Calculating the pH of 0.1 M hydrobromic acid results in a pH of 1.00, taking into account the reaction of a strong acid with water to form hydronium and its conjugate base.
For a strong base like potassium hydroxide, the pH calculation involves the dissociation of the base into potassium and hydroxide ions, with the pH being determined by the hydroxide ion concentration and the ion product of water (kW).
The pH of a 1.0 x 10^-8 M potassium hydroxide solution is incorrectly calculated as 6.00 initially, but the mistake is recognizing the autoionization of water and the need for a systematic treatment of equilibrium.
In dilute solutions of strong bases, the pH calculation must consider the hydroxide ions from the autoionization of water, leading to a quadratic equation that can be solved to find the correct hydrogen ion concentration and pH.
The importance of understanding the dissociation constant (Ka) for weak acids and the relationship between Ka, Kb, and the ion product of water (kW) is emphasized for accurate pH calculations.
The Henderson-Hasselbalch equation is introduced as a tool for calculating the pH of a buffer solution, which is a mixture of a weak acid and its conjugate base.
Buffers are crucial in maintaining pH stability in biological systems, such as in cells where different pH levels are required for proper protein function.
The concept of buffer capacity, or the ability of a solution to resist pH changes upon addition of strong acids or bases, is explained and related to the pKa of the buffer components.
Practical considerations for preparing buffers, such as the absorption of CO2 from the air and the impact of ionic strength on activity coefficients, are discussed to ensure accurate pH control.
The impact of temperature on the pKa value and thus the pH of a buffer solution is highlighted, with examples showing how a change in temperature can affect pH significantly.
The calculation of pH for a solution containing both a weak acid and its conjugate base involves the use of mass balance and charge balance equations to determine the correct concentrations for pH calculation.
The limitations of the Henderson-Hasselbalch equation in predicting pH when concentrations are low or when hydrogen ion or hydroxide ion concentrations are high are discussed, emphasizing the need for activity coefficient inclusion.
A detailed example is provided for calculating the pH of a solution with a specific number of moles of a weak acid and its conjugate base, demonstrating the use of quadratic equations and the impact of ignoring certain ion concentrations.
The concept of the buffer effect, or the ability of a buffer to resist changes in pH, is introduced and quantified through the calculation of the number of moles of strong acid or base needed to change the pH by one unit.
The process of preparing a buffer solution in a real-world scenario is described, including the step-by-step procedure and considerations for achieving the desired pH.
The impact of the ratio of acid to conjugate base on the pH of a buffer solution is discussed, with the pH being approximately equal to the pKa when the ratio is one-to-one.
The calculation of buffer capacity and its relationship to the pH and pKa of a buffer system is explained, highlighting the highest buffer capacity near the pKa value.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: