4 | FRQ (with Experimental or Lab-Based Component) | Practice Sessions | AP Physics C: Mechanics
TLDRIn this educational video, Dr. Julie Hood explores the concept of terminal velocity with a lab-based experiment involving falling stacks of coffee filters. She guides viewers through understanding forces in equilibrium, graphing non-linear data to find a linear relationship, and calculating mechanical energy dissipation due to air resistance. The video emphasizes the importance of free body diagrams, linearization techniques, and accurate graph plotting to solve physics problems related to motion under resistance.
Takeaways
- 📚 The video is an educational lab-based tutorial by Dr. Julie Hood, an AP physics teacher, focusing on the relationship between terminal velocity and mass of falling paper coffee filters.
- 🔍 Students are expected to understand forces in equilibrium and demonstrate this through an experiment involving varying the mass of a stack of coffee filters.
- 📉 The experiment involves creating a linear relationship from nonlinear data, which requires the use of a free body diagram to analyze forces acting on the falling stack.
- 📈 Students must graph the relationship between terminal velocity and mass to determine a constant, which involves linearizing the data by squaring the terminal velocity values.
- 📝 The script emphasizes the importance of correctly labeling axes, including units, and scaling graphs to effectively represent data.
- 📐 The tutorial explains how to derive an expression relating terminal velocity to mass using the assumption that drag force is proportional to the square of the velocity.
- 📊 The process of linearization is detailed, showing how to transform a non-linear relationship into a linear graph for easier analysis and understanding.
- 🔢 The script provides a step-by-step guide on how to calculate the constant 'C' from the graph, which is crucial for understanding the relationship between terminal velocity and mass.
- 🚀 The video also covers how to sketch a graph of speed versus time for a falling body, approaching terminal velocity, and explains the concept of mechanical energy dissipation due to air resistance.
- ⚖️ The final part of the script involves applying the conservation of energy principle to determine the amount of mechanical energy lost during the fall due to air resistance.
- 📚 The tutorial concludes with a reminder of the importance of graphing skills, labeling, and scaling, and encourages further practice on similar problems.
Q & A
What is the main topic of the video by Dr. Julie Hood?
-The main topic of the video is to work through an experimental lab-based free response question involving a resistive force, specifically investigating the relationship between the terminal speed of a stack of falling paper coffee filters and its mass.
What is the procedure for the experiment described in the video?
-The procedure involves stacking a number of coffee filters and dropping the stack from rest. Students change the number of filters in the stack to vary the mass while keeping the shape the same, observing the effect of air resistance or drag force on the falling stack.
What is the expected outcome of the students' investigation in the experiment?
-The expected outcome is for students to demonstrate an understanding of forces in equilibrium, create a linear relationship from nonlinear data, graph this relationship to determine a constant, and ultimately determine an expression for the amount of mechanical energy dissipated due to air resistance during the fall.
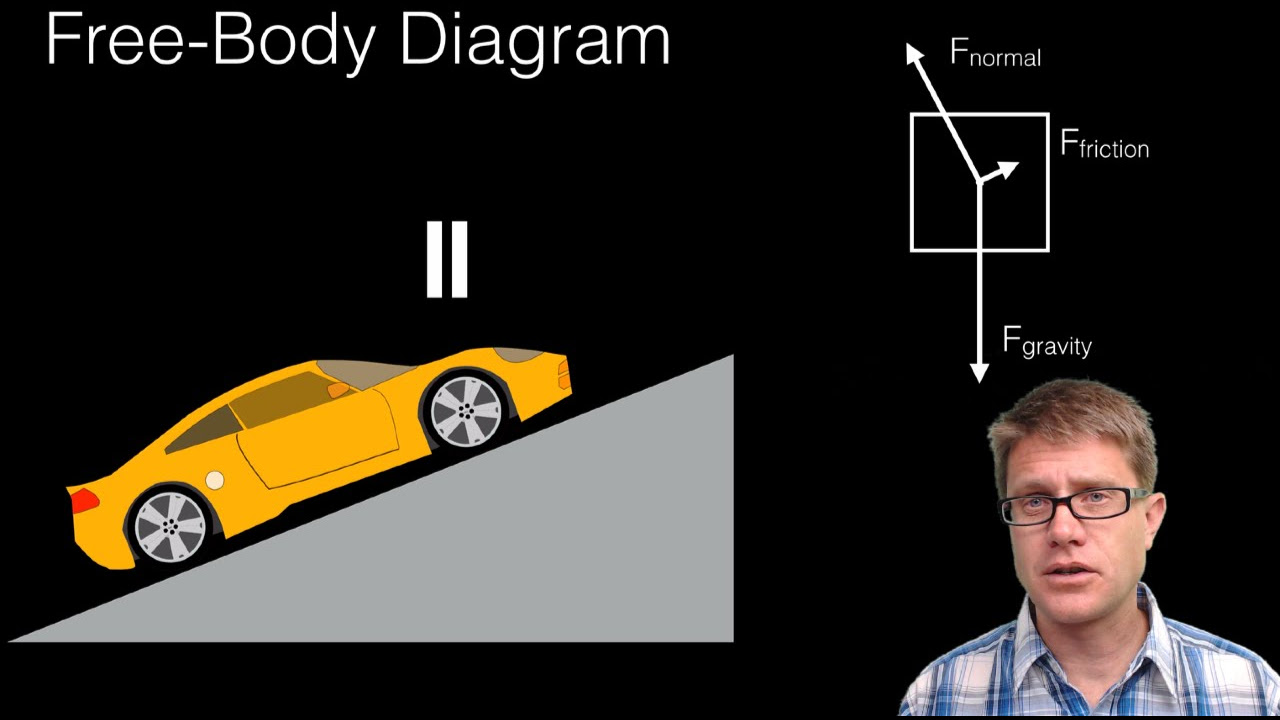
Why is it important for students to understand free body diagrams in physics?
-Free body diagrams are crucial for visualizing all the forces acting on an object. They help in solving physics problems by providing a clear representation of the forces, which can then be used to apply the principles of physics, such as Newton's laws.
What is the relationship between terminal velocity and the drag force acting on the coffee filters?
-At terminal velocity, the object is no longer accelerating, meaning the net force acting on it is zero. The drag force, which is proportional to the square of the velocity, balances out with the force of gravity, resulting in a constant falling speed.
How can the drag force be linearized for graphing purposes?
-The drag force can be linearized by squaring the terminal velocity data. This transforms the non-linear relationship into a linear one, allowing for a straight-line graph when plotted against the mass of the coffee filters.
What is the significance of squaring the terminal velocity data in the experiment?
-Squaring the terminal velocity data is a technique to linearize the relationship between terminal velocity and mass, making it easier to graph and analyze the data to find a linear trend.
How does the graph of terminal velocity squared versus mass help in determining the constant C?
-The graph of terminal velocity squared versus mass is a linear representation of the relationship. The slope of the best-fit line on this graph represents the constant C, which is a coefficient in the drag force equation.
What is the method to find the approximate distance the filters fell using the velocity-time graph?
-The approximate distance the filters fell can be found by calculating the area under the velocity-time curve. This area represents the displacement of the filters as they fall.
How is the mechanical energy dissipated due to air resistance calculated in the experiment?
-The mechanical energy dissipated due to air resistance is calculated by finding the difference between the initial and final potential and kinetic energies. The work done against the drag force, which is not recovered, represents the energy dissipated.
What are some key graphing skills emphasized in the video?
-Key graphing skills emphasized include labeling axes with units, scaling the graph to use the entire available space effectively, plotting data points accurately, and drawing the best-fit line to analyze the linear relationship.
Outlines
📚 Physics Lab on Terminal Velocity
Dr. Julie Hood introduces an experimental lab-based problem involving the terminal speed of falling paper coffee filters and their mass. The video aims to guide viewers through understanding the relationship between terminal velocity and mass, considering the effects of air resistance. It emphasizes the importance of forces in equilibrium and the process of creating a linear relationship from nonlinear data. The task involves graphing this relationship to determine a constant and representing the velocity of a falling body with air resistance considered. The video also covers how to find distance using the plotted graph and determining the mechanical energy dissipated due to air resistance during the fall. The concept of drag force proportional to the square of velocity is introduced, and the method of free body diagrams is highlighted as essential for solving physics problems.
📉 Linearization of Nonlinear Data
This section of the script focuses on the technique of linearization to transform the nonlinear relationship between terminal velocity and mass into a linear graph. Dr. Hood explains the process of squaring the terminal velocity data to linearize it and then plotting it against mass. She provides guidance on graphing, including scaling the axes and ensuring the graph is well-labeled with units. The objective is to find the slope of the best-fit line to determine the constant 'C' from the drag force equation. Additionally, she discusses sketching the velocity-time graph for a stack of filters approaching terminal velocity and emphasizes the importance of graphing skills and understanding the linearization process.
🔄 Energy Dissipation Due to Air Resistance
The final part of the script discusses the application of conservation of energy to determine the mechanical energy dissipated due to air resistance as the stack of filters falls. It explains how to calculate the approximate distance fallen using the area under the velocity-time graph and how to express the energy lost due to drag force. The script wraps up by reviewing the key concepts covered, including linearization, energy conservation, and calculus, and stresses the importance of graphing skills such as labeling axes, scaling, and using the entire graph effectively. Dr. Hood encourages further practice on these topics and ends the video with a reminder to aim for excellence.
Mindmap
Keywords
💡Terminal Speed
💡Air Resistance
💡Free Body Diagram
💡Equilibrium
💡Linear Relationship
💡Graphing
💡Mechanical Energy
💡Conservation of Energy
💡Integral
💡Linearization
💡Drag Force
Highlights
Introduction to an experimental lab-based free response question involving resistive force.
Investigation of the relationship between terminal speed and mass of falling paper coffee filters.
Demonstration of understanding forces in equilibrium and creating a linear relationship from nonlinear data.
Graphical representation of the velocity of a falling body considering air resistance.
Explanation of how to find distance using a plotted graph.
Determination of mechanical energy dissipated due to air resistance during the fall.
Assumption that drag force is proportional to the square of speed.
Use of free body diagrams to solve physics problems.
Derivation of an expression relating terminal speed to mass.
Linearization technique to transform a nonlinear relationship into a linear graph.
Squaring of terminal velocity data to create a linear graph.
Scaling of data and graph for better visualization and analysis.
Calculation of the coefficient C from the slope of the linear graph.
Sketching an approximate graph of speed versus time for a falling stack of filters.
Finding displacement from a velocity-time graph by calculating the area under the curve.
Application of conservation of energy to determine energy dissipation due to air resistance.
Practical advice on graphing skills, labeling axes, and scaling for effective data representation.
Encouragement to practice linearization and graphing for better understanding of physics concepts.
Transcripts
Browse More Related Video

2021 Live Review 8 | AP Physics C: Mechanics | Understanding Resistive Forces
3 | FRQ | Practice Sessions | AP Physics C: Mechanics
Free-Body Diagrams
2 | FRQ | Practice Sessions | AP Physics C: Mechanics
Force | Free Body Diagrams | Physics | Don't Memorise
1 | MCQ | Practice Sessions | AP Physics C: Mechanics
5.0 / 5 (0 votes)
Thanks for rating: